Difference between revisions of "Aufgaben:Exercise 2.13: Quadrature Amplitude Modulation"
m |
|||
| (One intermediate revision by one other user not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Modulation Methods/Further AM Variants |
}} | }} | ||
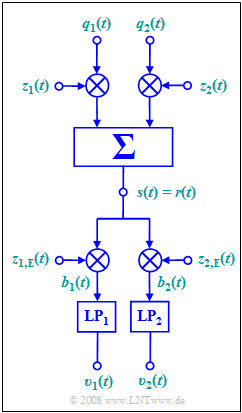
| − | [[File: | + | [[File:EN_Mod_A_2_11.png|right|frame|QAM model under consideration]] |
The "quadrature amplitude modulation" (QAM) explained by the diagram allows the transmission of two source signals q1(t) and q2(t) over the same channel | The "quadrature amplitude modulation" (QAM) explained by the diagram allows the transmission of two source signals q1(t) and q2(t) over the same channel | ||
*under certain boundary conditions, | *under certain boundary conditions, | ||
Latest revision as of 16:27, 9 April 2022
The "quadrature amplitude modulation" (QAM) explained by the diagram allows the transmission of two source signals q1(t) and q2(t) over the same channel
- under certain boundary conditions,
- which are to be determined in this exercise.
In this exercise, with A1=A2=2 V:
- q1(t)=A1⋅cos(2π⋅f1⋅t),
- q2(t)=A2⋅sin(2π⋅f2⋅t).
For ω_{\rm T} = 2π · 25\ \rm kHz, the four carrier signals shown in the diagram are:
- z_1(t) = \cos(\omega_{\rm T} \cdot t),
- z_2(t) = \sin(\omega_{\rm T} \cdot t),
- z_{1,\hspace{0.05cm}{\rm E}}(t) = 2 \cdot \cos(\omega_{\rm T} \cdot t + \Delta \phi_{\rm T}),
- z_{2,\hspace{0.05cm}{\rm E}}(t) = 2 \cdot \sin(\omega_{\rm T} \cdot t + \Delta \phi_{\rm T})\hspace{0.05cm}.
Both lowpass filters \rm LP_1 and \rm LP_2 with input signals b_1(t) resp. b_2(t) , remove all frequency components |f| > f_{\rm T}.
Hints:
- This exercise belongs to the chapter Further AM Variants.
- Particular reference is made to the page Quadrature Amplitude Modulation (QAM).
- It is worth noting that the carrier signals z_2(t) and z_{2,\hspace{0.05cm}{\rm E}}(t) are applied with positive signs here.
- Often – as in the theory section – these carrier signals are given as "minus-sine".
- The following trigonometric transformations are given:
- \cos(\alpha) \cdot \cos(\beta) = 1/2 \cdot \big[ \cos(\alpha - \beta)+ \cos(\alpha + \beta) \big],
- \sin(\alpha) \cdot \sin(\beta) = 1/2 \cdot \big[ \cos(\alpha - \beta)- \cos(\alpha + \beta) \big],
- \sin(\alpha) \cdot \cos(\beta) = 1/2 \cdot \big[ \sin(\alpha - \beta)+ \sin(\alpha + \beta) \big] \hspace{0.05cm}.
Questions
Solution
- s(t) = A_1 \cdot \cos(\omega_{\rm 1} \cdot t)\cdot \cos(\omega_{\rm T} \cdot t) + A_2 \cdot \sin(\omega_{\rm 2} \cdot t)\cdot \sin(\omega_{\rm T} \cdot t)
- \Rightarrow \hspace{0.3cm}s(t) = \frac{A_1}{2}\cdot \cos((\omega_{\rm T} - \omega_{\rm 1})\cdot t) + \frac{A_1}{2}\cdot \cos((\omega_{\rm T} + \omega_{\rm 1})\cdot t) + \frac{A_2}{2}\cdot \cos((\omega_{\rm T} - \omega_{\rm 2})\cdot t) - \frac{A_2}{2}\cdot \cos((\omega_{\rm T} + \omega_{\rm 2})\cdot t)\hspace{0.05cm}.
- The second answer is correct.
(2) With A_1 = A_2 = 2 \ \rm V and f_1 = f_2 = 5\ \rm kHz, the first and third cosine oscillations constructively overlap and the other two cancel completely.
- Thus, the following simple result is obtained:
- s(t) = 2\,{\rm V} \cdot \cos(2 \pi \cdot 20\,{\rm kHz} \cdot t) \hspace{0.3cm}\Rightarrow \hspace{0.3cm} s(t = 50\,{\rm µ s}) \hspace{0.15cm}\underline {= 2\,{\rm V}} \hspace{0.05cm}.
(3) The first answer is correct:
- For phase-synchronous demodulation (Δϕ_T = 0), the signals before the low-pass filters according to subtask (2) are obtained as:
- b_1(t) = 2\,{\rm V} \cdot \cos(\omega_{\rm 20} \cdot t)\cdot 2 \cdot \cos(\omega_{\rm 25} \cdot t) = 2\,{\rm V} \cdot \cos(\omega_{\rm 5} \cdot t) + 2\,{\rm V} \cdot \cos(\omega_{\rm 45} \cdot t),
- b_2(t) = 2\,{\rm V} \cdot \cos(\omega_{\rm 20} \cdot t)\cdot 2 \cdot \sin(\omega_{\rm 25} \cdot t) = 2\,{\rm V} \cdot \sin(\omega_{\rm 5} \cdot t) + 2\,{\rm V} \cdot \sin(\omega_{\rm 45} \cdot t)\hspace{0.05cm}.
- Thus, after eliminating the respective 45\ \rm kHz components, we get v_1(t) = q_1(t) and v_2(t) = q_2(t).
(4) Analogously to subtask (3) it now holds that:
- b_1(t) = 2\,{\rm V} \cdot \cos(\omega_{\rm 20} \cdot t)\cdot 2 \cdot \cos(\omega_{\rm 25} \cdot t+ \Delta \phi_{\rm T})= 2\,{\rm V} \cdot \cos(\omega_{\rm 5} \cdot t + \Delta \phi_{\rm T}) + {(45 \,\rm kHz-Anteil )},
- b_2(t)= 2\,{\rm V} \cdot \cos(\omega_{\rm 20} \cdot t)\cdot 2 \cdot \sin(\omega_{\rm 25} \cdot t+ \Delta \phi_{\rm T})= 2\,{\rm V} \cdot \sin(\omega_{\rm 5} \cdot t + \Delta \phi_{\rm T}) + {(45 \,\rm kHz-Anteil )}\hspace{0.05cm}.
- The sink signals v_1(t) and v_2(t) in this constellation exhibit delays and thus phase distortions compared with q_1(t) and q_2(t).
- These belong to the class of linear distortions ⇒ Answer 2.
(5) In general, it holds for the received signal:
- r(t) = s(t) = q_1(t) \cdot \cos(\omega_{\rm T} \cdot t) + q_2(t) \cdot \sin(\omega_{\rm T} \cdot t) \hspace{0.05cm}.
Multiplication by the receiver-side carrier signals z_{1,\hspace{0.05cm}{\rm E}}(t) and z_{2,\hspace{0.05cm}{\rm E}}(t) and band-limiting leads to the signals
- v_1(t) = \cos(\Delta \phi_{\rm T}) \cdot q_1(t) - \sin(\Delta \phi_{\rm T}) \cdot q_2(t),
- v_2(t) = \sin(\Delta \phi_{\rm T}) \cdot q_1(t) + \cos(\Delta \phi_{\rm T}) \cdot q_2(t) \hspace{0.05cm}.
From this it can be seen:
- With a phase offset of Δϕ_{\rm T} = 30^\circ, the sink signal v_1(t) includes not only the signal q_1(t) attenuated by about \cos(30^\circ) = 0.866,
but also the frequency f_2 is contained in q_2(t). - This is weighted by the factor \sin(30^\circ) = 0.5.
- Thus, nonlinear distortions are present ⇒ Answer 3.