Difference between revisions of "Aufgaben:Exercise 1.6Z: Interpretation of the Frequency Response"
| (50 intermediate revisions by 8 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Linear_and_Time_Invariant_Systems/Some_Low-Pass_Functions_in_Systems_Theory}} |
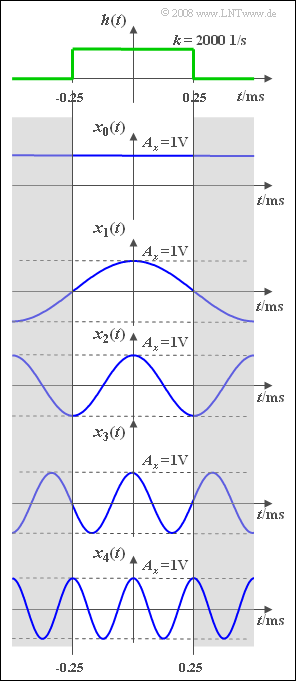
| − | [[File:P_ID862__LZI_Z_1_6.png|right| | + | [[File:P_ID862__LZI_Z_1_6.png|right|frame|Impulse response and input signals]] |
| − | xi(t)=Ax⋅cos(2πfit) | + | The task is meant to investigate the influence of a low-pass filter H(f) on cosinusoidal signals of the form |
| − | + | :$$x_i(t) = A_x \cdot {\rm cos}(2\pi f_i t ).$$ | |
| + | In the graph you can see the signals xi(t) where the index i indicates the frequency in $\rm kHz$ . So, x2(t) describes a $2 \hspace{0.09cm} \rm kHz$–signal. | ||
| − | + | The signal amplitude in each case is $A_x = 1 \hspace{0.05cm} \rm V$. The direct (DC) signal x0(t) is to be interpreted as a limiting case of a cosine signal with frequeny $f_0 =0$. | |
| − | + | The upper sketch shows the rectangular impulse response h(t) of the low-pass filter. Its frequency response is: | |
| − | $$H(f) = {\rm si}(\pi | + | :$$H(f) = {\rm si}(\pi {f}/{ {\rm \Delta}f}) .$$ |
| − | + | Due to linearity and the fact that H(f) is real and even the output signals are also cosine-shaped: | |
| − | yi(t)=Ai⋅cos(2πfit). | + | :yi(t)=Ai⋅cos(2πfit). |
| − | + | *The signal amplitudes Ai at the output for different frequencies fi are searched-for and the solution is to be found in the time domain only. | |
| − | + | *This somewhat circuitous solution is intended to make the basic relationship between the time and frequency domains clear. | |
| − | === | + | |
| + | |||
| + | |||
| + | |||
| + | |||
| + | ''Please note:'' | ||
| + | *The exercise belongs to the chapter [[Linear_and_Time_Invariant_Systems/Some_Low-Pass_Functions_in_Systems_Theory|Some Low-Pass Functions in Systems Theory]]. | ||
| + | *Contrary to the usual definition of the amplitude, the "A_i" may well be negative. Then, this corresponds to the function "minus-cosine". | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which low-pass filter is at hand here? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - Ideal low-pass filter, |
| − | + | + | + slit low-pass filter, |
| − | - | + | - Gaussian low-pass filter. |
| − | { | + | {State the equivalent bandwidth of H(f) . |
|type="{}"} | |type="{}"} | ||
| − | \Delta f = { 2 } kHz | + | $\Delta f \ =\ $ { 2 3% } $\ \rm kHz$ |
| − | { | + | {In general, compute the amplitude A_i as a function of x_i(t) and h(t). Which of the following should be considered in the calculations? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + For the cosine signal, A_i = y_i(t = 0) holds. |
| − | - | + | - The following holds: y_i(t) = x_i(t) · h(t). |
| − | + | + | + The following holds: y_i(t) = x_i(t) ∗ h(t). |
| − | { | + | {Which of the following results are true for A_0, A_2 and A_4 ? The following still holds: A_i = y_i(t = 0). |
|type="[]"} | |type="[]"} | ||
| − | - A_0 = | + | - $A_0 = 0$. |
| − | + $A_0 = | + | + $A_0 = 1 \hspace{0.05cm} \rm V $. |
| − | + A_2 = | + | + $A_2 = 0$. |
| − | - $A_2 = | + | - $A_2 = 1 \hspace{0.05cm} \rm V $. |
| − | + A_4 = | + | + $A_4 = 0$. |
| − | - $A_4 = | + | - $A_4 =1 \hspace{0.05cm} \rm V $. |
| − | { | + | {Compute the amplitudes A_1 and A_3 for a $1 \ \rm kHz– and 3 \ \rm kHz$–signal. <br>Interpret the results using the spectral functions. |
|type="{}"} | |type="{}"} | ||
| − | A_1 = { 0.637 5% } V | + | $A_1 \ = \ $ { 0.637 5% } $\ \rm V$ |
| − | A_3 = {-0.215- -0.205 | + | $A_3 \ = \ $ { -0.215--0.205 } $\ \rm V$ |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | + | '''(1)''' <u>Approach 2</u> is correct: It is a <u>slit low-pass filter</u>. | |
| − | + | ||
| − | :''' | + | |
| − | : | + | '''(2)''' The (equivalent) time duration of the impulse response is Δt = 0.5 \ \rm ms. The equivalent bandwidth is equal to the reciprocal: |
| − | :''' | + | :Δf = 1/Δt \ \rm \underline{= \ 2 \ kHz}. |
| − | :''' | + | |
| − | + | ||
| + | '''(3)''' <u>Approaches 1 and 3</u> are correct: | ||
| + | *The amplitude is A_i = y_i(t = 0) since y_i(t) is cosine-shaped. The output signal is calculated by convolution for this purpose: | ||
| + | :$$A_i = y_i (t=0) = \int\limits_{ - \infty }^{ + \infty } {x_i ( \tau )} \cdot h ( {0 - \tau } ) \hspace{0.1cm}{\rm d}\tau.$$ | ||
| + | *Considering the symmetry and the time limitation of $h(t)$ the following result is obtained: | ||
| + | :A_i = \frac{A_x}{\Delta t} \cdot \int\limits_{ - \Delta t /2 }^{ + \Delta t /2 } {\rm cos}(2\pi f_i \tau )\hspace{0.1cm}{\rm d}\tau. | ||
| + | |||
| + | |||
| + | |||
| + | '''(4)''' <u>Approaches 2, 3 and 5</u> are correct: | ||
| + | *For the direct (DC) signal x_0(t) = A_x , set f_i = 0 and one obtains A_0 = A_x \ \rm \underline{ = \ 1 \hspace{0.05cm} V}. | ||
| + | *In contrast to this, for the cosine frequencies f_2 = 2 \ \rm kHz and f_4 = 4 \ \rm kHz the integral vanishes in each case because then it is integrated over one and two periods, respectively: A_2 \ \rm \underline{ = \hspace{0.05cm} 0} und A_4 \hspace{0.05cm} \rm \underline{ = \ 0}. | ||
| + | *In the frequency domain, the cases which are dealt with here correspond to: | ||
| + | :H(f=0) = 1, \hspace{0.3cm}H(f=\Delta f) = 0, \hspace{0.3cm}H(f=2\Delta f) = 0. | ||
| + | |||
| + | |||
| + | |||
| + | '''(5)''' The result of subtask '''(3)''' – considering the symmetry – is for f_i = f_1: | ||
| + | :$$A_1= \frac{2A_x}{\Delta t} \cdot \int\limits_{ 0 }^{ \Delta t /2 } {\rm cos}(2\pi f_1 \tau )\hspace{0.1cm}{\rm | ||
| + | d}\tau = \frac{2A_x}{2\pi f_1 \cdot \Delta t} \cdot {\rm sin}(2\pi f_1 \frac{\Delta t}{2} | ||
| + | )= A_x \cdot {\rm si}(\pi f_1 \Delta t ).$$ | ||
| + | *Taking f_1 · Δt = 0.5 into account the result is: | ||
| + | :A_1 = A_x \cdot {\rm si}(\frac{\pi}{2} ) = \frac{2A_x}{\pi} \hspace{0.15cm}\underline{= 0.637\,{\rm V}}. | ||
| + | *Correspondingly, the following is obtained using f_3 · Δt = 1.5: | ||
| + | :A_3 = A_x \cdot {\rm si}({3\pi}/{2} ) = -\frac{2A_x}{3\pi} = -{A_1}/{3}\hspace{0.15cm}\underline{= -0.212\,{\rm V}}. | ||
| + | *The exact same results are obtained – but much faster – by applying the equation: | ||
| + | :A_i = A_x · H(f = f_i). | ||
| + | |||
| + | *From the graphs on the information page it is already obvious that the integral over x_1(t) is positive in the marked area and the integral over x_3(t) is negative. | ||
| + | *However, it should be noted that in general amplitude is usually referred to as the magnitude (See notice on the information page). | ||
| + | |||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category: | + | [[Category:Linear and Time-Invariant Systems: Exercises|^1.3 Some Low-Pass Functions in Systems Theory^]] |
Latest revision as of 19:33, 7 September 2021
The task is meant to investigate the influence of a low-pass filter H(f) on cosinusoidal signals of the form
- x_i(t) = A_x \cdot {\rm cos}(2\pi f_i t ).
In the graph you can see the signals x_i(t) where the index i indicates the frequency in \rm kHz . So, x_2(t) describes a 2 \hspace{0.09cm} \rm kHz–signal.
The signal amplitude in each case is A_x = 1 \hspace{0.05cm} \rm V. The direct (DC) signal x_0(t) is to be interpreted as a limiting case of a cosine signal with frequeny f_0 =0.
The upper sketch shows the rectangular impulse response h(t) of the low-pass filter. Its frequency response is:
- H(f) = {\rm si}(\pi {f}/{ {\rm \Delta}f}) .
Due to linearity and the fact that H(f) is real and even the output signals are also cosine-shaped:
- y_i(t) = A_i \cdot {\rm cos}(2\pi f_i t ) .
- The signal amplitudes A_i at the output for different frequencies f_i are searched-for and the solution is to be found in the time domain only.
- This somewhat circuitous solution is intended to make the basic relationship between the time and frequency domains clear.
Please note:
- The exercise belongs to the chapter Some Low-Pass Functions in Systems Theory.
- Contrary to the usual definition of the amplitude, the "A_i" may well be negative. Then, this corresponds to the function "minus-cosine".
Questions
Solution
(2) The (equivalent) time duration of the impulse response is Δt = 0.5 \ \rm ms. The equivalent bandwidth is equal to the reciprocal:
- Δf = 1/Δt \ \rm \underline{= \ 2 \ kHz}.
(3) Approaches 1 and 3 are correct:
- The amplitude is A_i = y_i(t = 0) since y_i(t) is cosine-shaped. The output signal is calculated by convolution for this purpose:
- A_i = y_i (t=0) = \int\limits_{ - \infty }^{ + \infty } {x_i ( \tau )} \cdot h ( {0 - \tau } ) \hspace{0.1cm}{\rm d}\tau.
- Considering the symmetry and the time limitation of h(t) the following result is obtained:
- A_i = \frac{A_x}{\Delta t} \cdot \int\limits_{ - \Delta t /2 }^{ + \Delta t /2 } {\rm cos}(2\pi f_i \tau )\hspace{0.1cm}{\rm d}\tau.
(4) Approaches 2, 3 and 5 are correct:
- For the direct (DC) signal x_0(t) = A_x , set f_i = 0 and one obtains A_0 = A_x \ \rm \underline{ = \ 1 \hspace{0.05cm} V}.
- In contrast to this, for the cosine frequencies f_2 = 2 \ \rm kHz and f_4 = 4 \ \rm kHz the integral vanishes in each case because then it is integrated over one and two periods, respectively: A_2 \ \rm \underline{ = \hspace{0.05cm} 0} und A_4 \hspace{0.05cm} \rm \underline{ = \ 0}.
- In the frequency domain, the cases which are dealt with here correspond to:
- H(f=0) = 1, \hspace{0.3cm}H(f=\Delta f) = 0, \hspace{0.3cm}H(f=2\Delta f) = 0.
(5) The result of subtask (3) – considering the symmetry – is for f_i = f_1:
- A_1= \frac{2A_x}{\Delta t} \cdot \int\limits_{ 0 }^{ \Delta t /2 } {\rm cos}(2\pi f_1 \tau )\hspace{0.1cm}{\rm d}\tau = \frac{2A_x}{2\pi f_1 \cdot \Delta t} \cdot {\rm sin}(2\pi f_1 \frac{\Delta t}{2} )= A_x \cdot {\rm si}(\pi f_1 \Delta t ).
- Taking f_1 · Δt = 0.5 into account the result is:
- A_1 = A_x \cdot {\rm si}(\frac{\pi}{2} ) = \frac{2A_x}{\pi} \hspace{0.15cm}\underline{= 0.637\,{\rm V}}.
- Correspondingly, the following is obtained using f_3 · Δt = 1.5:
- A_3 = A_x \cdot {\rm si}({3\pi}/{2} ) = -\frac{2A_x}{3\pi} = -{A_1}/{3}\hspace{0.15cm}\underline{= -0.212\,{\rm V}}.
- The exact same results are obtained – but much faster – by applying the equation:
- A_i = A_x · H(f = f_i).
- From the graphs on the information page it is already obvious that the integral over x_1(t) is positive in the marked area and the integral over x_3(t) is negative.
- However, it should be noted that in general amplitude is usually referred to as the magnitude (See notice on the information page).