Difference between revisions of "Aufgaben:Exercise 1.2: Entropy of Ternary Sources"
m (Text replacement - "autocorrelation" to "auto-correlation") |
|||
| (26 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Information_Theory/Discrete_Memoryless_Sources}} |
| − | }} | ||
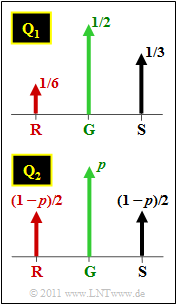
| − | [[File: | + | [[File:Inf_A_1_2_vers2.png|right|frame|Probabilities of two ternary sources]] |
| − | + | The entropy of a discrete memoryless source with $M$ possible symbols is: | |
:$$H = \sum_{\mu = 1}^M p_{\mu} \cdot {\rm log}_2\hspace{0.1cm}\frac{1}{p_\mu}\hspace{0.05cm},\hspace{0.3cm} | :$$H = \sum_{\mu = 1}^M p_{\mu} \cdot {\rm log}_2\hspace{0.1cm}\frac{1}{p_\mu}\hspace{0.05cm},\hspace{0.3cm} | ||
| − | {\rm | + | {\rm pseudo unit\hspace{-0.15cm}: \hspace{0.15cm}bit}\hspace{0.05cm}.$$ |
| − | + | Here, the $p_\mu$ denote the occurrence probabilities of the individual symbols or events. In the present example, the events are denoted by $\rm R$(ed), $\rm G$(reen) and $\rm S$(chwarz) with "Schwarz" being the German word for "Black". | |
| − | + | *For a binary source with the occurrence probabilities $p and 1-p$ this can be written: | |
:$$H = H_{\rm bin}(p) = p \cdot {\rm log}_2\hspace{0.1cm}\frac{1}{p}+ (1-p) \cdot | :$$H = H_{\rm bin}(p) = p \cdot {\rm log}_2\hspace{0.1cm}\frac{1}{p}+ (1-p) \cdot | ||
| − | {\rm log}_2\hspace{0.1cm}\frac{1}{1-p}\hspace{0.05cm}.$$ | + | {\rm log}_2\hspace{0.1cm}\frac{1}{1-p}\hspace{0.05cm},\hspace{0.3cm} |
| − | + | \text{pseudo–unit: bit}\hspace{0.05cm}.$$ | |
| + | *The entropy of a multilevel source can often be expressed with this "binary entropy function" $H_{\rm bin}(p)$. | ||
| + | |||
| − | + | In this task, two ternary sources with the symbol probabilities according to the above graph are considered: | |
| − | + | # source Q1 with $p_{\rm G }= 1/2$, $p_{\rm S }= 1/3 and p_{\rm R }= 1/6$, | |
| + | # source Q2 with $p_{\rm G }= p and p_{\rm S } = p_{\rm R } = (1-p)/2$. | ||
| − | |||
| − | + | *The ternary source Q2 can also be applied to "Roulette" when a player bets only on the squares $\rm R$(ed), $\rm S$(chwarz) and $\rm G$(reen) (the "zero"). This type of game is referred to as $\text{Roulette 1}$ in the question section. | |
| − | + | *In contrast, $\text{Roulette 2}$ indicates that the player bets on single numbers $(0$, ... , $36)$. | |
| − | |||
| − | === | + | |
| + | |||
| + | |||
| + | |||
| + | ''Hint:'' | ||
| + | *The task belongs to the chapter [[Information_Theory/Gedächtnislose_Nachrichtenquellen|Discrete Memoryless Sources]]. | ||
| + | |||
| + | |||
| + | |||
| + | ===Questions=== | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {What is the entropy $H of the source \rm \underline{Q_1}$? |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $H \ = \ { 1.46 3% }\ \rm bit$ |
| − | { | + | {Which of the following statements are true if $\rm R$, $\rm G$ and $\rm S$ are represented by the numerical values $-1$, $0 and +1$ ? |
| − | |type=" | + | |type="()"} |
| − | - | + | - The result is a smaller entropy. |
| − | + | + | + The entropy remains the same. |
| − | - | + | - The result is a greater entropy. |
| − | { | + | {Determine the entropy of the source Q2_ using the binary entropy function $H_{\rm bin}(p)$. What value results for $\underline{p = 0.5}$? |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $H \ = \ { 1.5 3% }\ \rm bit$ |
| − | { | + | {For which $p$–value of the source Q2_ does the maximum entropy result: H → H_\text{max}? |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $p \ = \ $ { 0.333 3% } |
| − | { | + | {What is the entropy of the source model $\text{Roulette 1}$, i.e. with respect to the events $\rm R$(ed), $\rm S$(chwarz) and $\rm G$(reen) (the "zero")? |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $H \ = \ { 1.152 3% }\ \rm bit$ |
| − | { | + | {What is the entropy of $\text{Roulette 2}$ , i.e. with regard to the numbers $0$, ... , $36$? |
|type="{}"} | |type="{}"} | ||
| − | $ | + | $H \ = \ { 5.209 3% }\ \rm bit$ |
| Line 65: | Line 74: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | + | '''(1)''' With the "symbol" probabilities $1/2$, $1/3 and 1/6$ we get the following entropy value: | |
| − | :$$H \hspace{0.1cm} = \hspace{0.1cm} 1/2 \cdot {\rm log}_2\hspace{0.1cm}(2) +1/3 \cdot {\rm log}_2\hspace{0.1cm}(3) +1/6 \cdot {\rm log}_2\hspace{0.1cm}(6) = | + | :$$H \hspace{0.1cm} = \hspace{0.1cm} 1/2 \cdot {\rm log}_2\hspace{0.1cm}(2) +1/3 \cdot {\rm log}_2\hspace{0.1cm}(3) +1/6 \cdot {\rm log}_2\hspace{0.1cm}(6) =(1/2 + 1/6)\cdot {\rm log}_2\hspace{0.1cm}(2) + (1/3 + 1/6)\cdot {\rm log}_2\hspace{0.1cm}(3) \hspace{0.15cm}\underline {\approx 1.46 \, {\rm bit}} \hspace{0.05cm}.$$ |
| − | + | ||
| − | + | ||
| − | + | ||
| + | '''(2)''' <u>Proposed solution 2</u> is correct: | ||
| + | *The entropy depends only on the probabilities of occurrence. | ||
| + | *It does not matter which numerical values or physical quantities one assigns to the individual symbols. | ||
| + | *It is different with mean values or the ACF (auto correlation function) calculation. If only symbols are given, no moments can be calculated for them. | ||
| + | *Moreover, the mean values, auto-correlation, etc. depend on whether one agrees on the assignment bipolar (−1,0,+1) or unipolar (0,1,2) . | ||
| + | |||
| + | |||
| + | |||
| + | '''(3)''' The entropy of source $\rm Q_2$ can be expressed as follows: | ||
| + | :$$H \hspace{0.1cm} = \hspace{0.1cm} p \cdot {\rm log}_2\hspace{0.1cm}\frac {1}{p}+ 2 \cdot \frac{1-p}{2} \cdot {\rm log}_2\hspace{0.1cm}\frac {2}{1-p}= p \cdot {\rm log}_2\hspace{0.1cm}\frac {1}{p}+ (1-p) \cdot {\rm log}_2\hspace{0.1cm}\frac {1}{1-p} + (1-p)\cdot {\rm log}_2\hspace{0.1cm}(2)= H_{\rm bin}(p) + 1-p \hspace{0.05cm}.$$ | ||
| + | *For p=0.5 ⇒ Hbin(p)=1 , we get H=1.5bit_. | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | '''(4)''' The maximum entropy of a memoryless source with symbol set size $M is obtained when all M$ symbols are equally probable. | |
| + | *For the special case $M=3$ it follows: | ||
:$$p_{\rm R} + p_{\rm G} + p_{\rm S} = 1 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | :$$p_{\rm R} + p_{\rm G} + p_{\rm S} = 1 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} | ||
| − | \underline {p = 1/3}\hspace{0.05cm}.$$ | + | \underline {p = 1/3 \approx 0.333}\hspace{0.05cm}.$$ |
| − | + | *Thus, using the result of sub-task '''(3)''' , we obtain the following entropy: | |
| − | :$$H | + | :$$H = H_{\rm bin}(1/3) + 1-1/3 = 1/3 \cdot |
| − | {\rm log}_2\hspace{0.1cm}(3) + 2/3 \cdot {\rm log}_2\hspace{0.1cm}(3/2) + 2/3 | + | {\rm log}_2\hspace{0.1cm}(3) + 2/3 \cdot {\rm log}_2\hspace{0.1cm}(3/2) + 2/3 $$ |
| − | + | :$$\Rightarrow \hspace{0.3cm}H = 1/3 \cdot {\rm log}_2\hspace{0.1cm}(3) + 2/3 \cdot | |
{\rm log}_2\hspace{0.1cm}(3) - 2/3 \cdot {\rm log}_2\hspace{0.1cm}(2)+ 2/3 = | {\rm log}_2\hspace{0.1cm}(3) - 2/3 \cdot {\rm log}_2\hspace{0.1cm}(2)+ 2/3 = | ||
{\rm log}_2\hspace{0.1cm}(3) = {1.585 \, {\rm bit}} | {\rm log}_2\hspace{0.1cm}(3) = {1.585 \, {\rm bit}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | ||
| + | |||
| + | '''(5)''' The source model Roulette 1 is information theoretically equal to the configuration Q2 with $p = 1/37$: | ||
:pG=p=137,pR=pS=1−p2=1837. | :pG=p=137,pR=pS=1−p2=1837. | ||
| − | + | *Thus, using the result of subtask '''(3)''', we obtain: | |
| − | :$$H | + | :$$H = H_{\rm bin}(1/37) + \frac{36}{37} = \frac{1}{37} \cdot {\rm log}_2\hspace{0.1cm}(37) + \frac{36}{37} \cdot {\rm log}_2\hspace{0.1cm}(37) - \frac{36}{37} \cdot {\rm log}_2\hspace{0.1cm}36 + \frac{36}{37} = |
| − | + | {\rm log}_2\hspace{0.1cm}(37) + \frac{36}{37} \cdot ( 1- {\rm log}_2\hspace{0.1cm}(36)) = 5.209 - 4.057 \hspace{0.15cm} \underline { = 1.152 \, {\rm bit}} | |
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | ||
| + | |||
| + | '''(6)''' If we bet on single numbers in roulette ⇒ source model $\text{Roulette 2}$, all numbers from $0 to 36$ are equally probable and we get: | ||
:$$H = {\rm log}_2\hspace{0.1cm}(37) \hspace{0.15cm} \underline { = 5.209 \, {\rm bit}} | :$$H = {\rm log}_2\hspace{0.1cm}(37) \hspace{0.15cm} \underline { = 5.209 \, {\rm bit}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| Line 105: | Line 125: | ||
| − | [[Category: | + | [[Category:Information Theory: Exercises|^1.1 Memoryless Sources^]] |
Latest revision as of 13:29, 17 February 2022
The entropy of a discrete memoryless source with M possible symbols is:
- H=M∑μ=1pμ⋅log21pμ,pseudounit:bit.
Here, the pμ denote the occurrence probabilities of the individual symbols or events. In the present example, the events are denoted by R(ed), G(reen) and S(chwarz) with "Schwarz" being the German word for "Black".
- For a binary source with the occurrence probabilities p and 1−p this can be written:
- H=Hbin(p)=p⋅log21p+(1−p)⋅log211−p,pseudo–unit: bit.
- The entropy of a multilevel source can often be expressed with this "binary entropy function" Hbin(p).
In this task, two ternary sources with the symbol probabilities according to the above graph are considered:
- source Q1 with pG=1/2, pS=1/3 and pR=1/6,
- source Q2 with pG=p and pS=pR=(1−p)/2.
- The ternary source Q2 can also be applied to "Roulette" when a player bets only on the squares R(ed), S(chwarz) and G(reen) (the "zero"). This type of game is referred to as Roulette 1 in the question section.
- In contrast, Roulette 2 indicates that the player bets on single numbers (0, ... , 36).
Hint:
- The task belongs to the chapter Discrete Memoryless Sources.
Questions
Solution
- H=1/2⋅log2(2)+1/3⋅log2(3)+1/6⋅log2(6)=(1/2+1/6)⋅log2(2)+(1/3+1/6)⋅log2(3)≈1.46bit_.
(2) Proposed solution 2 is correct:
- The entropy depends only on the probabilities of occurrence.
- It does not matter which numerical values or physical quantities one assigns to the individual symbols.
- It is different with mean values or the ACF (auto correlation function) calculation. If only symbols are given, no moments can be calculated for them.
- Moreover, the mean values, auto-correlation, etc. depend on whether one agrees on the assignment bipolar (−1,0,+1) or unipolar (0,1,2) .
(3) The entropy of source Q2 can be expressed as follows:
- H=p⋅log21p+2⋅1−p2⋅log221−p=p⋅log21p+(1−p)⋅log211−p+(1−p)⋅log2(2)=Hbin(p)+1−p.
- For p=0.5 ⇒ Hbin(p)=1 , we get H=1.5bit_.
(4) The maximum entropy of a memoryless source with symbol set size M is obtained when all M symbols are equally probable.
- For the special case M=3 it follows:
- pR+pG+pS=1⇒p=1/3≈0.333_.
- Thus, using the result of sub-task (3) , we obtain the following entropy:
- H=Hbin(1/3)+1−1/3=1/3⋅log2(3)+2/3⋅log2(3/2)+2/3
- ⇒H=1/3⋅log2(3)+2/3⋅log2(3)−2/3⋅log2(2)+2/3=log2(3)=1.585bit.
(5) The source model Roulette 1 is information theoretically equal to the configuration Q2 with p=1/37:
- pG=p=137,pR=pS=1−p2=1837.
- Thus, using the result of subtask (3), we obtain:
- H=Hbin(1/37)+3637=137⋅log2(37)+3637⋅log2(37)−3637⋅log236+3637=log2(37)+3637⋅(1−log2(36))=5.209−4.057=1.152bit_.
(6) If we bet on single numbers in roulette ⇒ source model Roulette 2, all numbers from 0 to 36 are equally probable and we get:
- H=log2(37)=5.209bit_.