Difference between revisions of "Signal Representation/Analytical Signal and its Spectral Function"
| (58 intermediate revisions by 5 users not shown) | |||
| Line 1: | Line 1: | ||
{{Header | {{Header | ||
| − | |Untermenü= | + | |Untermenü=Band-Pass Signals |
| − | |Vorherige Seite= | + | |Vorherige Seite=Differences and Similarities of LP and BP Signals |
| − | |Nächste Seite= | + | |Nächste Seite=Equivalent Low-Pass Signal and Its Spectral Function |
}} | }} | ||
| − | ==Definition | + | ==Definition in the frequency domain== |
| + | <br> | ||
| + | We consider a real band-pass signal $x(t)$ with the corresponding band-pass spectrum $X(f)$, which has an even real and an odd imaginary part with respect to the frequency zero point. It is assumed that the carrier frequency $f_{\rm T}$ is much larger than the bandwidth of the band-pass signal $x(t)$. | ||
| − | + | {{BlaueBox|TEXT= | |
| + | $\text{Definition:}$ The »'''analytical signal'''« $x_+(t)$ belonging to the physical signal $x(t)$ is that time function, whose spectrum fulfills the following property: | ||
| + | [[File:EN_Sig_T_4_2_S1a.png|right|frame|Analytical signal in the frequency domain]] | ||
| + | :$$X_+(f)=\big[1+{\rm sign}(f)\big] \cdot X(f) = \left\{ {2 \cdot | ||
| + | X(f) \; \hspace{0.2cm}\rm for\hspace{0.2cm} {\it f} > 0, \atop {\,\,\,\, \rm 0 \; \hspace{0.9cm}\rm for\hspace{0.2cm} {\it f} < 0.} }\right.$$ | ||
| − | + | The »'''sign function'''« is equal to $+1$ for positive $f$–values and for negative $f$-values equal to $-1$. | |
| − | + | *The $($double sided$)$ limit value returns $\sign(0) = 0$. | |
| − | + | *The index "+" should make clear that $X_+(f)$ has only parts at positive frequencies. | |
| − | |||
| − | |||
| − | + | From the graphic you can see the calculation rule for $X_+(f)$: The actual band-pass spectrum $X(f)$ will | |
| + | *be doubled at the positive frequencies, and | ||
| + | *set to zero at the negative frequencies.}} | ||
| + | <br clear=all> | ||
| + | {{GraueBox|TEXT= | ||
| + | $\text{Example 1:}$ The graph | ||
| + | [[File:P_ID711__Sig_T_4_2_S1b_neu.png|right|frame|Spectrum $X(f)$ and Spectrum $X_{+}(f)$ of the analytical signal ]] | ||
| − | + | *on the left shows the $($discrete and complex$)$ spectrum $X(f)$ of the "physical band-pass signal" | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| + | :$$x(t) = 4\hspace{0.05cm}{\rm V} | ||
| + | \cdot {\cos} ( 2 \pi f_{\rm u} \hspace{0.03cm}t) + 6\hspace{0.05cm}{\rm V} | ||
| + | \cdot {\sin} ( 2 \pi f_{\rm o} \hspace{0.03cm}t),$$ | ||
| + | *on the right the $($also discrete and complex$)$ spectrum $X_{+}(f)$ of the corresponding "analytical signal" $x_{+}(t)$.}} | ||
| + | ==General calculation rule in the time domain== | ||
| + | <br> | ||
| + | Now we will take a closer look at the spectrum $X_+(f)$ of the analytical signal and divide it with respect to $f = 0$ into | ||
| + | [[File:Sig_T_4_2_S2a_Version2.png|right|frame|For a clear explanation of the analytical signal]] | ||
| + | *an even $($German: "gerade" ⇒ "$\rm g"$) part $X_{\rm +g}(f)$, and | ||
| − | {{ | + | *an odd $($German: "ungerade" ⇒ "$\rm u$") part $X_{\rm +u}(f)$: |
| + | :$$X_+(f) = X_{\rm +g}(f) + X_{\rm +u}(f).$$ | ||
| + | All these spectra are generally complex. | ||
| − | + | If one considers the [[Signal_Representation/Fourier_Transform_Theorems#Assignment_Theorem|»Assignment Theorem«]] of the Fourier transform, then the following statements are possible on basis of the graph: | |
| − | + | *The even part $X_{\rm +g}(f)$ of $X_{+}(f)$ leads after the Fourier transform to a real time signal, and the odd part $X_{\rm +u}(f)$ to an imaginary one. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | *It is obvious that $X_{\rm +g}(f)$ is equal to the physical Fourier spectrum $X(f)$ and thus the real part of $x_{\rm +g}(t)$ is equal to the given physical signal $x(t)$ with band-pass properties. | |
| − | |||
| − | + | *If we denote the imaginary part with $y(t)$, the analytical signal is: | |
| − | + | :$$x_+(t)= x(t) + {\rm j} \cdot y(t) .$$ | |
| − | + | *According to the generally valid laws of Fourier transform corresponding to the [[Signal_Representation/Fourier_Transform_Theorems#Assignment_Theorem|»Assignment Theorem«]], the following applies to the spectral function of the imaginary part: | |
| − | + | :$${\rm j} \cdot Y(f) = X_{\rm +u}(f)= {\rm sign}(f) \cdot X(f) | |
| − | |||
| − | |||
| − | |||
| − | * | ||
| − | |||
| − | |||
| − | $$x_+(t)= x(t) + {\rm j} \cdot y(t) .$$ | ||
| − | * | ||
| − | $${\rm j} \cdot Y(f) = X_{\rm +u}(f)= {\rm sign}(f) \cdot X(f) | ||
\hspace{0.3cm}\Rightarrow\hspace{0.3cm}Y(f) = \frac{{\rm | \hspace{0.3cm}\Rightarrow\hspace{0.3cm}Y(f) = \frac{{\rm | ||
sign}(f)}{ {\rm j}}\cdot X(f).$$ | sign}(f)}{ {\rm j}}\cdot X(f).$$ | ||
| − | * | + | *After transforming this equation into the time domain, the multiplication becomes the [[Signal_Representation/The_Convolution_Theorem_and_Operation|»convolution«]], and one gets: |
| − | $$y(t) = \frac{1}{ {\rm \pi} t} \hspace{0.05cm}\star | + | :$$y(t) = \frac{1}{ {\rm \pi} t} \hspace{0.05cm}\star |
\hspace{0.05cm}x(t) = \frac{1}{ {\rm \pi}} \cdot | \hspace{0.05cm}x(t) = \frac{1}{ {\rm \pi}} \cdot | ||
\hspace{0.03cm}\int_{-\infty}^{+\infty}\frac{x(\tau)}{ {t - | \hspace{0.03cm}\int_{-\infty}^{+\infty}\frac{x(\tau)}{ {t - | ||
\tau}}\hspace{0.15cm} {\rm d}\tau.$$ | \tau}}\hspace{0.15cm} {\rm d}\tau.$$ | ||
| + | ==Representation with Hilbert transform== | ||
| + | <br> | ||
| + | At this point it is necessary to briefly discuss a further spectral transformation, which is dealt thoroughly in the book [[Linear_and_Time_Invariant_Systems/Conclusions_from_the_Allocation_Theorem#Hilbert_transform|»Linear and Time-invariant Systems«]] . | ||
| + | {{BlaueBox|TEXT= | ||
| + | $\text{Definition:}$ For the »'''Hilbert transform'''« $ {\rm H}\left\{x(t)\right\}$ of a time function $x(t)$ applies: | ||
| + | |||
| + | :$$y(t) = {\rm H}\left\{x(t)\right\} = \frac{1}{ {\rm \pi} } \cdot | ||
| + | \hspace{0.03cm}\int_{-\infty}^{+\infty}\frac{x(\tau)}{ {t - | ||
| + | \tau} }\hspace{0.15cm} {\rm d}\tau.$$ | ||
| − | + | *This particular integral cannot be solved in a simple, conventional way, but must be evaluated using the [https://en.wikipedia.org/wiki/Cauchy_principal_value »Cauchy principal value«]. | |
| − | + | *Correspondingly valid in the frequency domain: | |
| − | |||
| − | $$ | + | :$$Y(f) = - {\rm j} \cdot {\rm sign}(f) \cdot X(f) \hspace{0.05cm} .$$}} |
| − | + | ||
| − | |||
| − | + | Thus, the result of the last section can be summarized with this definition as follows: | |
| + | *You get from the real, physical band-pass signal $x(t)$ the analytic signal $x_+(t)$ by adding to $x(t)$ an imaginary part according to the Hilbert transform: | ||
| − | $$ | + | :$$x_+(t) = x(t)+{\rm j} \cdot {\rm H}\left\{x(t)\right\} .$$ |
| − | {{ | + | *The Hilbert transform $\text{H}\{x(t)\}$ disappears only in the case of $x(t) = \rm const.$ ⇒ DC signal. With all other signal forms the analytic signal $x_+(t)$ is always complex. |
| + | *From the analytical signal $x_+(t)$ the real band-pass signal can be easily determined by real part formation: | ||
| + | :$$x(t) = {\rm Re}\left\{x_+(t)\right\} .$$ | ||
| − | + | {{GraueBox|TEXT= | |
| − | * | + | $\text{Example 2:}$ The principle of the Hilbert transformation is illustrated here by the following diagram: |
| + | *According to the left representation $\rm (A)$, one gets the analytical signal $x_+(t)$ from the physical signal $x(t)$ by adding an imaginary part ${\rm j} \cdot y(t)$. | ||
| − | $ | + | *Here, $y(t) = {\rm H}\left\{x(t)\right\}$ is a real time function, which can be calculated easily in the spectral domain by multiplying the spectrum $X(f)$ with $- {\rm j} \cdot \sign(f)$. |
| − | + | [[File:P_ID2729__Sig_T_4_2_S2b_neu.png|right|frame|Illustration of the Hilbert transform]] | |
| − | |||
| − | |||
| − | + | The right representation $\rm (B)$ is equivalent to $\rm (A)$: | |
| + | *With the imaginary function $z(t)$ one obtains: | ||
| + | :$$x_+(t) = x(t) + z(t).$$ | ||
| + | *A comparison of both models shows that it is indeed true: | ||
| + | :$$z(t) = {\rm j} \cdot y(t).$$}} | ||
| − | |||
| − | |||
| + | ==Pointer diagram representation of the harmonic oscillation== | ||
| + | <br> | ||
| + | The spectral function $X(f)$ of a harmonic oscillation $x(t) = A \cdot \text{cos}(2\pi f_{\rm T}t - \varphi)$ consists of two Dirac delta functions at frequencies | ||
| + | * $+f_{\rm T}$ with complex weight $A/2 \cdot \text{e}^{-\text{j}\hspace{0.05cm}\varphi}$, | ||
| − | + | * $-f_{\rm T}$ with complex weight $A/2 \cdot \text{e}^{+\text{j}\hspace{0.05cm}\varphi}$. | |
| − | |||
| − | |||
| − | |||
| − | + | Thus, the spectrum of the analytical signal is $($without the Dirac delta function at the frequency $f =-f_{\rm T})$: | |
| − | $$X_+(f) = A \cdot {\rm e}^{-{\rm j} \varphi}\cdot\delta (f - f_{\rm | + | :$$X_+(f) = A \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\varphi}\cdot\delta (f - f_{\rm |
T}) .$$ | T}) .$$ | ||
| − | + | The corresponding time function is obtained by applying the [[Signal_Representation/Fourier_Transform_Theorems#Shifting_Theorem|»Shifting Theorem«]]: | |
| − | $$x_+(t) = A \cdot {\rm e}^{ {\rm j}( 2 \pi f_{\rm T} t | + | :$$x_+(t) = A \cdot {\rm e}^{\hspace{0.05cm} {\rm j}\hspace{0.05cm}\cdot\hspace{0.05cm}( 2 \pi f_{\rm T} t |
\hspace{0.05cm}-\hspace{0.05cm} \varphi)}.$$ | \hspace{0.05cm}-\hspace{0.05cm} \varphi)}.$$ | ||
| − | + | This equation describes a rotating pointer with constant angular velocity $\omega_{\rm T} = 2\pi f_{\rm T}$. | |
| − | + | In the following, we will also refer to the time course of an analytical and frequency-discrete signal $x_+(t)$ as »'''pointer diagram'''«. | |
| + | {{GraueBox|TEXT= | ||
| + | $\text{Example 3:}$ For illustrative reasons the coordinate system here is rotated $($real part upwards, imaginary part to the left$)$, contrary to the usual representation by $90^\circ$. | ||
| − | + | [[File:P_ID712__Sig_T_4_2_S3.png|right|frame|Pointer diagram of a harmonic oscillation]] | |
| − | |||
| − | |||
| − | |||
| − | |||
| + | On the basis of this diagram the following statements are possible: | ||
| + | *At the start time $t = 0$ the pointer of length $A$ $($amplitude$)$ lies with angle $-\varphi$ in the complex plane. In the drawn example, $\varphi = 45^\circ$. | ||
| + | *For times $t > 0$ the pointer rotates with constant angular velocity $($circular frequency$)$ $\omega_{\rm T}$ in mathematically positive direction, i.e. counterclockwise. | ||
| − | + | *The top of the pointer thus always lies on a circle with radius $A$ and requires exactly the time $T_0$, i.e. the »period duration« of the harmonic oscillation $x(t)$ for one rotation. | |
| − | + | *The projection of the analytical signal $x_+(t)$ onto the real axis, marked by red dots, provides the instantaneous values of $x(t)$.}} | |
| + | |||
| + | |||
| + | |||
| + | ==Pointer diagram of a sum of harmonic oscillations== | ||
| + | <br> | ||
| + | For the further description we assume the following spectrum for the analytical signal: | ||
| + | |||
| + | [[File:P_ID715__Sig_T_4_2_S4.png|right|frame|Pointer diagram of a sum of three oscillations]] | ||
| − | $$X_+(f) = \sum_{i=1}^{I}A_i \cdot {\rm e}^{-{\rm j} | + | :$$X_+(f) = \sum_{i=1}^{I}A_i \cdot {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot\hspace{0.05cm} |
\varphi_i}\cdot\delta (f - f_{i}) .$$ | \varphi_i}\cdot\delta (f - f_{i}) .$$ | ||
| − | + | #The left graphic shows such a spectrum for the example $I = 3$. | |
| + | #If one chooses $I$ relatively large and the distance between adjacent spectral lines correspondingly small, then with this equation frequency–continuous spectral functions $X_+(f)$ can also be approximated. | ||
| + | |||
| + | |||
| + | In the right graphic the corresponding time function is indicated. This is in general: | ||
| + | |||
| + | :$$x_+(t) = \sum_{i=1}^{I}A_i \cdot {\rm e}^{ {\rm j}\hspace{0.05cm}\cdot\hspace{0.05cm}(\omega_i | ||
| + | \hspace{0.05cm}\cdot\hspace{0.05cm} t \hspace{0.05cm}-\hspace{0.05cm} \varphi_i)}.$$ | ||
| + | |||
| + | To note about this graphic: | ||
| + | *The sketch shows the initial position of the pointers at start time $t = 0$ corresponding to the amplitudes $A_i$ and the phase positions $\varphi_i$. | ||
| − | [ | + | *The tip of the resulting pointer compound is marked by the violet cross. One obtains by vectorial addition of the three individual pointers for the time $t = 0$: |
| + | :$$x_+(t= 0) = \big [1 \cdot \cos(60^\circ) - 1 \cdot {\rm j} \cdot \sin(60^\circ) \big ]+ 2 \cdot \cos(0^\circ)+1 \cdot \cos(180^\circ) = 1.500 - {\rm j} \cdot 0.866.$$ | ||
| + | *For times $t > 0$ the three pointers rotate at different angular velocities $\omega_i = 2\pi f_i$. The red pointer rotates faster than the green one, but slower than the blue one. | ||
| − | + | *Since all pointers rotate counterclockwise, the resulting pointer $x_+(t)$ will also tend to move in this direction. | |
| − | $ | + | *At time $t = 1\,µ\text {s}$ the tip of the resulting pointer for the given parameter values is |
| − | \ | ||
| − | + | :$$ \begin{align*}x_+(t = 1 {\rm \hspace{0.05cm}µ s}) & = 1 \cdot {\rm e}^{-{\rm | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | $$ \begin{align*}x_+(t = 1 {\rm \hspace{0.05cm} | ||
j}\hspace{0.05cm}\cdot \hspace{0.05cm}60^\circ}\cdot {\rm e}^{{\rm | j}\hspace{0.05cm}\cdot \hspace{0.05cm}60^\circ}\cdot {\rm e}^{{\rm | ||
j}\hspace{0.05cm}2 \pi \hspace{0.05cm}\cdot \hspace{0.1cm}40 | j}\hspace{0.05cm}2 \pi \hspace{0.05cm}\cdot \hspace{0.1cm}40 | ||
| Line 159: | Line 187: | ||
\hspace{0.05cm} \cdot \hspace{0.1cm} 0.001}- 1\cdot {\rm e}^{{\rm | \hspace{0.05cm} \cdot \hspace{0.1cm} 0.001}- 1\cdot {\rm e}^{{\rm | ||
j}\hspace{0.05cm}2 \pi \hspace{0.05cm}\cdot \hspace{0.1cm}60 | j}\hspace{0.05cm}2 \pi \hspace{0.05cm}\cdot \hspace{0.1cm}60 | ||
| − | \hspace{0.05cm} \cdot \hspace{0.1cm} 0.001} \\ | + | \hspace{0.05cm} \cdot \hspace{0.1cm} 0.001} = \\ |
& = 1 \cdot {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot | & = 1 \cdot {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot | ||
\hspace{0.05cm}45.6^\circ} + 2\cdot {\rm e}^{{\rm | \hspace{0.05cm}45.6^\circ} + 2\cdot {\rm e}^{{\rm | ||
| Line 165: | Line 193: | ||
e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}21.6^\circ} \approx | e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}21.6^\circ} \approx | ||
1.673- {\rm j} \cdot 0.464.\end{align*}$$ | 1.673- {\rm j} \cdot 0.464.\end{align*}$$ | ||
| − | * | + | *The resulting pointer tip does not lie on a circle like a single oscillation, but a complicated geometric figure is created. |
| + | |||
| + | |||
| + | The interactive applet [[Applets:Physical_Signal_%26_Analytic_Signal|»Physical Signal and Analytical Signal«]] illustrates $x_+(t)$ for the sum of three harmonic oscillations. | ||
| + | |||
| + | ==Exercises for the chapter== | ||
| + | <br> | ||
| + | [[Aufgaben:Exercise 4.3: Vector Diagram Representation|Exercise 4.3: Vector Diagram Representation]] | ||
| + | |||
| + | [[Aufgaben:Exercise 4.3Z: Hilbert Transformator|Exercise 4.3Z: Hilbert Transformator]] | ||
| + | |||
| + | [[Aufgaben:Exercise 4.4: Vector Diagram for DSB-AM|Exercise 4.4: Vector Diagram for DSB-AM]] | ||
| − | + | [[Aufgaben:Exercise 4.4Z: Vector Diagram for DSB-AM|Exercise 4.4Z: Vector Diagram for SSB-AM]] | |
| + | |||
| + | |||
| − | |||
| − | |||
| − | |||
Latest revision as of 16:48, 19 June 2023
Contents
Definition in the frequency domain
We consider a real band-pass signal $x(t)$ with the corresponding band-pass spectrum $X(f)$, which has an even real and an odd imaginary part with respect to the frequency zero point. It is assumed that the carrier frequency $f_{\rm T}$ is much larger than the bandwidth of the band-pass signal $x(t)$.
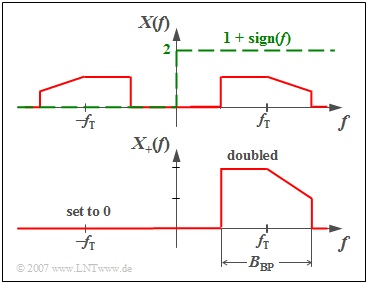
$\text{Definition:}$ The »analytical signal« $x_+(t)$ belonging to the physical signal $x(t)$ is that time function, whose spectrum fulfills the following property:
- $$X_+(f)=\big[1+{\rm sign}(f)\big] \cdot X(f) = \left\{ {2 \cdot X(f) \; \hspace{0.2cm}\rm for\hspace{0.2cm} {\it f} > 0, \atop {\,\,\,\, \rm 0 \; \hspace{0.9cm}\rm for\hspace{0.2cm} {\it f} < 0.} }\right.$$
The »sign function« is equal to $+1$ for positive $f$–values and for negative $f$-values equal to $-1$.
- The $($double sided$)$ limit value returns $\sign(0) = 0$.
- The index "+" should make clear that $X_+(f)$ has only parts at positive frequencies.
From the graphic you can see the calculation rule for $X_+(f)$: The actual band-pass spectrum $X(f)$ will
- be doubled at the positive frequencies, and
- set to zero at the negative frequencies.
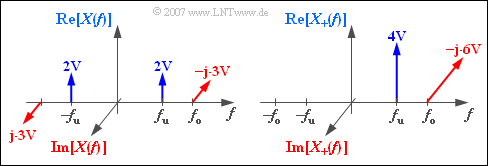
$\text{Example 1:}$ The graph
- on the left shows the $($discrete and complex$)$ spectrum $X(f)$ of the "physical band-pass signal"
- $$x(t) = 4\hspace{0.05cm}{\rm V} \cdot {\cos} ( 2 \pi f_{\rm u} \hspace{0.03cm}t) + 6\hspace{0.05cm}{\rm V} \cdot {\sin} ( 2 \pi f_{\rm o} \hspace{0.03cm}t),$$
- on the right the $($also discrete and complex$)$ spectrum $X_{+}(f)$ of the corresponding "analytical signal" $x_{+}(t)$.
General calculation rule in the time domain
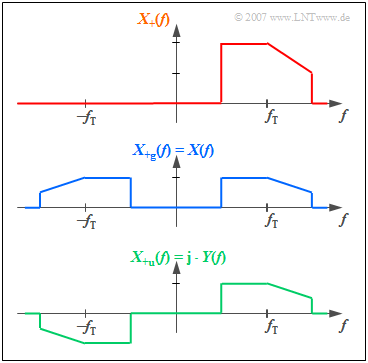
Now we will take a closer look at the spectrum $X_+(f)$ of the analytical signal and divide it with respect to $f = 0$ into
- an even $($German: "gerade" ⇒ "$\rm g"$) part $X_{\rm +g}(f)$, and
- an odd $($German: "ungerade" ⇒ "$\rm u$") part $X_{\rm +u}(f)$:
- $$X_+(f) = X_{\rm +g}(f) + X_{\rm +u}(f).$$
All these spectra are generally complex.
If one considers the »Assignment Theorem« of the Fourier transform, then the following statements are possible on basis of the graph:
- The even part $X_{\rm +g}(f)$ of $X_{+}(f)$ leads after the Fourier transform to a real time signal, and the odd part $X_{\rm +u}(f)$ to an imaginary one.
- It is obvious that $X_{\rm +g}(f)$ is equal to the physical Fourier spectrum $X(f)$ and thus the real part of $x_{\rm +g}(t)$ is equal to the given physical signal $x(t)$ with band-pass properties.
- If we denote the imaginary part with $y(t)$, the analytical signal is:
- $$x_+(t)= x(t) + {\rm j} \cdot y(t) .$$
- According to the generally valid laws of Fourier transform corresponding to the »Assignment Theorem«, the following applies to the spectral function of the imaginary part:
- $${\rm j} \cdot Y(f) = X_{\rm +u}(f)= {\rm sign}(f) \cdot X(f) \hspace{0.3cm}\Rightarrow\hspace{0.3cm}Y(f) = \frac{{\rm sign}(f)}{ {\rm j}}\cdot X(f).$$
- After transforming this equation into the time domain, the multiplication becomes the »convolution«, and one gets:
- $$y(t) = \frac{1}{ {\rm \pi} t} \hspace{0.05cm}\star \hspace{0.05cm}x(t) = \frac{1}{ {\rm \pi}} \cdot \hspace{0.03cm}\int_{-\infty}^{+\infty}\frac{x(\tau)}{ {t - \tau}}\hspace{0.15cm} {\rm d}\tau.$$
Representation with Hilbert transform
At this point it is necessary to briefly discuss a further spectral transformation, which is dealt thoroughly in the book »Linear and Time-invariant Systems« .
$\text{Definition:}$ For the »Hilbert transform« $ {\rm H}\left\{x(t)\right\}$ of a time function $x(t)$ applies:
- $$y(t) = {\rm H}\left\{x(t)\right\} = \frac{1}{ {\rm \pi} } \cdot \hspace{0.03cm}\int_{-\infty}^{+\infty}\frac{x(\tau)}{ {t - \tau} }\hspace{0.15cm} {\rm d}\tau.$$
- This particular integral cannot be solved in a simple, conventional way, but must be evaluated using the »Cauchy principal value«.
- Correspondingly valid in the frequency domain:
- $$Y(f) = - {\rm j} \cdot {\rm sign}(f) \cdot X(f) \hspace{0.05cm} .$$
Thus, the result of the last section can be summarized with this definition as follows:
- You get from the real, physical band-pass signal $x(t)$ the analytic signal $x_+(t)$ by adding to $x(t)$ an imaginary part according to the Hilbert transform:
- $$x_+(t) = x(t)+{\rm j} \cdot {\rm H}\left\{x(t)\right\} .$$
- The Hilbert transform $\text{H}\{x(t)\}$ disappears only in the case of $x(t) = \rm const.$ ⇒ DC signal. With all other signal forms the analytic signal $x_+(t)$ is always complex.
- From the analytical signal $x_+(t)$ the real band-pass signal can be easily determined by real part formation:
- $$x(t) = {\rm Re}\left\{x_+(t)\right\} .$$
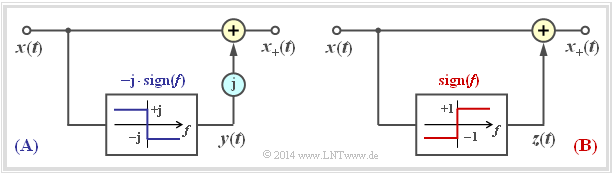
$\text{Example 2:}$ The principle of the Hilbert transformation is illustrated here by the following diagram:
- According to the left representation $\rm (A)$, one gets the analytical signal $x_+(t)$ from the physical signal $x(t)$ by adding an imaginary part ${\rm j} \cdot y(t)$.
- Here, $y(t) = {\rm H}\left\{x(t)\right\}$ is a real time function, which can be calculated easily in the spectral domain by multiplying the spectrum $X(f)$ with $- {\rm j} \cdot \sign(f)$.
The right representation $\rm (B)$ is equivalent to $\rm (A)$:
- With the imaginary function $z(t)$ one obtains:
- $$x_+(t) = x(t) + z(t).$$
- A comparison of both models shows that it is indeed true:
- $$z(t) = {\rm j} \cdot y(t).$$
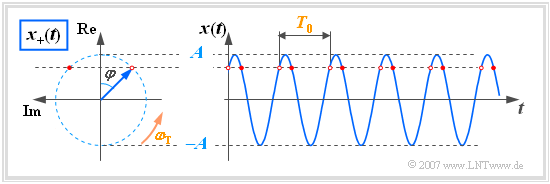
Pointer diagram representation of the harmonic oscillation
The spectral function $X(f)$ of a harmonic oscillation $x(t) = A \cdot \text{cos}(2\pi f_{\rm T}t - \varphi)$ consists of two Dirac delta functions at frequencies
- $+f_{\rm T}$ with complex weight $A/2 \cdot \text{e}^{-\text{j}\hspace{0.05cm}\varphi}$,
- $-f_{\rm T}$ with complex weight $A/2 \cdot \text{e}^{+\text{j}\hspace{0.05cm}\varphi}$.
Thus, the spectrum of the analytical signal is $($without the Dirac delta function at the frequency $f =-f_{\rm T})$:
- $$X_+(f) = A \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\varphi}\cdot\delta (f - f_{\rm T}) .$$
The corresponding time function is obtained by applying the »Shifting Theorem«:
- $$x_+(t) = A \cdot {\rm e}^{\hspace{0.05cm} {\rm j}\hspace{0.05cm}\cdot\hspace{0.05cm}( 2 \pi f_{\rm T} t \hspace{0.05cm}-\hspace{0.05cm} \varphi)}.$$
This equation describes a rotating pointer with constant angular velocity $\omega_{\rm T} = 2\pi f_{\rm T}$.
In the following, we will also refer to the time course of an analytical and frequency-discrete signal $x_+(t)$ as »pointer diagram«.
$\text{Example 3:}$ For illustrative reasons the coordinate system here is rotated $($real part upwards, imaginary part to the left$)$, contrary to the usual representation by $90^\circ$.
On the basis of this diagram the following statements are possible:
- At the start time $t = 0$ the pointer of length $A$ $($amplitude$)$ lies with angle $-\varphi$ in the complex plane. In the drawn example, $\varphi = 45^\circ$.
- For times $t > 0$ the pointer rotates with constant angular velocity $($circular frequency$)$ $\omega_{\rm T}$ in mathematically positive direction, i.e. counterclockwise.
- The top of the pointer thus always lies on a circle with radius $A$ and requires exactly the time $T_0$, i.e. the »period duration« of the harmonic oscillation $x(t)$ for one rotation.
- The projection of the analytical signal $x_+(t)$ onto the real axis, marked by red dots, provides the instantaneous values of $x(t)$.
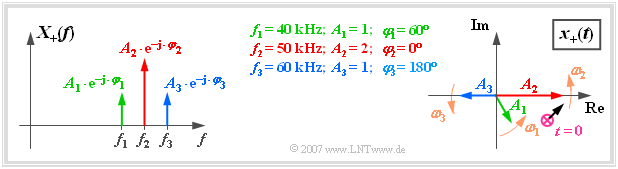
Pointer diagram of a sum of harmonic oscillations
For the further description we assume the following spectrum for the analytical signal:
- $$X_+(f) = \sum_{i=1}^{I}A_i \cdot {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot\hspace{0.05cm} \varphi_i}\cdot\delta (f - f_{i}) .$$
- The left graphic shows such a spectrum for the example $I = 3$.
- If one chooses $I$ relatively large and the distance between adjacent spectral lines correspondingly small, then with this equation frequency–continuous spectral functions $X_+(f)$ can also be approximated.
In the right graphic the corresponding time function is indicated. This is in general:
- $$x_+(t) = \sum_{i=1}^{I}A_i \cdot {\rm e}^{ {\rm j}\hspace{0.05cm}\cdot\hspace{0.05cm}(\omega_i \hspace{0.05cm}\cdot\hspace{0.05cm} t \hspace{0.05cm}-\hspace{0.05cm} \varphi_i)}.$$
To note about this graphic:
- The sketch shows the initial position of the pointers at start time $t = 0$ corresponding to the amplitudes $A_i$ and the phase positions $\varphi_i$.
- The tip of the resulting pointer compound is marked by the violet cross. One obtains by vectorial addition of the three individual pointers for the time $t = 0$:
- $$x_+(t= 0) = \big [1 \cdot \cos(60^\circ) - 1 \cdot {\rm j} \cdot \sin(60^\circ) \big ]+ 2 \cdot \cos(0^\circ)+1 \cdot \cos(180^\circ) = 1.500 - {\rm j} \cdot 0.866.$$
- For times $t > 0$ the three pointers rotate at different angular velocities $\omega_i = 2\pi f_i$. The red pointer rotates faster than the green one, but slower than the blue one.
- Since all pointers rotate counterclockwise, the resulting pointer $x_+(t)$ will also tend to move in this direction.
- At time $t = 1\,µ\text {s}$ the tip of the resulting pointer for the given parameter values is
- $$ \begin{align*}x_+(t = 1 {\rm \hspace{0.05cm}µ s}) & = 1 \cdot {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}60^\circ}\cdot {\rm e}^{{\rm j}\hspace{0.05cm}2 \pi \hspace{0.05cm}\cdot \hspace{0.1cm}40 \hspace{0.05cm} \cdot \hspace{0.1cm} 0.001} + 2\cdot {\rm e}^{{\rm j}\hspace{0.05cm}2 \pi \hspace{0.05cm}\cdot \hspace{0.1cm}50 \hspace{0.05cm} \cdot \hspace{0.1cm} 0.001}- 1\cdot {\rm e}^{{\rm j}\hspace{0.05cm}2 \pi \hspace{0.05cm}\cdot \hspace{0.1cm}60 \hspace{0.05cm} \cdot \hspace{0.1cm} 0.001} = \\ & = 1 \cdot {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}45.6^\circ} + 2\cdot {\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}18^\circ}- 1\cdot {\rm e}^{{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}21.6^\circ} \approx 1.673- {\rm j} \cdot 0.464.\end{align*}$$
- The resulting pointer tip does not lie on a circle like a single oscillation, but a complicated geometric figure is created.
The interactive applet »Physical Signal and Analytical Signal« illustrates $x_+(t)$ for the sum of three harmonic oscillations.
Exercises for the chapter
Exercise 4.3: Vector Diagram Representation
Exercise 4.3Z: Hilbert Transformator
Exercise 4.4: Vector Diagram for DSB-AM
Exercise 4.4Z: Vector Diagram for SSB-AM