Difference between revisions of "Aufgaben:Exercise 3.5: GMSK Modulation"
From LNTwww
m (Javier moved page Exercises:Exercise 3.5: GMSK Modulation to Exercise 3.5: GMSK Modulation: Text replacement - "Exercises:Exercise" to "Aufgaben:Exercise") |
|||
| Line 11: | Line 11: | ||
The graphic illustrates the situation: | The graphic illustrates the situation: | ||
| − | *The digital message is represented by the amplitude coefficients aμ∈{±1} which are applied to a Dirac pulse. It should be noted that the sequence drawn in is assumed for the subtask '''(3)''. | + | *The digital message is represented by the amplitude coefficients aμ∈{±1} which are applied to a Dirac pulse. It should be noted that the sequence drawn in is assumed for the subtask '''(3)'''. |
*The symmetrical rectangular pulse with duration T=TB (GSM bit duration) is dimensionless: | *The symmetrical rectangular pulse with duration T=TB (GSM bit duration) is dimensionless: | ||
| Line 19: | Line 19: | ||
*The Gaussian low pass is given by its frequency response or impulse response: | *The Gaussian low pass is given by its frequency response or impulse response: | ||
:HG(f)=e−π⋅(f2fG)2∙−−−∘hG(t)=2fG⋅e−π⋅(2fG⋅t)2, | :HG(f)=e−π⋅(f2fG)2∙−−−∘hG(t)=2fG⋅e−π⋅(2fG⋅t)2, | ||
| − | : | + | :where the system theoretical cut-off frequency fG is used. In the GSM specification, however, the 3dB cut-off frequency is specified with f3dB=0.3/T . From this, fG can be calculated directly - see subtask '''(2)'''. |
| − | * | + | *The signal after the gauss low pass is thus |
:qG(t)=qR(t)⋆hG(t)=∑νaν⋅g(t−ν⋅T). | :qG(t)=qR(t)⋆hG(t)=∑νaν⋅g(t−ν⋅T). | ||
| − | + | Here g(t) is referred to as ''frequency pulse''. For this one: | |
:g(t)=qR(t)⋆hG(t). | :g(t)=qR(t)⋆hG(t). | ||
| − | * | + | *With the low pass filtered signal qG(t), the carrier frequency fT and the frequency deviation ΔfA can thus be written for the instantaneous frequency at the output of the FSK modulator::fA(t)=fT+ΔfA⋅qG(t). |
| − | :fA(t)=fT+ΔfA⋅qG(t). | ||
| Line 38: | Line 37: | ||
*This exercise belongs to the chapter [[Mobile_Kommunikation/Die_Charakteristika_von_GSM|Die Charakteristika von GSM]]. | *This exercise belongs to the chapter [[Mobile_Kommunikation/Die_Charakteristika_von_GSM|Die Charakteristika von GSM]]. | ||
| − | * | + | *Reference is also made to the chapter [[Beispiele_von_Nachrichtensystemen/Funkschnittstelle|Funkschnittstelle]] in the book „Beispiele von Nachrichtensystemen”. |
| − | * | + | *For your calculations use the exemplary values $f_{\rm T} = 900 \ \ \rm MHz$ and ΔfA=68 kHz. |
| − | * | + | *Use the Gaussian integral to solve the task (some numerical values are given in the table) |
| − | [[File:P_ID2226__Bei_A_3_4b.png|right|frame| | + | [[File:P_ID2226__Bei_A_3_4b.png|right|frame|some values of the Gaussian integral]] |
:ϕ(x)=1√2π⋅∫x−∞e−u2/2du. | :ϕ(x)=1√2π⋅∫x−∞e−u2/2du. | ||
<br clear=all> | <br clear=all> | ||
| Line 52: | Line 51: | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | {In | + | {In what range of values can the instantaneous frequency fA(t) fluctuate? Which requirements must be met? |
|type="{}"} | |type="{}"} | ||
Max [fA(t)] = { 900.068 0.01% } MHz | Max [fA(t)] = { 900.068 0.01% } MHz | ||
Min [fA(t)] = { 899.932 0.01% } MHz | Min [fA(t)] = { 899.932 0.01% } MHz | ||
| − | { | + | {Which (normalized) system-theoretical cut-off frequency of the Gaussian low pass results from the requirement f3dB⋅T=0.3? |
|type="{}"} | |type="{}"} | ||
fG⋅T = { 0.45 3% } | fG⋅T = { 0.45 3% } | ||
| − | { | + | {Calculate the frequency pulse g(t) using the function ϕ(x). How large is the pulse value g(t=0)? |
|type="{}"} | |type="{}"} | ||
g(t=0) = { 0.737 3% } | g(t=0) = { 0.737 3% } | ||
| − | { | + | {Which signal value results for qG(t=3T) with $a_{3} = -1$ and aμ≠3=+1? What is the instantaneous frequency fA(t=3T)? |
|type="{}"} | |type="{}"} | ||
qG(t=3T) = { -0.51822--0.42978 } | qG(t=3T) = { -0.51822--0.42978 } | ||
| − | { | + | {Calculate the pulse values g(t=±T) of the frequency pulse |
|type="{}"} | |type="{}"} | ||
g(t=±T) = { 0.131 3% } | g(t=±T) = { 0.131 3% } | ||
| − | { | + | {The amplitude coefficients are alternating. What is the maximum amount of qG(t) ? Consider g(t≥2T)≈0. |
|type="{}"} | |type="{}"} | ||
Max |qG(t)| = { 0.475 3% } | Max |qG(t)| = { 0.475 3% } | ||
| Line 84: | Line 83: | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' If all amplitude coefficients aμ are equal to +1, then qR(t)=1 is a constant. Thus, the Gaussian low pass has no influence and qG(t)=1 results. |
| − | * | + | *The maximum frequency is thus |
:Max [fA(t)]=fT+ΔfA=900.068MHz_. | :Max [fA(t)]=fT+ΔfA=900.068MHz_. | ||
| − | * | + | *The minimum instantaneous frequency |
:Min [fA(t)]=fT−ΔfA=899.932MHz_ | :Min [fA(t)]=fT−ΔfA=899.932MHz_ | ||
| − | + | is obtained when all amplitude coefficients are negative. In this case qR(t)=qG(t)=−1. | |
| − | '''(2)''' | + | '''(2)''' The frequency at which the logarithmic power transfer function is 3 dB less than f=0 is called the 3dB cut-off frequency. |
| − | * | + | *This can also be expressed as follows: |
:|H(f=f3dB)||H(f=0)|=1√2. | :|H(f=f3dB)||H(f=0)|=1√2. | ||
| − | * | + | *In particular the Gauss low pass because of H(f=0)=1: |
:$$ H(f = f_{\rm 3dB})= {\rm e}^{-\pi\cdot ({f_{\rm 3dB}}/{2 f_{\rm G}})^2} = \frac{1}{\sqrt{2}}\hspace{0.3cm} | :$$ H(f = f_{\rm 3dB})= {\rm e}^{-\pi\cdot ({f_{\rm 3dB}}/{2 f_{\rm G}})^2} = \frac{1}{\sqrt{2}}\hspace{0.3cm} | ||
\Rightarrow \hspace{0.3cm}(\frac{f_{\rm 3dB}}{2 f_{\rm G}})^2 = \frac{{\rm ln}\hspace{0.1cm}\sqrt{2}}{\pi} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}f_{\rm G} = \sqrt{\frac{\pi}{4 \cdot {\rm ln}\hspace{0.1cm}\sqrt{2}}}\cdot f_{\rm 3dB}\hspace{0.05cm}.$$ | \Rightarrow \hspace{0.3cm}(\frac{f_{\rm 3dB}}{2 f_{\rm G}})^2 = \frac{{\rm ln}\hspace{0.1cm}\sqrt{2}}{\pi} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}f_{\rm G} = \sqrt{\frac{\pi}{4 \cdot {\rm ln}\hspace{0.1cm}\sqrt{2}}}\cdot f_{\rm 3dB}\hspace{0.05cm}.$$ | ||
| − | * | + | *The numerical evaluation leads to fG≈1.5⋅f3dB. |
| − | * | + | *{\f_{\rm 3dB} \cdot T = 0.3$ is followed by fG⋅T≈0.45_. |
| − | |||
| Line 130: | Line 128: | ||
*Es ergeben sich also Impulsinterferenzen und man erhält: | *Es ergeben sich also Impulsinterferenzen und man erhält: | ||
:Max[qG(t)]=g(t=0)−2⋅g(t=T)=0.737−2⋅0.131=0.475_. | :Max[qG(t)]=g(t=0)−2⋅g(t=T)=0.737−2⋅0.131=0.475_. | ||
| + | |||
| + | |||
| + | |||
| + | {{ML-Fuß}} | ||
| + | |||
| + | |||
| + | |||
| + | [[Category:Exercises for Mobile Communications|^3.3 Characteristics of GSM^]] | ||
| + | |||
| + | {{quiz-Header|Buchseite=Mobile Kommunikation/Die Charakteristika von GSM | ||
| + | }} | ||
| + | |||
| + | [[File:EN_Mob_A_3_5.png|right|frame|Verschiedene Signale bei GMSK-Modulation]] | ||
| + | The modulation method used for GSM is ''Gaussian Minimum Shift Keying'', short GMSK. This is a special type of FSK (''Frequency Shift Keying'') with CP-FSK (''Continuous Phase Matching''), where | ||
| + | *the modulation index has the smallest value that just satisfies the orthogonality condition: h=0.5 ⇒ ''Minimum Shift Keying'', | ||
| + | *a Gaussian low pass with the impulse response hG(t) is inserted before the FSK modulator, with the aim of saving even more bandwidth. | ||
| + | |||
| + | |||
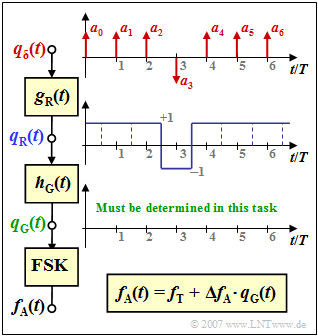
| + | The graphic illustrates the situation: | ||
| + | |||
| + | *The digital message is represented by the amplitude coefficients aμ∈{±1} which are applied to a Dirac pulse. It should be noted that the sequence drawn in is assumed for the subtask '''(3)'''. | ||
| + | |||
| + | *The symmetrical rectangular pulse with duration T=TB (GSM bit duration) is dimensionless: | ||
| + | :gR(t)={10f¨urf¨ur|t|<T/2,|t|>T/2. | ||
| + | *This results for the rectangular signal | ||
| + | :qR(t)=qδ(t)⋆gR(t)=∑νaν⋅gR(t−ν⋅T). | ||
| + | *The Gaussian low pass is given by its frequency response or impulse response: | ||
| + | :HG(f)=e−π⋅(f2fG)2∙−−−∘hG(t)=2fG⋅e−π⋅(2fG⋅t)2, | ||
| + | :where the system theoretical cut-off frequency fG is used. In the GSM specification, however, the 3dB cut-off frequency is specified with f3dB=0.3/T . From this, fG can be calculated directly - see subtask '''(2)'''. | ||
| + | |||
| + | *The signal after the gauss low pass is thus | ||
| + | :qG(t)=qR(t)⋆hG(t)=∑νaν⋅g(t−ν⋅T). | ||
| + | Here g(t) is referred to as ''frequency pulse''. For this one: | ||
| + | :g(t)=qR(t)⋆hG(t). | ||
| + | |||
| + | *With the low pass filtered signal qG(t), the carrier frequency fT and the frequency deviation ΔfA can thus be written for the instantaneous frequency at the output of the FSK modulator::fA(t)=fT+ΔfA⋅qG(t). | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | ''Notes:'' | ||
| + | |||
| + | *This exercise belongs to the chapter [[Mobile_Kommunikation/Die_Charakteristika_von_GSM|Die Charakteristika von GSM]]. | ||
| + | *Reference is also made to the chapter [[Beispiele_von_Nachrichtensystemen/Funkschnittstelle|Funkschnittstelle]] in the book „Beispiele von Nachrichtensystemen”. | ||
| + | |||
| + | *For your calculations use the exemplary values fT=900 MHz and ΔfA=68 kHz. | ||
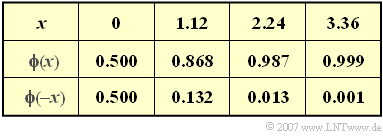
| + | *Use the Gaussian integral to solve the task (some numerical values are given in the table) | ||
| + | [[File:P_ID2226__Bei_A_3_4b.png|right|frame|some values of the Gaussian integral]] | ||
| + | :ϕ(x)=1√2π⋅∫x−∞e−u2/2du. | ||
| + | <br clear=all> | ||
| + | |||
| + | |||
| + | |||
| + | ===Fragebogen=== | ||
| + | |||
| + | <quiz display=simple> | ||
| + | |||
| + | {In what range of values can the instantaneous frequency fA(t) fluctuate? Which requirements must be met? | ||
| + | |type="{}"} | ||
| + | Max [fA(t)] = { 900.068 0.01% } MHz | ||
| + | Min [fA(t)] = { 899.932 0.01% } MHz | ||
| + | |||
| + | {Which (normalized) system-theoretical cut-off frequency of the Gaussian low pass results from the requirement f3dB⋅T=0.3? | ||
| + | |type="{}"} | ||
| + | fG⋅T = { 0.45 3% } | ||
| + | |||
| + | {Calculate the frequency pulse g(t) using the function ϕ(x). How large is the pulse value g(t=0)? | ||
| + | |type="{}"} | ||
| + | g(t=0) = { 0.737 3% } | ||
| + | |||
| + | {Which signal value results for qG(t=3T) with a3=−1 and aμ≠3=+1? What is the instantaneous frequency fA(t=3T)? | ||
| + | |type="{}"} | ||
| + | qG(t=3T) = { -0.51822--0.42978 } | ||
| + | |||
| + | {Calculate the pulse values g(t=±T) of the frequency pulse | ||
| + | |type="{}"} | ||
| + | g(t=±T) = { 0.131 3% } | ||
| + | |||
| + | {The amplitude coefficients are alternating. What is the maximum amount of qG(t) ? Consider g(t≥2T)≈0. | ||
| + | |type="{}"} | ||
| + | Max |qG(t)| = { 0.475 3% } | ||
| + | |||
| + | |||
| + | |||
| + | </quiz> | ||
| + | |||
| + | ===Sample solution=== | ||
| + | {{ML-Kopf}} | ||
| + | |||
| + | '''(1)''' If all amplitude coefficients aμ are equal to +1, then qR(t)=1 is a constant. Thus, the Gaussian low pass has no influence and qG(t)=1 results. | ||
| + | *The maximum frequency is thus | ||
| + | :Max [fA(t)]=fT+ΔfA=900.068MHz_. | ||
| + | *The minimum instantaneous frequency | ||
| + | :Min [fA(t)]=fT−ΔfA=899.932MHz_ | ||
| + | is obtained when all amplitude coefficients are negative. In this case qR(t)=qG(t)=−1. | ||
| + | |||
| + | |||
| + | |||
| + | '''(2)''' The frequency at which the logarithmic power transfer function is 3 dB less than f=0 is called the 3dB cut-off frequency. | ||
| + | *This can also be expressed as follows: | ||
| + | :|H(f=f3dB)||H(f=0)|=1√2. | ||
| + | *In particular the Gauss low pass because of H(f=0)=1: | ||
| + | :$$ H(f = f_{\rm 3dB})= {\rm e}^{-\pi\cdot ({f_{\rm 3dB}}/{2 f_{\rm G}})^2} = \frac{1}{\sqrt{2}}\hspace{0.3cm} | ||
| + | \Rightarrow \hspace{0.3cm}(\frac{f_{\rm 3dB}}{2 f_{\rm G}})^2 = \frac{{\rm ln}\hspace{0.1cm}\sqrt{2}}{\pi} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}f_{\rm G} = \sqrt{\frac{\pi}{4 \cdot {\rm ln}\hspace{0.1cm}\sqrt{2}}}\cdot f_{\rm 3dB}\hspace{0.05cm}.$$ | ||
| + | *The numerical evaluation leads to fG≈1.5⋅f3dB. | ||
| + | |||
| + | |||
Revision as of 09:47, 30 June 2020
The modulation method used for GSM is Gaussian Minimum Shift Keying, short GMSK. This is a special type of FSK (Frequency Shift Keying) with CP-FSK (Continuous Phase Matching), where
- the modulation index has the smallest value that just satisfies the orthogonality condition: h=0.5 ⇒ Minimum Shift Keying,
- a Gaussian low pass with the impulse response hG(t) is inserted before the FSK modulator, with the aim of saving even more bandwidth.
The graphic illustrates the situation:
- The digital message is represented by the amplitude coefficients aμ∈{±1} which are applied to a Dirac pulse. It should be noted that the sequence drawn in is assumed for the subtask (3).
- The symmetrical rectangular pulse with duration T=TB (GSM bit duration) is dimensionless:
- gR(t)={10f¨urf¨ur|t|<T/2,|t|>T/2.
- This results for the rectangular signal
- qR(t)=qδ(t)⋆gR(t)=∑νaν⋅gR(t−ν⋅T).
- The Gaussian low pass is given by its frequency response or impulse response:
- HG(f)=e−π⋅(f2fG)2∙−−−∘hG(t)=2fG⋅e−π⋅(2fG⋅t)2,
- where the system theoretical cut-off frequency fG is used. In the GSM specification, however, the 3dB cut-off frequency is specified with f3dB=0.3/T . From this, fG can be calculated directly - see subtask (2).
- The signal after the gauss low pass is thus
- qG(t)=qR(t)⋆hG(t)=∑νaν⋅g(t−ν⋅T).
Here g(t) is referred to as frequency pulse. For this one:
- g(t)=qR(t)⋆hG(t).
- With the low pass filtered signal qG(t), the carrier frequency fT and the frequency deviation ΔfA can thus be written for the instantaneous frequency at the output of the FSK modulator::fA(t)=fT+ΔfA⋅qG(t).
Notes:
- This exercise belongs to the chapter Die Charakteristika von GSM.
- Reference is also made to the chapter Funkschnittstelle in the book „Beispiele von Nachrichtensystemen”.
- For your calculations use the exemplary values fT=900 MHz and ΔfA=68 kHz.
- Use the Gaussian integral to solve the task (some numerical values are given in the table)
- ϕ(x)=1√2π⋅∫x−∞e−u2/2du.
Fragebogen
Sample solution
(1) If all amplitude coefficients aμ are equal to +1, then qR(t)=1 is a constant. Thus, the Gaussian low pass has no influence and qG(t)=1 results.
- The maximum frequency is thus
- Max [fA(t)]=fT+ΔfA=900.068MHz_.
- The minimum instantaneous frequency
- Min [fA(t)]=fT−ΔfA=899.932MHz_
is obtained when all amplitude coefficients are negative. In this case qR(t)=qG(t)=−1.
(2) The frequency at which the logarithmic power transfer function is 3 dB less than f=0 is called the 3dB cut-off frequency.
- This can also be expressed as follows:
- |H(f=f3dB)||H(f=0)|=1√2.
- In particular the Gauss low pass because of H(f=0)=1:
- H(f=f3dB)=e−π⋅(f3dB/2fG)2=1√2⇒(f3dB2fG)2=ln√2π⇒fG=√π4⋅ln√2⋅f3dB.
- The numerical evaluation leads to fG≈1.5⋅f3dB.
- {\f_{\rm 3dB} \cdot T = 0.3isfollowedbyf_{\rm G} \cdot T \underline{\approx 0.45}.‴{\rm g}(t) ergibt sich aus der Faltung von Rechteckfunktion g_{\rm R}(t) mit der Impulsantwort h_{\rm G}(t): :'"`UNIQ-MathJax54-QINU`"' *Mit der Substitution u^{2} = 8π \cdot {f_{G}}^{2} \cdot \tau^{2} und der Funktion \phi (x) kann man hierfür auch schreiben: :'"`UNIQ-MathJax55-QINU`"' *Für die Zeit t = 0 gilt unter Berücksichtigung von \phi (-x) = 1 - \phi (x) und f_{\rm G} \cdot T = 0.45: :'"`UNIQ-MathJax56-QINU`"' '''(4)''' Mit a_{3} = +1 würde sich q_{\rm G}(t = 3 T) = 1 ergeben. Aufgrund der Linearität gilt somit: :'"`UNIQ-MathJax57-QINU`"' '''(5)''' Mit dem Ergebnis aus (3) und f_{\rm G} \cdot T = 0.45 erhält man: :'"`UNIQ-MathJax58-QINU`"' *Der Impulswert g(t = -T) ist aufgrund der Symmetrie des Gaußtiefpasses genau so groß. '''(6)''' Bei alternierender Folge sind aus Symmetriegründen die Beträge |q_{\rm G}(\mu \cdot T)| bei allen Vielfachen der Bitdauer T alle gleich. *Alle Zwischenwerte bei t \approx \mu \cdot T sind dagegen kleiner. *Unter Berücksichtigung von g(t ≥ 2T) \approx 0 wird jeder einzelne Impulswert g(0) durch den vorangegangenen Impuls mit g(t = T) verkleinert, ebenso vom folgenden Impuls mit g(t = -T). *Es ergeben sich also Impulsinterferenzen und man erhält: :'"`UNIQ-MathJax59-QINU`"' '"`UNIQ--html-00000004-QINU`"' [[Category:Exercises for Mobile Communications|^3.3 Characteristics of GSM^]] '"`UNIQ--html-00000005-QINU`"' [[Mobile Kommunikation/Die Charakteristika von GSM | Return to book]] '"`UNIQ--html-00000006-QINU`"' [[File:EN_Mob_A_3_5.png|right|frame|Verschiedene Signale bei GMSK-Modulation]] The modulation method used for GSM is ''Gaussian Minimum Shift Keying'', short GMSK. This is a special type of FSK (''Frequency Shift Keying'') with CP-FSK (''Continuous Phase Matching''), where *the modulation index has the smallest value that just satisfies the orthogonality condition: h = 0.5 ⇒ ''Minimum Shift Keying'', *a Gaussian low pass with the impulse response h_{\rm G}(t) is inserted before the FSK modulator, with the aim of saving even more bandwidth. The graphic illustrates the situation: *The digital message is represented by the amplitude coefficients a_{\mu} ∈ \{±1\} which are applied to a Dirac pulse. It should be noted that the sequence drawn in is assumed for the subtask '''(3)'''. *The symmetrical rectangular pulse with duration T = T_{\rm B} (GSM bit duration) is dimensionless: :'"`UNIQ-MathJax60-QINU`"' *This results for the rectangular signal :'"`UNIQ-MathJax61-QINU`"' *The Gaussian low pass is given by its frequency response or impulse response: :'"`UNIQ-MathJax62-QINU`"' :where the system theoretical cut-off frequency f_{\rm G} is used. In the GSM specification, however, the 3dB cut-off frequency is specified with f_{\rm 3dB} = 0.3/T . From this, f_{\rm G} can be calculated directly - see subtask '''(2)'''. *The signal after the gauss low pass is thus :'"`UNIQ-MathJax63-QINU`"' Here g(t) is referred to as ''frequency pulse''. For this one: :'"`UNIQ-MathJax64-QINU`"' *With the low pass filtered signal q_{\rm G}(t), the carrier frequency f_{\rm T} and the frequency deviation \Delta f_{\rm A} can thus be written for the instantaneous frequency at the output of the FSK modulator::'"`UNIQ-MathJax65-QINU`"' ''Notes:'' *This exercise belongs to the chapter [[Mobile_Kommunikation/Die_Charakteristika_von_GSM|Die Charakteristika von GSM]]. *Reference is also made to the chapter [[Beispiele_von_Nachrichtensystemen/Funkschnittstelle|Funkschnittstelle]] in the book „Beispiele von Nachrichtensystemen”. *For your calculations use the exemplary values f_{\rm T} = 900 \ \ \rm MHz and \Delta f_{\rm A} = 68 \ \rm kHz. *Use the Gaussian integral to solve the task (some numerical values are given in the table) [[File:P_ID2226__Bei_A_3_4b.png|right|frame|some values of the Gaussian integral]] :'"`UNIQ-MathJax66-QINU`"' <br clear="all"> ==='"`UNIQ--h-2--QINU`"'Fragebogen=== '"`UNIQ--quiz-00000007-QINU`"' ==='"`UNIQ--h-3--QINU`"'Sample solution=== '"`UNIQ--html-00000003-QINU`"' '''(1)''' If all amplitude coefficients a_{\mu} are equal to +1, then q_{\rm R}(t) = 1 is a constant. Thus, the Gaussian low pass has no influence and q_{\rm G}(t) = 1 results. *The maximum frequency is thus :'"`UNIQ-MathJax67-QINU`"' *The minimum instantaneous frequency :'"`UNIQ-MathJax68-QINU`"' is obtained when all amplitude coefficients are negative. In this case q_{\rm R}(t) = q_{\rm G}(t) = -1. '''(2)''' The frequency at which the logarithmic power transfer function is 3 \ \rm dB less than f = 0 is called the 3dB cut-off frequency. *This can also be expressed as follows: :'"`UNIQ-MathJax69-QINU`"' *In particular the Gauss low pass because of H(f = 0) = 1: :'"`UNIQ-MathJax70-QINU`"' *The numerical evaluation leads to f_{\rm G} \approx 1.5 \cdot f_{\rm 3dB}$.