Difference between revisions of "Aufgaben:Exercise 5.5: Fast Fourier Transform"
m (Text replacement - "Category:Exercises for Signal Representation" to "Category:Signal Representation: Exercises") |
|||
| Line 3: | Line 3: | ||
}} | }} | ||
| − | [[File:EN_Sig_A_5_5.png|right|frame|FFT | + | [[File:EN_Sig_A_5_5.png|right|frame|FFT algorithm for N=8]] |
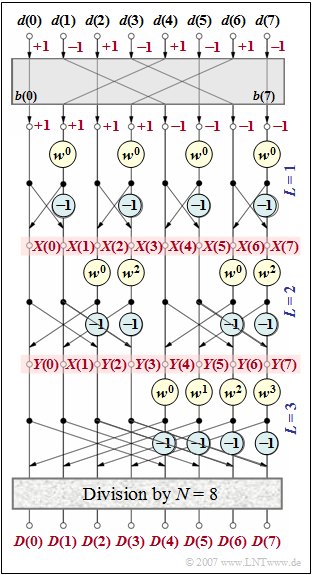
| − | + | The graph shows the signal flow diagram of the FFT for N = 8. The associated spectral coefficients $D(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , D(7)$ are determined from the time coefficients $d(0), \hspace{0.03cm}\text{...} \hspace{0.1cm}, d(7)$ . The following applies to these with 0 ≤ μ ≤ 7: | |
:$$D(\mu) = \frac{1}{N}\cdot \sum_{\nu = 0 }^{N-1} | :$$D(\mu) = \frac{1}{N}\cdot \sum_{\nu = 0 }^{N-1} | ||
| Line 11: | Line 11: | ||
\hspace{0.05cm}\mu}\hspace{0.05cm},$$ | \hspace{0.05cm}\mu}\hspace{0.05cm},$$ | ||
| − | + | where the complex rotation factor $w = \text{e}^{-\text{j}\hspace{0.05cm} \cdot | |
| − | \hspace{0.05cm}2\pi /N}$ | + | \hspace{0.05cm}2\pi /N}$ is to be used, i.e. $w = \text{e}^{-\text{j}\hspace{0.05cm} \cdot |
\hspace{0.05cm}\pi /4} für N = 8$. | \hspace{0.05cm}\pi /4} für N = 8$. | ||
| − | * | + | *The alternating ±1 sequence \langle\hspace{0.05cm} d(ν)\hspace{0.05cm}\rangle is applied to the input. |
| − | * | + | *After the bit reversal operation, this results in the sequence \langle \hspace{0.05cm}b(\kappa)\hspace{0.05cm}\rangle. |
| − | + | It holds that b(κ) = d(ν), if ν is represented as a dual number and the resulting three bits are written as κ in reverse order. For example | |
| − | * | + | * ν = 1 (binary 001) is followed by κ = 4 (binary 100), |
| − | * | + | * d(2) remains at the same position 2 (binary 010). |
| − | + | The actual FFT algorithm happens for the example N = 8 in \log_2 N = 3 stages, denoted L = 1, L =2 and L = 3 . Further: | |
| − | * In | + | * In each stage, four basic operations - so-called ''butterflies'' - are to be performed. |
| − | * | + | * The values at the output of the first stage are designated in this task as X(0),\hspace{0.03cm}\text{...} \hspace{0.1cm} , X(7) , those of the second as Y(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , Y(7). |
| − | * | + | * After the third and last stage, all values must be divided by N . The final result D(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , D(7) is then available here. |
| Line 35: | Line 35: | ||
| − | '' | + | ''Hint:'' |
| − | * | + | *This task belongs to the chapter [[Signal_Representation/Fast_Fourier_Transform_(FFT)|Fast Fourier Transform (FFT)]]. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the DFT coefficient D(3). |
|type="{}"} | |type="{}"} | ||
D(3) \ = \ { 0. } | D(3) \ = \ { 0. } | ||
| − | { | + | {Calculate the DFT coefficient D(4). |
|type="{}"} | |type="{}"} | ||
D(4) \ = \ { 1 3% } | D(4) \ = \ { 1 3% } | ||
| − | { | + | {Determine the initial values X(0), ... , X(7) of the first stage. Which of the following statements are true? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - All X–values with even indices are equal to 2. |
| − | + | + | + All X–values with odd indices are equal to 0. |
| + | |||
| + | {Determine the initial values Y(0), ... , Y(7) of the second stage. Enter the values Y(0) and Y(4) as a check. | ||
| − | |||
|type="{}"} | |type="{}"} | ||
Y(0) \ = \ { 4 3% } | Y(0) \ = \ { 4 3% } | ||
Y(4) \ = \ { -4.12--3.88 } | Y(4) \ = \ { -4.12--3.88 } | ||
| − | { | + | {Calculate all N spectral values D(\mu), in particular |
|type="{}"} | |type="{}"} | ||
D(\mu = 4) \ = \ { 1 3% } | D(\mu = 4) \ = \ { 1 3% } | ||
D(\mu \neq 4) \ = \ { 0. } | D(\mu \neq 4) \ = \ { 0. } | ||
| − | { | + | {What would be the spectral coefficients for d(ν = 4) = 1 and d(ν \neq 4) = 0 ? <br>Enter the values D(3) and D(4) as a check. |
|type="{}"} | |type="{}"} | ||
D(\mu = 3) \ = \ { -1.03--0.97 } | D(\mu = 3) \ = \ { -1.03--0.97 } | ||
| Line 76: | Line 77: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' According to the general DFT equation given on the specification sheet, with $w = \text{e}^{-\text{j}\hspace{0.05cm} \cdot |
| − | \hspace{0.05cm}\pi /4}$ | + | \hspace{0.05cm}\pi /4}$ taking into account the alternating time coefficients: |
:$$8 \cdot D(3) = w^0 - w^3 + w^6- w^9+ w^{12}- w^{15}+ w^{18}- | :$$8 \cdot D(3) = w^0 - w^3 + w^6- w^9+ w^{12}- w^{15}+ w^{18}- | ||
| Line 86: | Line 87: | ||
w^{5}\hspace{0.05cm}.$$ | w^{5}\hspace{0.05cm}.$$ | ||
| − | * | + | *Here it is taken into account that due to the periodicity w_9 = w_1, w_{12} = w_4, w_{15} = w_7, w_{18} = w_2 und w_{21} = w_5 ist. |
| − | * | + | *After re-sorting, the same applies: |
:8 \cdot D(3) = (w^0 + w^4) - (w^1 + w^5)+ (w^2 + w^6) - (w^3 + w^7) = (1 + w + w^2+ w^3) \cdot (w^0 + w^4)\hspace{0.05cm}. | :8 \cdot D(3) = (w^0 + w^4) - (w^1 + w^5)+ (w^2 + w^6) - (w^3 + w^7) = (1 + w + w^2+ w^3) \cdot (w^0 + w^4)\hspace{0.05cm}. | ||
| − | * | + | *Thus, because w_0 = 1 and w_4 = \text{e}^{-\text{j}\pi } = \hspace{0.08cm} - \hspace{-0.08cm}1 , we obtain \underline {D(3) = 0}. |
| − | '''(2)''' In | + | '''(2)''' In analogy to sub-taske '''(1)''' , we now get: |
:$$ 8 \cdot D(4) = w^0 - w^4 + w^8- w^{12}+ w^{16}- w^{20}+ | :$$ 8 \cdot D(4) = w^0 - w^4 + w^8- w^{12}+ w^{16}- w^{20}+ | ||
| Line 101: | Line 102: | ||
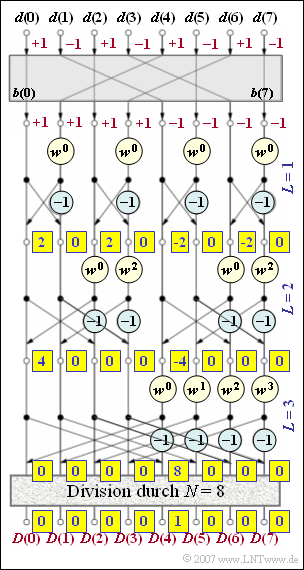
| − | [[File:P_ID1178__Sig_A_5_5c_neu.png|right|frame| | + | [[File:P_ID1178__Sig_A_5_5c_neu.png|right|frame|Example for the FFT algorithm]] |
| − | '''(3)''' | + | '''(3)''' <u>Proposed solution 2</u> is correct: |
| − | * | + | *The term w^0 = 1 does not have to be taken into account. |
| − | * | + | *All output values with odd indices are zero by subtracting two identical input values. |
| − | * | + | *The first statement is not true: Es gilt X(0) = X(2) = +2 and X(4) = X(6) = - 2. |
| − | '''(4)''' | + | '''(4)''' The multiplication with w^{2} = -{\rm j} can be dispensed with, since in the signal flow diagram the corresponding input values are zero. |
| − | * | + | *One thus obtains Y(0) \;\underline{= 4} and Y(4) \;\underline{= - \hspace{-0.03cm}4}. |
| − | * | + | *All other values are zero. |
| − | '''(5)''' | + | '''(5)''' Because of Y(5) = Y(6) =Y(7) = 0 , the multiplications with w, w^2 and w^3 do not matter in the third stage either. All spectral coefficients D(\mu) therefore result in zero with the exception of |
:$$\hspace{0.15 cm}\underline{D(4)} = {1}/{N}\cdot \left[Y(0) - Y(4) \right ] \hspace{0.15 cm}\underline{= 1} | :$$\hspace{0.15 cm}\underline{D(4)} = {1}/{N}\cdot \left[Y(0) - Y(4) \right ] \hspace{0.15 cm}\underline{= 1} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | + | This result agrees with the results from '''(1)''' und '''(2)''' . | |
| − | '''(6)''' | + | '''(6)''' Since both the time coefficients d(ν) and all spectral coefficients D(\mu) are purely real, there is no difference between the FFT and the IFFT. |
| − | * | + | *This means at the same time: The input and output values can be interchanged. |
| − | * | + | *Subtask '''(5)''' gave the following result: |
| − | :$$d({\rm | + | :$$d({\rm even}\hspace{0.15cm}\nu) = +1, \hspace{0.2cm}d({\rm |
| − | + | odd}\hspace{0.15cm}\nu)= -1$$ | |
:$$\Rightarrow | :$$\Rightarrow | ||
\hspace{0.3cm}D(\mu = 4)= 1,\hspace{0.2cm}D(\mu \ne 4)= 0.$$ | \hspace{0.3cm}D(\mu = 4)= 1,\hspace{0.2cm}D(\mu \ne 4)= 0.$$ | ||
| − | * | + | *By swapping the input and output values, we arrive at problem '''(6)''': |
:$$d(\nu = 4)= 1, \hspace{0.2cm}d(\nu \ne 4)= 0 \hspace{0.3cm} | :$$d(\nu = 4)= 1, \hspace{0.2cm}d(\nu \ne 4)= 0 \hspace{0.3cm} | ||
| Line 141: | Line 142: | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *In particular, this results in D(3) \; \underline{= -1} und D(4) \; \underline{= +1}. |
{{ML-Fuß}} | {{ML-Fuß}} | ||
__NOEDITSECTION__ | __NOEDITSECTION__ | ||
[[Category:Signal Representation: Exercises|^5.5 Fast Fourier Transform ^]] | [[Category:Signal Representation: Exercises|^5.5 Fast Fourier Transform ^]] | ||
Revision as of 15:36, 23 March 2021
The graph shows the signal flow diagram of the FFT for N = 8. The associated spectral coefficients D(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , D(7) are determined from the time coefficients d(0), \hspace{0.03cm}\text{...} \hspace{0.1cm}, d(7) . The following applies to these with 0 ≤ μ ≤ 7:
- D(\mu) = \frac{1}{N}\cdot \sum_{\nu = 0 }^{N-1} d(\nu) \cdot {w}^{\hspace{0.03cm}\nu \hspace{0.05cm} \cdot \hspace{0.05cm}\mu}\hspace{0.05cm},
where the complex rotation factor w = \text{e}^{-\text{j}\hspace{0.05cm} \cdot \hspace{0.05cm}2\pi /N} is to be used, i.e. w = \text{e}^{-\text{j}\hspace{0.05cm} \cdot \hspace{0.05cm}\pi /4} für N = 8.
- The alternating ±1 sequence \langle\hspace{0.05cm} d(ν)\hspace{0.05cm}\rangle is applied to the input.
- After the bit reversal operation, this results in the sequence \langle \hspace{0.05cm}b(\kappa)\hspace{0.05cm}\rangle.
It holds that b(κ) = d(ν), if ν is represented as a dual number and the resulting three bits are written as κ in reverse order. For example
- ν = 1 (binary 001) is followed by κ = 4 (binary 100),
- d(2) remains at the same position 2 (binary 010).
The actual FFT algorithm happens for the example N = 8 in \log_2 N = 3 stages, denoted L = 1, L =2 and L = 3 . Further:
- In each stage, four basic operations - so-called butterflies - are to be performed.
- The values at the output of the first stage are designated in this task as X(0),\hspace{0.03cm}\text{...} \hspace{0.1cm} , X(7) , those of the second as Y(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , Y(7).
- After the third and last stage, all values must be divided by N . The final result D(0), \hspace{0.03cm}\text{...} \hspace{0.1cm} , D(7) is then available here.
Hint:
- This task belongs to the chapter Fast Fourier Transform (FFT).
Questions
Solution
- 8 \cdot D(3) = w^0 - w^3 + w^6- w^9+ w^{12}- w^{15}+ w^{18}- w^{21} = w^0 - w^3 + w^2- w^1+ w^{4}- w^{7}+ w^{6}- w^{5}\hspace{0.05cm}.
- Here it is taken into account that due to the periodicity w_9 = w_1, w_{12} = w_4, w_{15} = w_7, w_{18} = w_2 und w_{21} = w_5 ist.
- After re-sorting, the same applies:
- 8 \cdot D(3) = (w^0 + w^4) - (w^1 + w^5)+ (w^2 + w^6) - (w^3 + w^7) = (1 + w + w^2+ w^3) \cdot (w^0 + w^4)\hspace{0.05cm}.
- Thus, because w_0 = 1 and w_4 = \text{e}^{-\text{j}\pi } = \hspace{0.08cm} - \hspace{-0.08cm}1 , we obtain \underline {D(3) = 0}.
(2) In analogy to sub-taske (1) , we now get:
- 8 \cdot D(4) = w^0 - w^4 + w^8- w^{12}+ w^{16}- w^{20}+ w^{24}- w^{28}= 4 \cdot (w^0 - w^4)= 8 \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\hspace{0.15 cm}\underline{D(4) = 1}\hspace{0.05cm}.
(3) Proposed solution 2 is correct:
- The term w^0 = 1 does not have to be taken into account.
- All output values with odd indices are zero by subtracting two identical input values.
- The first statement is not true: Es gilt X(0) = X(2) = +2 and X(4) = X(6) = - 2.
(4) The multiplication with w^{2} = -{\rm j} can be dispensed with, since in the signal flow diagram the corresponding input values are zero.
- One thus obtains Y(0) \;\underline{= 4} and Y(4) \;\underline{= - \hspace{-0.03cm}4}.
- All other values are zero.
(5) Because of Y(5) = Y(6) =Y(7) = 0 , the multiplications with w, w^2 and w^3 do not matter in the third stage either. All spectral coefficients D(\mu) therefore result in zero with the exception of
- \hspace{0.15 cm}\underline{D(4)} = {1}/{N}\cdot \left[Y(0) - Y(4) \right ] \hspace{0.15 cm}\underline{= 1} \hspace{0.05cm}.
This result agrees with the results from (1) und (2) .
(6) Since both the time coefficients d(ν) and all spectral coefficients D(\mu) are purely real, there is no difference between the FFT and the IFFT.
- This means at the same time: The input and output values can be interchanged.
- Subtask (5) gave the following result:
- d({\rm even}\hspace{0.15cm}\nu) = +1, \hspace{0.2cm}d({\rm odd}\hspace{0.15cm}\nu)= -1
- \Rightarrow \hspace{0.3cm}D(\mu = 4)= 1,\hspace{0.2cm}D(\mu \ne 4)= 0.
- By swapping the input and output values, we arrive at problem (6):
- d(\nu = 4)= 1, \hspace{0.2cm}d(\nu \ne 4)= 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm}D({\rm gerades}\hspace{0.15cm}\mu) = +1, \hspace{0.2cm}D({\rm ungerades}\hspace{0.15cm}\mu)= -1 \hspace{0.05cm}.
- In particular, this results in D(3) \; \underline{= -1} und D(4) \; \underline{= +1}.