Difference between revisions of "Aufgaben:Exercise 1.4: "Pointer diagram" and "Locality Curve""
From LNTwww
m |
|||
| Line 2: | Line 2: | ||
{{quiz-Header|Buchseite=Modulation_Methods/General_Model_of_Modulation}} | {{quiz-Header|Buchseite=Modulation_Methods/General_Model_of_Modulation}} | ||
[[File:P_ID966__Mod_A_1_4_neu.png|right|frame|Vorgegebenes analytisches Signal in der komplexen Ebene]] | [[File:P_ID966__Mod_A_1_4_neu.png|right|frame|Vorgegebenes analytisches Signal in der komplexen Ebene]] | ||
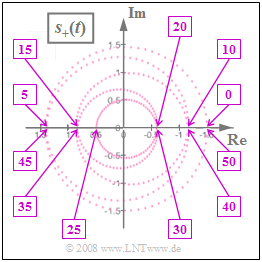
| − | + | The accompanying graph shows the analytical signal $s_+(t)$ in the complex plane. | |
| − | * | + | *The numbers shown in the rectangles indicate the time points in microseconds. |
| − | * | + | *For all multiples of $5 \ \rm µ s$ , $s_+(t)$ is always real with the following values: |
:$$s_+(t = 0) =s_+(t = 50\;{\rm µ s})= 1.500\hspace{0.05cm},$$ | :$$s_+(t = 0) =s_+(t = 50\;{\rm µ s})= 1.500\hspace{0.05cm},$$ | ||
:$$s_+(t = 5\;{\rm µ s}) = s_+(t = 45\;{\rm µ s})= -1.405\hspace{0.05cm},$$ | :$$s_+(t = 5\;{\rm µ s}) = s_+(t = 45\;{\rm µ s})= -1.405\hspace{0.05cm},$$ | ||
| Line 10: | Line 10: | ||
:$$\text{.....................................} $$ | :$$\text{.....................................} $$ | ||
:$$s_+(t = 25\;{\rm µ s}) = -0.500\hspace{0.05cm}.$$ | :$$s_+(t = 25\;{\rm µ s}) = -0.500\hspace{0.05cm}.$$ | ||
| − | + | It is assumed that the corresponding physical signal has the following form: | |
:$$s(t) = A_{\rm T} \cdot \cos \left(\omega_{\rm T}\cdot t\right) + {A_0}/{2}\cdot \cos\left(\left(\omega_{\rm T} + \omega_{\rm 0}\right)\cdot t \right) + {A_0}/{2}\cdot \cos\left(\left(\omega_{\rm T} - \omega_{\rm 0}\right)\cdot t \right)\hspace{0.05cm}.$$ | :$$s(t) = A_{\rm T} \cdot \cos \left(\omega_{\rm T}\cdot t\right) + {A_0}/{2}\cdot \cos\left(\left(\omega_{\rm T} + \omega_{\rm 0}\right)\cdot t \right) + {A_0}/{2}\cdot \cos\left(\left(\omega_{\rm T} - \omega_{\rm 0}\right)\cdot t \right)\hspace{0.05cm}.$$ | ||
| − | * | + | *The frequency of the carrier signal is given as $f_{\rm T} = 100\text{ kHz}$. |
| − | * | + | *The three further parameters $f_0$, $A_{\rm T}$ and $A_0$ are to be determined. |
| − | + | Reference will also be made to the [[Modulation_Methods/Allgemeines_Modell_der_Modulation#Beschreibung_von_s.28t.29_mit_Hilfe_des_analytischen_Signals|equivalent lowpass signal]] $s_{\rm TP}(t)$, with the following relationship to the analytical signal: | |
:$$s_{\rm TP}(t) = s_+(t) \cdot {\rm e}^{-{\rm j}\hspace{0.03cm} \cdot \hspace{0.03cm}\omega_{\rm T}\cdot \hspace{0.05cm}t} \hspace{0.05cm}.$$ | :$$s_{\rm TP}(t) = s_+(t) \cdot {\rm e}^{-{\rm j}\hspace{0.03cm} \cdot \hspace{0.03cm}\omega_{\rm T}\cdot \hspace{0.05cm}t} \hspace{0.05cm}.$$ | ||
| Line 26: | Line 26: | ||
| − | '' | + | ''Hints:'' |
| − | * | + | *This exercise belongs to the chapter [[Modulation_Methods/Allgemeines_Modell_der_Modulation|General Model of Modulation]]. |
| − | * | + | *Particular reference is made to the page [[Modulation_Methods/Allgemeines_Modell_der_Modulation#Beschreibung_des_physikalischen_Signals_mit_Hilfe_des_.C3.A4quivalenten_TP-Signals|Describing the physical signal using the equivalent lowpass signal]]. |
| − | * | + | *You will find further information on these topics in these chapters of the book „Signal Representation”: |
| − | ::(1) [[ Signal_Representation/Harmonic_Oscillation| | + | ::(1) [[ Signal_Representation/Harmonic_Oscillation|Harmonic Oscillation]], |
| − | ::(2) [[Signal_Representation/Analytical_Signal_and_Its_Spectral_Function| | + | ::(2) [[Signal_Representation/Analytical_Signal_and_Its_Spectral_Function|Analytical Signal and its Spectral Function]], |
| − | ::(3) [[Signal_Representation/Equivalent_Low_Pass_Signal_and_Its_Spectral_Function| | + | ::(3) [[Signal_Representation/Equivalent_Low_Pass_Signal_and_Its_Spectral_Function| Equivalent Low-Pass Signal and its Spectral Function]]. |
| − | *In | + | *In our tutorial $\rm LNTwww$, the plot of the analytical signal $s_+(t)$ n the complex plane is sometimes referred to as the "pointer diagram", while the "locus curve" gives the time course of the equivalent lowpass signal $s_{\rm TP}(t)$ . We refer you to the corresponding interactive Applets |
| − | ::(1) [[Applets:Physikalisches_Signal_%26_Analytisches_Signal| | + | ::(1) [[Applets:Physikalisches_Signal_%26_Analytisches_Signal|Physical & Analytic Signal]], |
| − | ::(2) [[Applets:Physikalisches_Signal_%26_Äquivalentes_TP-Signal| | + | ::(2) [[Applets:Physikalisches_Signal_%26_Äquivalentes_TP-Signal|Physical Signal & Equivalent Lowpass Signal]]. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Using $s(t)$, give the equation for $s_+(t)$ and simplify it. Which equation is valid for the equivalent low-pass signal? |
|type="()"} | |type="()"} | ||
- Es gilt $s_{\rm TP}(t) = A_0 · {\rm e}^{–{\rm j}ω_0t}.$ | - Es gilt $s_{\rm TP}(t) = A_0 · {\rm e}^{–{\rm j}ω_0t}.$ | ||
Revision as of 17:19, 11 November 2021
The accompanying graph shows the analytical signal $s_+(t)$ in the complex plane.
- The numbers shown in the rectangles indicate the time points in microseconds.
- For all multiples of $5 \ \rm µ s$ , $s_+(t)$ is always real with the following values:
- $$s_+(t = 0) =s_+(t = 50\;{\rm µ s})= 1.500\hspace{0.05cm},$$
- $$s_+(t = 5\;{\rm µ s}) = s_+(t = 45\;{\rm µ s})= -1.405\hspace{0.05cm},$$
- $$s_+(t = 10\;{\rm µ s}) = s_+(t = 40\;{\rm µ s})= 1.155\hspace{0.05cm},$$
- $$\text{.....................................} $$
- $$s_+(t = 25\;{\rm µ s}) = -0.500\hspace{0.05cm}.$$
It is assumed that the corresponding physical signal has the following form:
- $$s(t) = A_{\rm T} \cdot \cos \left(\omega_{\rm T}\cdot t\right) + {A_0}/{2}\cdot \cos\left(\left(\omega_{\rm T} + \omega_{\rm 0}\right)\cdot t \right) + {A_0}/{2}\cdot \cos\left(\left(\omega_{\rm T} - \omega_{\rm 0}\right)\cdot t \right)\hspace{0.05cm}.$$
- The frequency of the carrier signal is given as $f_{\rm T} = 100\text{ kHz}$.
- The three further parameters $f_0$, $A_{\rm T}$ and $A_0$ are to be determined.
Reference will also be made to the equivalent lowpass signal $s_{\rm TP}(t)$, with the following relationship to the analytical signal:
- $$s_{\rm TP}(t) = s_+(t) \cdot {\rm e}^{-{\rm j}\hspace{0.03cm} \cdot \hspace{0.03cm}\omega_{\rm T}\cdot \hspace{0.05cm}t} \hspace{0.05cm}.$$
Hints:
- This exercise belongs to the chapter General Model of Modulation.
- Particular reference is made to the page Describing the physical signal using the equivalent lowpass signal.
- You will find further information on these topics in these chapters of the book „Signal Representation”:
- In our tutorial $\rm LNTwww$, the plot of the analytical signal $s_+(t)$ n the complex plane is sometimes referred to as the "pointer diagram", while the "locus curve" gives the time course of the equivalent lowpass signal $s_{\rm TP}(t)$ . We refer you to the corresponding interactive Applets
Questions
Musterlösung
(1) Alle Cosinusfunktionen sind in entsprechende komplexe Exponentialfunktionen umzuwandeln:
- $$s_+(t) = A_{\rm T} \cdot {\rm e}^{\hspace{0.03cm}{\rm j} \cdot \hspace{0.03cm}\omega_{\rm T}\cdot \hspace{0.03cm}t} + \frac{A_0}{2}\cdot {\rm e}^{\hspace{0.03cm}{\rm j} \cdot \hspace{0.03cm}(\omega_{\rm T} + \omega_{\rm 0})\hspace{0.03cm}\cdot \hspace{0.05cm}t} + \frac{A_0}{2}\cdot {\rm e}^{\hspace{0.03cm}{\rm j} \cdot \hspace{0.03cm}(\omega_{\rm T} - \omega_{\rm 0})\hspace{0.03cm}\cdot \hspace{0.03cm}t} = {\rm e}^{\hspace{0.03cm}{\rm j} \cdot \hspace{0.03cm}\omega_{\rm T}\cdot \hspace{0.03cm}t} \cdot \left[ A_{\rm T}+ \frac{A_0}{2} \cdot \left( {\rm e}^{\hspace{0.03cm}{\rm j} \cdot \hspace{0.03cm}\omega_{\rm 0}\cdot \hspace{0.05cm}t} + {\rm e}^{\hspace{0.03cm}-{\rm j} \cdot \hspace{0.03cm}\omega_{\rm 0}\cdot \hspace{0.05cm}t}\right)\right]\hspace{0.05cm}.$$
- Mit der Gleichung ${\rm e}^{{\rm j} \hspace{0.05cm}· \hspace{0.05cm}α} + {\rm e}^{-{\rm j} \hspace{0.05cm}·\hspace{0.05cm} α} = 2 · \cos(α)$ folgt weiter:
- $$s_+(t) = {\rm e}^{\hspace{0.03cm}{\rm j} \hspace{0.03cm}\cdot \hspace{0.03cm}\omega_{\rm T}\cdot \hspace{0.03cm}t} \cdot \left[ A_{\rm T}+ {A_0} \cdot \cos (\omega_{\rm 0}\cdot t) \right] \hspace{0.05cm}.$$
- Damit erhält man für das äquivalente Tiefpass–Signal:
- $$s_{\rm TP}(t) = s_+(t) \cdot {\rm e}^{-{\rm j}\hspace{0.03cm} \cdot \hspace{0.03cm}\omega_{\rm T}\cdot \hspace{0.05cm}t} = A_{\rm T}+ {A_0} \cdot \cos (\omega_{\rm 0}\cdot t) \hspace{0.05cm}.$$
Richtig ist also der letzte Lösungsvorschlag.
- Im Kapitel "Hüllkurvendemodulation" des vorliegenden Buches werden wir sehen, dass es sich dabei um die Zweiseitenband–Amplitudenmodulation eines Cosinussignals mit cosinusförmigem Träger handelt.
(2) Die Periodendauer des analytischen Signals $s_+(t)$ beträgt $T_0 = 50$ μs.
- Das physikalische Signal $s(t)$ hat die gleiche Periodendauer.
- Unter der Voraussetzung, dass $f_{\rm T}$ ein ganzzahliges Vielfaches von $f_0$ ist (was stets zu überprüfen ist, aber für dieses Beispiel zutrifft), ergibt sich
- $$f_0 = 1/T_0 \hspace{0.15cm}\underline{ = 20 \ \rm kHz}.$$
(3) Bei den gegebenen Zeitpunkten (Vielfache von $5$ μs) gilt für den komplexen Drehzeiger des Trägers:
- $${\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 2 \pi \cdot \hspace{0.05cm} {100\,{\rm kHz}}\cdot \hspace{0.05cm}(k \hspace{0.05cm}\cdot \hspace{0.05cm} 5\,{\rm \mu s})} = {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}k \hspace{0.03cm} \cdot \hspace{0.05cm} \pi } = \left\{ \begin{array}{c} +1 \\ -1 \\ \end{array} \right. \begin{array}{*{10}c} {\rm{falls}} \\ {\rm{falls}} \\ \end{array}\begin{array}{*{20}c} k \hspace{0.1cm}{\rm gerade} , \\ k \hspace{0.1cm}{\rm ungerade} . \\ \end{array}$$
- Deshalb folgt aus der in der Teilaufgabe (1) berechneten Gleichung:
- $$k = 0 \Rightarrow \hspace{0.2cm} s_{\rm +}(t = 0) = A_{\rm T}+ {A_0} \cdot \cos (\omega_{\rm 0}\cdot 0) = A_{\rm T}+ {A_0} \hspace{0.05cm},$$
- $$k = 5 \Rightarrow \hspace{0.2cm} s_{\rm +}(t = 25\;{\rm µ s}) = - \left[ A_{\rm T}+ {A_0} \cdot \cos (\omega_{\rm 0}\cdot {T_0}/{2}) \right] = -A_{\rm T}+ {A_0} \hspace{0.05cm}.$$
- Ein Vergleich mit der ersten und letzten Gleichung auf dem Angabenblatt zeigt:
- $$ s_{\rm +}(t = 0) = A_{\rm T}+ {A_0}=1.5 \hspace{0.05cm}, $$
- $$ s_{\rm +}(t = 25\;{\rm \mu s}) = -A_{\rm T}+ {A_0} = -0.5 \hspace{0.05cm}.$$
- Daraus erhält man $A_{\rm T} \hspace{0.15cm}\underline{ = 1}$ und $A_0 \hspace{0.15cm}\underline{ = 0.5}$.
(4) Zum Zeitpunkt $t = 15$ μs $(k = 3$, ungerade$)$ gilt:
- $$ s_{\rm +}(t = 15\;{\rm µ s}) = - \left[ 1+ 0.5 \cdot \cos (2 \pi \cdot 20\,{\rm kHz} \cdot 0.015\,{\rm ms}) \right] \hspace{0.05cm} = -1- 0.5 \cdot \cos (108^{\circ})\hspace{0.15cm}\underline {= -0.845} \hspace{0.05cm}.$$
- Dagegen ergibt sich für den Zeitpunkt $t = 20$ μs $(k = 4$, gerade$)$:
- $$ s_{\rm +}(t = 20\;{\rm µ s}) = 1 + 0.5 \cdot \cos (144^{\circ})\hspace{0.15cm}\underline {= 0.595} \hspace{0.05cm}.$$
Bei allen diesen betrachteten Zeitpunkten ist das physikalische Signal $s(t) = {\rm Re}[s_+(t)]$ genau so groß.