Difference between revisions of "Aufgaben:Exercise 3.7Z: Partial Fraction Decomposition"
| Line 21: | Line 21: | ||
Two of the four configurations given are so-called ''all-pass filters''. | Two of the four configurations given are so-called ''all-pass filters''. | ||
*This refers to two-port networks for which the Fourier spectral function satisfies the condition $|H(f)| = 1$ ⇒ $a(f) = 0$ . | *This refers to two-port networks for which the Fourier spectral function satisfies the condition $|H(f)| = 1$ ⇒ $a(f) = 0$ . | ||
| − | *In [[Aufgaben:Exercise_3.4Z:_Various_All-Pass_Filters|Exercise 3.4Z]] | + | *In [[Aufgaben:Exercise_3.4Z:_Various_All-Pass_Filters|Exercise 3.4Z]] it is given how the poles and zeros of such an all-pass filter must be positioned. |
| − | + | Furthermore, in this exercise the $p$–transfer function | |
:$$H_{\rm L}^{(5)}(p) =\frac{p/A}{\left (\sqrt{p/A}+\sqrt{A/p} \right )^2} | :$$H_{\rm L}^{(5)}(p) =\frac{p/A}{\left (\sqrt{p/A}+\sqrt{A/p} \right )^2} | ||
\hspace{0.05cm}$$ | \hspace{0.05cm}$$ | ||
| − | ⇒ " | + | ⇒ "configuration $(5)$" will be examined in more detail, which can be represented by one of the four pole–zero diagrams given in the graph if the parameter $A$ is chosen correctly. |
| Line 33: | Line 33: | ||
| − | '' | + | ''Please note:'' |
*The exercise belongs to the chapter [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform|Inverse Laplace Transform]]. | *The exercise belongs to the chapter [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform|Inverse Laplace Transform]]. | ||
*In particular, reference is made to the page [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform#Partial_fraction_decomposition|Partial fraction decomposition]]. | *In particular, reference is made to the page [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform#Partial_fraction_decomposition|Partial fraction decomposition]]. | ||
| Line 43: | Line 43: | ||
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which of the sketched two-port networks are all-pass filters? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + Configuration $(1)$, |
| − | + | + | + configuration $(2)$, |
| − | - | + | - configuration $(3)$, |
| − | - | + | - configuration $(4)$. |
| − | { | + | {Which quadripole has the transfer function $H_{\rm L}^{(5)}(p)$? |
|type="()"} | |type="()"} | ||
| − | - | + | - Configuration $(1)$, |
| − | - | + | - configuration $(2)$, |
| − | - | + | - configuration $(3)$, |
| − | + | + | + configuration $(4)$. |
| − | { | + | {Compute the function $H_{\rm L}\hspace{0.01cm}'(p)$ after a partial fraction decomposition for configuration '''(1)'''. <br>Enter the function value for $p = 0$ . |
|type="{}"} | |type="{}"} | ||
$H_{\rm L}\hspace{0.01cm}'(p = 0) \ = \ $ { 2 3% } | $H_{\rm L}\hspace{0.01cm}'(p = 0) \ = \ $ { 2 3% } | ||
| − | { | + | {Compute $H_{\rm L}\hspace{0.01cm}'(p)$ for the configuration $(2)$. Which statements are true here? |
|type="[]"} | |type="[]"} | ||
| − | - $H_{\rm L}\hspace{0.01cm}'(p)$ | + | - $H_{\rm L}\hspace{0.01cm}'(p)$ has the same zeros as $H_{\rm L}(p)$. |
| − | + $H_{\rm L}\hspace{0.01cm}'(p)$ | + | + $H_{\rm L}\hspace{0.01cm}'(p)$ has the same poles as $H_{\rm L}(p)$. |
| − | + | + | + The constant factor of $H_{\rm L}\hspace{0.01cm}'(p)$ is $K' = 8$. |
| − | { | + | {Compute $H_{\rm L}\hspace{0.01cm}'(p)$ for the configuration $(3)$. Which statements are true here? |
|type="[]"} | |type="[]"} | ||
| − | - $H_{\rm L}\hspace{0.01cm}'(p)$ | + | - $H_{\rm L}\hspace{0.01cm}'(p)$ has the same zeros as $H_{\rm L}(p)$. |
| − | + $H_{\rm L}\hspace{0.01cm}'(p)$ | + | + $H_{\rm L}\hspace{0.01cm}'(p)$ has the same poles as $H_{\rm L}(p)$. |
| − | - | + | - The constant factor of $H_{\rm L}\hspace{0.01cm}'(p)$ is $K' = 8$. |
| − | { | + | {Compute $H_{\rm L}\hspace{0.01cm}'(p)$ for the configuration $(4)$. Which statements are true here? |
|type="[]"} | |type="[]"} | ||
| − | - $H_{\rm L}\hspace{0.01cm}'(p)$ | + | - $H_{\rm L}\hspace{0.01cm}'(p)$ has the same zeros as $H_{\rm L}(p)$. |
| − | + $H_{\rm L}\hspace{0.01cm}'(p)$ | + | + $H_{\rm L}\hspace{0.01cm}'(p)$ has the same poles as $H_{\rm L}(p)$. |
| − | - | + | - The constant factor of $H_{\rm L}\hspace{0.01cm}'(p)$ is $K' = 8$. |
| Line 90: | Line 90: | ||
===Solution=== | ===Solution=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' The <u> suggested solutions 1 and 2</u> are correct: |
| − | * | + | *According to the criteria given in exercise 3.4Z, there is always an all-pass filter at hand if there is a zero $p_{\rm o} = + A + {\rm j} \cdot B$ in the right half-plane for each pole $p_{\rm x} = - A + {\rm j} \cdot B$ in the left $p$–half-plane. |
*Mit $K = 1$ ist dann die Dämpfungsfunktion $a(f) = 0 \ \rm Np$ ⇒ $|H(f)| = 1$. | *Mit $K = 1$ ist dann die Dämpfungsfunktion $a(f) = 0 \ \rm Np$ ⇒ $|H(f)| = 1$. | ||
*Aus der Grafik auf der Angabenseite erkennt man: Die Konfigurationen $(1)$ und $(2)$ erfüllen genau diese Symmetrieeigenschaften. | *Aus der Grafik auf der Angabenseite erkennt man: Die Konfigurationen $(1)$ und $(2)$ erfüllen genau diese Symmetrieeigenschaften. | ||
| Line 97: | Line 97: | ||
| − | '''(2)''' | + | '''(2)''' The <u> suggested solution 4</u> is correct: |
| − | * | + | *The transfer function $H_{\rm L}^{(5)}(p)$ is also described by configuration $(4)$ as the following calculation shows: |
:$$H_{\rm L}^{(5)}(p) \hspace{0.25cm} = \hspace{0.2cm} \frac{p/A}{(\sqrt{p/A}+\sqrt{A/p})^2} | :$$H_{\rm L}^{(5)}(p) \hspace{0.25cm} = \hspace{0.2cm} \frac{p/A}{(\sqrt{p/A}+\sqrt{A/p})^2} | ||
=\frac{p/A}{{p/A}+2+ {A/p}} | =\frac{p/A}{{p/A}+2+ {A/p}} | ||
Revision as of 08:22, 26 October 2021
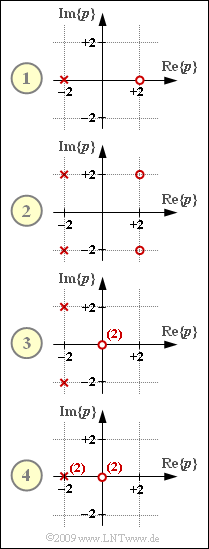
In the graph, four two-port networks are given by their pole–zero diagrams $H_{\rm L}(p)$ .
- They all have in common that the number $Z$ of zeros is equal to the number $N$ of poles.
- The constant factor in each case is $K=1$.
In the special case $Z = N$ the residue theorem cannot be applied directly to compute the impulse response $h(t)$ .
Rather, a partial fraction decomposition corresponding to
- $$H_{\rm L}(p) =1- H_{\rm L}\hspace{0.05cm}'(p) \hspace{0.05cm}$$
must be made beforehand. Then,
- $$h(t) = \delta(t)- h\hspace{0.03cm}'(t) \hspace{0.05cm}$$ holds for the impulse response.
$h\hspace{0.03cm}'(t)$ is the inverse Laplace transform of $H_{\rm L}\hspace{0.05cm}'(p)$ , where the condition $Z' < N'$ is satisfied.
Two of the four configurations given are so-called all-pass filters.
- This refers to two-port networks for which the Fourier spectral function satisfies the condition $|H(f)| = 1$ ⇒ $a(f) = 0$ .
- In Exercise 3.4Z it is given how the poles and zeros of such an all-pass filter must be positioned.
Furthermore, in this exercise the $p$–transfer function
- $$H_{\rm L}^{(5)}(p) =\frac{p/A}{\left (\sqrt{p/A}+\sqrt{A/p} \right )^2} \hspace{0.05cm}$$
⇒ "configuration $(5)$" will be examined in more detail, which can be represented by one of the four pole–zero diagrams given in the graph if the parameter $A$ is chosen correctly.
Please note:
- The exercise belongs to the chapter Inverse Laplace Transform.
- In particular, reference is made to the page Partial fraction decomposition.
Questions
Solution
- According to the criteria given in exercise 3.4Z, there is always an all-pass filter at hand if there is a zero $p_{\rm o} = + A + {\rm j} \cdot B$ in the right half-plane for each pole $p_{\rm x} = - A + {\rm j} \cdot B$ in the left $p$–half-plane.
- Mit $K = 1$ ist dann die Dämpfungsfunktion $a(f) = 0 \ \rm Np$ ⇒ $|H(f)| = 1$.
- Aus der Grafik auf der Angabenseite erkennt man: Die Konfigurationen $(1)$ und $(2)$ erfüllen genau diese Symmetrieeigenschaften.
(2) The suggested solution 4 is correct:
- The transfer function $H_{\rm L}^{(5)}(p)$ is also described by configuration $(4)$ as the following calculation shows:
- $$H_{\rm L}^{(5)}(p) \hspace{0.25cm} = \hspace{0.2cm} \frac{p/A}{(\sqrt{p/A}+\sqrt{A/p})^2} =\frac{p/A}{{p/A}+2+ {A/p}} = \hspace{0.2cm}\frac{p^2}{p^2 + 2A \cdot p + A^2} = \frac{p^2}{(p+A)^2 }= H_{\rm L}^{(4)}(p) \hspace{0.05cm}.$$
- Die doppelte Nullstelle liegt bei $p_{\rm o} = 0$, der doppelte Pol bei $p_{\rm x} = -A = -2$.
(3) Für die Konfiguration $(1)$ gilt:
- $$H_{\rm L}(p) =\frac{p-2}{p+2}=\frac{p+2-4}{p+2}= 1 - \frac{4}{p+2}=1- H_{\rm L}\hspace{-0.05cm}'(p) \hspace{0.3cm} \Rightarrow \hspace{0.3cm}H_{\rm L}\hspace{-0.05cm}'(p) = \frac{4}{p+2} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}\hspace{0.15cm}\underline{H_{\rm L}\hspace{-0.05cm}'(p =0) =2} \hspace{0.05cm}.$$
(4) In gleicher Weise ergibt sich für die Konfiguration $(2)$:
- $$H_{\rm L}(p) =\frac{(p-2 - {\rm j} \cdot 2)(p-2 + {\rm j} \cdot 2)}{(p+2 - {\rm j} \cdot 2)(p+2 + {\rm j} \cdot 2)}= \frac{p^2 -4\cdot p +8 }{p^2 +4\cdot p +8}= \hspace{0.2cm}\frac{p^2 +4\cdot p +8 -8\cdot p}{p^2 +4\cdot p +8} =1- \frac{8\cdot p}{p^2 +4\cdot p +8}=1- H_{\rm L}\hspace{-0.05cm}'(p)$$
- $$\Rightarrow \hspace{0.3cm}H_{\rm L}\hspace{0.05cm}'(p) = 8 \cdot \frac{p}{(p+2 - {\rm j} \cdot 2)(p+2 + {\rm j} \cdot 2)} \hspace{0.05cm}.$$
Richtig sind also die Lösungsvorschläge 2 und 3 im Gegensatz zur Aussage 1:
- Während $H_{\rm L}(p)$ zwei konjugiert–komplexe Nullstellen aufweist,
- besitzt $H_{\rm L}\hspace{0.01cm}'(p)$ nur eine einzige Nullstelle bei $p_{\rm o}\hspace{0.01cm}' = 0$.
(5) Für die Konfiguration $(3)$ gilt:
- $$H_{\rm L}(p) = \frac{p^2 }{p^2 +4\cdot p +8}=\frac{p^2 +4\cdot p +8 -4\cdot p -8 }{p^2 +4\cdot p +8} = 1- H_{\rm L}\hspace{-0.05cm}'(p)$$
- $$\Rightarrow \hspace{0.3cm}H_{\rm L}\hspace{-0.05cm}'(p) = 4 \cdot \frac{p+2}{(p+2 - {\rm j} \cdot 2)(p+2 + {\rm j} \cdot 2)} \hspace{0.05cm}.$$
- Die Nullstelle von $H_{\rm L}\hspace{0.01cm}'(p)$ liegt nun bei $p_{\rm o}\hspace{0.01cm}' = -2$.
- Die Konstante ist $K\hspace{0.01cm}' = 4$ ⇒ richtig ist hier nur der Lösungsvorschlag 2.
(6) Schließlich gilt für die Konfiguration $(4)$:
- $$H_{\rm L}(p) = \frac{p^2 }{(p+2)^2}=\frac{p^2 +4\cdot p +4 -4\cdot p -4 }{p^2 +4\cdot p +4} = 1- \frac{4\cdot p +4 }{p^2 +4\cdot p +4} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}H_{\rm L}\hspace{0.05cm}'(p) = 4 \cdot \frac{p+1}{(p+2)^2} \hspace{0.05cm}.$$
Richtig ist auch hier der Lösungsvorschlag 2. Allgemein lässt sich sagen:
- Durch die Partialbruchzerlegung wird die Anzahl und die Lage der Nullstellen verändert.
- Die Pole von $H_{\rm L}\hspace{0.01cm}'(p)$ sind dagegen stets identisch mit denen von $H_{\rm L}(p)$.