Difference between revisions of "Exercise 2.4Z: Repetition to IDFT"

Revision as of 19:22, 7 March 2023

Five sample sets for $\rm IDFT$

In the Discrete Fourier Transform $\rm (DFT)$ from the time samples $d(\nu) \hspace{0.15cm} {\rm with} \hspace{0.15cm} \nu = 0$, ... , $N - 1$ the discrete spectral coefficients $D(\mu) \hspace{0.15cm} {\rm with} \hspace{0.15cm} \mu = 0$, ... , $N - 1$ calculated as follows:

$$D(\mu) = \frac{1}{N} \cdot \sum_{\nu = 0 }^{N-1} d(\nu)\cdot {w}^{\hspace{0.05cm}\nu \hspace{0.03cm} \cdot \hspace{0.05cm}\mu} \hspace{0.05cm}.$$

Here $w$ abbreviates the complex rotation factor, which is defined as follows:

$$w = {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 2 \pi /N} = \cos \left( {2 \pi}/{N}\right)-{\rm j} \cdot \sin \left( {2 \pi}/{N}\right) \hspace{0.05cm}.$$

Thus, for the Inverse Discrete Fourier Transform $\rm (IDFT)$ as an inverse function of $\rm DFT$:

$$ d(\nu) = \sum_{\mu = 0 }^{N-1} D(\mu) \cdot {w}^{-\nu \hspace{0.03cm} \cdot \hspace{0.05cm}\mu} \hspace{0.05cm}.$$

In this exercise, for different example sequences $D(\mu)$ - which in the above table are denoted by $\boldsymbol{\rm A}$, ... , $\boldsymbol{\rm E}$ - the time coefficients $d(\nu)$ are determined. Thus, it is always $N = 8$.

Hints:

This exercise refers to the theoretical foundations of the chapter "Discrete Fourier Transform" of the book "Signal Representation" and is identical to the one there "Exercise 5.2".

You can check your solution using the interactive applet "Discrete Fourier Transform" .

DFT and IDFT also play a major role in "DSM/DSL" .
In the corresponding chapter, spectral coefficients are denoted by $D_k$ and time samples are denoted by $s_l$. We apologize for this nomenclature discrepancy.
For the two running variables, with the DFT parameter $N = 8$: $0 \le k \le 7, \hspace{0.2cm}0 \le l \le 7 \hspace{0.05cm}.$

Questions

$d(0) \ = \ $

$d(1) \ = \ $

$d(0) \ = \ $

$d(1) \ = \ $

$d(0) \ = \ $

$d(1) \ = \ $

$d(0) \ = \ $

$d(1) \ = \ $

$d(0) \ = \ $

$d(1) \ = \ $

Solution

(1) From the IDFT equation, $D(\mu) = 0$ for $\mu \neq 0$ is obtained for the time coefficients with indices $0 ≤ \nu ≤ 7$:

$$d(\nu) = D(0) \cdot w^0 = D(0) \ = \ 1\hspace{0.3cm} \rightarrow\hspace{0.3cm}\underline{d(0) = d(1) \ = \ 1}.$$

This set of parameters thus describes the discrete form of the Fourier correspondence of the DC signal:

$$x(t) = 1 \hspace{0.2cm}\circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\, \hspace{0.2cm} X(f) = {\delta}(f) \hspace{0.05cm}.$$

(2) Here all spectral coefficients are $0$ except $D_{1} = D_{7} = 0.5$. It follows that for $0 ≤ \nu ≤ 7$:

$$d(\nu) = 0.5 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} + 0.5 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (7\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} \hspace{0.05cm}.$$

However, due to periodicity, the following is also true:

$$d(\nu) \ = \ 0.5 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} + 0.5 \cdot {\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = \cos \left({\pi}/{4} \cdot \nu \right)\hspace{0.3cm} \Rightarrow \hspace{0.3cm}\underline{d(0) = 1, \hspace{0.2cm}d(1) = {1}/{\sqrt{2}} \approx 0.707 \hspace{0.05cm}}.$$

Thus, it is the discrete-time equivalent of

$$x(t) = \cos(2 \pi \cdot f_{\rm A} \cdot t) \hspace{0.2cm}\circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\, \hspace{0.2cm} X(f) = {1}/{2} \cdot {\delta}(f + f_{\rm A}) + {1}/{2} \cdot {\delta}(f - f_{\rm A}) \hspace{0.05cm},$$

where $f_{\rm A}$ denotes the smallest frequency that can be represented in the DFT.

(3) Compared to the subtask (2), the frequency is now twice as large, namely $2 \cdot f_{\rm A}$ instead of $f_{\rm A}$:

$$x(t) = \cos(2 \pi \cdot (2f_{\rm A}) \cdot t) \hspace{0.2cm}\circ\!-\!-\!-\!-\!-\!-\!-\!-\bullet\, \hspace{0.2cm} X(f) = {1}/{2} \cdot {\delta}(f + 2f_{\rm A}) + {1}/{2}\cdot {\delta}(f - 2f_{\rm A}) \hspace{0.05cm},$$

Thus the sequence $〈d(\nu)〉$ describes two periods of the cosine oscillation, and it holds for $0 ≤ \nu ≤ 7$:

$$d(\nu) \ = \ 0.5 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /2) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} + 0.5 \cdot {\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /2) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = \cos \left({\pi}/{2} \cdot \nu \right)\hspace{0.3cm} \Rightarrow \hspace{0.3cm}\underline{d(0) = 1, \hspace{0.2cm}d(1) = 0 }\hspace{0.05cm}.$$

(4) By further doubling the cosine frequency to $4f_{\rm A}$, one finally arrives at the continuous-time Fourier correspondence

$$d(\nu) = 0.5 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} \pi \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} + 0.5 \cdot {\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} \pi \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = \cos \left(\pi \cdot \nu \right) \hspace{0.05cm}$$

and thus to the time coefficients

$$d(0) = d(2) =d(4) =d(6)= +1, \hspace{0.2cm}d(1) =d(3) =d(5) =d(7) = -1 \hspace{0.3cm} \Rightarrow \hspace{0.3cm}\underline{d(0) = +1, \hspace{0.2cm}d(1) = -1 }\hspace{0.05cm}.$$

Note that the two Dirac functions coincide in the discrete-time representation due to periodicity.
That is, The coefficients $D(4) = 0.5$ and $D(-4) = 0.5$ add up to $D(4) = 1$.

(5) The Discrete Fourier Transform is also linear. Therefore, the superposition principle is still applicable.

The coefficients $D(\mu)$ from column $\boldsymbol{\rm E}$ are obtained as the sums of columns $\boldsymbol{\rm A}$ and $\boldsymbol{\rm D}$.
Therefore, the alternating sequence $〈d(\nu)〉$ according to subtask (4) becomes the sequence shifted up by 1:

$$d(0) =d(2) =d(4) =d(6)= 2, \hspace{0.2cm}d(1) =d(3) =d(5) =d(7) = 0\hspace{0.3cm} \Rightarrow \hspace{0.3cm}\underline{d(0) = 2, \hspace{0.2cm}d(1) =0 }\hspace{0.05cm}. $$

@@ Line 1: / Line 1: @@
-{{quiz-Header|Buchseite=Beispiele von Nachrichtensystemen/xDSL als Übertragungstechnik
+{{quiz-Header|Buchseite=Examples_of_Communication_Systems/xDSL_as_Transmission_Technology
 }}
-[[File:P_ID1971__Sig_A_5_2.png|right|frame|Fünf Mustersätze zur&nbsp; $\rm IDFT$]]
+[[File:P_ID1971__Sig_A_5_2.png|right|frame|Five sample sets for&nbsp; $\rm IDFT$]]
-Bei der Diskreten Fouriertransformation&nbsp; $\rm (DFT)$&nbsp; werden aus den Zeitabtastwerten&nbsp; $d(\nu) \hspace{0.15cm}  {\rm mit} \hspace{0.15cm}  \nu = 0$, ... , $N – 1$&nbsp; die diskreten Spektralkoeffizienten&nbsp; $D(\mu) \hspace{0.15cm} {\rm mit} \hspace{0.15cm}  \mu = 0$, ... , $N – 1$&nbsp; wie folgt berechnet:
+In the Discrete Fourier Transform&nbsp; $\rm (DFT)$&nbsp; from the time samples&nbsp; $d(\nu) \hspace{0.15cm} {\rm with} \hspace{0.15cm}  \nu = 0$, ... , $N - 1$&nbsp; the discrete spectral coefficients&nbsp; $D(\mu) \hspace{0.15cm} {\rm with} \hspace{0.15cm}  \mu = 0$, ... , $N - 1$&nbsp; calculated as follows:
 :$$D(\mu) = \frac{1}{N} \cdot \sum_{\nu = 0 }^{N-1} d(\nu)\cdot {w}^{\hspace{0.05cm}\nu \hspace{0.03cm} \cdot \hspace{0.05cm}\mu} \hspace{0.05cm}.$$
-Hierbei ist mit&nbsp; $w$&nbsp; der komplexe Drehfaktor abgekürzt, der folgendermaßen definiert ist:
+Here&nbsp; $w$&nbsp; abbreviates the complex rotation factor, which is defined as follows:
-:$$w = {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 2 \pi /N} = \cos \left( {2 \pi}/{N}\right)-{\rm j} \cdot \sin \left(  {2 \pi}/{N}\right) \hspace{0.05cm}.$$
+:$$w = {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 2 \pi /N} = \cos \left( {2 \pi}/{N}\right)-{\rm j} \cdot \sin \left( {2 \pi}/{N}\right) \hspace{0.05cm}.$$
-Somit gilt für die Inverse Diskrete Fouriertransformation&nbsp; $\rm (IDFT)$&nbsp; als Umkehrfunktion der&nbsp; $\rm DFT$:
+Thus, for the Inverse Discrete Fourier Transform&nbsp; $\rm (IDFT)$&nbsp; as an inverse function of&nbsp; $\rm DFT$:
 :$$ d(\nu) = \sum_{\mu = 0 }^{N-1} D(\mu) \cdot {w}^{-\nu \hspace{0.03cm} \cdot \hspace{0.05cm}\mu} \hspace{0.05cm}.$$
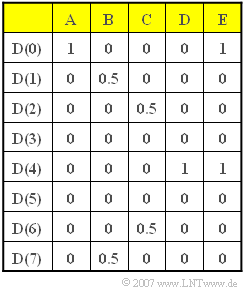
-In dieser Aufgabe sollen für verschiedene Beispielfolgen&nbsp; $D(\mu)$ – die in obiger Tabelle mit&nbsp; $\boldsymbol{\rm A}$, ... , &nbsp;$\boldsymbol{\rm E}$&nbsp; bezeichnet sind – die Zeitkoeffizienten&nbsp; $d(\nu)$&nbsp; ermittelt werden. Es gilt somit stets&nbsp; $N = 8$.
+In this exercise, for different example sequences&nbsp; $D(\mu)$ - which in the above table are denoted by&nbsp; $\boldsymbol{\rm A}$, ... , &nbsp;$\boldsymbol{\rm E}$&nbsp; - the time coefficients&nbsp; $d(\nu)$&nbsp; are determined. Thus, it is always&nbsp; $N = 8$.
@@ Line 21: / Line 21: @@
-''Hinweise:''
+Hints:
-*Die Aufgabe bezieht sich auf die theoretischen Grundlagen des Kapitels&nbsp; [[Signal_Representation/Discrete_Fourier_Transform_(DFT)|Diskrete Fouriertransformation]]&nbsp; des Buches „Signaldarstellung” und ist identisch mit der dortigen&nbsp; [[Aufgaben:Aufgabe_5.2:_Inverse_Diskrete_Fouriertransformation|Aufgabe 5.2]].
+*This exercise refers to the theoretical foundations of the chapter&nbsp; [[Signal_Representation/Discrete_Fourier_Transform_(DFT)|"Discrete Fourier Transform"]]&nbsp; of the book "Signal Representation" and is identical to the one there&nbsp; [[Aufgaben:Exercise_5.2:_Inverse_Discrete_Fourier_Transform|"Exercise 5.2"]].
-*Sie können Ihre Lösung mit dem interaktiven Applet&nbsp;  [[Applets:Diskrete_Fouriertransformation_(Applet)|Diskrete Fouriertransformation]]&nbsp; überprüfen.
+You can check your solution using the interactive applet&nbsp;  [[Applets:Diskrete_Fouriertransformation_(Applet)|"Discrete Fourier Transform"]]&nbsp;.
-*DFT und IDFT spielen auch bei&nbsp; [[Examples_of_Communication_Systems/xDSL_als_Übertragungstechnik#DMT.E2.80.93Realisierung_mit_IDFT.2FDFT|DSM/DSL]]&nbsp; eine große Rolle.
+*DFT and IDFT also play a major role in&nbsp; [[Examples_of_Communication_Systems/xDSL_as_Transmission_Technology#DMT_realization_with_IDFT.2FDFT|"DSM/DSL"]]&nbsp;.
-*Im entsprechenden Kapitel werden die Spektralkoeffizienten mit&nbsp; $D_k$&nbsp; bezeichnet, die Zeitabtastwerte mit&nbsp; $s_l$. Wir bitten, diese Nomenklaturdiskrepanz zu entschuldigen.
+*In the corresponding chapter, spectral coefficients are denoted by&nbsp; $D_k$&nbsp; and time samples are denoted by&nbsp; $s_l$. We apologize for this nomenclature discrepancy.
-*Für die beiden Laufvariablen gelten mit dem DFT–Parameter&nbsp; $N = 8$: &nbsp; $0 \le k \le 7, \hspace{0.2cm}0 \le l \le 7 \hspace{0.05cm}.$
+*For the two running variables, with the DFT parameter&nbsp; $N = 8$: &nbsp; $0 \le k \le 7, \hspace{0.2cm}0 \le l \le 7 \hspace{0.05cm}.$
@@ Line 33: / Line 33: @@
-===Fragebogen===
+===Questions===
 <quiz display=simple>
-Wie lauten die Zeitkoeffizienten&nbsp; $d(\nu)$&nbsp; für&nbsp; $D(\mu)$&nbsp; gemäß Spalte&nbsp; $\boldsymbol{\rm A}$?
+What are the time coefficients&nbsp; $d(\nu)$&nbsp; for&nbsp; $D(\mu)$&nbsp; according to column&nbsp; $\boldsymbol{\rm A}$?
 |type="{}"}
 $d(0) \ = \ ${ 1 }
@@ Line 43: / Line 43: @@
-{Wie lauten die Zeitkoeffizienten&nbsp; $d(\nu)$&nbsp; für&nbsp; $D(\mu)$&nbsp; gemäß Spalte&nbsp; $\boldsymbol{\rm B}$?
+{What are the time coefficients&nbsp; $d(\nu)$&nbsp; for&nbsp; $D(\mu)$&nbsp; according to column&nbsp; $\boldsymbol{\rm B}$?
 |type="{}"}
 $d(0) \ = \ ${ 1 }
 $d(1) \ = \ ${ 0.707 3% }
-{Wie lauten die Zeitkoeffizienten&nbsp; $d(\nu)$&nbsp; für&nbsp; $D(\mu)$&nbsp; gemäß Spalte&nbsp; $\boldsymbol{\rm C}$?
+{What are the time coefficients&nbsp; $d(\nu)$&nbsp; for&nbsp; $D(\mu)$&nbsp; according to column&nbsp; $\boldsymbol{\rm C}$?
 |type="{}"}
 $d(0) \ = \ ${ 1 }
@@ Line 54: / Line 54: @@
-{Wie lauten die Zeitkoeffizienten&nbsp; $d(\nu)$&nbsp; für&nbsp; $D(\mu)$&nbsp; gemäß Spalte&nbsp; $\boldsymbol{\rm D}$?
+{What are the time coefficients&nbsp; $d(\nu)$&nbsp; for&nbsp; $D(\mu)$&nbsp; according to column&nbsp; $\boldsymbol{\rm D}$?
 |type="{}"}
 $d(0) \ = \ ${ 1 }
@@ Line 60: / Line 60: @@
-{Wie lauten die Zeitkoeffizienten&nbsp; $d(\nu)$&nbsp; für&nbsp; $D(\mu)$&nbsp; gemäß Spalte&nbsp; $\boldsymbol{\rm E}$?
+{What are the time coefficients&nbsp; $d(\nu)$&nbsp; for&nbsp; $D(\mu)$&nbsp; according to column&nbsp; $\boldsymbol{\rm E}$?
 |type="{}"}
 $d(0) \ = \ ${ 2 }
@@ Line 68: / Line 68: @@
 </quiz>
-===Musterlösung===
+===Solution===
 {{ML-Kopf}}
-'''(1)'''&nbsp; Aus der IDFT–Gleichung erhält man mit $D(\mu) = 0$ für $\mu \neq 0$ für die Zeitkoeffizienten mit den Indizes&nbsp; $0 ≤ \nu ≤ 7$:
+'''(1)'''&nbsp; From the IDFT equation, $D(\mu) = 0$ for $\mu \neq 0$ is obtained for the time coefficients with indices&nbsp; $0 ≤ \nu ≤ 7$:
-:$$d(\nu) = D(0) \cdot w^0 = D(0) \ = \ 1\hspace{0.3cm} \Rightarrow\hspace{0.3cm}\underline{d(0) = d(1) \ = \ 1}.$$
+:$$d(\nu) = D(0) \cdot w^0 = D(0) \ = \ 1\hspace{0.3cm} \rightarrow\hspace{0.3cm}\underline{d(0) = d(1) \ = \ 1}.$$
-Dieser Parametersatz beschreibt somit die diskrete Form der Fourierkorrespondenz des Gleichsignals:
+This set of parameters thus describes the discrete form of the Fourier correspondence of the DC signal:
 :$$x(t) = 1 \hspace{0.2cm}\circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\, \hspace{0.2cm} X(f) = {\delta}(f) \hspace{0.05cm}.$$
-'''(2)'''&nbsp; Hier sind alle Spektralkoeffizienten $0$ außer $D_{1} = D_{7} = 0.5$. Daraus folgt für $0 ≤ \nu ≤ 7$:
+'''(2)'''&nbsp; Here all spectral coefficients are $0$ except $D_{1} = D_{7} = 0.5$. It follows that for $0 ≤ \nu ≤ 7$:
 :$$d(\nu) = 0.5 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} + 0.5 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (7\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} \hspace{0.05cm}.$$
-Aufgrund der Periodizität gilt aber auch:
+However, due to periodicity, the following is also true:
 :$$d(\nu) \ = \ 0.5 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} + 0.5 \cdot {\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /4) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = \cos \left({\pi}/{4} \cdot \nu \right)\hspace{0.3cm}
   \Rightarrow  \hspace{0.3cm}\underline{d(0) = 1, \hspace{0.2cm}d(1) = {1}/{\sqrt{2}} \approx 0.707 \hspace{0.05cm}}.$$
-Es handelt sich also um das zeitdiskrete Äquivalent zu
+Thus, it is the discrete-time equivalent of
 :$$x(t) = \cos(2 \pi \cdot f_{\rm A} \cdot t) \hspace{0.2cm}\circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\, \hspace{0.2cm} X(f) = {1}/{2} \cdot {\delta}(f + f_{\rm A}) + {1}/{2} \cdot {\delta}(f - f_{\rm A}) \hspace{0.05cm},$$
-wobei $f_{\rm A}$ die kleinste in der DFT darstellbare Frequenz bezeichnet.
+where $f_{\rm A}$ denotes the smallest frequency that can be represented in the DFT.
-'''(3)'''&nbsp; Gegenüber der Teilaufgabe '''(2)''' ist nun die Frequenz doppelt so groß, nämlich $2 \cdot f_{\rm A}$ anstelle von $f_{\rm A}$:
+'''(3)'''&nbsp; Compared to the subtask '''(2)''', the frequency is now twice as large, namely $2 \cdot f_{\rm A}$ instead of $f_{\rm A}$:
-:$$x(t) = \cos(2 \pi \cdot (2f_{\rm A}) \cdot t) \hspace{0.2cm}\circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\, \hspace{0.2cm} X(f) = {1}/{2} \cdot {\delta}(f + 2f_{\rm A}) + {1}/{2}\cdot {\delta}(f - 2f_{\rm A}) \hspace{0.05cm},$$
+:$$x(t) = \cos(2 \pi \cdot (2f_{\rm A}) \cdot t) \hspace{0.2cm}\circ\!-\!-\!-\!-\!-\!-\!-\!-\bullet\, \hspace{0.2cm} X(f) = {1}/{2} \cdot {\delta}(f + 2f_{\rm A}) + {1}/{2}\cdot {\delta}(f - 2f_{\rm A}) \hspace{0.05cm},$$
-Damit beschreibt die Folge $〈d(\nu)〉$ zwei Perioden der Cosinusschwingung, und es gilt für $0 ≤ \nu ≤ 7$:
+Thus the sequence $〈d(\nu)〉$ describes two periods of the cosine oscillation, and it holds for $0 ≤ \nu ≤ 7$:
 :$$d(\nu) \ = \ 0.5 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /2) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} + 0.5 \cdot {\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} (\pi /2) \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = \cos \left({\pi}/{2} \cdot \nu \right)\hspace{0.3cm}
   \Rightarrow  \hspace{0.3cm}\underline{d(0) = 1, \hspace{0.2cm}d(1) = 0 }\hspace{0.05cm}.$$
+'''(4)'''&nbsp; By further doubling the cosine frequency to $4f_{\rm A}$, one finally arrives at the continuous-time Fourier correspondence
-'''(4)'''&nbsp; Durch eine weitere Verdoppelung der Cosinusfrequenz auf $4f_{\rm A}$ kommt man schließlich zur zeitkontinuierlichen Fourierkorrespondenz
 :$$d(\nu) = 0.5 \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} \pi \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} + 0.5 \cdot {\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} \pi \hspace{0.05cm}\cdot \hspace{0.05cm} \nu} = \cos \left(\pi \cdot \nu \right) \hspace{0.05cm}$$
-und damit zu den Zeitkoeffizienten
+and thus to the time coefficients
 :$$d(0) = d(2) =d(4) =d(6)= +1, \hspace{0.2cm}d(1) =d(3) =d(5) =d(7) = -1 \hspace{0.3cm}
-  \Rightarrow  \hspace{0.3cm}\underline{d(0) = +1, \hspace{0.2cm}d(1) = -1 }\hspace{0.05cm}.$$
+  \Rightarrow \hspace{0.3cm}\underline{d(0) = +1, \hspace{0.2cm}d(1) = -1 }\hspace{0.05cm}.$$
-*Zu beachten ist, dass die beiden Diracfunktionen in der zeitdiskreten Darstellung aufgrund der Periodizität zusammenfallen.
+*Note that the two Dirac functions coincide in the discrete-time representation due to periodicity.
-*Das heißt: &nbsp; Die Koeffizienten $D(4) = 0.5$ und $D(-4) = 0.5$ ergeben zusammen $D(4) = 1$.
+*That is, &nbsp; The coefficients $D(4) = 0.5$ and $D(-4) = 0.5$ add up to $D(4) = 1$.
-'''(5)'''&nbsp; Auch die Diskrete Fouriertransformation ist linear. Deshalb ist das Superpositionsprinzip weiterhin anwendbar.
+'''(5)'''&nbsp; The Discrete Fourier Transform is also linear. Therefore, the superposition principle is still applicable.
-*Die Koeffizienten $D(\mu)$ aus Spalte $\boldsymbol{\rm E}$ ergeben sich als die Summen der Spalten $\boldsymbol{\rm A}$ und $\boldsymbol{\rm D}$.
+*The coefficients $D(\mu)$ from column $\boldsymbol{\rm E}$ are obtained as the sums of columns $\boldsymbol{\rm A}$ and $\boldsymbol{\rm D}$.
-*Deshalb wird aus der alternierenden Folge $〈d(\nu)〉$ entsprechend Teilaufgabe '''(4)''' die um 1 nach oben verschobene Folge:
+*Therefore, the alternating sequence $〈d(\nu)〉$ according to subtask '''(4)''' becomes the sequence shifted up by 1:
 :$$d(0) =d(2) =d(4) =d(6)= 2, \hspace{0.2cm}d(1) =d(3) =d(5) =d(7) = 0\hspace{0.3cm}
   \Rightarrow  \hspace{0.3cm}\underline{d(0) = 2, \hspace{0.2cm}d(1) =0 }\hspace{0.05cm}. $$