Aufgaben:Exercise 1.3: System Comparison at AWGN Channel

• Return to book

System Comparison for AWGN Channel

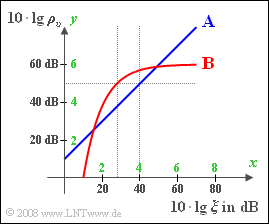
For the comparison of different modulation and demodulation methods with regard to noise sensitivity, we usually start from the so-called AWGN Channel  and present the following double logarithmic diagram:

• The y-axis indicates the sinkt-to-noise ratio (logarithmic SNR)  $10 · \lg ρ_v$  in dB.
•  $10 · \lg ξ$  is plotted on the x-axis; the normalized power parameter ("performance parameter") is characterized by:

$$ \xi = \frac{P_{\rm S} \cdot \alpha_{\rm K}^2 }{{N_0} \cdot B_{\rm NF}}\hspace{0.05cm}.$$

• Thus, the transmission  $P_{\rm S}$, the channel attenuation factor $α_{\rm K}$, the noise power density  $N_0$  and the bandwidth  $B_{\rm NF}$  of the message signal are suitably summarised together in  $ξ$ .
• Unless explicitly stated otherwise, the following values shall be assumed in the exercise:

$$P_{\rm S}= 5 \;{\rm kW}\hspace{0.05cm}, \hspace{0.2cm} \alpha_{\rm K} = 0.001\hspace{0.05cm}, \hspace{0.2cm} {N_0} = 10^{-10}\;{\rm W}/{\rm Hz}\hspace{0.05cm}, \hspace{0.2cm} B_{\rm NF}= 5\; {\rm kHz}\hspace{0.05cm}.$$

Two systems are plotted in the graph and their   $(x, y)$-curve can be described as follows:

• $\text{System A}$  is characterized by the following equation:

$$y = x+1.$$

• Accordingly,  $\text{System B}$ is characterized by:

$$ y= 6 \cdot \left(1 - {\rm e}^{-x+1} \right)\hspace{0.05cm}.$$

The additional axis labels drawn in green have the following meaning:

$$ x = \frac{10 \cdot {\rm lg} \hspace{0.1cm}\xi} {10 \,{\rm dB}}\hspace{0.05cm}, \hspace{0.3cm}y = \frac{10 \cdot {\rm lg} \hspace{0.1cm}\rho_v} {10 \,{\rm dB}}\hspace{0.05cm}.$$

Thus  $x = 4$  represents  $10 · \lg ξ = 40\text{ dB}$  or  $ξ = 10^4$  and  $y = 5$  represents  $10 · \lg ρ_v= 50\text{ dB}$ , i.e.,  $ρ_v = 10^5$.

Hints:

• This exercise belongs to the chapter   Quality Criteria.
• Particular reference is made to the page   Investigating at the AWGN Channel.
• Durch die Angabe der Leistungen in  $\rm W$att sind diese unabhängig vom Bezugswiderstand  $R$.

Quiz

1

Welcher Sinken–Störabstand (in dB) ergibt sich bei  $\text{System A}$  mit  $P_{\rm S}= 5 \;{\rm kW}$,   $\alpha_{\rm K} = 0.001$,   $N_0 = 10^{-10}\;{\rm W}/{\rm Hz}$,   $B_{\rm NF}= 5\; {\rm kHz}$?

$10 · \lg \hspace{0.05cm}ρ_v \ = \ $ $\ \text{dB}$

2

Es wird nun  $10 · \lg \hspace{0.05cm} ρ_v ≥ 60\text{ dB}$  gefordert.  Durch welche Maßnahmen (jeweils für sich allein) ist dies zu erreichen?

	Erhöhung der Sendeleistung von  $P_{\rm S}= 5\text{ kW}$  auf $10\text{ kW}$ .
	Erhöhung des Kanalübertragungsfaktors von  $α_{\rm K} = 0.001$  auf  $0.004$.
	Reduzierung der Rauschleistungsdichte auf  $N_0=10^{–11 }\text{ W/Hz}$.
	Erhöhung der NF–Bandbreite von  $B_{\rm NF}= 5\text{ kHz}$  auf  $\text{ kHz}$.

3

Welcher Störabstand ergibt sich bei  $\text{System B}$  mit  $10 · \lg ξ = 40\text{ dB}$?

$10 · \lg \hspace{0.05cm}ρ_v \ = \ $ $\ \text{dB}$

4

Gefordert wird der Störabstand  $10 · \lg ρ_v = 50\text{ dB}$.  Welche Sendeleistung  $P_{\rm S}$ genügt bei  $\text{System B}$, um diese Qualität zu erzielen?

$P_{\rm S} \ = \ $ $\ \text{ kW }$

5

Für welchen Wert von  $10 · \lg ξ$  ist die Verbesserung von  $\text{System B}$  gegenüber  $\text{System A}$  am größten?

$10 · \lg \hspace{0.05cm} ξ \ = \ $ $\ \text{dB}$

Musterlösung

Solution

(1)  Die normierte Leistungskenngröße ergibt sich mit diesen Werten zu

$$\xi = \frac{5 \cdot 10^3\,{\rm W}\cdot 10^{-6} }{10^{-10}\,{\rm W}/{\rm Hz} \cdot 5 \cdot 10^3\,{\rm Hz}} = 10^4 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg} \hspace{0.1cm}\xi = 40\,{\rm dB} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} x=4 \hspace{0.05cm}.$$

• Damit ergibt sich der Hilfsordinatenwert  $y = 5$, was zum Sinken-Störabstand  $10 · \lg \hspace{0.05cm} ρ_v\hspace{0.15cm}\underline{ = 50 \ \rm dB}$  führt.

(2)  Richtig sind die Alternativen 2 und 3:

Diese Forderung entspricht gegenüber dem bisherigen System einer Erhöhung des Störabstandes um  $10$  dB, so dass auch  $10 · \lg \hspace{0.05cm}ξ$  um  $10$  dB erhöht werden muss:

$$10 \cdot {\rm lg} \hspace{0.1cm}\xi = 50\,{\rm dB} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \xi=10^5 \hspace{0.05cm}.$$

Ein  $10$–fach größerer  $ξ$–Wert wird erreicht – vorausgesetzt die anderen Parameter bleiben jeweils gleich:

• durch die Sendeleistung  $P_{\rm S} = 50$  kW  statt  $5$  kW,
• durch den Kanalübertragungsfaktor  $α_{\rm K} = 0.00316$  anstelle von  $0.001$,
• durch die Rauschleistungsdichte  $N_0 = 10^{ –11 }$  W/Hz  statt  $10^{ –10 }$  W/Hz,
• durch die Bandbreite  $B_{\rm NF} = 0.5$  kHz  statt  $5$  kHz.

(3)  Für  $10 · \lg \hspace{0.05cm} ξ = 40$  dB ist die Hilfsgröße  $x = 4$.  Damit ergibt sich für die Hilfsgröße der Ordinate:

$$y= 6 \cdot \left(1 - {\rm e}^{-3} \right)\approx 5.7 \hspace{0.05cm}.$$

• Dies entspricht dem Sinken–Störabstand  $10 · \lg \hspace{0.05cm} ρ_v\hspace{0.15cm}\underline{ = 57 \ \rm dB}$, also einer Verbesserung gegenüber dem  $\text{System A}$  um  $7$  dB.

(4)  Diese Problemstellung wird durch folgende Gleichung beschrieben:

$$ y= 6 \cdot \left(1 - {\rm e}^{-x+1} \right) = 5 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} {\rm e}^{-x+1} ={1}/{6}\hspace{0.3cm} \Rightarrow \hspace{0.3cm} x = 1+ {\rm ln} \hspace{0.1cm}6 \approx 2.79 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg} \hspace{0.1cm}\xi = 27.9\,{\rm dB}\hspace{0.05cm}.$$

• Bei  $\text{System A}$  war hierfür  $10 · \lg \hspace{0.05cm} \xi = 40$  dB notwendig, was bei den weiter gegebenen Zahlenwerten durch  $P_{\rm S} = 5$  kW erreicht wurde. 
• Nun kann die Sendeleistung um etwa  $12.1$  dB verringert werden:

$$ 10 \cdot {\rm lg} \hspace{0.1cm} \frac{P_{\rm S}}{ 5 \;{\rm kW}}= -12.1\,{\rm dB} \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \frac{P_{\rm S}}{ 5 \;{\rm kW}} = 10^{-1.21}\approx 0.06\hspace{0.05cm}.$$

• Das bedeutet:  Bei  $\text{System B}$  wird mit nur  $6\%$  der Sendeleistung von  $\text{System A}$  – also mit nur  $P_{\rm S} \hspace{0.15cm}\underline{ = 0.3 \ \rm kW}$ – die gleiche Systemqualität erzielt.

(5)  Wir bezeichnen mit  $V$  (steht für "Verbesserung")  den größeren Sinken–Störabstand von  $\text{System B}$  gegenüber  $\text{System A}$ :

$$V = 10 \cdot {\rm lg} \hspace{0.1cm}\rho_v \hspace{0.1cm}{\rm (System\;B)} - 10 \cdot {\rm lg} \hspace{0.1cm}\rho_v \hspace{0.1cm}{\rm (System\;A)} = \left[6 \cdot \left(1 - {\rm e}^{-x+1} \right) -x -1 \right] \cdot 10\,{\rm dB}\hspace{0.05cm}.$$

• Durch Nullsetzen der Ableitung ergibt sich derjenige  $x$–Wert, der zur maximalen Verbesserung führt:

$$ \frac{{\rm d}V}{{\rm d}x} = 6 \cdot {\rm e}^{-x+1} -1\Rightarrow \hspace{0.3cm} x = 1+ {\rm ln} \hspace{0.1cm}6 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg} \hspace{0.1cm}\xi = \hspace{0.15cm}\underline {27.9\,{\rm dB}}\hspace{0.05cm}.$$

• Es ergibt sich also genau der in der Teilaufgabe  (4)  behandelte Fall mit  $10 · \lg ρ_υ = 50$  dB, während der Störabstand bei  $\text{System A}$  nur  $37.9$  dB beträgt. 
• Die Verbesserung ist demnach  $12.1$  dB.