Aufgaben:Exercise 2.13: Quadrature Amplitude Modulation

• Return to book

  $\rm QAM$ model under consideration

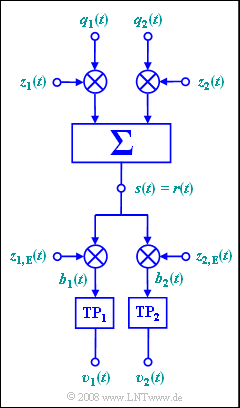
The quadrature amplitude modulation  $\rm (QAM)$  explained by the diagram allows the transmission of two source signals  $q_1(t)$  and  $q_2(t)$  over the same channel, under certain boundary conditions, which are to be determined in this task.

In this exercise, with  $A_1 = A_2 = 2\ \rm V$, let:

$$q_1(t) = A_1 \cdot \cos(2 \pi \cdot f_{\rm 1} \cdot t),$$

$$q_2(t) = A_2 \cdot \sin(2 \pi \cdot f_{\rm 2} \cdot t)\hspace{0.05cm}.$$

For  $ω_{\rm T} = 2π · 25\ \rm kHz$, the four carrier signals shown in the diagram are:

$$z_1(t) = \cos(\omega_{\rm T} \cdot t),$$

$$ z_2(t) = \sin(\omega_{\rm T} \cdot t),$$

$$ z_{1,\hspace{0.05cm}{\rm E}}(t) = 2 \cdot \cos(\omega_{\rm T} \cdot t + \Delta \phi_{\rm T}),$$

$$ z_{2,\hspace{0.05cm}{\rm E}}(t) = 2 \cdot \sin(\omega_{\rm T} \cdot t + \Delta \phi_{\rm T})\hspace{0.05cm}.$$

Both lowpass filters   $\rm TP_1$  and  $\rm TP_2$  with input signals  $b_1(t)$  and  $b_2(t)$ , respectively, remove all frequency components  $|f| > f_{\rm T}$.

Hints:

• This exercise belongs to the chapter  Further AM Variants.
• Particular reference is made to the page  Quadrature Amplitude Modulation (QAM).

• It is worth noting that the carrier signals  $z_2(t)$  and  $z_{2,\hspace{0.05cm}{\rm E}}(t)$  are applied with positive signs here.
• Often – as in the theory section – these carrier signals are given as "minus-sine".
• The following trigonometric transformations are given:

$$ \cos(\alpha) \cdot \cos(\beta) = 1/2 \cdot \big[ \cos(\alpha - \beta)+ \cos(\alpha + \beta) \big],$$

$$ \sin(\alpha) \cdot \sin(\beta) = 1/2 \cdot \big[ \cos(\alpha - \beta)- \cos(\alpha + \beta) \big],$$

$$ \sin(\alpha) \cdot \cos(\beta) = 1/2 \cdot \big[ \sin(\alpha - \beta)+ \sin(\alpha + \beta) \big] \hspace{0.05cm}.$$

Questions

1

Calculate the transmitted signal  $s(t)$  in the case that  $f_1 ≠ f_2$.  Which of the following statements apply?

	$s(t)$  is composed of two cosine and two sine oscillations.
	$s(t)$  is composed of four cosine oscillations.
	$s(t)$  is composed of four sine oscillations.

2

What is  $s(t)$  when  $f_1 = f_2 = 5 \ \rm kHz$.  What signal value arises for  $t = 50 \ \rm µ s$ ?

$s(t = 50 \ \rm µ s) \ = \ $ $\ \rm V$

3

Calculate the sink signals  $v_1(t)$  and  $v_2(t)$ for  $f_1 = f_2$  and  $Δϕ_{\rm T} = 0$  (no phase offset).  Which statements are true?

	$v_1(t) = q_1(t)$  and  $v_2(t) = q_2(t)$ both hold.
	Linear distortions occur.
	Nonlinear distortions occur.

4

Calculate the sink signals  $v_1(t)$  and  $v_2(t)$  for  $f_1 = f_2$  and a phase offset  $Δϕ_{\rm T} = 30^\circ$.  Which statements are true?

	$v_1(t) = q_1(t)$  and  $v_2(t) = q_2(t)$ both hold.
	Linear distortions occur.
	Nonlinear distortions occur.

5

Which of the following statements apply when  $f_1 ≠ f_2$  and  $Δϕ_{\rm T} ≠ 0$  (with an arbitrary phase offset)?

	$v_1(t) = q_1(t)$  and  $v_2(t) = q_2(t)$ both hold.
	Linear distortions occur.
	Nonlinear distortions occur.

Solution

Solution

(1)  Mit den angegebenen trigonometrischen Umformungen erhält man:

$$s(t) = A_1 \cdot \cos(\omega_{\rm 1} \cdot t)\cdot \cos(\omega_{\rm T} \cdot t) + A_2 \cdot \sin(\omega_{\rm 2} \cdot t)\cdot \sin(\omega_{\rm T} \cdot t) $$

$$\Rightarrow \hspace{0.3cm}s(t) = \frac{A_1}{2}\cdot \cos((\omega_{\rm T} - \omega_{\rm 1})\cdot t) + \frac{A_1}{2}\cdot \cos((\omega_{\rm T} + \omega_{\rm 1})\cdot t) + \frac{A_2}{2}\cdot \cos((\omega_{\rm T} - \omega_{\rm 2})\cdot t) - \frac{A_2}{2}\cdot \cos((\omega_{\rm T} + \omega_{\rm 2})\cdot t)\hspace{0.05cm}.$$

• Richtig ist demnach der zweite Lösungsvorschlag.

(2)  Mit $A_1 = A_2 = 2 \ \rm V$ und $f_1 = f_2 = 5\ \rm kHz$ überlagern sich die erste und die dritte Cosinusschwingungen konstruktiv und die beiden anderen heben sich vollständig auf.

• Es ergibt sich somit das folgende einfache Ergebnis:

$$ s(t) = 2\,{\rm V} \cdot \cos(2 \pi \cdot 20\,{\rm kHz} \cdot t) \hspace{0.3cm}\Rightarrow \hspace{0.3cm} s(t = 50\,{\rm µ s}) \hspace{0.15cm}\underline {= 2\,{\rm V}} \hspace{0.05cm}.$$

(3)  Richtig ist der erste Lösungsvorschlag:

• Bei phasensynchroner Demodulation  $(Δϕ_T = 0)$  erhält man für die Signale vor den Tiefpässen gemäß der Teilaufgabe  (2):

$$b_1(t) = 2\,{\rm V} \cdot \cos(\omega_{\rm 20} \cdot t)\cdot 2 \cdot \cos(\omega_{\rm 25} \cdot t) = 2\,{\rm V} \cdot \cos(\omega_{\rm 5} \cdot t) + 2\,{\rm V} \cdot \cos(\omega_{\rm 45} \cdot t),$$

$$ b_2(t) = 2\,{\rm V} \cdot \cos(\omega_{\rm 20} \cdot t)\cdot 2 \cdot \sin(\omega_{\rm 25} \cdot t) = 2\,{\rm V} \cdot \sin(\omega_{\rm 5} \cdot t) + 2\,{\rm V} \cdot \sin(\omega_{\rm 45} \cdot t)\hspace{0.05cm}.$$

• Nach Eliminierung der jeweiligen  $45\ \rm kHz$–Anteile ergibt sich somit  $v_1(t) = q_1(t)$  und  $v_2(t) = q_2(t)$.

(4)  Analog zur Teilaufgabe  (3)  gilt nun:

$$ b_1(t) = 2\,{\rm V} \cdot \cos(\omega_{\rm 20} \cdot t)\cdot 2 \cdot \cos(\omega_{\rm 25} \cdot t+ \Delta \phi_{\rm T})= 2\,{\rm V} \cdot \cos(\omega_{\rm 5} \cdot t + \Delta \phi_{\rm T}) + {(45 \,\rm kHz-Anteil )},$$

$$b_2(t)= 2\,{\rm V} \cdot \cos(\omega_{\rm 20} \cdot t)\cdot 2 \cdot \sin(\omega_{\rm 25} \cdot t+ \Delta \phi_{\rm T})= 2\,{\rm V} \cdot \sin(\omega_{\rm 5} \cdot t + \Delta \phi_{\rm T}) + {(45 \,\rm kHz-Anteil )}\hspace{0.05cm}.$$

• Die Sinkensignale  $v_1(t)$  und  $v_2(t)$  weisen bei dieser Konstellation gegenüber  $q_1(t)$  und  $q_2(t)$  Laufzeiten und damit Phasenverzerrungen auf.
• Diese gehören zur Klasse der linearen Verzerrungen   ⇒   Antwort 2.

(5)  Allgemein gilt für das Empfangssignal:

$$r(t) = s(t) = q_1(t) \cdot \cos(\omega_{\rm T} \cdot t) + q_2(t) \cdot \sin(\omega_{\rm T} \cdot t) \hspace{0.05cm}.$$

Die Multiplikation mit den empfängerseitigen Trägersignalen  $z_{1,\hspace{0.05cm}{\rm E}}(t)$  und  $z_{2,\hspace{0.05cm}{\rm E}}(t)$  und Bandbegrenzung führt zu den Signalen

$$v_1(t) = \cos(\Delta \phi_{\rm T}) \cdot q_1(t) - \sin(\Delta \phi_{\rm T}) \cdot q_2(t),$$

$$ v_2(t) = \sin(\Delta \phi_{\rm T}) \cdot q_1(t) + \cos(\Delta \phi_{\rm T}) \cdot q_2(t) \hspace{0.05cm}.$$

Daraus ist zu ersehen:

• Bei einem Phasenversatz von  $Δϕ_{\rm T} = 30^\circ$  beinhaltet das Sinkensignal  $v_1(t)$  nicht nur das um  $\cos(30^\circ) = 0.866$  gedämpfte Signal  $q_1(t)$, sondern auch die in  $q_2(t)$  enthaltene Frequenz  $f_2$.
• Diese ist mit dem Faktor  $\sin(30^\circ) = 0.5$  gewichtet.
• Es liegen somit nichtlineare Verzerrungen vor   ⇒   Antwort 3.