Exercise 2.2: Distortion Power

Input signal and output signals

A rectangular pulse $x(t)$ with amplitude $1 \hspace{0.08cm} \rm V$ and duration $4 \hspace{0.08cm} \rm ms$ is applied to the input of a communication system. Then, the pulse $y_1(t)$ , whose signal parameters can be taken from the middle sketch, is measured at the system output.

At the output of another system $S_2$ , the signal $y_2(t)$ shown in the lower sketch is obtained with the same input signal $x(t)$ .

Let the following definition apply to the error signal used in this task:

$$\varepsilon(t) = y(t) - \alpha \cdot x(t - \tau) .$$

The parameters $\alpha$ and $\tau$ are to be determined such that the distortion power (the mean squared error) is minimal. For this, the following holds:

$$P_{\rm V} = \overline{\varepsilon^2(t)} = \frac{1}{T_{\rm M}} \cdot \int\limits_{ ( T_{\rm M})} {\varepsilon^2(t) }\hspace{0.1cm}{\rm d}t$$

These definitions already take into account that a frequency-independent damping just as a runtime which is constant for all frequencies does not contribute to the distortion.

The integration interval has to be chosen appropriately in each case:

Use the interval $0$ ... $4 \hspace{0.08cm} \rm ms$ for $y_1(t)$ and the interval $1 \hspace{0.08cm} {\rm ms}$ ... $5 \hspace{0.08cm} \rm ms$ for $y_2(t)$ .
Thus, the measurement time is $T_{\rm M} = 4 \hspace{0.08cm} \rm ms$ in both cases.
It is obvious that with respect to $y_1(t)$ die Parameter $\alpha = 1$ and $\tau = 0$ respectively result in the minimum distortion power.

The so-called signal–zu–Verzerrungs–Leistungsverhältnis berechnet sich im allgemeinen Fall zu

$$\rho_{\rm V} = \frac{ \alpha^2 \cdot P_{x}}{P_{\rm V}} \hspace{0.05cm}.$$

Hierbei bezeichnet

$P_x$ die Leistung des Signals $x(t)$, und
$\alpha^2 \cdot P_x$ die Leistung von $y(t) = \alpha \cdot x(t - \tau)$, die sich bei Abwesenheit von Verzerrungen ergeben würde.

Meist – so auch in dieser Aufgabe – wird dieses S/N-Verhältnis $\rho_{\rm V}$ logarithmisch in $\rm dB$ angegeben.

Please note:

The exercise belongs to the chapter Classification of the Distortions.
In particular, consider the pages

Quantitative measure for the signal distortions and also

Berücksichtigung von Dämpfung und Laufzeit.

Questions

$P_{\rm V1} \ = \ $

$\ \cdot 10^{-3} \ {\rm V}^2$

$10 \cdot {\rm lg} \ \rho_\text{V1} \ = \ $

$\ \rm dB$

$\alpha \ = \ $

$\tau \ = \ $

$\ \rm ms$

$P_{\rm V2} \ = \ $

$\ \cdot 10^{-3} \ {\rm V}^2$

$10 \cdot {\rm lg} \ \rho_\text{V2} \ = \ $

$\ \rm dB$

Solution

Resultierende Fehlersignale

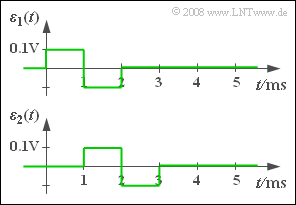
(1) Mit den gegebenen Parametern $\alpha = 1$ und $\tau= 0$ erhält man das in der Grafik dargestellte Fehlersignal $\varepsilon_1(t)$. Die Verzerrungsleistung ist somit gleich:

$$P_{\rm V1} = \frac{ {1 \, \rm ms}}{4 \, \rm ms} \cdot \big[ ({0.1 \, \rm V})^2 + ({-0.1 \, \rm V})^2\big]\hspace{0.3cm}\Rightarrow \hspace{0.3cm}P_{\rm V1} \hspace{0.15cm}\underline{ = 5 \cdot 10^{-3} \, \rm V^2}. $$

(2) Die Leistung des Eingangssignals beträgt:

$$P_{x} = \frac{1}{4 \, \rm ms} \cdot ({1 \, \rm V})^2 \cdot {4 \, \rm ms}\hspace{0.15cm}{ = {1 \, \rm V^2}}.$$

Mit dem Ergebnis aus (1) erhält man somit für das Signal–zu–Verzerrungs–Leistungsverhältnis:

$$\rho_{\rm V1} = \frac{ P_{x}}{P_{\rm V1}}= \frac{ {1 \, \rm V^2}}{0.005 \, \rm V^2}\hspace{0.05cm}\rm = 200\hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm V1}\hspace{0.15cm}\underline{ = {23.01 \, \rm dB}}.$$

(3) Die Skizze auf dem Angabenblatt macht deutlich, dass sich auch ohne die auftretenden Verzerrungen – sondern allein durch Dämpfung und Laufzeit das Signal $y(t)$ von $x(t)$ – deutlich unterscheiden würde.

Es würde sich $y(t) = 0.5 \cdot x(t-1\ {\rm ms}) $ ergeben.

Wenn jemand diese Werte nicht sofort aus der Grafik erkennt, so müsste er für sehr (unendlich) viele $\alpha$– und $\tau$–Werte zunächst das Fehlersignal

$$\varepsilon_2(t) = y_2(t) - \alpha \cdot x(t - \tau)$$

und anschließend den mittleren quadratischen Fehler ermitteln, wobei das Integrationsintervall jeweils an $\tau$ anzupassen ist.

Auch dann würde man das kleinstmögliche Ergebnis für $\alpha \; \underline{= 0.5}$ und $\tau \; \underline{= 1 \ \rm ms}$ erhalten. Für diese Optimierung von $\alpha$ und $\tau$ sollte man sich allerdings schon ein Computerprogramm gönnen.

(4) Die obige Skizze zeigt, dass $\varepsilon_2(t)$ bis auf eine Verschiebung um $1 \ \rm ms$ gleich dem Fehlersignal $\varepsilon_1(t)$ ist. Mit dem Integrationsintervall $1 \ {\rm ms}$ ... $5 \ {\rm ms}$ ergibt sich somit auch die gleiche Verzerrungsleistung:

$$P_{\rm V2} = P_{\rm V1} \hspace{0.15cm}\underline{ = 5 \cdot 10^{-3} \, \rm V^2}.$$

(5) Entsprechend dem Angabenblatt gilt:

$$\rho_{\rm V2} = \frac{ \alpha^2 \cdot P_{x}}{P_{\rm V2}}= \frac{ 0.5^2 \cdot {1 \, \rm V^2}}{0.005 \, \rm V^2}\hspace{0.05cm}\rm = 50\hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm V2} \hspace{0.15cm}\underline{= {16.99 \, \rm dB}}.$$

Trotz gleicher Verzerrungsleistung ist $10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm V2}$ gegenüber $10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm V1}$ um etwa $6 \ \rm dB$ geringer.
Das Signal $y_2(t)$ ist also hinsichtlich des SNR deutlich ungünstiger als $y_1(t)$.
Es ist berücksichtigt, dass nun wegen $\alpha = 0.5$ die Leistung des Ausgangssignals nur noch ein Viertel der Eingangsleistung beträgt.
Würde man diese Dämpfung am Ausgang durch eine Verstärkung um $1/\alpha$ kompensieren, so würde zwar die Verzerrungsleistung um $\alpha^2$ größer.

Das Signal-zu-Verzerrungs-Leistungsverhältnis $\rho_{\rm V2}$ bliebe jedoch erhalten, weil auch das "Nutzsignal" um den gleichen Betrag angehoben wird.