Exercise 2.7Z: DSB-AM and Envelope Demodulator

Spectrum $R_{\rm TP}(f)$ of the received signal in the equivalent low-pass range

Assume a source signal

$$ q(t) = 2 \,{\rm V} \cdot \cos(2 \pi \cdot 2\,{\rm kHz} \cdot t ) + 2 \,{\rm V} \cdot \sin(2 \pi \cdot 5\,{\rm kHz} \cdot t )\hspace{0.05cm}.$$

This is modulated according to the modulation method "DSB-AM with carrier" and transmitted through an ideal channel. The influence of noise can be disregarded.

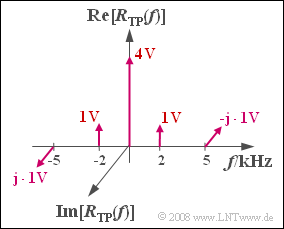
The graph shows the spectrum $R_{\rm TP}(f)$ of the received signal in the equivalent low-pass region, which is composed of Dirac lines at $f = 0$ (originating from the carrier), at $±2\ \rm kHz$ (originating from the cosine component) and at $±5\ \rm kHz$ (originating from the sine component) .

The locus curve is the plot of the equivalent low-pass signal $r_{\rm TP}(t)$ in the complex plane,
where $r_{\rm TP}(t)$ is the Fourier retransform of $R_{\ \rm TP}(f)$ .

Hints:

This exercise belongs to the chapter Envelope Demodulation.
Particular reference is made to the page Description using the equivalent low-pass signal.

Questions

Solution

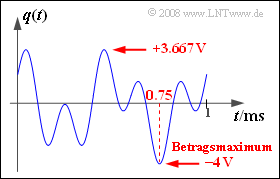
Quellensignal im Bereich bis $1\text{ ms}$

(1) Die Grafik zeigt, dass das Quellensignal alle Werte zwischen $–4 \ \rm V$ und $+3.667\ \rm V$ annehmen kann.

Der maximale Betrag tritt zum Beispiel zum Zeitpunkt $t = t_0 =0.75\ \rm ms$ auf:

$$q(t = t_0) = 2 \,{\rm V} \cdot \cos(2 \pi \cdot 2\,{\rm kHz} \cdot t_0 ) + 2 \,{\rm V} \cdot \sin(2 \pi \cdot 5\,{\rm kHz} \cdot t_0 )$$

$$\Rightarrow \hspace{0.3cm}q(t = 0.75 \,{\rm ms}) = 2 \,{\rm V} \cdot \cos(3 \pi) + 2 \,{\rm V} \cdot \sin(7.5 \pi)= -4 \,{\rm V}\hspace{0.05cm}.$$

Daraus folgt für den maximalen Betrag: $q_{\rm max}\hspace{0.15cm}\underline{ = 4 \ \rm V}$.

(2) In der Angabenseite–Grafik gibt das Gewicht der Diraclinie bei $f = 0$ die Amplitude des zugesetzten Trägers an.

Diese ist $A_{\rm T}\hspace{0.15cm}\underline{ = 4\ \rm V }$.
Daraus erhält man den Modulationsgrad $m = q_{\rm max}/A_{\rm T} \hspace{0.15cm}\underline{ = 1}$.

(3) Richtig sind die Lösungsvorschläge 2 und 3:

Da der Modulationsgrad nicht größer als $m = 1$ ist, führt auch der Hüllkurvendemodulator nicht zu Verzerrungen.
Der wesentliche Vorteil der Hüllkurvendemodulation ist, dass keine Frequenz– und Phasensynchronität notwendig ist.
Nachteilig ist, dass im Gegensatz zur Synchrondemodulation beim Sender eine deutlich höhere Leistung aufgebracht werden muss.
Bei $m = 1$ ergibt sich gegenüber der ZSB–AM ohne Träger die dreifache Sendeleistung.

Äquivalentes Tiefpass–Signal
in der komplexen Ebene

(4) Richtig sind die Lösungsvorschläge 1 und 3:

Mit $ω_2 = 2 π · 2 \ \rm kHz$ und $ω_5 = 2 π · \ \rm 5 kHz$ gilt:

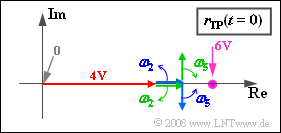
$$ r_{\rm TP}(t) = 4 \,{\rm V} \hspace{-0.05cm}+\hspace{-0.05cm} 1 \,{\rm V} \cdot {\rm e}^{{\rm j} \cdot \hspace{0.03cm}\omega_{\rm 2}\cdot \hspace{0.03cm}t} \hspace{-0.05cm}+\hspace{-0.05cm} 1 \,{\rm V} \cdot {\rm e}^{-{\rm j} \cdot \hspace{0.03cm}\omega_{\rm 2}\cdot \hspace{0.03cm}t} \hspace{-0.05cm}-\hspace{-0.05cm} \hspace{0.15cm}{\rm j} \cdot1 \,{\rm V} \cdot {\rm e}^{{\rm j} \cdot \hspace{0.03cm}\omega_{\rm 5}\cdot \hspace{0.03cm}t} \hspace{-0.05cm}+\hspace{-0.05cm} {\rm j} \cdot1 \,{\rm V} \cdot {\rm e}^{-{\rm j} \cdot \hspace{0.03cm}\omega_{\rm 5}\cdot \hspace{0.03cm}t} \hspace{0.05cm}. \hspace{0.1cm}$$

Bei der Konstruktion der Ortskurve $r_{TP}(t)$ sind somit genau fünf Zeiger zu berücksichtigen ⇒ Antwort 1 ist richtig. Die Grafik zeigt eine Momentaufnahme zum Zeitpunkt $t = 0$.

Der (rote) Träger ist für alle Zeiten durch den reellen Zeiger der Länge $4 \ \rm V$ gegeben. Im Gegensatz zum Zeigerdiagramm (Darstellung des analytischen Signals) dreht dieser nicht ⇒ Antwort 2 ist falsch.
Die dritte Aussage ist ebenso wie die Aussage 1 richtig: Die Drehzeiger bei negativen Frequenzen drehen in mathematisch negativer Richtung (im Uhrzeigersinn) im Gegensatz zu den beiden Zeigern mit $f > 0$.
Die letzte Aussage trifft nicht zu. Je größer die Frequenz $f$ ist, um so schneller dreht der zugehörige Zeiger.

Ortskurve für verzerrungsfreie Hüllkurvendemodulation

(5) Richtig sind die Aussagen 1 und 2:

Im betrachteten Beispiel kann für das äquivalente TP–Signal auch geschrieben werden:

$$r_{\rm TP}(t) = q(t) + A_{\rm T} \hspace{0.05cm}.$$

Damit ist offensichtlich, dass $r_{\rm TP}(t)$ stets reell ist. Aus den Teilaufgaben (1) und (2) folgt zudem $r_{\rm TP}(t) ≥ 0$.

Das bedeutet:

Die Ortskurve ist hier eine horizontale Gerade auf der reellen Gerade und liegt stets in der rechten Halbebene.
Dies sind die beiden notwendigen Bedingungen, dass mit einem Hüllkurvendemodulator das Nachrichtensignal verzerrungsfrei wiedergewonnen werden kann.
Ist eine dieser Voraussetzungen nicht erfüllt, so kommt es zu nichtlinearen Verzerrungen, nicht zu linearen ⇒ Antwort 3 ist falsch.

	The locus curve $r_{\rm TP}(t)$ is composed of five pointers.
	The carrier rotates with a rotation speed $ω_{\rm T}$.
	The rotational pointers of the negative frequencies rotate clockwise.
	The pointer for $2 \ \rm kHz$ rotates twice as fast as the one for $5 \ \rm kHz$.

	With the envelope demodulator, distortion-free demodulation is not possible in the example considered.
	One can do without frequency and phase synchronization.
	A smaller transmit power would be needed using a synchronous demodulator.

	A distortionless demodulation is only possible when $r_{\rm TP}(t)$ is real at all times.
	A distortionless demodulation is only possible when $r_{\rm TP}(t)$ does not become negative at any point in time.
	If the first two conditions mentioned are not met, linear distortions will occur.