Exercise 2.7Z: DSB-AM and Envelope Demodulator

Spectrum $R_{\rm TP}(f)$ of the received signal in the equivalent low-pass range

Assume a source signal

$$ q(t) = 2 \,{\rm V} \cdot \cos(2 \pi \cdot 2\,{\rm kHz} \cdot t ) + 2 \,{\rm V} \cdot \sin(2 \pi \cdot 5\,{\rm kHz} \cdot t )\hspace{0.05cm}.$$

This is modulated according to the modulation method "DSB-AM with carrier" and transmitted through an ideal channel. The influence of noise can be disregarded.

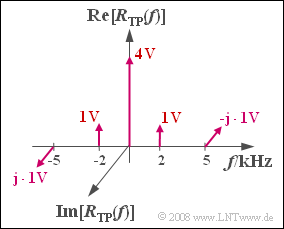
The graph shows the spectrum $R_{\rm TP}(f)$ of the received signal in the equivalent low-pass region, which is composed of Dirac lines

at $f = 0$ (originating from the carrier),
at $±2\ \rm kHz$ (originating from the cosine component) and
at $±5\ \rm kHz$ (originating from the sine component).

The locus curve is the plot of the equivalent low-pass signal $r_{\rm TP}(t)$ in the complex plane, where $r_{\rm TP}(t)$ is the Fourier retransform of $R_{\ \rm TP}(f)$ .

Hints:

This exercise belongs to the chapter Envelope Demodulation.
Particular reference is made to the section Description using the equivalent low-pass signal.

Questions

Solution

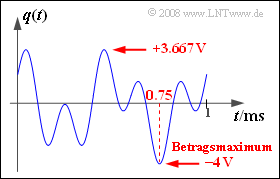
Source signal in the region up to $1\text{ ms}$

(1) The graph shows that the source signal can take on all values between $–4 \ \rm V$ and $+3.667\ \rm V$.

For example, the maximum magnitude occurs at time $t = t_0 =0.75\ \rm ms$:

$$q(t = t_0) = 2 \,{\rm V} \cdot \cos(2 \pi \cdot 2\,{\rm kHz} \cdot t_0 ) + 2 \,{\rm V} \cdot \sin(2 \pi \cdot 5\,{\rm kHz} \cdot t_0 )$$

$$\Rightarrow \hspace{0.3cm}q(t = 0.75 \,{\rm ms}) = 2 \,{\rm V} \cdot \cos(3 \pi) + 2 \,{\rm V} \cdot \sin(7.5 \pi)= -4 \,{\rm V}\hspace{0.05cm}.$$

From this, it follows for the maximum magnitude: $q_{\rm max}\hspace{0.15cm}\underline{ = 4 \ \rm V}$.

(2) In the graph on the information page, the weight of the Dirac line at $f = 0$ indicates the amplitude of the added carrier. This is $A_{\rm T}\hspace{0.15cm}\underline{ = 4\ \rm V }$.

From this, we get the modulation depth $m = q_{\rm max}/A_{\rm T} \hspace{0.15cm}\underline{ = 1}$.

(3) Answers 2 and 3 are correct:

Since the modulation depth is not greater than $m = 1$, the envelope demodulator does not cause distortion either.
The main advantage of envelope demodulation is that no frequency and phase synchronization is necessary.
A disadvantage is that a significantly higher power must be applied at the transmitter relative to synchronous demodulation.
When $m = 1$, this results in three times the transmit power compared to DSB-AM without a carrier.

Equivalent low-pass signal
in the complex plane

(4) Answers 1 and 3 are correct: When $ω_2 = 2 π · 2 \ \rm kHz$ and $ω_5 = 2 π · \ \rm 5 kHz$:

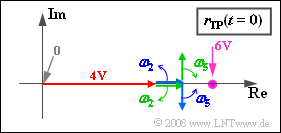
$$ r_{\rm TP}(t) = 4 \,{\rm V} \hspace{-0.05cm}+\hspace{-0.05cm} 1 \,{\rm V} \cdot {\rm e}^{{\rm j} \cdot \hspace{0.03cm}\omega_{\rm 2}\cdot \hspace{0.03cm}t} \hspace{-0.05cm}+\hspace{-0.05cm} 1 \,{\rm V} \cdot {\rm e}^{-{\rm j} \cdot \hspace{0.03cm}\omega_{\rm 2}\cdot \hspace{0.03cm}t} \hspace{-0.05cm}-\hspace{-0.05cm} \hspace{0.15cm}{\rm j} \cdot1 \,{\rm V} \cdot {\rm e}^{{\rm j} \cdot \hspace{0.03cm}\omega_{\rm 5}\cdot \hspace{0.03cm}t} \hspace{-0.05cm}+\hspace{-0.05cm} {\rm j} \cdot1 \,{\rm V} \cdot {\rm e}^{-{\rm j} \cdot \hspace{0.03cm}\omega_{\rm 5}\cdot \hspace{0.03cm}t} \hspace{0.05cm}. \hspace{0.1cm}$$

Thus, in constructing the locus $r_{TP}(t)$, there are exactly five pointers to consider ⇒ answer 1 is correct. The graph shows a snapshot at time $t = 0$.

The (red) carrier is given by the real pointer of length $4 \ \rm V$ for all time points. In contrast to the pointer diagram (showing the analytic signal), this does not rotate ⇒ Answer 2 is false.
The third statement is similarly correct: The rotating pointers at negative frequencies rotate in mathematically negative direction ("clockwise") in contrast to the two pointers with $f > 0$.
The last statement is false. The larger the frequency $f$, the faster the associated pointer rotates.

Locus curve for
distortionless envelope demodulation

(5) Statements 1 and 2 are correct:

In the example considered, the equivalent low-pass signal can be written as:

$$r_{\rm TP}(t) = q(t) + A_{\rm T} \hspace{0.05cm}.$$

Thus, it is obvious that $r_{\rm TP}(t)$ is always real.
Moreover, it follows from subtasks (1) and (2) that $r_{\rm TP}(t) ≥ 0$.

This means:

Here, the locus curve is a horizontal line on the real plane and always lies in the right half-plane.
These are the two necessary conditions for an envelope demodulator to recover the signal without distortion.
If one of these conditions is not satisfied, nonlinear distortions arise, not linear ones ⇒ Answer 3 is wrong.

	The locus curve $r_{\rm TP}(t)$ is composed of five pointers.
	The carrier rotates with a rotation speed $ω_{\rm T}$.
	The rotational pointers of the negative frequencies rotate clockwise.
	The pointer for $2 \ \rm kHz$ rotates twice as fast as the one for $5 \ \rm kHz$.

	With the envelope demodulator, distortion-free demodulation is not possible in the example considered.
	One can do demodulation without frequency and phase synchronization.
	A smaller transmission power would be needed using a synchronous demodulator.

	A distortionless demodulation is only possible when $r_{\rm TP}(t)$ is real at all times.
	A distortionless demodulation is only possible when $r_{\rm TP}(t)$ does not become negative at any point in time.
	If the first two conditions mentioned are not met, linear distortions will occur.