Exercise 2.6: GF(P power m). Which P, which m?
From LNTwww
Additions– und Multiplikationstabelle
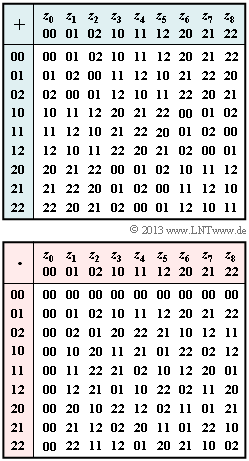
Es soll ein Galoisfeld ${\rm GF}(q)$ mit $q = P^m$ Elementen analysiert werden, das durch die nebenstehenden Tabellen für Addition (gekennzeichnet mit „$+$”) und Multiplikation (gekennzeichnet mit „$\cdot$”) vorgegeben ist. Dieses Galoisfeld
- $${\rm GF}(q) = \{\hspace{0.1cm}z_0,\hspace{0.1cm} z_1,\hspace{0.1cm} ... , \hspace{0.1cm}z_{q-1}\}$$
erfüllt alle Anforderungen an einen endlichen Körper, die im Kapitel 2.1 aufgeführt sind. Kommutativ–, Assoziativ– und Distributivgesetz werden erfüllt. Weiterhin gibt es
- ein neutrales Element hinsichtlich Addition ⇒ $N_{\rm A}$:
- $$\exists \hspace{0.15cm} z_j \in {\rm GF}(q) : \hspace{0.25cm}z_i + z_j = z_i $$
- $$\hspace{0.25cm} \Rightarrow \hspace{0.25cm} z_j = N_{\rm A} = {\rm "0"} \hspace{0.25cm}{\rm (Nullelement)} \hspace{0.05cm},$$
- ein neutrales Element hinsichtlich Multiplikation ⇒ $N_{\rm M}$:
- $$\exists \hspace{0.15cm} z_j \in {\rm GF}(q) : \hspace{0.25cm}z_i \cdot z_j = z_i $$
- $$ \Rightarrow \hspace{0.25cm} z_j = N_{\rm M} = {\rm "1"} \hspace{0.25cm}{\rm (Einselement)} \hspace{0.05cm},$$
- für alle Elemente $z_i$ eine additive Inverse ⇒ ${\rm Inv_A}(z_i)$:
- $$\forall \hspace{0.15cm} z_i \in {\rm GF}(q)\hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_A}(z_i) \in {\rm GF}(q):$$
- $$z_i + {\rm Inv_A}(z_i) = N_{\rm A} = {\rm "0"}\hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm kurz:}\hspace{0.15cm} {\rm Inv_A}(z_i) = - z_i \hspace{0.05cm}, $$
- für alle Elemente $z_i$ mit Ausnahme des Nullelements eine multiplikative Inverse ⇒ ${\rm Inv_M}(z_i)$:
- $$\forall \hspace{0.15cm} z_i \in {\rm GF}(q),\hspace{0.15cm} z_i \ne N_{\rm A} \hspace{0.15cm} \exists \hspace{0.15cm} {\rm Inv_M}(z_i) \in {\rm GF}(q):$$
- $$z_i \cdot {\rm Inv_M}(z_i) = N_{\rm M} = {\rm "1"} \hspace{0.25cm} \Rightarrow \hspace{0.25cm}{\rm kurz:}\hspace{0.15cm} {\rm Inv_M}(z_i) = z_i^{-1} \hspace{0.05cm}. $$
Hinweise:
- Die Aufgabe bezieht sich auf das Kapitel Erweiterungskörper.
- In den Tabellen sind die Elemente $z_0, \ ... \ , \ z_8$ als Koeffizientenvektoren bezeichnet. So steht zum Beispiel „$21$” für die ausführliche Schreibweise $2 \cdot \alpha + 1$.
- Sollte die Eingabe des Zahlenwertes „0” erforderlich sein, so geben Sie bitte „0.” ein.
Fragebogen
Musterlösung
(1)
(2)
(3)
(4)
(5)
