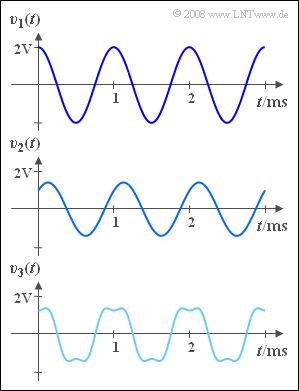
Exercise 1.2: Distortions? Or no Distortion?

Observed Sink signals for the
given input signal $q(t)$

The communication systems $S_1$, $S_2$ and $S_3$ analyzed in terms of the distortions they cause. For this purpose, the cosine-shaped test signal with signal frequency $f_{\rm N} = 1\text{ kHz}$ is applied to the input of each system:

$$q(t) = 2 \;{\rm V} \cdot \cos(2 \pi f_{\rm N} t )$$

The three signals at the system output are measured, as shown in the graph:

$$v_1(t) = 2 \;{\rm V} \cdot \cos(2 \pi f_{\rm N} t )\hspace{0.05cm},$$

$$v_2(t) = 1 \;{\rm V} \cdot \cos(2 \pi f_{\rm N} t + 1 \;{\rm V} \cdot \sin(2 \pi f_{\rm N} t) \hspace{0.05cm},$$

$$v_3(t)= 1.5 \;{\rm V} \cdot \cos(2 \pi f_{\rm N} t) - 0.3 \;{\rm V} \cdot \cos(6 \pi f_{\rm N} t)\hspace{0.05cm}.$$

The noise components that are always present in practice will be assumed to be negligible here.
Hints:

This exercise belongs to the chapter Quality criteria. Particular reference is made to the page Signal-to-noise power ratio and to the chapter Non-linear distortions in the book "Linear and Time-Invariant Systems".
For nonlinear distortion, the sink SNR is $ρ_v = 1/K^2$, where the distortion factor $K$ is the ratio of the rms values of all harmonics to the rms value of the fundamental frequency.

Questions

Solution

(1)&nbspAnswers 1, 2 and 3 are correct:

System $S_1$ could well be an ideal system, namely if for all frequencies $f_{\rm N}$ the condition $v(t) = q(t)$ were satisfied.
The second alternative is also possible, since the ideal system is a special case of distortion-free systems.
However, if at a different message frequency $f_{\rm N} \ne 1$ kHz the condition $v(t) = q(t)$ were not satisfied, then a linearly distorting system would exist whose frequency response would happen to be equal to $1$ at frequency $f_{\rm N}$ .
In contrast, a nonlinearly distorting system (Answer 4) can be excluded due to the lack of harmonics.

(2) Entsprechend den Ausführungen im Kapitel „Harmonische Schwingung” im Buch „Signaldarstellung” gelten folgende Gleichungen:

$$A \cdot \cos(\omega_{\rm N} t ) + B \cdot \sin(\omega_{\rm N} t ) = C \cdot \cos(\omega_{\rm N} t - \varphi)\hspace{0.3cm} \Rightarrow \hspace{0.3cm} C = \sqrt{A^2 + B^2},\hspace{0.5cm}\varphi ={\rm arctan}\hspace{0.1cm} ({A}/{B})\hspace{0.05cm}$$

Angewandt auf das vorliegende Beispiel erhält man

$$C = \sqrt{(1 \,{\rm V})^2 + (1 \,{\rm V})^2}= 1.414\,{\rm V}\hspace{0.05cm}.$$

Der Dämpfungsfaktor des Systems hat somit den Wert $α = 1.414/2 \hspace{0.15cm}\underline{= 0.707}$, und für die Phase gilt:

$$ \varphi ={\rm arctan}\hspace{0.1cm}\frac {1 \,{\rm V}}{1 \,{\rm V}} = 45^{\circ} = {\pi}/{4}\hspace{0.05cm}.$$

Die Umformung $\cos(\omega_{\rm N} t - \varphi)= \cos[\omega_{\rm N} (t - \tau)]$ erlaubt Aussagen über die Laufzeit:

$$\tau =\frac {\varphi}{2\pi f_{\rm N}} = \frac {\pi /4}{2\pi f_{\rm N}} = \frac {1}{8 \cdot 1 \,{\rm kHz}} \hspace{0.15cm}\underline {= 125\,{\rm µ s}}\hspace{0.05cm}.$$

(3) Richtig sind die Lösungsvorschläge 2 und 3:

Das System $S_2$ ist nach den Ausführungen zur Teilaufgabe (1) weder ideal noch nichtlinear verzerrend.
Dagegen sind die Alternativen 2 und 3 möglich, je nachdem, ob die berechneten Werte von $α$ und $τ$ für alle Frequenzen erhalten bleiben oder nicht.
Mit einer einzigen Messung bei nur einer Frequenz kann allerdings diese Frage nicht geklärt werden.

(4) Das Signal $v_3(t)$ beinhaltet eine Oberwelle dritter Ordnung. Deshalb ist die Verzerrung nichtlinear ⇒ Lösungsvorschlag 2.

(5) Mit den Amplituden $A_1 = 1.5 \ \rm V$ und $A_3 = -0.3\ \rm V$ erhält man für den Klirrfaktor:

$$ K_3 =\frac {|A_3|}{|A_1|} = 0.2\hspace{0.05cm}.$$

Deshalb beträgt das Sinken–SNR entsprechend der angegebenen Gleichung $ρ_{v3} = 1/K_3^{ 2 } = 25$.

Zum gleichen Ergebnis kommt man nach der allgemeinen Berechnung.

Aus den Amplituden von Quellensignal und Grundwelle des Sinkensignals erhält man für den frequenzunabhängigen Dämpfungsfaktor:

$$ \alpha =\frac {1.5 \,{\rm V}}{2 \,{\rm V}} = 0.75\hspace{0.05cm}.$$

Das von den nichtlinearen Verzerrungen herrührende Fehlersignal lautet deshalb:

$$\varepsilon_3(t) = v_3(t) - \alpha \cdot q(t) = - 0.3 \,{\rm V} \cdot \cos(6 \pi f_{\rm N} t)\hspace{0.05cm}.$$

Damit ergibt sich die Verzerrungsleistung:

$$P_{\varepsilon 3}= {1}/{2} \cdot (0.3 \,{\rm V})^2 = 0.045 \,{\rm V}^2\hspace{0.05cm}.$$

Mit der Leistung des Quellensignals,

$$P_{q}= {1}/{2} \cdot (2\,{\rm V})^2 = 2 \,{\rm V}^2\hspace{0.05cm},$$

erhält man unter Berücksichtigung des gerade berechneten Dämpfungsfaktors $ \alpha = 0.75 $:

$$\rho_{v3} = \frac{\alpha^2 \cdot P_{q}}{P_{\varepsilon 3}} = \frac{0.75^2 \cdot 2 {\rm V}^2}{0.045 } \hspace{0.15cm}\underline {= 25}\hspace{0.05cm}.$$

	$S_1$ could be an ideal system.
	$S_1$ could be a distortionless system.
	$S_1$ could be a linearly distorting system.
	$S_1$ could be a nonlinearly distorting system.

	$S_2$ could be an ideal system.
	$S_2$ could be a distortionless system.
	$S_2$ could be a linearly distorting system.
	$S_2$ could be a nonlinearly distorting system.

	They are linear distortions.
	They are nonlinear distortions.