Properties of Coaxial Cables

Complex propagation function of coaxial cables

Coaxial cables consist of an inner conductor and - separated by a dielectric - an outer conductor. Two different types of cable have been standardized, with the diameters of the inner and outer conductors mentioned for identification purposes:

the standard coaxial cable whose inner conductor has a diameter of $\text{2.6 mm}$ and whose outer diameter is $\text{9.5 mm}$ ,
the small coaxial cable with dimensions $\text{1.2 mm}$ and $\text{4.4 mm}$.

The cable frequency response $H_{\rm K}(f)$ results from the cable length $l$ and the complex propagation function (per unit length)

$$\gamma(f) = \alpha_0 + \alpha_1 \cdot f + \alpha_2 \cdot \sqrt {f}+ {\rm j}\cdot (\beta_1 \cdot f + \beta_2 \cdot \sqrt {f})\hspace{0.05cm}\hspace{0.3cm} \Rightarrow \hspace{0.3cm}H_{\rm K}(f) = {\rm e}^{-\gamma(f)\hspace{0.05cm} \cdot \hspace{0.05cm} l} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}|H_{\rm K}(f)| = {\rm e}^{-\alpha(f)\hspace{0.05cm} \cdot \hspace{0.05cm} l}\hspace{0.05cm}.$$

The cable specific constants for the standard coaxial cable $\text{(2.6/9.5 mm)}$ are:

$$\begin{align*}\alpha_0 & = 0.00162\, \frac{ {\rm Np} }{ {\rm km} }\hspace{0.05cm}, \hspace{0.2cm} \alpha_1 = 0.000435\, \frac{ {\rm Np} }{ {\rm km \cdot MHz} }\hspace{0.05cm}, \hspace{0.2cm} \alpha_2 = 0.2722\, \frac{ {\rm Np} }{ {\rm km \cdot \sqrt{MHz} } }\hspace{0.05cm}, \\ \beta_1 & = 21.78\, \frac{ {\rm rad} }{ {\rm km \cdot MHz} }\hspace{0.05cm}, \hspace{0.2cm} \beta_2 = 0.2722\, \frac{ {\rm rad} }{ {\rm km \cdot \sqrt{MHz} } } \hspace{0.05cm}.\end{align*}$$

Accordingly, the kilometric attenuation and phase constants for the small coaxial cable $\text{(1.2/4.4 mm)}$:

$$\begin{align*}\alpha_0 & = 0.00783\, \frac{ {\rm Np} }{ {\rm km} }\hspace{0.05cm}, \hspace{0.2cm} \alpha_1 = 0.000443\, \frac{ {\rm Np} }{ {\rm km \cdot MHz} }\hspace{0.05cm}, \hspace{0.2cm} \alpha_2 = 0.5984\, \frac{ {\rm Np} }{ {\rm km \cdot \sqrt{MHz} } }\hspace{0.05cm}, \\ \beta_1 & = 22.18\, \frac{ {\rm rad} }{ {\rm km \cdot MHz} }\hspace{0.05cm}, \hspace{0.2cm} \beta_2 = 0.5984\, \frac{ {\rm rad} }{ {\rm km \cdot \sqrt{MHz} } } \hspace{0.05cm}.\end{align*}$$

These values can be calculated from the geometric dimensions of the cables and have been confirmed by measurements at the Fernmeldetechnisches Zentralamt in Darmstadt - see [Wel77]^[1]. They apply to a temperature of $20^\circ\ \text{C (293 K)}$ and frequencies greater than $\text{200 kHz}$.

There is the following connection to the primary line parameters:

The ohmic losses originating from the frequency-independent component $R\hspace{0.05cm}'$ are modeled by the parameter $α_0$ and cause a (small for coaxial cables) frequency-independent attenuation.
The component $α_1 · f$ of the attenuation function (per unit length) is due to the derivation losses $(G\hspace{0.08cm}’)$ and the frequency-proportional term $β_1 · f$ causes only delay but no distortion.
The components $α_2$ and $β_2$ are due to the skin effect, which causes the current density inside the conductor to be lower than at the surface in the case of higher-frequency alternating current. As a result, the serial resistance (per unit length) $R\hspace{0.05cm}’$ of an electric line increases with the square root of the frequency.

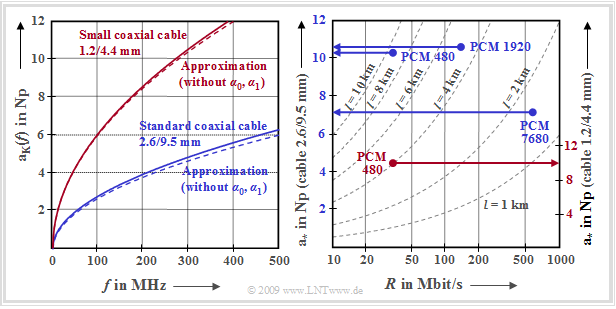
Characteristic cable attenuation

The graph shows the frequency-dependent attenuation curve for the normal coaxial cable and the small coaxial cable. Shown on the left is the cable attenuation of the two coaxial cable types in the frequency range up to $\text{500 MHz}$:

Attenuation function and characteristic attenuation of coaxial cables

$${\rm a}_{\rm K}(f) \hspace{-0.05cm} = \hspace{-0.05cm}\big [ \alpha_0 \hspace{-0.05cm}+ \hspace{-0.05cm} \alpha_1 \cdot f \hspace{-0.05cm}+ \hspace{-0.05cm} \alpha_2 \hspace{-0.05cm}\cdot \hspace{-0.05cm}\sqrt {f} \hspace{0.01cm} \hspace{0.1cm} \big ] \cdot l \hspace{0.01cm}.$$

Notes on graphical representation:

The ordinate labeling is given here in "Np/km".

Often it is also expressed with "dB/km", where the following conversion applies:
$1 \ \rm dB = 0.11513\text{... Np}$, since $\ln(10)/20 = 0.11513\text{...}$

The attenuation curve is here labeled ${\rm a}_{\rm K}(f)$ rather than ${a}_{\rm K}(f)$ ⇒ italicized to make the difference between the attenuation function per unit length „alpha” and the attenuation function „a” (after multiplication with length) more apparent.

It can be seen from the left graph that the error is still tolerable if the frequency-independent component $α_0$ and the frequency-proportional term $(α_1\cdot f)$ are neglected. In the following, we therefore assume the following simplified attenuation function:

$${\rm a}_{\rm K}(f) = \alpha_2 \cdot \sqrt {f} \cdot l = {\rm a}_{\rm \star}\cdot \sqrt { {2f}/{R}} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}|H_{\rm K}(f)| = {\rm e}^{- {\rm a}_{\rm K}(f)}\hspace{0.05cm}, \hspace{0.2cm} {\rm a}_{\rm K}(f)\hspace{0.15cm}{\rm in }\hspace{0.15cm}{\rm Np}\hspace{0.05cm}.$$

$\text{Definition:}$ We denote the characteristic cable attenuation $\rm a_∗$ as the attenuation of a coaxial cable at half the bit rate neglecting the terms $α_0$ and $α_1$:

$${\rm a}_{\rm \star} = {\rm a}_{\rm K}(f = {R}/{2}) = \alpha_2 \cdot \sqrt {{R}/{2}} \cdot l\hspace{0.05cm}.$$

This value is particularly suitable for comparing different conducted transmission systems with different

coaxial cable types (for example, normal or small coaxial cable), each identified by the parameter $\alpha_2$,
bit rates $(R)$ and
cable lengths $(l)$.

The right diagram shows the characteristic cable attenuation $\rm a_∗$ in "Neper" (Np) as a function of the bit rate $R$ and the cable length $l$

for the normal coaxial cable (left ordinate labeling) and
for the small coaxial cable (right ordinate labeling).

This diagram shows the PCM systems of hierarchy levels $3$ to $5$ proposed by the ITU-T (ITU Telecommunication Standardization Sector) in the 1970s. One recognizes:

For all these systems for PCM speech transmission, the characteristic cable attenuation assumes values between $7 \ \rm Np \ \ (≈ 61 \ dB)$ and $10.6 \ \rm Np \ \ (≈ 92 \ dB)$ .
The system $\text{PCM 480}$ – designed for 480 simultaneous telephone calls - with the bit rate $R ≈ 35 \ \rm Mbit/s$ was specified for both the normal coaxial cable $($with $l = 9.3 \ \rm km)$ and for the small coaxial cable $($with $l = 4 \ \rm km)$ specified. The $\rm a_∗$values $10.4\ \rm Np$ and $9.9\ \rm Np$ respectively are in the same order of magnitude.
The transmission system $\text{PCM 1920}$ of the fourth hierarchy level (specified for the normal coaxial cable) with $R ≈ 140 \ \rm Mbit/s$ and $l = 4.65 \ \rm km$ is parameterized by $\rm a_∗ = 10.6 \ \rm Np$ or $10.6 \ {\rm Np}· 8.688 \ \rm dB/Np ≈ 92\ \rm dB$ .
Although the system $\text{PCM 7680}$ in contrast has four times the capacity $R ≈ 560 \rm Mbit/s$ , the characteristic cable attenuation of $\rm a_∗ ≈ 61 \ dB$ due to the better medium "normal coaxial cable" and the shorter cable sections by a factor of $3$ $(l = 1. 55 \ \rm km)$ significantly lower.
These numerical values also show that for coaxial cable systems, the cable length $l$ is more critical than the bit rate $R$. If one wants to double the cable length, one has to reduce the bit rate by a factor $4$ .

You can view the topic described here with the interactive applet Attenuation of copper cables .

Impulse response of a coaxial cable

To calculate the impulse response, the first two attenuation components of the five components of the complex propagation function (per unit length) can be neglected (the reasoning can be found in the previous section). So we start from the following equation:

$$\gamma(f) = \alpha_0 + \alpha_1 \cdot f + {\rm j} \cdot \beta_1 \cdot f +\alpha_2 \cdot \sqrt {f}+ {\rm j}\cdot \beta_2 \cdot \sqrt {f} \approx {\rm j} \cdot \beta_1 \cdot f +\alpha_2 \cdot \sqrt {f}+ {\rm j}\cdot \beta_2 \cdot \sqrt {f} \hspace{0.05cm}.$$

Considering

the cable length $l$,
the characteristic cable attenuation $\rm a_∗$ and
that $α_2$ (in Np) and $β_2$ (in rad) are numerically equal,

thus applies to the frequency response of the coaxial cable:

$$H_{\rm K}(f) = {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} b_1 f} \cdot {\rm e}^{-{\rm a}_{\rm \star}\hspace{0.05cm}\cdot \hspace{0.05cm} \sqrt{2f/R} }\cdot {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}{\rm a}_{\rm \star}\hspace{0.05cm}\cdot \hspace{0.02cm} \sqrt{2f/R}}= {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} b_1 f} \cdot {\rm e}^{-2{\rm a}_{\rm \star}\hspace{0.03cm}\cdot \hspace{0.03cm} \sqrt{ {\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}f/R}} \hspace{0.05cm}.$$

The following abbreviations are used here:

$$b_1\hspace{0.1cm}{(\rm in }\hspace{0.15cm}{\rm rad)}= \beta_1 \cdot l \hspace{0.05cm}, \hspace{0.8cm} {\rm a}_{\rm \star}\hspace{0.1cm}{(\rm in }\hspace{0.15cm}{\rm Np)}= \alpha_2 \cdot \sqrt {R/2} \cdot l \hspace{0.05cm}.$$

The time domain display is obtained by applying the Fourier inverse transform and the Convolution theorem:

$$h_{\rm K}(t) = \mathcal{F}^{-1} \left \{ {\rm e}^{-{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm} b_1 f}\right \} \star\mathcal{F}^{-1} \left \{ {\rm e}^{-2{\rm a}_{\rm \star}\hspace{0.03cm}\cdot \hspace{0.03cm} \sqrt{ {\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}f/R} }\right \} \hspace{0.05cm}.$$

To be considered here:

The first term yields the Dirac function $δ(t – τ_{\rm P})$ shifted by the phase delay $τ_{\rm P} = b_1/2π$ .
The second term can be given analytically closed. We write $h_{\rm K}(t + τ_P)$, so that the phase delay $τ_{\rm P}$ need not be considered further.

$$h_{\rm K}(t + \tau_{\rm P}) = \frac {{\rm a}_{\rm \star}}{\pi \cdot \sqrt{2 \cdot R \cdot t^3}}\cdot {\rm exp} \left [ -\frac {{\rm a}_{\rm \star}^2}{ {2\pi \cdot R\cdot t}} \right ]\hspace{0.05cm},\hspace{0.2cm}{\rm a}_{\rm \star}\hspace{0.15cm}{\rm in\hspace{0.15cm} Np}\hspace{0.05cm}.$$

Since also the bit rate $R$ has already been considered in the definition of the characteristic cable attenuation $a_∗$ this equation can be easily represented with the normalized time $t\hspace{0.05cm}' = t/T$ :

$$h_{\rm K}(t\hspace{0.05cm}' + \tau_{\rm P}\hspace{0.05cm} ') = \frac {1}{T} \cdot \frac {{\rm a}_{\rm \star}}{\pi \cdot \sqrt{2 \cdot t\hspace{0.05cm}'\hspace{0.05cm}^3}}\cdot {\rm exp} \left [ -\frac {{\rm a}_{\rm \star}^2}{ {2\pi \cdot t\hspace{0.05cm}'}} \right ]\hspace{0.05cm},\hspace{0.2cm}{\rm a}_{\rm \star}\hspace{0.15cm}{\rm in\hspace{0.15cm} Np}\hspace{0.05cm}.$$

Here $T = 1/R$ denotes the symbol duration of a binary system and it holds $τ_{\rm P} \hspace{0.05cm}' = τ_{\rm P}/T$.

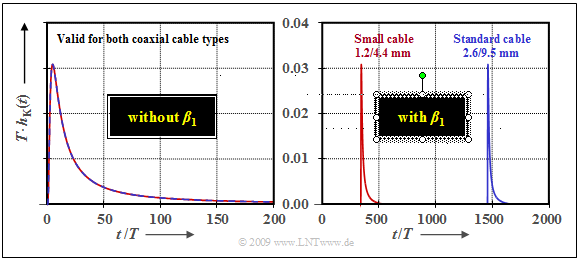
$\text{Example 1:}$ The results of this page are illustrated by the following graph as an example.

The normalized impulse response $T · h_{\rm K}(t)$ of a coaxial cable with $\rm a_∗ = 60 \ dB \ \ (6.9\ Np)$ is shown.
The attenuation function parameters $α_0$ and $α_1$ are thus neglected.
For the left graph, the parameter $β_1 = 0$ was also set.

Impulse response of a coaxial cable with $\rm a_∗ = 60 \ dB$

Due to the parameterization by means of the characteristic cable attenuation $a_∗$ and the normalization of the time to the symbol duration $T$ the left curve is equally valid for systems with small or normal coaxial cable, different lengths and different bit rates, for example for a

normal coaxial cable $\text{2.6/9.5 mm}$, bit rate $R = 140 \ \rm Mbit/s$, cable length $l = 3 \ \rm km$ ⇒ system $\rm A$,
small coaxial cable $\text{1.2/4.4 mm mm}$, bit rate $R = 35 \ \rm Mbit/s$, cable length $l = 2.8 \ \rm km$ ⇒ system $\rm B$.

It can be seen that even at this moderate cable attenuation $\rm a_∗ = 60 \ \rm dB$ the impulse response already extends over more than $200$ symbol durations due to the skin effect $(α_2 = β_2 ≠ 0)$ . Since the integral over $h_{\rm K}(t)$ is equal to $H_{\rm K}(f = 0) = 1$ , the maximum value becomes very small:

$${\rm Max}\big [h_{\rm K}(t)\big ] \approx 0.03.$$

In the diagram on the right, the effects of the phase parameter $β_1$ can be seen. Note the different time scales of the left and the right diagram:

For system $\rm A$ $(β_1 = 21.78 \ \rm rad/(km · MHz)$, $T = 7.14\ \rm ns)$ results in $β_1$ a running time of

$$\tau_{\rm A}= \frac {\beta_1 \cdot l}{2\pi} =\frac {21.78\, { {\rm rad} }/{ {(\rm km \cdot MHz)} }\cdot 3\,{\rm km} }{2\pi} = 10.4\,{\rm \mu s}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}\tau_{\rm A}\hspace{0.05cm}' = {\tau_{\rm A} }/{T} \approx 1457\hspace{0.05cm}.$$

On the other hand, the following can be obtained for system $\rm B$ $(β_1 = 22.18 \ \rm rad/(km · MHz)$, $T = 30 \ \rm ns)$:

$$\tau_{\rm B}= \frac {\beta_1 \cdot l}{2\pi} =\frac {22.18\, { {\rm rad} }/{ {(\rm km \cdot MHz)} }\cdot 2.8\,{\rm km} }{2\pi} = 9.9\,{\rm µ s}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}\tau_{\rm B}\hspace{0.05cm}' ={\tau_{\rm B} }/{T} \approx 330\hspace{0.05cm}.$$

Although $τ_{\rm A} ≈ τ_{\rm B}$ holds, completely different ratios result because of the time normalization to $T = 1/R$ .

$\text{Conclusion:}$ When simulating and optimizing message systems, one usually omits the phase term with $b_1 = β_1 · l$, since this results exclusively in a (often not disturbing) running time, but no signal distortion.

Basic receiver impulse

With the basic transmitting impulse $g_s(t)$ and the impulse response $h_{\rm K}(t)$ the result for the basic receiver impulse is:

$$g_r(t) = g_s(t) \star h_{\rm K}(t)\hspace{0.05cm}.$$

If an NRZ square wave pulse $g_s(t)$ with amplitude $s_0$ and duration $Δt_s = T$ is used at the transmitter, the following results for the basic pulse at the output of the coaxial cable:

$$g_r(t) = 2 s_0 \cdot \left [ {\rm Q} \left (\frac {{\rm a}_{\rm \star}/\sqrt {\pi}}{ \sqrt{ (t/T - 0.5)}}\right ) - {\rm Q} \left (\frac {{\rm a}_{\rm \star}/\sqrt {\pi}}{ \sqrt{ (t/T + 0.5)}}\right ) \right ]\hspace{0.05cm}.$$

Here $\rm a_∗$ denotes the characteristic cable attenuation in Neper and ${\rm Q}(x)$ the complementary Gaussian error function.

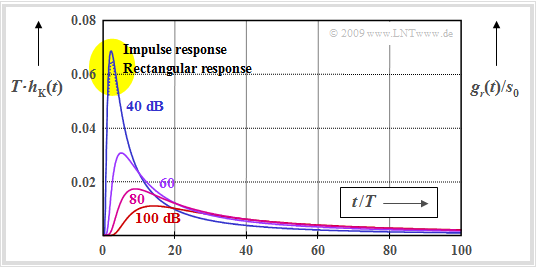
$\text{Example 2:}$ The figure shows the characteristic cable attenuations $\rm a_∗ = 40 \ \rm dB$, $60 \ \rm dB$, $80 \ \rm dB$ and $100 \ \rm dB$ , respectively

the normalized coaxial cable impulse response $T · h_{\rm K}(t)$ ⇒ impulse response (solid curves), and
the basic receiver impulse $g_r(t)$ normalized to the transmission amplitude $s_0$ ⇒ square-wave response (dotted line).

Smaller values of $\rm a_∗$ are not relevant for practice.

Impulse response and square-wave response (basic receiver impulse) of the coaxial cable

One recognizes the following from this diagram:

With $\rm a_∗ = 40 \ \rm dB$ , the normalized square-wave response $g_r(t)/s_0$ is slightly (about a factor of $0.95)$ smaller at the peak than the normalized impulse response $T · h_{\rm K}(t)$. Here there is a small difference between impulse response and square-wave response.
In contrast, for the case $a_∗ ≥ 60 \ \rm dB$ , the square wave response and the impulse response are indistinguishable within the character accuracy.
For a RZ pulse, the above equation for the basic receiver impulse would still need to be multiplied by the duty cycle $Δt_s/T$ . In this case $g_r(t)/s_0$ is smaller than $T · h_{\rm K}(t)$ by at least this factor.
The equation modified in this way is also a good approximation for other basic transmitting pulses as long as $\rm a_∗≥ 60 \ \rm dB$ is sufficiently large. $Δt_s$ then indicates the equivalent pulse duration of the basic transmitting pulse.

We draw your attention to the interactive applet Zeitverhalten von Kupferkabeln , which deals with the topic discussed here.

Special features of coaxial cable systems

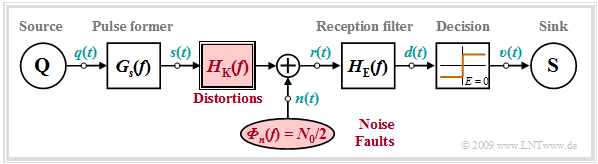
Assuming binary transmission with NRZ rectangular pulses $($symbol duration $T)$ and a coaxial transmission channel, the following system model is obtained:

Binary transmission system with coaxial cable

In particular, it should be noted:

In a simulation, the propagation time of the coaxial cable is conveniently left out of consideration. Then the basic receiver impulse $g_r(t)$ is approximated:

$$g_r(t) \approx s_0 \cdot T \cdot h_{\rm K}(t) = \frac {s_0 \cdot {\rm a}_{\rm \star}/\pi}{ \sqrt{2 \cdot(t/T)^3}}\cdot {\rm e}^{ -{{\rm a}_{\rm \star}^2}/( {2\pi \hspace{0.05cm}\cdot \hspace{0.05cm}t/T}) } \hspace{0.05cm}, \hspace{0.2cm} \hspace{0.15cm} {\rm mit}\hspace{0.15cm}{\rm a}_{\rm \star}\hspace{0.15cm} {\rm in}\hspace{0.15cm} {\rm Neper}\hspace{0.05cm}.$$

Because of the good shielding of the coaxial cables against other interferences, the thermal noise is the dominant source of interference. In this case, the interference signal $n(t)$ is Gaussian distributed and white and is described by the (two-sided) noise power density $N_0/2$ .
By far the largest noise component arises in the input stage of the receiver, so that it is expedient to add the noise signal $n(t)$ at the "cable-receiver" interface. With the amplitude coefficients $a_{\nu}$ , the following then applies to the received signal:

$$r(t) = \sum_{\nu = - \infty}^{+ \infty}a_{\nu}\cdot g_r(t - \nu \cdot T)+ n(t) \hspace{0.05cm} .$$

This noise addition point is also useful because the frequency response $H_{\rm K}(f)$ decisively attenuates all noise accumulated along the cable.

Exercises for the chapter

Exercise 4.4: Coaxial Cable - Frequency Response

Exercise 4.5: Coaxial Cable - Impulse Response

Exercise 4.5Z: Impulse Response once again

List of sources

↑ Wellhausen, H. W.: Dämpfung, Phase und Laufzeiten bei Weitverkehrs–Koaxialpaaren. Frequenz 31, S. 23-28, 1977.

[1] Wellhausen, H. W.: Dämpfung, Phase und Laufzeiten bei Weitverkehrs–Koaxialpaaren. Frequenz 31, S. 23-28, 1977.

[1]