Exercise 3.09: Correlation Receiver for Unipolar Signaling

Example correlation values

The joint decision of $N = 3$ binary symbols ("bits") by means of the correlation receiver is considered.

The $M = 8$ possible source symbol sequences $Q_i$ all have the same probability and they are defined by the following unipolar amplitude coefficients:

$$Q_0 = 000, \hspace{0.15cm}Q_1 = 001,\hspace{0.15cm}Q_2 = 010,\hspace{0.15cm}Q_3 = 011 \hspace{0.05cm},\hspace{0.15cm} Q_4 = 100, \hspace{0.15cm}Q_5 = 101,\hspace{0.15cm}Q_6 = 110,\hspace{0.15cm}Q_7 = 111 \hspace{0.05cm}.$$

Further applies:

The possible transmitted signals $s_i(t)$ – each with duration $3T$ – are all rectangular with the exception of $s_0(t) \equiv 0$.

The signals $s_1(t)$, $s_2(t)$ and $s_4(t)$ with only one "$1$" each have the signal energy $E_{\rm B}$ (stands for "energy per bit"), while for example the energy of $s_7(t)=3E_{\rm B}$.

The correlation receiver forms from the noisy received signal $r(t) = s(t) + n(t)$ a total of $2^3 = 8$ decision variables (metrics)

$$W_i = I_i - {E_i}/{2 }\hspace{0.3cm}{\rm with}\hspace{0.3cm} I_i =\int_{0}^{3T} r(t) \cdot s_i(t) \,{\rm d} t \hspace{0.3cm}( i = 0,\text{...} , 7)$$

and sets the sink symbol sequence $V = Q_j$, if $W_j$ is larger than all other $W_{i \ne j}$. Thus, it makes an optimal decision in the sense of "maximum likelihood".

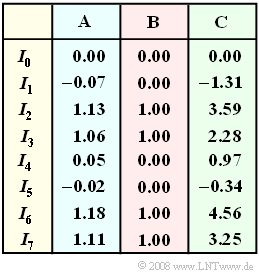
In the table, the (uncorrected) correlation values $I_0, \ \text{...} \ , I_7$ for three different systems differing in terms of noise $n(t)$ and labeled $\rm A$, $\rm B$ or $\rm C$.

One of these columns stands for "no noise",
one for "minor noise", and
another one for "strong noise".

Note:

The exercise belongs to the chapter "Optimal Receiver Strategies".

The same source symbol sequence was always sent to determine the metrics for the three system variants.

Question

	$\rm System \ A$,
	$\rm System \ B$,
	$\rm System \ C$.

$k \ = \ $

${\rm System \ A} \text{:} \hspace{0.2cm} j \ = \ $

${\rm System \ C} \text{:} \hspace{0.2cm} j \ = \ $

	$\rm System \ A$,
	$\rm System \ B$,
	$\rm System \ C$.

	The difference between $W_2$ and the next largest value $W_{i \ne 2}$ is smaller the stronger the noise is.
	When falsification occurs, the receiver is most likely to decide in favor of the symbol sequence $Q_6$.
	The probabilities for erroneous decisions in favor of $Q_0$, $Q_3$ and $Q_6$, respectively, are equal.

Solution

(1) Solution 2 is correct:

For system $\rm B$, metrics $0$ occur four times and metrics $1$ occur four times.
This points to $n(t) = 0$, otherwise – as in systems $\rm A$ and $\rm C$ – all $I_i$ would have to differ.

(2) For system $\rm B$, the decision values $W_i = I_i \ – E_i/2$, each normalized to $E_{\rm B}$, are as follows:

$$W_0 = 0 - 0 = 0, \hspace{0.2cm}W_1 = 0 - 0.5 = -0.5 \hspace{0.05cm},$$

$$W_2 = 1 - 0.5 = 0.5, \hspace{0.2cm}W_3 = 1 - 1 = 0 \hspace{0.05cm},$$

$$W_4 = 0 - 0.5 = -0.5, \hspace{0.2cm}W_5 = 0 - 1 = -1 \hspace{0.05cm}.$$

$$W_6 = 1 - 1 = 0, \hspace{0.2cm}W_7 = 1 - 1.5 = -0.5 \hspace{0.05cm}.$$

The maximum value $W_2 = 0.5$ ⇒ $i = 2$.
Thus, the correlation receiver decides to use $V = Q_2$.
Since there is no noise, $Q_2 =$ "$\rm 010$" was indeed also sent ⇒ $\underline { k= 2}$.

(3) For the decision values of system $\rm A$ holds:

$$W_0 = 0.00 - 0.00 = 0.00, \hspace{0.2cm}W_1 = -0.07 - 0.50 = -0.57, $$

$$W_2 = 1.13 - 0.50 = 0.63, \hspace{0.2cm}W_3 = 1.06 - 1.00 = 0.06 \hspace{0.05cm},$$

$$W_4 = 0.05 - 0.50 = -0.45, \hspace{0.2cm}W_5 = -0.02 - 1.00 = -1.02\hspace{0.05cm},$$

$$W_6 = 1.18 - 1.00 = 0.18, \hspace{0.2cm}W_7 = 1.11 - 1.50 = -0.39 \hspace{0.05cm}.$$

The maximum is $W_j = W_2$ ⇒ $\underline { j= 2}$.
This means that the correlation receiver also makes the correct decision $V = Q_2$ for system $\rm A$.
However, without the correction term $(– E_i/2)$, the receiver would have made the wrong decision $V = Q_6$.

(4) The correlation receiver $\rm C$ has to compare the following values:

$$W_0 = 0.00 - 0.00 = 0.00, \hspace{0.2cm}W_1 = -1.31 - 0.50 = -1.81 \hspace{0.05cm},$$

$$W_2 = 3.59 - 0.50 = 3.09, \hspace{0.2cm}W_3 = 2.28 - 1.00 = 1.28 \hspace{0.05cm},$$

$$W_4 = 0.97 - 0.50 = 0.47, \hspace{0.2cm}W_5 = -0.34 - 1.00 = -1.34 \hspace{0.05cm},$$

$$W_6 = 4.56 - 1.00 = 3.56, \hspace{0.2cm}W_7 = 3.25 - 1.50 = 1.75 \hspace{0.05cm}.$$

The maximization here gives $\underline {j = 6}$ ⇒ $V = Q_6$.

But since $Q_2$ was sent, the correlation receiver decides wrong here.
The noise is too strong.

(5) Solution 3 is correct:

The noise is greatest for system $\rm C$ and is even so great for the current received values that the correlation receiver makes an incorrect decision.

(6) Statements 1 and 3 are correct:

In the error-free case (system $\rm B$), the difference between $W_2 = 0.5$ and the next largest values $W_0 = W_3 = W_6 = 0$ is equal to $D_{\hspace{0.02cm}\rm min} =0.5$ in each case.
In system $\rm A$ (light noise), the difference between $W_2 = 0.63$ and the next largest value $W_6 = 0.18$ is still $D_{\hspace{0.02cm}\rm min} = 0.45$.
If the noise power is increased by a factor of $50$, the correlation receiver still decides correctly, but then the minimum difference $D_{\hspace{0.02cm}\rm min} = 0.16$ is significantly smaller.
For system $\rm C$, where the correlation receiver is overcharged ⇒ subtask (4), a noise power larger by a factor of 400 compared to system $\rm A$ was used as a basis.
If the correlation receiver decides the transmitted sequence $Q_2$ incorrectly, a falsification to the sequences $Q_0$, $Q_3$ and $Q_6$, respectively, is most likely, since all these three sequences differ from $Q_2$ only in one bit each.
The fact that $W_6$ is always larger than $W_0$ or $W_3$ in the described simulation is "coincidence" and should not be overinterpreted.