Exercise 4.09: Recursive Systematic Convolutional Codes

State transition diagram of an RSC code

In the "Exercise 4.8" important properties of convolutional codes have already been derived from the state transition diagram, assuming a non-recursive filter structure.

Now a rate $1/2$ RSC code is treated in a similar manner. Here "RSC" stands for "Recursive Systematic Convolutional".

The transfer function matrix of an RSC convolutional code can be specified as follows:

$${\boldsymbol{\rm G}}(D) = \left [ 1\hspace{0.05cm},\hspace{0.3cm} G^{(2)}(D)/G^{(1)}(D) \right ] \hspace{0.05cm}.$$

Otherwise, exactly the same conditions apply here as in exercise 4.8. We refer again to the following theory pages:

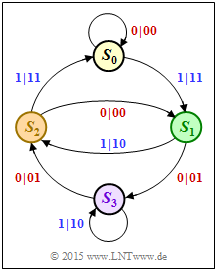
In the state transition diagram, the state $S_0$ is always assumed. Two arrows go from each state. The label is "$u_i \hspace{0.05cm}| \hspace{0.05cm} x_i^{(1)}x_i^{(2)}$". For a systematic code, this involves:

The first code bit is identical to the information bit: $\hspace{0.2cm} x_i^{(1)} = u_i ∈ \{0, \, 1\}$.
The second code bit is the parity-check bit: $\hspace{0.2cm} x_i^{(2)} = p_i ∈ \{0, \, 1\}$.

Hints:

The exercise refers to the chapter "Basics of Turbo Codes".
Similar exercises can be found in chapters 3.1 through 3.3.

The following vectorial quantities are used in the questions for this exercise:
- the information sequence: $\hspace{0.2cm} \underline{u} = (u_1, \, u_2, \text{...} \hspace{0.05cm} )$,
- the parity-check sequence: $\hspace{0.2cm} \underline{p} = (p_1, \, p_2, \text{...} \hspace{0.05cm})$,
- the impulse response: $\hspace{0.2cm} \underline{g} = (g_1, \, g_2, \text{...} \hspace{0.05cm} ); \hspace{0.2cm}$ this is equal to the parity sequence $\underline{p}$ for $\underline{u} = (1, \, 0, \, 0, \text{...} \hspace{0.05cm} )$.

Questions

Musterlösung

Solution

(1) Verfolgt man die Übergänge im Zustandsdiagramm für die Sequenz $\underline{u} = (1, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0)$ am Eingang, so erhält man den Weg

$$S_0 → S_1 → S_3 → S_2 → S_1 → S_3 → S_2 → S_1 → S_3 → \hspace{0.05cm}\text{...} \hspace{0.05cm}$$

Bei jedem Übergang ist das erste Codesymbol $x_i^{(1)}$ gleich dem Informationsbit $u_i$ und das Codesymbol $x_i^{(2)}$ gibt das Paritybit $p_i$ an.
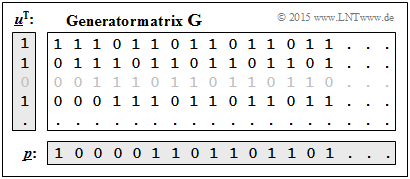
Damit erhält man das Ergebnis entsprechend dem Lösungsvorschlag 1:

$$\underline{p}= (\hspace{0.05cm}1\hspace{0.05cm}, \hspace{0.05cm}1\hspace{0.05cm}, \hspace{0.05cm}1\hspace{0.05cm}, \hspace{0.05cm} 0\hspace{0.05cm}, \hspace{0.05cm} 1\hspace{0.05cm}, \hspace{0.05cm} 1\hspace{0.05cm}, \hspace{0.05cm} 0\hspace{0.05cm}, \hspace{0.05cm} 1\hspace{0.05cm}, \hspace{0.05cm} 1\hspace{0.05cm},\hspace{0.05cm}\text{...} \hspace{0.05cm}) = \underline{g}\hspace{0.05cm}.$$

Bei einem jeden RSC–Code ist die Impulsantwort $\underline{g}$ unendlich lang und wird irgendwann periodisch, in diesem Beispiel mit der Periode $P = 3$ und "$0, \, 1, \, 1$".

Verdeutlichung von $\underline{p} = (1, \, 1, \, 0, \, 1)^{\rm T} \cdot \mathbf{G}$

(2) Die Grafik zeigt die Lösung dieser Aufgabe entsprechend der Gleichung $\underline{p} = \underline{u}^{\rm T} \cdot \mathbf{G}$.

Hierbei ist die Generatormatrix $\mathbf{G}$ nach unten und rechts unendlich weit ausgedehnt.
Richtig ist der Lösungsvorschlag 2.

(3) Richtig sind die Lösungsvorschläge 1 und 2:

Zwischen der Impulsantwort $\underline{g}$ und der $D$–Übertragungsfunktion $\mathbf{G}(D)$ besteht der Zusammenhang gemäß dem ersten Lösungsvorschlag:

$$\underline{g}= (\hspace{-0.05cm}1\hspace{-0.05cm}, \hspace{-0.05cm}1\hspace{-0.05cm}, \hspace{-0.05cm}1\hspace{-0.05cm}, \hspace{-0.05cm}0\hspace{-0.05cm}, \hspace{-0.05cm}1\hspace{-0.05cm}, \hspace{-0.05cm}1\hspace{-0.05cm}, \hspace{-0.05cm}0\hspace{-0.05cm}, \hspace{-0.05cm}1\hspace{-0.05cm}, \hspace{-0.05cm}1\hspace{-0.05cm}, ... ) \quad \circ\!\!-\!\!\!-^{\hspace{-0.25cm}D}\!\!\!-\!\!\bullet\quad G(D) = 1\hspace{-0.05cm}+\hspace{-0.05cm} D\hspace{-0.05cm} +\hspace{-0.05cm} D^2\hspace{-0.05cm} +\hspace{-0.05cm} D^4 \hspace{-0.05cm}+\hspace{-0.05cm} D^5 \hspace{-0.05cm}+\hspace{-0.05cm} D^7 \hspace{-0.05cm}+\hspace{-0.05cm} D^8 + \hspace{0.05cm} \text{...} \hspace{0.05cm}.$$

Überprüfen wir nun den zweiten Vorschlag:

$$G(D) = \frac{1+ D^2}{1+ D + D^2} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} G(D) \cdot [1+ D + D^2] = 1+ D^2 \hspace{0.05cm}.$$

Die folgende Rechnung zeigt, dass diese Gleichung tatsächlich stimmt:

$$(1+ D+ D^2+ D^4 +D^5 + D^7 + D^8 + \hspace{0.05cm} \text{...}) \cdot (1+ D+ D^2 ) =$$

$$=1+ D+ D^2\hspace{1.05cm} +D^4 + D^5 \hspace{1.05cm} +D^7 + D^8 \hspace{1.05cm} + D^{10}+ \hspace{0.05cm} \text{...}$$

$$+ \hspace{0.8cm}D+ D^2+D^3 \hspace{1.05cm}+ D^5 + D^6 \hspace{1.05cm} +D^8 + D^9 \hspace{1.25cm} +\hspace{0.05cm} \text{...} $$

$$+ \hspace{1.63cm} D^2+D^3+ D^4 \hspace{1.05cm}+ D^6 +D^7 \hspace{1.05cm}+ D^9 + D^{10} \hspace{0.12cm}+ \hspace{0.05cm} \text{...}$$

$$=\underline{1\hspace{0.72 cm}+ D^2} \hspace{0.05cm}.$$

Da aber die Gleichung (2) stimmt, muss die letzte Gleichung falsch sein.

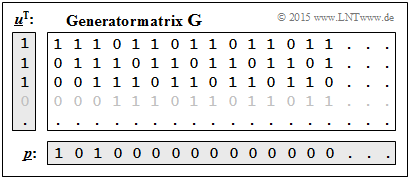
(4) Richtig ist nur der Lösungsvorschlag 1:

Aus $\underline{u} = (1, \, 1, \, 1)$ folgt $U(D) = 1 + D + D^2$. Damit gilt auch:

$$P(D) = U(D) \cdot G(D) = (1+D+D^2) \cdot \frac{1+D^2}{1+D+D^2}= 1+D^2\hspace{0.3cm} \Rightarrow\hspace{0.3cm} \underline{p}= (\hspace{0.05cm}1\hspace{0.05cm},\hspace{0.05cm} 0\hspace{0.05cm},\hspace{0.05cm} 1\hspace{0.05cm},\hspace{0.05cm} 0\hspace{0.05cm},\hspace{0.05cm} 0\hspace{0.05cm},\hspace{0.05cm}\text{...}\hspace{0.05cm})\hspace{0.05cm}. $$

Wären die Größen $u_i$ und $g_i$ reellwertig, so würde die (diskrete) Faltung $\underline{p} = \underline{u} * \underline{g}$ stets zu einer Verbreiterung führen ⇒ $\underline{p}$ wäre in diesem Fall breiter als $\underline{u}$ und auch breiter als $\underline{g}$.
Bei $u_i ∈ {\rm GF}(2)$ und $g_i ∈ {\rm GF}(2)$ kann es (muss es aber nicht) dagegen vorkommen, dass auch bei unbegrenztem $\underline{u}$ oder bei unbegrenztem $\underline{g}$ das Faltungsprodukt $\underline{p} = \underline{u} * \underline{g}$ begrenzt ist.

Verdeutlichung von $\underline{p} = (1, \, 1, \, 1, \, 0, \, ...)^{\rm T} \cdot \mathbf{G}$

Das Ergebnis wird abschließend noch entsprechend der Gleichung $\underline{p} = \underline{u}^{\rm T} \cdot \mathbf{G}$ überprüft.

(5) Nach ähnlicher Vorgehensweise wie in der Aufgabe A4.8, (4) erkennt man:

Die freie Distanz wird auch hier durch den Pfad $S_0 → S_0 → S_1 → S_2 → S_0 → S_0 → \hspace{0.05cm}\text{...}\hspace{0.05cm}$ bestimmt.
Die zugehörige Codesequenz $\underline{x}$ ist nun aber " $00 \ 11 \ 10 \ 11 \ 00 \ ... $".
Damit ergibt sich die freie Distanz zu $d_{\rm F} \ \underline{= 5}$.
Beim nichtrekursiven Code (Aufgabe 4.8) war dagegen die freie Distanz $d_{\rm F} = 3$.

	Es gilt $\underline{g} = (1, \, 1, \, 1, \, 0, \, 1, \, 1, \, 0, \, 1, \, 1, \text{...} \hspace{0.05cm})$.
	Es gilt $\underline{g} = (1, \, 0, \, 1, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \text{...} \hspace{0.05cm})$.

	Es gilt $\underline{p} = (1, \, 0, \, 1, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \text{...} \hspace{0.05cm})$.
	Es gilt $\underline{p} = (1, \, 0, \, 0, \, 0, \, 0, \, 1, \, 1, \, 0, \, 1, \, 1, \, 0, \, 1, \text{...} \hspace{0.05cm})$.
	Bei begrenzter Informationssequenz $\underline{u}$ ist stets auch $\underline{p}$ begrenzt.

	Es gilt $G(D) = 1 + D + D^2 + D^4 + D^5 + D^7 + D^8 + \text{...} \hspace{0.05cm}$
	Es gilt $G(D) = (1 + D^2)/(1 + D + D^2)$.
	Es gilt $G(D) = (1 + D + D^2)/(1 + D^2)$.

	Es gilt $\underline{p} = (1, \, 0, \, 1, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \, 0, \text{...} \hspace{0.05cm})$.
	Es gilt $\underline{p} = (1, \, 0, \, 0, \, 0, \, 0, \, 1, \, 1, \, 0, \, 1, \, 1, \, 0, \, 1, \text{...} \hspace{0.05cm})$.
	Bei unbegrenzter Impulsantwort $\underline{g}$ ist stets auch $\underline{p}$ unbegrenzt.