Difference between revisions of "Aufgaben:Exercise 1.4Z: Representation of Oscillations"
m |
m |
||
| Line 35: | Line 35: | ||
::(3) [[Signal_Representation/Equivalent_Low_Pass_Signal_and_Its_Spectral_Function| Equivalent Low-Pass Signal and its Spectral Function]]. | ::(3) [[Signal_Representation/Equivalent_Low_Pass_Signal_and_Its_Spectral_Function| Equivalent Low-Pass Signal and its Spectral Function]]. | ||
| − | *In our tutorial $\rm LNTwww$, the | + | *In our tutorial $\rm LNTwww$, the plot of the analytical signal $s_+(t)$ in the complex plane is sometimes referred to as the "pointer diagram", while the "locus curve" gives the time course of the equivalent lowpass signal $s_{\rm TP}(t)$ . We refer you to the corresponding interactive Applets |
| − | ::(1) [[Applets:Physikalisches_Signal_%26_Analytisches_Signal| | + | ::(1) [[Applets:Physikalisches_Signal_%26_Analytisches_Signal|Physical & Analytic Signal]], |
| − | ::(2) [[Applets:Physikalisches_Signal_%26_Äquivalentes_TP-Signal| | + | ::(2) [[Applets:Physikalisches_Signal_%26_Äquivalentes_TP-Signal|Physical Signal & Equivalent Lowpass Signal]]. |
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Calculate the signal parameters $A_{\rm T}$, $f_{\rm T}$ and $ω_{\rm T}$. |
|type="{}"} | |type="{}"} | ||
$A_{\rm T} \ = \ $ { 2 3% } | $A_{\rm T} \ = \ $ { 2 3% } | ||
| Line 51: | Line 51: | ||
$\omega_{\rm T} \ = \ $ { 3141.5 3% } $\ \text{1/s}$ | $\omega_{\rm T} \ = \ $ { 3141.5 3% } $\ \text{1/s}$ | ||
| − | { | + | {Determine the phase $\phi_{\rm T}$ $($zwischen $±180^\circ)$ and the duration $τ$. |
|type="{}"} | |type="{}"} | ||
$\phi_{\rm T} \ = \ $ { -139--131 } $\ \text{Grad}$ | $\phi_{\rm T} \ = \ $ { -139--131 } $\ \text{Grad}$ | ||
$τ \ = \ $ { 0.75 3% } $\ \text{ms}$ | $τ \ = \ $ { 0.75 3% } $\ \text{ms}$ | ||
| − | { | + | {At what time $t_1 > 0$ does the analytical signal $z_+(t)$ first become imaginary? |
|type="{}"} | |type="{}"} | ||
$t_1 \ = \ $ { 0.25 3% } $\ \text{ms}$ | $t_1 \ = \ $ { 0.25 3% } $\ \text{ms}$ | ||
| − | { | + | {What is the equivalent low-pass signal $z_{\rm TP}(t)$? Enter the value at $t = 1 \text{ ms}$ ms to check. |
|type="{}"} | |type="{}"} | ||
${\rm Re}\big[z_{\rm TP}(t = 1\ \rm ms)\big] \ = \ $ { -1.454--1.374 } | ${\rm Re}\big[z_{\rm TP}(t = 1\ \rm ms)\big] \ = \ $ { -1.454--1.374 } | ||
${\rm Im}\big[z_{\rm TP}(t = 1\ \rm ms)\big] \ = \ $ { -1.454--1.374 } | ${\rm Im}\big[z_{\rm TP}(t = 1\ \rm ms)\big] \ = \ $ { -1.454--1.374 } | ||
| − | { | + | {Which of these statements are valid for all harmonic oscillations? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The spectrum $Z(f)$ consists of two Dirac functions at $±f_{\rm T}$. |
| − | - | + | - The spectrum $Z_+(f)$ has one Dirac function at $–f_{\rm T}$. |
| − | + | + | + The spectrum $Z_{\rm TP}(f)$ contains a Dirac function at $f = 0$. |
| − | + | + | + The analytical signal $z_+(t)$ is always complex. |
| − | - | + | - The equivalent lowpass signal $z_{\rm TP}(t)$ is always complex. |
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
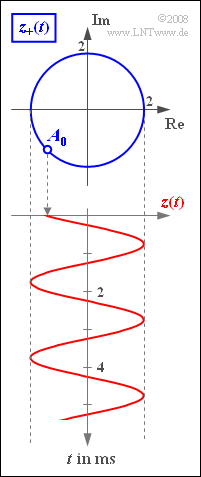
| − | '''(1)''' | + | '''(1)''' In the graphical representation of the time function $z(t)$ , one can identify |
| − | * | + | *the (normalized) amplitude $A_{\rm T}\hspace{0.15cm}\underline{ = 2}$ and the period $T_0=2$ milliseconds. |
| − | * | + | *Therefore, the signal frequency is $f_{\rm T} = 1/T_0\hspace{0.15cm}\underline{ = 500}$ Hz and the angular frequency is $ω_{\rm T}= 2πf_{\rm T} \hspace{0.15cm}\underline{ = 3141.5}$ 1/s. |
| − | '''(2)''' | + | '''(2)''' The analytical signal is generally: |
:$$z_+(t) = A_{\rm T} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm}(\omega_{\rm T}\cdot \hspace{0.05cm}t + \phi_{\rm T})} = A_{\rm T} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} \phi_{\rm T}} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} \hspace{0.03cm}\omega_{\rm T}\cdot \hspace{0.05cm}t }\hspace{0.05cm}.$$ | :$$z_+(t) = A_{\rm T} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm}(\omega_{\rm T}\cdot \hspace{0.05cm}t + \phi_{\rm T})} = A_{\rm T} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} \phi_{\rm T}} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} \hspace{0.03cm}\omega_{\rm T}\cdot \hspace{0.05cm}t }\hspace{0.05cm}.$$ | ||
| − | * | + | *At the same time the relationship: |
:$$A_0 = z_+(t = 0) = A_{\rm T} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} \phi_{\rm T}} \hspace{0.05cm}.$$ | :$$A_0 = z_+(t = 0) = A_{\rm T} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} \phi_{\rm T}} \hspace{0.05cm}.$$ | ||
| − | * | + | *The complex amplitude $A_0$ can be read from the upper plot. |
:$$A_0 = - \sqrt{2} - {\rm j} \cdot \sqrt{2} = A_{\rm 0} \cdot {\rm e}^{-{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} 0.75 \pi} \hspace{0.05cm}.$$ | :$$A_0 = - \sqrt{2} - {\rm j} \cdot \sqrt{2} = A_{\rm 0} \cdot {\rm e}^{-{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} 0.75 \pi} \hspace{0.05cm}.$$ | ||
| − | * | + | *A comparison of both equations leads to the result: |
:$$ \phi_{\rm T} = - 0.75 \pi \hspace{0.15cm}\underline {= - 135^{\circ}} \hspace{0.05cm}.$$ | :$$ \phi_{\rm T} = - 0.75 \pi \hspace{0.15cm}\underline {= - 135^{\circ}} \hspace{0.05cm}.$$ | ||
| − | * | + | *Thereby, the following relationship exists with the duration $τ$: |
:$$\phi_{\rm T} = - 2 \pi \cdot f_{\rm T} \cdot \tau \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \tau = \frac{-\phi_{\rm T}}{2 \pi \cdot f_{\rm T}} = \frac{0.75 \pi}{2 \pi \cdot 0.5\,{\rm kHz}} \hspace{0.15cm}\underline {= 0.75 \,{\rm ms}} \hspace{0.05cm}.$$ | :$$\phi_{\rm T} = - 2 \pi \cdot f_{\rm T} \cdot \tau \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \tau = \frac{-\phi_{\rm T}}{2 \pi \cdot f_{\rm T}} = \frac{0.75 \pi}{2 \pi \cdot 0.5\,{\rm kHz}} \hspace{0.15cm}\underline {= 0.75 \,{\rm ms}} \hspace{0.05cm}.$$ | ||
Revision as of 19:23, 11 November 2021
Here, we consider a harmonic oscillation $z(t)$, which is shown in the graph together with the corresponding analytical signal $z_+(t)$ . These signals can be described mathematically as follows:
- $$z(t) = A_{\rm T} \cdot \cos(2 \pi f_{\rm T} t + \phi_{\rm T})= A_{\rm T} \cdot \cos(2 \pi f_{\rm T}( t - \tau)) \hspace{0.05cm},$$
- $$ z_+(t) = A_{\rm 0} \cdot {\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm T}\hspace{0.05cm}\cdot \hspace{0.05cm}t}$$
The two amplitude parameters $A_{\rm T} $ and $A_0$ are each dimensionless, the phase value $ϕ_{\rm T} $ is supposed to lie between $\text{±π}$ , and the duration τ $τ$ is non-negative.
Subtask (4) refers to the equivalent lowpass signal $z_{\rm TP}(t)$, which is related to $z_+(t)$ as follows:
- $$z_{\rm TP}(t) = z_+(t) \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm T}\hspace{0.05cm}\cdot \hspace{0.05cm}t}.$$
Further note that $ϕ_{\rm T}$ ppears in the above equation with a positive sign. See "Notes on Nomenclature" below for reasons for the differential usage of $φ_{\rm T}$ and $ϕ_{\rm T} = – φ_{\rm T}$.
Notes on Nomenclature:
- In this tutorial, as is common in other literature, the phase enters the equations with a negative sign when describing harmonic oscillation, Fourier series, and Fourier integrals, whereas in the context of modulation methods, the phase is always given a plus sign.
- To distinguish these two variants, we use $\phi_{\rm T}$ and $\varphi_{\rm T} = - \phi_{\rm T}$. Both symbols denote the lowercase Greek "phi", with the notation $\phi$ used predominantly in Anglo-American contexts, and $\varphi$ in German.
- The phase values $\varphi_{\rm T} = 90^\circ$ and $\phi_{\rm T} = -90^\circ$ are thus equivalent and both represent the sine function:
- $$\cos(2 \pi f_0 t - 90^{\circ}) = \cos(2 \pi f_0 t - \varphi_{\rm T}) = \cos(2 \pi f_0 t + \phi_{\rm T}) = \sin(2 \pi f_0 t ).$$
Further hints:
- This exercise belongs to the chapter General Model of Modulation.
- Particular reference is made to the page Describing the physical signal using the equivalent lowpass signal.
- Further information on this topic can be found in the following chapters of the book "Signal Representation":
- In our tutorial $\rm LNTwww$, the plot of the analytical signal $s_+(t)$ in the complex plane is sometimes referred to as the "pointer diagram", while the "locus curve" gives the time course of the equivalent lowpass signal $s_{\rm TP}(t)$ . We refer you to the corresponding interactive Applets
Questions
Solution
- the (normalized) amplitude $A_{\rm T}\hspace{0.15cm}\underline{ = 2}$ and the period $T_0=2$ milliseconds.
- Therefore, the signal frequency is $f_{\rm T} = 1/T_0\hspace{0.15cm}\underline{ = 500}$ Hz and the angular frequency is $ω_{\rm T}= 2πf_{\rm T} \hspace{0.15cm}\underline{ = 3141.5}$ 1/s.
(2) The analytical signal is generally:
- $$z_+(t) = A_{\rm T} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm}(\omega_{\rm T}\cdot \hspace{0.05cm}t + \phi_{\rm T})} = A_{\rm T} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} \phi_{\rm T}} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} \hspace{0.03cm}\omega_{\rm T}\cdot \hspace{0.05cm}t }\hspace{0.05cm}.$$
- At the same time the relationship:
- $$A_0 = z_+(t = 0) = A_{\rm T} \cdot {\rm e}^{{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} \phi_{\rm T}} \hspace{0.05cm}.$$
- The complex amplitude $A_0$ can be read from the upper plot.
- $$A_0 = - \sqrt{2} - {\rm j} \cdot \sqrt{2} = A_{\rm 0} \cdot {\rm e}^{-{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} 0.75 \pi} \hspace{0.05cm}.$$
- A comparison of both equations leads to the result:
- $$ \phi_{\rm T} = - 0.75 \pi \hspace{0.15cm}\underline {= - 135^{\circ}} \hspace{0.05cm}.$$
- Thereby, the following relationship exists with the duration $τ$:
- $$\phi_{\rm T} = - 2 \pi \cdot f_{\rm T} \cdot \tau \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \tau = \frac{-\phi_{\rm T}}{2 \pi \cdot f_{\rm T}} = \frac{0.75 \pi}{2 \pi \cdot 0.5\,{\rm kHz}} \hspace{0.15cm}\underline {= 0.75 \,{\rm ms}} \hspace{0.05cm}.$$
(3) Das analytische Signal legt in der Zeit $T_0$ genau eine Umdrehung zurück.
- Ausgehend von $A_0$ erreicht man somit nach $t_1 = T_0/8\hspace{0.15cm}\underline{ = 0.25}$ ms zum ersten Mal, dass das analytische Signal imaginär ist:
- $$z_+(t_1) = - 2 {\rm j}.$$
- Wegen der Beziehung $z(t) = {\rm Re}[z_+(t)]$ tritt zu diesem Zeitpunkt $t_1$ auch der erste Nulldurchgang des Signals $z(t)$ auf.
(4) Mit dem Ergebnis der Teilaufgabe (2) erhält man:
- $$ z_{\rm TP}(t) = z_+(t) \cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm T}\cdot \hspace{0.05cm}t} = A_0 = A_{\rm T} \cdot {\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} \phi_{\rm T}} = {\rm const.}$$
- Somit gilt für alle Zeiten $t$ und damit auch für $t = 1$ ms:
- $${\rm Re}[z_{\rm TP}(t)] = - \sqrt{2} \hspace{0.15cm}\underline {= -1.414} \hspace{0.05cm},$$
- $$ {\rm Im}[z_{\rm TP}(t)] = - \sqrt{2}\hspace{0.15cm}\underline {= -1.414} \hspace{0.05cm}.$$
(5) Richtig sind die Aussagen 1, 3 und 4:
- Die einzige Diracfunktion von $Z_+(f)$ liegt bei $f = f_{\rm T}$ und nicht bei $–f_{\rm T}$.
- Das analytische Signal einer harmonischen Schwingung ist immer komplex.
- Das äquivalente TP–Signal einer harmonischen Schwingung ist meistens komplex. Ausnahme:
- $$z(t) = ±A_{\rm T} · \cos(ω_{\rm T} · t) \ \Rightarrow \ z_{\rm TP}(t) = ±A_{\rm T}.$$