Difference between revisions of "Aufgaben:Exercise 2.13: Decoding at RSC (7, 3, 5) to Base 8"
m (Text replacement - "”" to """) |
|||
| (10 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Channel_Coding/Error_Correction_According_to_Reed-Solomon_Coding}} |
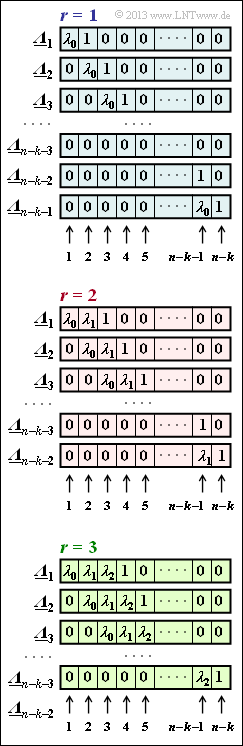
| − | [[File:P_ID2561__KC_A_2_12.png|right|frame| | + | [[File:P_ID2561__KC_A_2_12.png|right|frame|ELP allocation schemes for $r = 1, \ r = 2, \ r = 3$]] |
| − | In | + | In the [[Aufgaben:Exercise_2.12:_Decoding_at_RSC_(7,_4,_4)_to_Base_8|"Exercise 2.12"]] we used the so-called Petersen algorithm |
| + | *for error correction respective | ||
| − | In | + | *for decoding |
| + | |||
| + | |||
| + | the Reed–Solomon code $(7, \, 4, \, 4)_8$ which can due to the minimum distance $d_{\rm min} = 4$ $(t = 1)$ only correct one symbol error. | ||
| + | |||
| + | In this exercise we now consider the ${\rm RSC} \, (7, \, 3, \, 5)_8 \ \Rightarrow \ d_{\rm min} = 5 \ \Rightarrow \ t = 2$, whose parity-check matrix is as follows: | ||
:$${ \boldsymbol{\rm H}} = | :$${ \boldsymbol{\rm H}} = | ||
\begin{pmatrix} | \begin{pmatrix} | ||
| Line 13: | Line 19: | ||
\end{pmatrix} .$$ | \end{pmatrix} .$$ | ||
| − | + | The received word under consideration $\underline{y} = (\alpha^2, \, \alpha^3, \, \alpha, \, \alpha^5, \, \alpha^4, \, \alpha^2, \, 1)$ results in the syndrome | |
:$$\underline{s} = \underline{y} \cdot \mathbf{H}^{\rm T} = (0, \, 1, \, \alpha^5, \, \alpha^2).$$ | :$$\underline{s} = \underline{y} \cdot \mathbf{H}^{\rm T} = (0, \, 1, \, \alpha^5, \, \alpha^2).$$ | ||
| − | + | The further decoding procedure is done according to the following theory pages: | |
| − | * | + | * Step $\rm (B)$: [[Channel_Coding/Error_Correction_According_to_Reed-Solomon_Coding#Step_.28B.29:_Set_up.2Fevaluate_the_ELP_coefficient_vector| "Set up/evaluate the ELP coefficient vector"]]; "ELP" stands for "Error Locator Polynomial". |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| + | * Step $\rm (C)$: [[Channel_Coding/Error_Correction_According_to_Reed-Solomon_Coding#Step_.28C.29:_Localization_of_the_error_positions| "Localization of error positions"]], | ||
| + | * Step $\rm (D)$: [[Channel_Coding/Error_Correction_According_to_Reed-Solomon_Coding#Step_.28D.29:_Final_error_correction| "Final error correction"]]. | ||
| − | + | Hints: | |
| − | * | + | * This exercise belongs to the chapter [[Channel_Coding/Error_Correction_According_to_Reed-Solomon_Coding| "Error correction According to Reed–Solomon Coding"]]. |
| − | |||
| − | |||
| + | * In the above graphic you can see the assignments of the [[Channel_Coding/Error_Correction_According_to_Reed-Solomon_Coding#Error_Locator_Polynomial_-_Definition_and_Properties|"ELP coefficients"]] ${\it \underline{\Lambda}}_l$ assuming that there are symbol errors in the received words $r = 1, \ r = 2$ respectively. $r = 3$ . | ||
| − | === | + | ===Questions=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which allocation schemes might be relevant for this exercise? |
|type="[]"} | |type="[]"} | ||
| − | + | + | + The schema highlighted in blue $(r = 1)$. |
| − | + | + | + The schema highlighted in red $(r = 2)$. |
| − | - | + | - The scheme highlighted in green $(r = 3)$. |
| − | { | + | {Can the syndrome $\underline{s} = (0, \, 1, \, \alpha^5, \, \alpha^2)$ be caused by one symbol error? |
|type="()"} | |type="()"} | ||
| − | - | + | - YES. |
| − | + | + | + NO. |
| − | { | + | {Can the syndrome $\underline{s} = (0, \, 1, \, \alpha^5, \, \alpha^2)$ be caused by two symbol errors? |
|type="()"} | |type="()"} | ||
| − | + | + | + YES. |
| − | - | + | - NO. |
| − | { | + | {Which symbols of the code word have been falsified? |
|type="[]"} | |type="[]"} | ||
- Symbol 0, | - Symbol 0, | ||
| Line 64: | Line 66: | ||
- Symbol 6. | - Symbol 6. | ||
| − | { | + | {What is the error vector $\underline{e}$? Give also the decoding result $\underline{z} = \underline{y} + \underline{e}$ . |
|type="()"} | |type="()"} | ||
- $\underline{e} = (1, \, 0, \, 0, \, 0, \, 0, \, 0, \, \alpha^6)$, | - $\underline{e} = (1, \, 0, \, 0, \, 0, \, 0, \, 0, \, \alpha^6)$, | ||
| Line 72: | Line 74: | ||
</quiz> | </quiz> | ||
| − | === | + | ===Solution=== |
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Correct are the <u>proposed solutions 1 and 2</u>: |
| − | * | + | *The ${\rm RSC} \, (7, \, 3, \, 5)_8$ can correct up to $t = 2$ symbol errors. |
| − | * | + | |
| + | *The actual symbol error count $r$ must not be larger. | ||
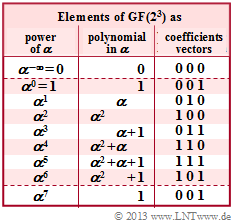
| − | [[File: | + | [[File:EN_KC_Z_2_5_neu.png|right|frame|Conversion tables for $\rm GF(2^3)$]] |
| − | '''(2)''' | + | '''(2)''' Assuming $r = 1$, the $n-k-1$ governing equations ${\it \underline{\Lambda}}_l \cdot \underline{s}^{\rm T} = 0$ for $\lambda_0$ are as follows : |
| − | :$$\lambda_0 \cdot 0 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} 1 = 0 \hspace{0.75cm} \Rightarrow \hspace{0.3cm} \lambda_0 \hspace{0.15cm} {\rm | + | :$$\lambda_0 \cdot 0 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} 1 = 0 \hspace{0.75cm} \Rightarrow \hspace{0.3cm} \lambda_0 \hspace{0.15cm} {\rm unspecified} \hspace{0.05cm},$$ |
:$$\lambda_0 \cdot 1 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \alpha^5 = 0 \hspace{0.55cm} \Rightarrow \hspace{0.3cm} \lambda_0 = \alpha^5 \hspace{0.05cm},$$ | :$$\lambda_0 \cdot 1 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \alpha^5 = 0 \hspace{0.55cm} \Rightarrow \hspace{0.3cm} \lambda_0 = \alpha^5 \hspace{0.05cm},$$ | ||
:$$\lambda_0 \cdot \alpha^5 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \alpha^2 = 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \lambda_0 = \alpha^{-3} = \alpha^4 \hspace{0.05cm}.$$ | :$$\lambda_0 \cdot \alpha^5 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \alpha^2 = 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \lambda_0 = \alpha^{-3} = \alpha^4 \hspace{0.05cm}.$$ | ||
| − | * | + | *The assumption $r = 1$ would be justified only if the same $\lambda_0$ value resulted from all these three equations. |
| − | * | + | |
| + | *This is not the case here ⇒ Answer <u>NO</u>. | ||
| − | '''(3)''' | + | '''(3)''' Assuming the occupation for $r = 2$, we obtain two equations of determination for $\lambda_0$ and $\lambda_1$: |
:$$\lambda_0 \cdot 0 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \lambda_1 \cdot 1 +\alpha^5 = 0 \hspace{0.38cm} \Rightarrow \hspace{0.3cm} \lambda_1 = \alpha^5 \hspace{0.05cm},$$ | :$$\lambda_0 \cdot 0 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \lambda_1 \cdot 1 +\alpha^5 = 0 \hspace{0.38cm} \Rightarrow \hspace{0.3cm} \lambda_1 = \alpha^5 \hspace{0.05cm},$$ | ||
:$$\lambda_0 \cdot 1 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \lambda_1 \cdot \alpha^5 +\alpha^2 = 0 \hspace{0.15cm} \Rightarrow \hspace{0.3cm} \lambda_0 = \alpha^{5+5} +\alpha^2 = \alpha^{3} +\alpha^2 =\alpha^{5} \hspace{0.05cm}.$$ | :$$\lambda_0 \cdot 1 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \lambda_1 \cdot \alpha^5 +\alpha^2 = 0 \hspace{0.15cm} \Rightarrow \hspace{0.3cm} \lambda_0 = \alpha^{5+5} +\alpha^2 = \alpha^{3} +\alpha^2 =\alpha^{5} \hspace{0.05cm}.$$ | ||
| − | * | + | *The system of equations can be solved under the assumption $r = 2$ ⇒ Answer <u>YES</u>. |
| − | * | + | |
| + | *The results $\lambda_0 = \lambda_1 = \alpha^5$ obtained here are processed in the next subtask. | ||
| − | '''(4)''' | + | '''(4)''' With the result $\lambda_0 = \lambda_1 = \alpha^5$, the error locator polynomial $($"key equation"$)$ is: |
:$${\it \Lambda}(x)=x \cdot \big ({\it \lambda}_0 + {\it \lambda}_1 \cdot x + x^2 \big ) | :$${\it \Lambda}(x)=x \cdot \big ({\it \lambda}_0 + {\it \lambda}_1 \cdot x + x^2 \big ) | ||
=x \cdot \big (\alpha^5 + \alpha^5 \cdot x + x^2 )\hspace{0.05cm}.$$ | =x \cdot \big (\alpha^5 + \alpha^5 \cdot x + x^2 )\hspace{0.05cm}.$$ | ||
| − | * | + | *This function has zeros for $x = \alpha^2$ and $x = \alpha^3$: |
:$${\it \Lambda}(x = \alpha^2 )\hspace{-0.15cm} \ = \ \hspace{-0.15cm} \alpha^2 \cdot \big ( \alpha^5 + \alpha^7 + \alpha^4 \big ) = \alpha^2 \cdot \big ( \alpha^5 + 1 + \alpha^4 \big )= 0\hspace{0.05cm},$$ | :$${\it \Lambda}(x = \alpha^2 )\hspace{-0.15cm} \ = \ \hspace{-0.15cm} \alpha^2 \cdot \big ( \alpha^5 + \alpha^7 + \alpha^4 \big ) = \alpha^2 \cdot \big ( \alpha^5 + 1 + \alpha^4 \big )= 0\hspace{0.05cm},$$ | ||
:$${\it \Lambda}(x = \alpha^3 )\hspace{-0.15cm} \ = \ \hspace{-0.15cm} \alpha^3 \cdot \big ( \alpha^5 + \alpha^8 + \alpha^6 \big ) = \alpha^3 \cdot \big ( \alpha^5 + \alpha + \alpha^6 \big )= 0\hspace{0.05cm}.$$ | :$${\it \Lambda}(x = \alpha^3 )\hspace{-0.15cm} \ = \ \hspace{-0.15cm} \alpha^3 \cdot \big ( \alpha^5 + \alpha^8 + \alpha^6 \big ) = \alpha^3 \cdot \big ( \alpha^5 + \alpha + \alpha^6 \big )= 0\hspace{0.05cm}.$$ | ||
| − | * | + | *Falsified are consequently the symbols at positions '''2''' and '''3 ⇒ <u>Solutions 3 and 4</u>. |
| − | '''(5)''' | + | '''(5)''' After the result of the subtask '''(4)''' only the last two proposed solutions come into question: |
:$$\underline{e} = (0, \, 0, \, e_2, \, e_3, \, 0, \, 0, \, 0).$$ | :$$\underline{e} = (0, \, 0, \, e_2, \, e_3, \, 0, \, 0, \, 0).$$ | ||
| − | * | + | *The approach is therefore accordingly $\underline{e} \cdot \mathbf{H}^{\rm T} = \underline{s}$: |
:$$(0, 0, e_2, e_3, 0, 0, 0) \cdot | :$$(0, 0, e_2, e_3, 0, 0, 0) \cdot | ||
\begin{pmatrix} | \begin{pmatrix} | ||
| Line 132: | Line 137: | ||
\hspace{0.6cm} e_2 \cdot \alpha^{1} + e_6 \cdot \alpha^{5} = \alpha^{2} \hspace{0.05cm}. $$ | \hspace{0.6cm} e_2 \cdot \alpha^{1} + e_6 \cdot \alpha^{5} = \alpha^{2} \hspace{0.05cm}. $$ | ||
| − | * | + | *All four equations are satisfied with $e_2 = 1$ as well as $e_3 = \alpha^6$ ⇒ <u>Proposed solution 3</u>: |
:$$\underline {e} = (0, \hspace{0.05cm}0, \hspace{0.05cm}1, \hspace{0.05cm}\alpha^6, \hspace{0.05cm}0, \hspace{0.05cm}0, \hspace{0.05cm}0) $$ | :$$\underline {e} = (0, \hspace{0.05cm}0, \hspace{0.05cm}1, \hspace{0.05cm}\alpha^6, \hspace{0.05cm}0, \hspace{0.05cm}0, \hspace{0.05cm}0) $$ | ||
| − | * | + | *With $\alpha + 1 = \alpha^3$ and $\alpha^5 + \alpha^6 = \alpha$ we get from the given received word $\underline{y} = (\alpha^2, \, \alpha^3, \, \alpha, \, \alpha^5, \, \alpha^4, \, \alpha^2, \, 1)$ to the decoding result |
:$$\underline {z} = (\alpha^2, \hspace{0.05cm}\alpha^3, \hspace{0.05cm}\alpha^3, \hspace{0.05cm}\alpha, \hspace{0.05cm}\alpha^4, \hspace{0.05cm}\alpha^2, \hspace{0.05cm}1) | :$$\underline {z} = (\alpha^2, \hspace{0.05cm}\alpha^3, \hspace{0.05cm}\alpha^3, \hspace{0.05cm}\alpha, \hspace{0.05cm}\alpha^4, \hspace{0.05cm}\alpha^2, \hspace{0.05cm}1) | ||
\hspace{0.05cm}. $$ | \hspace{0.05cm}. $$ | ||
| − | *In | + | *In the [[Aufgaben:Exercise_2.07:_Reed-Solomon_Code_(7,_3,_5)_to_Base_8|"Exercise 2.7"]] it was shown that this is a valid code word of ${\rm RSC} \, (7, \, 3, \, 5)_8$. |
| − | * | + | |
| + | *The corresponding information word is $\underline{u} = (\alpha^4, \, 1, \, \alpha^3)$. | ||
{{ML-Fuß}} | {{ML-Fuß}} | ||
| − | [[Category:Channel Coding: Exercises|^2.5 | + | [[Category:Channel Coding: Exercises|^2.5 Reed-Solomon Error Correction^]] |
Latest revision as of 17:30, 23 January 2023
In the "Exercise 2.12" we used the so-called Petersen algorithm
- for error correction respective
- for decoding
the Reed–Solomon code $(7, \, 4, \, 4)_8$ which can due to the minimum distance $d_{\rm min} = 4$ $(t = 1)$ only correct one symbol error.
In this exercise we now consider the ${\rm RSC} \, (7, \, 3, \, 5)_8 \ \Rightarrow \ d_{\rm min} = 5 \ \Rightarrow \ t = 2$, whose parity-check matrix is as follows:
- $${ \boldsymbol{\rm H}} = \begin{pmatrix} 1 & \alpha^1 & \alpha^2 & \alpha^3 & \alpha^4 & \alpha^5 & \alpha^6\\ 1 & \alpha^2 & \alpha^4 & \alpha^6 & \alpha^1 & \alpha^{3} & \alpha^{5}\\ 1 & \alpha^3 & \alpha^6 & \alpha^2 & \alpha^{5} & \alpha^{1} & \alpha^{4}\\ 1 & \alpha^4 & \alpha^1 & \alpha^{5} & \alpha^{2} & \alpha^{6} & \alpha^{3} \end{pmatrix} .$$
The received word under consideration $\underline{y} = (\alpha^2, \, \alpha^3, \, \alpha, \, \alpha^5, \, \alpha^4, \, \alpha^2, \, 1)$ results in the syndrome
- $$\underline{s} = \underline{y} \cdot \mathbf{H}^{\rm T} = (0, \, 1, \, \alpha^5, \, \alpha^2).$$
The further decoding procedure is done according to the following theory pages:
- Step $\rm (B)$: "Set up/evaluate the ELP coefficient vector"; "ELP" stands for "Error Locator Polynomial".
- Step $\rm (C)$: "Localization of error positions",
- Step $\rm (D)$: "Final error correction".
Hints:
- This exercise belongs to the chapter "Error correction According to Reed–Solomon Coding".
- In the above graphic you can see the assignments of the "ELP coefficients" ${\it \underline{\Lambda}}_l$ assuming that there are symbol errors in the received words $r = 1, \ r = 2$ respectively. $r = 3$ .
Questions
Solution
- The ${\rm RSC} \, (7, \, 3, \, 5)_8$ can correct up to $t = 2$ symbol errors.
- The actual symbol error count $r$ must not be larger.
(2) Assuming $r = 1$, the $n-k-1$ governing equations ${\it \underline{\Lambda}}_l \cdot \underline{s}^{\rm T} = 0$ for $\lambda_0$ are as follows :
- $$\lambda_0 \cdot 0 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} 1 = 0 \hspace{0.75cm} \Rightarrow \hspace{0.3cm} \lambda_0 \hspace{0.15cm} {\rm unspecified} \hspace{0.05cm},$$
- $$\lambda_0 \cdot 1 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \alpha^5 = 0 \hspace{0.55cm} \Rightarrow \hspace{0.3cm} \lambda_0 = \alpha^5 \hspace{0.05cm},$$
- $$\lambda_0 \cdot \alpha^5 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \alpha^2 = 0 \hspace{0.3cm} \Rightarrow \hspace{0.3cm} \lambda_0 = \alpha^{-3} = \alpha^4 \hspace{0.05cm}.$$
- The assumption $r = 1$ would be justified only if the same $\lambda_0$ value resulted from all these three equations.
- This is not the case here ⇒ Answer NO.
(3) Assuming the occupation for $r = 2$, we obtain two equations of determination for $\lambda_0$ and $\lambda_1$:
- $$\lambda_0 \cdot 0 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \lambda_1 \cdot 1 +\alpha^5 = 0 \hspace{0.38cm} \Rightarrow \hspace{0.3cm} \lambda_1 = \alpha^5 \hspace{0.05cm},$$
- $$\lambda_0 \cdot 1 \hspace{-0.15cm} \ + \ \hspace{-0.15cm} \lambda_1 \cdot \alpha^5 +\alpha^2 = 0 \hspace{0.15cm} \Rightarrow \hspace{0.3cm} \lambda_0 = \alpha^{5+5} +\alpha^2 = \alpha^{3} +\alpha^2 =\alpha^{5} \hspace{0.05cm}.$$
- The system of equations can be solved under the assumption $r = 2$ ⇒ Answer YES.
- The results $\lambda_0 = \lambda_1 = \alpha^5$ obtained here are processed in the next subtask.
(4) With the result $\lambda_0 = \lambda_1 = \alpha^5$, the error locator polynomial $($"key equation"$)$ is:
- $${\it \Lambda}(x)=x \cdot \big ({\it \lambda}_0 + {\it \lambda}_1 \cdot x + x^2 \big ) =x \cdot \big (\alpha^5 + \alpha^5 \cdot x + x^2 )\hspace{0.05cm}.$$
- This function has zeros for $x = \alpha^2$ and $x = \alpha^3$:
- $${\it \Lambda}(x = \alpha^2 )\hspace{-0.15cm} \ = \ \hspace{-0.15cm} \alpha^2 \cdot \big ( \alpha^5 + \alpha^7 + \alpha^4 \big ) = \alpha^2 \cdot \big ( \alpha^5 + 1 + \alpha^4 \big )= 0\hspace{0.05cm},$$
- $${\it \Lambda}(x = \alpha^3 )\hspace{-0.15cm} \ = \ \hspace{-0.15cm} \alpha^3 \cdot \big ( \alpha^5 + \alpha^8 + \alpha^6 \big ) = \alpha^3 \cdot \big ( \alpha^5 + \alpha + \alpha^6 \big )= 0\hspace{0.05cm}.$$
- Falsified are consequently the symbols at positions 2 and 3 ⇒ Solutions 3 and 4.
(5) After the result of the subtask (4) only the last two proposed solutions come into question:
- $$\underline{e} = (0, \, 0, \, e_2, \, e_3, \, 0, \, 0, \, 0).$$
- The approach is therefore accordingly $\underline{e} \cdot \mathbf{H}^{\rm T} = \underline{s}$:
- $$(0, 0, e_2, e_3, 0, 0, 0) \cdot \begin{pmatrix} 1 & 1 & 1 & 1\\ \alpha^1 & \alpha^2 & \alpha^3 & \alpha^4\\ \alpha^2 & \alpha^4 & \alpha^6 & \alpha^1\\ \alpha^3 & \alpha^6 & \alpha^2 & \alpha^5\\ \alpha^4 & \alpha^1 & \alpha^{5} & \alpha^2\\ \alpha^5 & \alpha^{3} & \alpha^{1} & \alpha^6\\ \alpha^6 & \alpha^{5} & \alpha^{4} & \alpha^3 \end{pmatrix} \hspace{0.15cm}\stackrel{!}{=} \hspace{0.15cm} (0, 1, \alpha^5, \alpha^2) \hspace{0.05cm}. $$
- $$\Rightarrow \hspace{0.3cm} e_2 \cdot \alpha^{2} \hspace{-0.15cm} \ + \ \hspace{-0.15cm} e_3 \cdot \alpha^{3} = 0 \hspace{0.05cm}, \hspace{0.8cm} e_2 \cdot \alpha^{4} + e_6 \cdot \alpha^{3} = 1\hspace{0.05cm},\hspace{0.8cm} e_2 \cdot \alpha^{6} \hspace{-0.15cm} \ + \ \hspace{-0.15cm} e_3 \cdot \alpha^{2} = \alpha^{5} \hspace{0.05cm}, \hspace{0.6cm} e_2 \cdot \alpha^{1} + e_6 \cdot \alpha^{5} = \alpha^{2} \hspace{0.05cm}. $$
- All four equations are satisfied with $e_2 = 1$ as well as $e_3 = \alpha^6$ ⇒ Proposed solution 3:
- $$\underline {e} = (0, \hspace{0.05cm}0, \hspace{0.05cm}1, \hspace{0.05cm}\alpha^6, \hspace{0.05cm}0, \hspace{0.05cm}0, \hspace{0.05cm}0) $$
- With $\alpha + 1 = \alpha^3$ and $\alpha^5 + \alpha^6 = \alpha$ we get from the given received word $\underline{y} = (\alpha^2, \, \alpha^3, \, \alpha, \, \alpha^5, \, \alpha^4, \, \alpha^2, \, 1)$ to the decoding result
- $$\underline {z} = (\alpha^2, \hspace{0.05cm}\alpha^3, \hspace{0.05cm}\alpha^3, \hspace{0.05cm}\alpha, \hspace{0.05cm}\alpha^4, \hspace{0.05cm}\alpha^2, \hspace{0.05cm}1) \hspace{0.05cm}. $$
- In the "Exercise 2.7" it was shown that this is a valid code word of ${\rm RSC} \, (7, \, 3, \, 5)_8$.
- The corresponding information word is $\underline{u} = (\alpha^4, \, 1, \, \alpha^3)$.