Difference between revisions of "Aufgaben:Exercise 3.1: Causality Considerations"
| Line 75: | Line 75: | ||
===Solution=== | ===Solution=== | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' <u>Proposed solution 2</u> is cocrrect: |
| − | * | + | *The given transfer function can be computed according to the voltage divider principle. The following holds: |
:$$H_1(f = 0) = 0, \hspace{0.2cm}H_1(f \rightarrow \infty) = 1$$ | :$$H_1(f = 0) = 0, \hspace{0.2cm}H_1(f \rightarrow \infty) = 1$$ | ||
| − | * | + | *This is a high-pass filter. |
| − | * | + | *For very low frequencies, the inductivity $L$ constitutes a short circuit. |
| − | '''(2)''' | + | '''(2)''' <u>Yes</u> is cocrrect: |
| − | * | + | *Every real network is causal. The impulse response $h(t)$ is equal to the output signal $y(t)$ if at time $t= 0$ an extremely short impulse – a so-called Dirac impulse – is applied to the input. |
| − | * | + | *Then, a signal of course cannot occur at the output already for times $t< 0$ for causality reasons: |
:$$y(t) = h(t) = 0 \hspace{0.2cm}{\rm{f\ddot{u}r}} \hspace{0.2cm} | :$$y(t) = h(t) = 0 \hspace{0.2cm}{\rm{f\ddot{u}r}} \hspace{0.2cm} | ||
t<0 \hspace{0.05cm}.$$ | t<0 \hspace{0.05cm}.$$ | ||
| − | * | + | *Formally, this can be shown as follows: The high-pass–transfer function $H_1(f)$ can be rearranged as follows: |
:$$H_1(f) = \frac{{\rm j}\cdot f/f_{\rm G}}{1+{\rm j}\cdot f/f_{\rm G}} | :$$H_1(f) = \frac{{\rm j}\cdot f/f_{\rm G}}{1+{\rm j}\cdot f/f_{\rm G}} | ||
= 1- \frac{1}{1+{\rm j}\cdot f/f_{\rm G}} | = 1- \frac{1}{1+{\rm j}\cdot f/f_{\rm G}} | ||
\hspace{0.05cm}.$$ | \hspace{0.05cm}.$$ | ||
| − | * | + | *The second transfer function describes the low-pass function equivalent to $H_1(f)$, which results in the exponential function in the time domain. |
| − | * | + | *The "$1$" becomes a Dirac function. Considering $T = 2\pi \cdot f_{\rm G}$ the following thus holds for $t \ge 0$: |
:$$h_1(t) = \delta(t) - {1}/{T} \cdot {\rm e}^{-t/T} \hspace{0.05cm}.$$ | :$$h_1(t) = \delta(t) - {1}/{T} \cdot {\rm e}^{-t/T} \hspace{0.05cm}.$$ | ||
| − | * | + | *In contrast, $h_1(t)= 0$ holds for $t< 0$ , which would prove causality. |
Revision as of 08:59, 24 September 2021
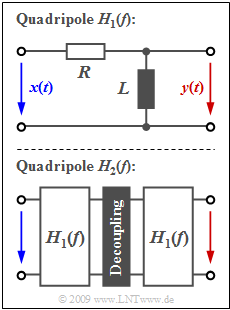
The graph shows above the two-port network with the transfer function
- $$H_1(f) = \frac{{\rm j}\cdot f/f_{\rm G}}{1+{\rm j}\cdot f/f_{\rm G}} \hspace{0.05cm},$$
where $f_{\rm G}$ represents the 3dB cut-off frequency:
- $$f_{\rm G} = \frac{R}{2 \pi \cdot L} \hspace{0.05cm}.$$
By cascading $n$ two-port networks $H_1(f)$ built the same way, the transfer function
- $$H_n(f) = \big [H_1(f)\big ]^n =\frac{\big [{\rm j}\cdot f/f_{\rm G}\big ]^n}{\big [1+{\rm j}\cdot f/f_{\rm G}\big ]^n} \hspace{0.05cm}$$ is obtained.
- Here, a suitable resistor decoupling is presumed, but this is not important for solving this exercise.
- The lower graph shows for example the realization of the transfer function $H_2(f)$.
In this exercise, such a two-port network is considered with respect to its causality properties.
For any causal system, the real and imaginary parts of the spectral function $H(f)$ satisfy the Hilbert transformation, which is expressed by the following abbreviation:
- $${\rm Im} \left\{ H(f) \right \} \quad \bullet\!\!-\!\!\!-\!\!\!-\!\!\hspace{-0.05cm}\rightarrow\quad {\rm Re} \left\{ H(f) \right \}\hspace{0.05cm}.$$
Since the Hilbert transformation provides important information not only for transfer functions but also for time signals, the correspondence is often expressed by the general variable $x$ , which is to be interpreted - depending on the application - as normalized frequency or normalized time.
Please note:
- The exercise belongs to the chapter Conclusions from the Allocation Theorem.
- Reference is also made to the theory pages Hilbert transformation and Partial fraction decomposition.
Questions
Solution
- The given transfer function can be computed according to the voltage divider principle. The following holds:
- $$H_1(f = 0) = 0, \hspace{0.2cm}H_1(f \rightarrow \infty) = 1$$
- This is a high-pass filter.
- For very low frequencies, the inductivity $L$ constitutes a short circuit.
(2) Yes is cocrrect:
- Every real network is causal. The impulse response $h(t)$ is equal to the output signal $y(t)$ if at time $t= 0$ an extremely short impulse – a so-called Dirac impulse – is applied to the input.
- Then, a signal of course cannot occur at the output already for times $t< 0$ for causality reasons:
- $$y(t) = h(t) = 0 \hspace{0.2cm}{\rm{f\ddot{u}r}} \hspace{0.2cm} t<0 \hspace{0.05cm}.$$
- Formally, this can be shown as follows: The high-pass–transfer function $H_1(f)$ can be rearranged as follows:
- $$H_1(f) = \frac{{\rm j}\cdot f/f_{\rm G}}{1+{\rm j}\cdot f/f_{\rm G}} = 1- \frac{1}{1+{\rm j}\cdot f/f_{\rm G}} \hspace{0.05cm}.$$
- The second transfer function describes the low-pass function equivalent to $H_1(f)$, which results in the exponential function in the time domain.
- The "$1$" becomes a Dirac function. Considering $T = 2\pi \cdot f_{\rm G}$ the following thus holds for $t \ge 0$:
- $$h_1(t) = \delta(t) - {1}/{T} \cdot {\rm e}^{-t/T} \hspace{0.05cm}.$$
- In contrast, $h_1(t)= 0$ holds for $t< 0$ , which would prove causality.
(3) Die Hintereinanderschaltung zweier Hochpässe führt zu folgender Übertragungsfunktion:
- $$H_2(f) = \big [H_1(f)\big ]^2 =\frac{\big [{\rm j}\cdot f/f_{\rm G}\big ]^2}{\big [1+{\rm j}\cdot f/f_{\rm G}\big ]^2} =\frac{\big [{\rm j}\cdot f/f_{\rm G}\big ]^2 \cdot \big [(1-{\rm j}\cdot f/f_{\rm G})\big ]^2} {\big [(1+{\rm j}\cdot f/f_{\rm G}) \cdot (1-{\rm j}\cdot f/f_{\rm G})\big ]^2}= \frac{(f/f_{\rm G})^4 - (f/f_{\rm G})^2 +{\rm j}\cdot 2 \cdot (f/f_{\rm G})^3)} {\big [1+(f/f_{\rm G})^2 \big ]^2}\hspace{0.05cm}.$$
- Mit $f = f_{\rm G}$ folgt daraus:
- $$H_2(f = f_{\rm G}) = \frac{1 - 1 +{\rm j}\cdot 2} {4}= {\rm j} /{2} \hspace{0.5cm}\Rightarrow \hspace{0.5cm}{\rm Re} \left\{ H_2(f = f_{\rm G}) \right \} \hspace{0.15cm}\underline{ = 0}, \hspace{0.4cm} {\rm Im} \left\{ H_2(f = f_{\rm G}) \right \} \hspace{0.15cm}\underline{ = 0.5}\hspace{0.05cm}.$$
(4) Richtig sind die beiden ersten Lösungsvorschläge:
- Da für $t < 0$ die Impulsantwort $h_1(t) = 0$ ist, erfüllt auch die Faltungsoperation $h_2(t) = h_1(t) \star h_1(t)$ die Kausalitätsbedingung. Ebenso ergibt die $n$–fache Faltung eine kausale Impulsantwort: $h_n(t) = 0 \hspace{0.2cm}{\rm{f\ddot{u}r}} \hspace{0.2cm} t<0 \hspace{0.05cm}.$
- Bei kausaler Impulsantwort $h_2(t)$ hängen aber der Real– und der Imaginärteil der Spektralfunktion $H_2(f)$ über die Hilbert–Transformation zusammen. Mit der Abkürzung $x = f/f_{\rm G}$ und dem Ergebnis der Teilaufgabe (3) gilt somit:
- $$\frac{x^4- x^2}{x^4+2 x^2+1} \quad \bullet\!\!-\!\!\!-\!\!\!-\!\!\hspace{-0.05cm}\rightarrow\quad \frac{2x^3}{x^4+2 x^2+1}\hspace{0.05cm}.$$