Difference between revisions of "Aufgaben:Exercise 5.4: Walsh Functions (PCCF, PACF)"

From LNTwww
m (Text replacement - "Category:Aufgaben zu Modulationsverfahren" to "Category:Modulation Methods: Exercises")
 
(6 intermediate revisions by 2 users not shown)
Line 1: Line 1:
  
−
{{quiz-Header|Buchseite=Modulationsverfahren/Spreizfolgen für CDMA
+
{{quiz-Header|Buchseite=Modulation_Methods/Spreading_Sequences_for_CDMA
 
}}
 
}}
  
−
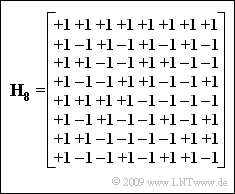
[[File:P_ID1889__Mod_A_5_4.png|right|frame|Hadamard–Matrix  ${\mathbf{H}_{8}}$]]
+
[[File:P_ID1889__Mod_A_5_4.png|right|frame|Hadamard matrix  ${\mathbf{H}_{8}}$]]
−
Häufig verwendet man zur Bandspreizung und Bandstauchung so genannte  ''Walsh–Funktionen'', die mittels der Hadamard–Matrix konstruiert werden können. Ausgehend von der Matrix
+
The so-called  "Walsh functions",  which can be constructed by means of the Hadamard matrix,  are often used for band spreading and band compression.  Starting from the matrix
 
:$${\mathbf{H}_{2}} = \left[ \begin{array}{ccc} +1 & +1 \\ +1 & -1 \end{array} \right] $$
 
:$${\mathbf{H}_{2}} = \left[ \begin{array}{ccc} +1 & +1 \\ +1 & -1 \end{array} \right] $$
−
lassen sich durch folgende Rekursion die weiteren Hadamard–Matrizen  $ {\mathbf{H}_{4}}$,  $ {\mathbf{H}_{8}}$, usw. herleiten:
+
the further Hadamard matrices  $ {\mathbf{H}_{4}}$,  $ {\mathbf{H}_{8}}$,  etc. can be derived by the following recursion:
 
:$$ {\mathbf{H}_{2J}} = \left[ \begin{array}{ccc} \mathbf{H}_J & \mathbf{H}_J \\ \mathbf{H}_J & -\mathbf{H}_J \end{array} \right] \hspace{0.05cm}.$$
 
:$$ {\mathbf{H}_{2J}} = \left[ \begin{array}{ccc} \mathbf{H}_J & \mathbf{H}_J \\ \mathbf{H}_J & -\mathbf{H}_J \end{array} \right] \hspace{0.05cm}.$$
−
Die Grafik zeigt die Matrix  $ {\mathbf{H}_{8}}$  für den Spreizfaktor  $J = 8$.  Daraus lassen sich die Spreizfolgen
+
The diagram shows the matrix  $ {\mathbf{H}_{8}}$  for the spreading factor  $J = 8$.  From this we can derive the spreading sequences
 
:$$ \langle w_\nu^{(1)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
 
:$$ \langle w_\nu^{(1)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
 
:$$ \langle w_\nu^{(2)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
 
:$$ \langle w_\nu^{(2)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
 
:$$...$$
 
:$$...$$
 
:$$\langle w_\nu^{(7)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}$$
 
:$$\langle w_\nu^{(7)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}$$
−
für sieben CDMA–Teilnehmer ablesen.  Die Spreizfolge  $ \langle w_\nu^{(0)}\rangle$  entsprechend der ersten Zeile in der Hadamard–Matrix wird meistens nicht vergeben, da sie nicht spreizt.
+
for seven CDMA subscribers.  The spreading sequence  $ \langle w_\nu^{(0)}\rangle$  corresponding to the first row in the Hadamard matrix is usually not assigned because it does not spread.
  
−
Die Fragen beziehen sich meist auf den Spreizfaktor  $J = 4$.  Damit können entsprechend mit den Spreizfolgen  $ \langle w_\nu^{(1)}\rangle$,  $ \langle w_\nu^{(2)}\rangle$  und  $ \langle w_\nu^{(3)}\rangle$  maximal drei CDMA–Teilnehmer versorgt werden, die sich aus der zweiten, dritten und vierten Zeile der Matrix $ {\mathbf{H}_{4}}$ ergeben.
+
The questions mostly refer to the spreading factor  $J = 4$.  Thus,  correspondingly,  a maximum of three CDMA subscribers can be supplied with the spreading sequences  $ \langle w_\nu^{(1)}\rangle$,  $ \langle w_\nu^{(2)}\rangle$  and  $ \langle w_\nu^{(3)}\rangle$,  which result from the second, third and fourth rows of the matrix $ {\mathbf{H}_{4}}$.
  
−
Hinsichtlich der Korrelationsfunktionen soll in dieser Aufgabe folgende Nomenklatur gelten:
+
Regarding the correlation functions, the following nomenclature shall apply in this exercise:
−
* Die  [[Modulation_Methods/Spreizfolgen_für_CDMA#Periodische_AKF_und_KKF|periodische Kreuzkorrelationsfunktion]]  (PKKF) zwischen den Folgen  $ \langle w_\nu^{(i)}\rangle$  und  $ \langle w_\nu^{(j)}\rangle$  wird mit  $φ_{ij}(λ)$  bezeichnet.  Hierbei gilt:
+
* The  [[Modulation_Methods/Spreading_Sequences_for_CDMA#Periodic_ACF_and_CCF|periodic cross-correlation function]]  $\rm (PCCF)$  between the sequences  $ \langle w_\nu^{(i)}\rangle$  and  $ \langle w_\nu^{(j)}\rangle$  is denoted by  $φ_{ij}(λ)$.   Here:
 
:$${\it \varphi}_{ij}(\lambda) = {\rm E}\left [ w_{\nu}^{(i)} \cdot w_{\nu+ \lambda}^{(j)} \right ] \hspace{0.05cm}.$$
 
:$${\it \varphi}_{ij}(\lambda) = {\rm E}\left [ w_{\nu}^{(i)} \cdot w_{\nu+ \lambda}^{(j)} \right ] \hspace{0.05cm}.$$
−
* Ist  $φ_{ij} \equiv 0$  $($das heißt:  $φ_{ij}(λ) = 0$  für alle Werte von  $λ)$, so stören sich die CDMA–Teilnehmer nicht, auch wenn diese unterschiedliche Laufzeiten aufweisen.
+
* If  $φ_{ij} \equiv 0$  $($that is:  $φ_{ij}(λ) = 0$  for all values of  $λ)$,  the CDMA subscribers do not interfere with each other,  even if they have different propagation times.
−
* Gilt zumindest  $φ_{ij}({\it λ} = 0) = 0$, so kommt es zumindest bei synchronem CDMA–Betrieb  $($keine oder gleiche Laufzeiten aller Teilnehmer$)$  zu keinen Interferenzen.
+
* If at least  $φ_{ij}({\it λ} = 0) = 0$  applies,  then no interference occurs,  at least in synchronous CDMA operation  $($no or equal propagation times of all subscribers$).$   
−
* Die   [[Modulation_Methods/Spreizfolgen_für_CDMA#Periodische_AKF_und_KKF|periodische  Autokorrelationsfunktion]]  (PAKF) der Walsh–Funktion  $ \langle w_\nu^{(i)}\rangle$  wird mit  $φ_{ii}(λ)$  bezeichnet, und es gilt:
+
* The  [[Modulation_Methods/Spreading_Sequences_for_CDMA#Periodic_ACF_and_CCF|periodic auto-correlation function]]  $\rm (PACF)$  of the Walsh function  $ \langle w_\nu^{(i)}\rangle$  is denoted by  $φ_{ii}(λ)$,  and it holds:
 
:$${\it \varphi}_{ii}(\lambda) = {\rm E}\left [ w_{\nu}^{(i)} \cdot w_{\nu+ \lambda}^{(i)} \right ] \hspace{0.05cm}.$$
 
:$${\it \varphi}_{ii}(\lambda) = {\rm E}\left [ w_{\nu}^{(i)} \cdot w_{\nu+ \lambda}^{(i)} \right ] \hspace{0.05cm}.$$
  
  
  
 +
Notes:
 +
*The exercise belongs to the chapter  [[Modulation_Methods/Spreizfolgen_für_CDMA|Spreading Sequences for CDMA]].
 +
*Reference is made in particular to the section  [[Modulation_Methods/Spreading_Sequences_for_CDMA#Walsh_functions|Walsh functions]]  in the theory part.
 +
* We would also like to draw your attention to the interactive applet  [[Applets:Generation_of_Walsh_functions|Generation of Walsh functions]]. 
 +
*The abscissa is normalized to the chip duration  $T_c$.  This means that  $λ = 1$  actually describes a shift by the delay time  $τ = T_c$. 
  
  
  
−
 
+
===Questions===
−
 
 
−
''Hinweise:''
 
−
*Die Aufgabe gehört zum  Kapitel  [[Modulation_Methods/Spreizfolgen_für_CDMA|Spreizfolgen für CDMA]].
 
−
*Bezug genommen wird insbesondere auf den Abschnitt  [[Modulation_Methods/Spreizfolgen_für_CDMA#Walsh.E2.80.93Funktionen |Walsh–Funktionen]]  im Theorieteil.
 
−
* Wir möchten Sie gerne auch auf das interaktive Applet  [[Applets:Zur_Erzeugung_von_Walsh-Funktionen_(neues_Applet)|Zur Erzeugung von Walsh-Funktionen]]  hinweisen.
 
−
 
−
*Die Abszisse ist auf die Chipdauer  $T_c$  normiert.  Das bedeutet, dass  $λ = 1$  eigentlich eine Verschiebung um die Verzögerungszeit  $τ = T_c$  beschreibt.
 
−
 
 
−
 
 
−
 
 
−
===Fragebogen===
 
  
 
<quiz display=simple>
 
<quiz display=simple>
−
{Wie lauten die Spreizfolgen für &nbsp;$J = 4$?
+
{What are the spreading sequences for &nbsp;$J = 4$?
 
|type="[]"}
 
|type="[]"}
 
+ $ \langle w_\nu^{(1)}\rangle = +\hspace{-0.05cm}1 -\hspace{-0.15cm}1 +\hspace{-0.15cm}1 -\hspace{-0.15cm}1$,
 
+ $ \langle w_\nu^{(1)}\rangle = +\hspace{-0.05cm}1 -\hspace{-0.15cm}1 +\hspace{-0.15cm}1 -\hspace{-0.15cm}1$,
Line 50: Line 44:
 
+ $ \langle w_\nu^{(3)}\rangle = +\hspace{-0.05cm}1 -\hspace{-0.15cm}1 -\hspace{-0.15cm}1 +\hspace{-0.15cm}1$.
 
+ $ \langle w_\nu^{(3)}\rangle = +\hspace{-0.05cm}1 -\hspace{-0.15cm}1 -\hspace{-0.15cm}1 +\hspace{-0.15cm}1$.
  
−
{Welche Aussagen gelten bezüglich der PKKF–Werte &nbsp;$φ_{ij}(λ = 0)$?
+
{Which statements are true regarding the PCCF values &nbsp;$φ_{ij}(λ = 0)$?
 
|type="[]"}
 
|type="[]"}
−
+ Für $J = 4$&nbsp; ist &nbsp;$φ_{12}(λ = 0) = 0$.
+
+ For $J = 4$,&nbsp; &nbsp;$φ_{12}(λ = 0) = 0$.
−
+ Für $J = 4$&nbsp; ist &nbsp;$φ_{13}(λ = 0) = 0$.
+
+ For $J = 4$,&nbsp; &nbsp;$φ_{13}(λ = 0) = 0$.
−
+ Für $J = 4$&nbsp; ist &nbsp;$φ_{23}(λ = 0) = 0$.
+
+ For $J = 4$,&nbsp; &nbsp;$φ_{23}(λ = 0) = 0$.
−
- Für $J = 8$&nbsp; kann durchaus &nbsp;$φ_{ij}(λ = 0) ≠ 0$&nbsp; gelten &nbsp;$(i ≠ j)$.
+
- For $J = 8$,&nbsp; &nbsp;$φ_{ij}(λ = 0) ≠ 0$&nbsp; may well hold &nbsp;$(i ≠ j)$.
−
+ Bei synchronem CDMA stören sich die Teilnehmer nicht.
+
+ In synchronous CDMA,&nbsp; the subscribers do not interfere with each other.
  
−
{Welche Aussagen gelten für die PKKF–Werte mit &nbsp;$λ ≠ 0$?
+
{Which statements are true for the PCCF values with &nbsp;$λ ≠ 0$?
 
|type="[]"}
 
|type="[]"}
−
+ Für alle Werte von &nbsp;$λ$&nbsp; ist die PKKF &nbsp;$φ_{12}(λ) = 0$.
+
+ For all values of &nbsp;$λ$,&nbsp; the PCCF is &nbsp;$φ_{12}(λ) = 0$.
−
+ Für alle Werte von &nbsp;$λ$&nbsp; ist die PKKF &nbsp;$φ_{13}(λ) = 0$.
+
+ For all values of &nbsp;$λ$,&nbsp; the PCCF is &nbsp;$φ_{13}(λ) = 0$.
−
- Für alle Werte von &nbsp;$λ$&nbsp; ist die PKKF &nbsp;$φ_{23}(λ) = 0$.
+
- For all values of &nbsp;$λ$,&nbsp; the PCCF is &nbsp;$φ_{23}(λ) = 0$.
−
- Bei asynchronem CDMA stören sich die Teilnehmer nicht.
+
- In asynchronous CDMA,&nbsp; the subscribers do not interfere with each other.
  
−
{Welche Aussagen gelten für die PAKF–Kurven?
+
{Which statements are true for the PACF curves?
 
|type="[]"}
 
|type="[]"}
−
+ Alle &nbsp;$φ_{ii}(λ)$–Kurven sind periodisch.
+
+ All&nbsp; &nbsp;$φ_{ii}(λ)$&nbsp; curves are periodic.
−
+ Es gilt &nbsp;$φ_{11}(λ = 0) = +\hspace{-0.05cm}1$&nbsp; und &nbsp;$φ_{11}(λ = 1) = -\hspace{-0.05cm}1$.
+
+ &nbsp;$φ_{11}(λ = 0) = +\hspace{-0.05cm}1$&nbsp; and &nbsp;$φ_{11}(λ = 1) = -\hspace{-0.05cm}1$&nbsp; hold.
−
- Es gilt &nbsp;$φ_{22}(λ) = φ_{11}(λ)$.
+
- &nbsp;$φ_{22}(λ) = φ_{11}(λ)$&nbsp; holds.
−
+ Es gilt &nbsp;$φ_{33}(λ) = φ_{22}(λ)$.
+
+ &nbsp;$φ_{33}(λ) = φ_{22}(λ)$&nbsp; holds.
 
</quiz>
 
</quiz>
  
−
===Musterlösung===
+
===Solution===
 
{{ML-Kopf}}
 
{{ML-Kopf}}
−
'''(1)'''&nbsp; <u>Alle Vorschläge</u> sind richtig:
+
'''(1)'''&nbsp; <u>All solutions</u>&nbsp; are correct:
−
*Die Matrix&nbsp; $ {\mathbf{H}_{4}}$&nbsp; ist die linke obere Teilmatrix von&nbsp; $ {\mathbf{H}_{8}}$.  
+
*The matrix&nbsp; $ {\mathbf{H}_{4}}$&nbsp; is the upper left submatrix of&nbsp; $ {\mathbf{H}_{8}}$.  
−
*Die Spreizfolgen ergeben sich aus den Zeilen 2, 3 und 4 von&nbsp; $ {\mathbf{H}_{4}}$, und stimmen mit den angegebenen Folgen überein.  
+
*The spreading sequences result from the rows 2,&nbsp; 3&nbsp; and 4&nbsp; of&nbsp; $ {\mathbf{H}_{4}}$,&nbsp; and agree with the given sequences.
  
  
−
 
+
'''(2)'''&nbsp; <u>Solutions 1, 2 and 3</u>&nbsp; are correct:
−
'''(2)'''&nbsp; Richtig sind die <u>Lösungsvorschläge 1, 2 und 3</u>:
+
*According to the equations in the data section,&nbsp; the following holds:
−
*Entsprechend den Gleichungen im Angabenteil gilt:
 
 
:$${\it \varphi}_{12}(\lambda = 0) = 1/4 \cdot \left [ (+1) \cdot (+1) + (-1) \cdot (+1) + (+1) \cdot (-1) + (-1) \cdot (-1) \right ] = 0\hspace{0.05cm},$$  
 
:$${\it \varphi}_{12}(\lambda = 0) = 1/4 \cdot \left [ (+1) \cdot (+1) + (-1) \cdot (+1) + (+1) \cdot (-1) + (-1) \cdot (-1) \right ] = 0\hspace{0.05cm},$$  
 
:$${\it \varphi}_{13}(\lambda = 0) = 1/4\cdot \left [ (+1) \cdot (+1) + (-1) \cdot (-1) + (+1) \cdot (-1) + (-1) \cdot (+1) \right ] = 0\hspace{0.05cm},$$  
 
:$${\it \varphi}_{13}(\lambda = 0) = 1/4\cdot \left [ (+1) \cdot (+1) + (-1) \cdot (-1) + (+1) \cdot (-1) + (-1) \cdot (+1) \right ] = 0\hspace{0.05cm},$$  
 
:$${\it \varphi}_{23}(\lambda = 0) =1/4 \cdot \left [ (+1) \cdot (+1) + (+1) \cdot (-1) + (-1) \cdot (-1) + (-1) \cdot (+1) \right ] = 0\hspace{0.05cm}.$$
 
:$${\it \varphi}_{23}(\lambda = 0) =1/4 \cdot \left [ (+1) \cdot (+1) + (+1) \cdot (-1) + (-1) \cdot (-1) + (-1) \cdot (+1) \right ] = 0\hspace{0.05cm}.$$
−
*Auch für größere Werte von&nbsp; $J$&nbsp; ist für&nbsp; $i ≠ j$&nbsp; der PKKF–Wert stets&nbsp; $φ_{ij}(λ = 0)= 0$.  
+
*Also,&nbsp; for larger values of&nbsp; $J$,&nbsp; for&nbsp; $i ≠ j$&nbsp; the PCCF value is always&nbsp; $φ_{ij}(λ = 0)= 0$.  
−
*Daraus folgt: &nbsp; Bei synchronem CDMA stören sich die Teilnehmer nicht.  
+
*It follows: &nbsp; In synchronous CDMA,&nbsp; the subscribers do not interfere with each other.
−
 
 
  
  
  
−
'''(3)'''&nbsp; Richtig sind die <u>Lösungsvorschläge 1 und 2</u>:
+
'''(3)'''&nbsp; <u>Solutions 1 and 2</u>&nbsp; are correct:
−
*Für alle Werte von&nbsp; $λ$&nbsp; ist die PKKF&nbsp; $φ_{12}(λ) = 0$, wie die folgenden Zeilen zeigen:
+
*For all values of&nbsp; $λ$,&nbsp; the PCCF is&nbsp; $φ_{12}(λ) = 0$,&nbsp; as shown by the following lines:
−
:$$\langle w_\nu^{(1)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$ $$\langle w_\nu^{(2)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}, \hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},$$  
+
:$$\langle w_\nu^{(1)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$ $$\langle w_\nu^{(2)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}, \hspace{0.3cm}{\rm product\hspace{0.1cm} with \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},$$  
−
:$$\langle w_{\nu+1}^{(2)}\rangle  =  {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}, \hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},$$  
+
:$$\langle w_{\nu+1}^{(2)}\rangle  =  {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}, \hspace{0.3cm}{\rm product\hspace{0.1cm} with \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},$$  
−
:$$\langle w_{\nu+2}^{(2)}\rangle  =  {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},\hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$  
+
:$$\langle w_{\nu+2}^{(2)}\rangle  =  {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},\hspace{0.3cm}{\rm product\hspace{0.1cm} with \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$  
−
:$$\langle w_{\nu+3}^{(2)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},\hspace{0.3cm}{\rm Produkt\hspace{0.1cm} mit \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$  
+
:$$\langle w_{\nu+3}^{(2)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},\hspace{0.3cm}{\rm product\hspace{0.1cm} with \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$  
 
:$$\langle w_{\nu+4}^{(2)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} = \langle w_\nu^{(2)}\rangle \hspace{0.05cm}.$$
 
:$$\langle w_{\nu+4}^{(2)}\rangle  =  {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} = \langle w_\nu^{(2)}\rangle \hspace{0.05cm}.$$
−
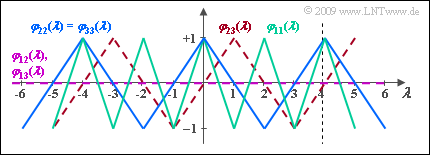
[[File:P_ID1890__Mod_A_5_4c.png|right|frame|Verschiedene PKKF&ndash; und PAKF&ndash;Kurven]]
+
[[File:P_ID1890__Mod_A_5_4c.png|right|frame|Some PCCF and PACF curves]]
−
*Das gleiche gilt für die PKKF&nbsp; $φ_{13}(λ)$.  
+
*The same is true for the PCCF&nbsp; $φ_{13}(λ)$.  
−
*Dagegen erhält man für die PKKF zwischen den Folgen&nbsp; $ \langle w_\nu^{(2)}\rangle$&nbsp; und&nbsp; $ \langle w_\nu^{(3)}\rangle$:
+
*In contrast,&nbsp; for the PCCF between the sequences&nbsp; $ \langle w_\nu^{(2)}\rangle$&nbsp; and&nbsp; $ \langle w_\nu^{(3)}\rangle$&nbsp; we obtain:
  
−
:$${\it \varphi}_{23}(\lambda ) = \left\{ \begin{array}{c}0 \\+1\\ -1 \\ \end{array} \right. \begin{array}{*{10}c} {\rm{f\ddot{u}r}} \\ {\rm{f\ddot{u}r}} \\ {\rm{f\ddot{u}r}} \\ \end{array} \begin{array}{*{20}c} \lambda = 0, \pm 2, \pm 4,\pm 6, ... \hspace{0.05cm}, \\ \hspace{0.14cm} \lambda = ... \hspace{0.05cm} , -3, +1, +5, ... \hspace{0.05cm}, \\ \hspace{0.14cm} \lambda = ... \hspace{0.05cm} , -5, -1, +3, ... \hspace{0.05cm}. \\ \end{array}$$
+
:$${\it \varphi}_{23}(\lambda ) = \left\{ \begin{array}{c}0 \\+1\\ -1 \\ \end{array} \right. \begin{array}{*{10}c} {\rm{for}} \\ {\rm{for}} \\ {\rm{for}} \\ \end{array} \begin{array}{*{20}c} \lambda = 0, \pm 2, \pm 4,\pm 6, ... \hspace{0.05cm}, \\ \hspace{0.14cm} \lambda = ... \hspace{0.05cm} , -3, +1, +5, ... \hspace{0.05cm}, \\ \hspace{0.14cm} \lambda = ... \hspace{0.05cm} , -5, -1, +3, ... \hspace{0.05cm}. \\ \end{array}$$
−
*Das bedeutet: &nbsp; Wird das Signal von Teilnehmer $3$ gegenüber Teilnehmer $2$ um ein Spreizchip verzögert oder umgekehrt, so lassen sich die Teilnehmer nicht mehr trennen und es kommt zu einer signifikanten Erhöhung der Fehlerwahrscheinlichkeit.  
+
*This means: &nbsp; If the signal from subscriber&nbsp; $3$&nbsp; is delayed by one spreading chip with respect to subscriber&nbsp; $2$&nbsp; or vice versa,&nbsp; the subscribers can no longer be separated and there is a significant increase in the error probability.
−
*In der Grafik sind die PKKF–Kurven gestrichelt eingezeichnet&nbsp; (violett und rot).
+
*In the diagram,&nbsp; the PCCF curves are drawn in dashed lines&nbsp; (violet and red).
  
  
  
−
'''(4)'''&nbsp; Richtig sind die <u>Aussagen 1, 2 und 4</u>:
+
'''(4)'''&nbsp; <u>Statements 1,&nbsp; 2&nbsp; and 4</u>&nbsp; are correct:
−
* Da die Walsh–Funktion Nr.&nbsp; $1$&nbsp; periodisch ist mit&nbsp; $T_0 = 2T_c$, ist auch die PAKF periodisch mit&nbsp; $λ = 2$.
+
* Since the Walsh function no.&nbsp; $1$&nbsp; is periodic with&nbsp; $T_0 = 2T_c$,&nbsp; the PACF is also periodic with&nbsp; $λ = 2$.
−
*Die zweite Aussage ist richtig, wie die folgende Rechnung zeigt&nbsp; (grüner Kurvenzug):
+
*The second statement is correct,&nbsp; as shown by the following calculation&nbsp; (green curve):
 
:$${\it \varphi}_{11}(\lambda = 0)  =  1/4 \cdot \big [ (+1) \cdot (+1) + (-1) \cdot (-1) + (+1) \cdot (+1) + (-1) \cdot (-1) \big ] = +1\hspace{0.05cm},$$  
 
:$${\it \varphi}_{11}(\lambda = 0)  =  1/4 \cdot \big [ (+1) \cdot (+1) + (-1) \cdot (-1) + (+1) \cdot (+1) + (-1) \cdot (-1) \big ] = +1\hspace{0.05cm},$$  
 
:$${\it \varphi}_{11}(\lambda = 1)  =  1/4 \cdot \big [ (+1) \cdot (-1) + (+1) \cdot (-1) + (+1) \cdot (-1) + (+1) \cdot (-1) \big ] = -1\hspace{0.05cm}.$$
 
:$${\it \varphi}_{11}(\lambda = 1)  =  1/4 \cdot \big [ (+1) \cdot (-1) + (+1) \cdot (-1) + (+1) \cdot (-1) + (+1) \cdot (-1) \big ] = -1\hspace{0.05cm}.$$
−
*Da sich die beiden Walsh–Funktionen Nr.&nbsp; $2$&nbsp; und&nbsp; $3$&nbsp; nur durch eine Verschiebung um&nbsp; $T_c$&nbsp; unterscheiden und sich eine Phase in der PAKF prinzipiell nicht auswirkt, ist tatsächlich entsprechend dem letzten Lösungsvorschlag&nbsp; $φ_{33}(λ) = φ_{22}(λ)$.&nbsp; Diese beiden PAKF–Funktionen sind blau eingezeichnet.
+
*Since the two Walsh functions no.&nbsp; $2$&nbsp; and&nbsp; $3$&nbsp; differ only by a shift around&nbsp; $T_c$&nbsp; and a phase in the PACF has no effect in principle,&nbsp; in fact,&nbsp; according to the last statement,&nbsp; $φ_{33}(λ) = φ_{22}(λ)$.&nbsp; These two PACF functions are plotted in blue.
−
*Dagegen unterscheidet sich&nbsp; $φ_{22}(λ)$&nbsp; von&nbsp; $φ_{11}(λ)$&nbsp; durch eine andere Periodizität: &nbsp; $φ_{22}(λ) = φ_{33}(λ)$&nbsp; ist doppelt so breit wie&nbsp; $φ_{11}(λ)$.
+
*In contrast,&nbsp; $φ_{22}(λ)$&nbsp; differs from&nbsp; $φ_{11}(λ)$&nbsp; by a different periodicity: &nbsp; $φ_{22}(λ) = φ_{33}(λ)$&nbsp; is twice as wide as&nbsp; $φ_{11}(λ)$.
  
 
{{ML-Fuß}}
 
{{ML-Fuß}}
Line 121: Line 113:
  
  
−
[[Category:Modulation Methods: Exercises|^5.3 Spreizfolgen für CDMA^]]
+
[[Category:Modulation Methods: Exercises|^5.3 Spread Sequences for CDMA^]]

Latest revision as of 16:31, 13 December 2021

Hadamard matrix  ${\mathbf{H}_{8}}$

The so-called  "Walsh functions",  which can be constructed by means of the Hadamard matrix,  are often used for band spreading and band compression.  Starting from the matrix

$${\mathbf{H}_{2}} = \left[ \begin{array}{ccc} +1 & +1 \\ +1 & -1 \end{array} \right] $$

the further Hadamard matrices  $ {\mathbf{H}_{4}}$,  $ {\mathbf{H}_{8}}$,  etc. can be derived by the following recursion:

$$ {\mathbf{H}_{2J}} = \left[ \begin{array}{ccc} \mathbf{H}_J & \mathbf{H}_J \\ \mathbf{H}_J & -\mathbf{H}_J \end{array} \right] \hspace{0.05cm}.$$

The diagram shows the matrix  $ {\mathbf{H}_{8}}$  for the spreading factor  $J = 8$.  From this we can derive the spreading sequences

$$ \langle w_\nu^{(1)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
$$ \langle w_\nu^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
$$...$$
$$\langle w_\nu^{(7)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm}{+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}$$

for seven CDMA subscribers.  The spreading sequence  $ \langle w_\nu^{(0)}\rangle$  corresponding to the first row in the Hadamard matrix is usually not assigned because it does not spread.

The questions mostly refer to the spreading factor  $J = 4$.  Thus,  correspondingly,  a maximum of three CDMA subscribers can be supplied with the spreading sequences  $ \langle w_\nu^{(1)}\rangle$,  $ \langle w_\nu^{(2)}\rangle$  and  $ \langle w_\nu^{(3)}\rangle$,  which result from the second, third and fourth rows of the matrix $ {\mathbf{H}_{4}}$.

Regarding the correlation functions, the following nomenclature shall apply in this exercise:

  • The  periodic cross-correlation function  $\rm (PCCF)$  between the sequences  $ \langle w_\nu^{(i)}\rangle$  and  $ \langle w_\nu^{(j)}\rangle$  is denoted by  $φ_{ij}(λ)$.   Here:
$${\it \varphi}_{ij}(\lambda) = {\rm E}\left [ w_{\nu}^{(i)} \cdot w_{\nu+ \lambda}^{(j)} \right ] \hspace{0.05cm}.$$
  • If  $φ_{ij} \equiv 0$  $($that is:  $φ_{ij}(λ) = 0$  for all values of  $λ)$,  the CDMA subscribers do not interfere with each other,  even if they have different propagation times.
  • If at least  $φ_{ij}({\it λ} = 0) = 0$  applies,  then no interference occurs,  at least in synchronous CDMA operation  $($no or equal propagation times of all subscribers$).$ 
  • The  periodic auto-correlation function  $\rm (PACF)$  of the Walsh function  $ \langle w_\nu^{(i)}\rangle$  is denoted by  $φ_{ii}(λ)$,  and it holds:
$${\it \varphi}_{ii}(\lambda) = {\rm E}\left [ w_{\nu}^{(i)} \cdot w_{\nu+ \lambda}^{(i)} \right ] \hspace{0.05cm}.$$


Notes:

  • The exercise belongs to the chapter  Spreading Sequences for CDMA.
  • Reference is made in particular to the section  Walsh functions  in the theory part.
  • We would also like to draw your attention to the interactive applet  Generation of Walsh functions. 
  • The abscissa is normalized to the chip duration  $T_c$.  This means that  $λ = 1$  actually describes a shift by the delay time  $τ = T_c$. 


Questions

1

What are the spreading sequences for  $J = 4$?

$ \langle w_\nu^{(1)}\rangle = +\hspace{-0.05cm}1 -\hspace{-0.15cm}1 +\hspace{-0.15cm}1 -\hspace{-0.15cm}1$,
$ \langle w_\nu^{(2)}\rangle = +\hspace{-0.05cm}1 +\hspace{-0.15cm}1 -\hspace{-0.15cm}1 -\hspace{-0.15cm}1$,
$ \langle w_\nu^{(3)}\rangle = +\hspace{-0.05cm}1 -\hspace{-0.15cm}1 -\hspace{-0.15cm}1 +\hspace{-0.15cm}1$.

2

Which statements are true regarding the PCCF values  $φ_{ij}(λ = 0)$?

For $J = 4$,   $φ_{12}(λ = 0) = 0$.
For $J = 4$,   $φ_{13}(λ = 0) = 0$.
For $J = 4$,   $φ_{23}(λ = 0) = 0$.
For $J = 8$,   $φ_{ij}(λ = 0) ≠ 0$  may well hold  $(i ≠ j)$.
In synchronous CDMA,  the subscribers do not interfere with each other.

3

Which statements are true for the PCCF values with  $λ ≠ 0$?

For all values of  $λ$,  the PCCF is  $φ_{12}(λ) = 0$.
For all values of  $λ$,  the PCCF is  $φ_{13}(λ) = 0$.
For all values of  $λ$,  the PCCF is  $φ_{23}(λ) = 0$.
In asynchronous CDMA,  the subscribers do not interfere with each other.

4

Which statements are true for the PACF curves?

All   $φ_{ii}(λ)$  curves are periodic.
 $φ_{11}(λ = 0) = +\hspace{-0.05cm}1$  and  $φ_{11}(λ = 1) = -\hspace{-0.05cm}1$  hold.
 $φ_{22}(λ) = φ_{11}(λ)$  holds.
 $φ_{33}(λ) = φ_{22}(λ)$  holds.


Solution

Solution

(1)  All solutions  are correct:

  • The matrix  $ {\mathbf{H}_{4}}$  is the upper left submatrix of  $ {\mathbf{H}_{8}}$.
  • The spreading sequences result from the rows 2,  3  and 4  of  $ {\mathbf{H}_{4}}$,  and agree with the given sequences.


(2)  Solutions 1, 2 and 3  are correct:

  • According to the equations in the data section,  the following holds:
$${\it \varphi}_{12}(\lambda = 0) = 1/4 \cdot \left [ (+1) \cdot (+1) + (-1) \cdot (+1) + (+1) \cdot (-1) + (-1) \cdot (-1) \right ] = 0\hspace{0.05cm},$$
$${\it \varphi}_{13}(\lambda = 0) = 1/4\cdot \left [ (+1) \cdot (+1) + (-1) \cdot (-1) + (+1) \cdot (-1) + (-1) \cdot (+1) \right ] = 0\hspace{0.05cm},$$
$${\it \varphi}_{23}(\lambda = 0) =1/4 \cdot \left [ (+1) \cdot (+1) + (+1) \cdot (-1) + (-1) \cdot (-1) + (-1) \cdot (+1) \right ] = 0\hspace{0.05cm}.$$
  • Also,  for larger values of  $J$,  for  $i ≠ j$  the PCCF value is always  $φ_{ij}(λ = 0)= 0$.
  • It follows:   In synchronous CDMA,  the subscribers do not interfere with each other.


(3)  Solutions 1 and 2  are correct:

  • For all values of  $λ$,  the PCCF is  $φ_{12}(λ) = 0$,  as shown by the following lines:
$$\langle w_\nu^{(1)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$ $$\langle w_\nu^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}, \hspace{0.3cm}{\rm product\hspace{0.1cm} with \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},$$
$$\langle w_{\nu+1}^{(2)}\rangle = {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm}, \hspace{0.3cm}{\rm product\hspace{0.1cm} with \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},$$
$$\langle w_{\nu+2}^{(2)}\rangle = {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},\hspace{0.3cm}{\rm product\hspace{0.1cm} with \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
$$\langle w_{\nu+3}^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.05cm},\hspace{0.3cm}{\rm product\hspace{0.1cm} with \hspace{0.1cm}}\langle w_\nu^{(1)}\rangle: {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} \hspace{0.05cm},$$
$$\langle w_{\nu+4}^{(2)}\rangle = {+\hspace{-0.05cm}1}\hspace{0.15cm} {+\hspace{-0.05cm}1} \hspace{0.15cm} {-\hspace{-0.05cm}1}\hspace{0.15cm} {-\hspace{-0.05cm}1} = \langle w_\nu^{(2)}\rangle \hspace{0.05cm}.$$
Some PCCF and PACF curves
  • The same is true for the PCCF  $φ_{13}(λ)$.
  • In contrast,  for the PCCF between the sequences  $ \langle w_\nu^{(2)}\rangle$  and  $ \langle w_\nu^{(3)}\rangle$  we obtain:
$${\it \varphi}_{23}(\lambda ) = \left\{ \begin{array}{c}0 \\+1\\ -1 \\ \end{array} \right. \begin{array}{*{10}c} {\rm{for}} \\ {\rm{for}} \\ {\rm{for}} \\ \end{array} \begin{array}{*{20}c} \lambda = 0, \pm 2, \pm 4,\pm 6, ... \hspace{0.05cm}, \\ \hspace{0.14cm} \lambda = ... \hspace{0.05cm} , -3, +1, +5, ... \hspace{0.05cm}, \\ \hspace{0.14cm} \lambda = ... \hspace{0.05cm} , -5, -1, +3, ... \hspace{0.05cm}. \\ \end{array}$$
  • This means:   If the signal from subscriber  $3$  is delayed by one spreading chip with respect to subscriber  $2$  or vice versa,  the subscribers can no longer be separated and there is a significant increase in the error probability.
  • In the diagram,  the PCCF curves are drawn in dashed lines  (violet and red).


(4)  Statements 1,  2  and 4  are correct:

  • Since the Walsh function no.  $1$  is periodic with  $T_0 = 2T_c$,  the PACF is also periodic with  $λ = 2$.
  • The second statement is correct,  as shown by the following calculation  (green curve):
$${\it \varphi}_{11}(\lambda = 0) = 1/4 \cdot \big [ (+1) \cdot (+1) + (-1) \cdot (-1) + (+1) \cdot (+1) + (-1) \cdot (-1) \big ] = +1\hspace{0.05cm},$$
$${\it \varphi}_{11}(\lambda = 1) = 1/4 \cdot \big [ (+1) \cdot (-1) + (+1) \cdot (-1) + (+1) \cdot (-1) + (+1) \cdot (-1) \big ] = -1\hspace{0.05cm}.$$
  • Since the two Walsh functions no.  $2$  and  $3$  differ only by a shift around  $T_c$  and a phase in the PACF has no effect in principle,  in fact,  according to the last statement,  $φ_{33}(λ) = φ_{22}(λ)$.  These two PACF functions are plotted in blue.
  • In contrast,  $φ_{22}(λ)$  differs from  $φ_{11}(λ)$  by a different periodicity:   $φ_{22}(λ) = φ_{33}(λ)$  is twice as wide as  $φ_{11}(λ)$.

Retrieved from "http://en.lntwww.de/index.php?title=Aufgaben:Exercise_5.4:_Walsh_Functions_(PCCF,_PACF)&oldid=43816"
  • Powered by MediaWiki