Difference between revisions of "Aufgaben:Exercise 3.7Z: Spread Spectrum in UMTS"
(Die Seite wurde neu angelegt: „ {{quiz-Header|Buchseite=Mobile Kommunikation/Die Charakteristika von UMTS }} right|frame|Quellensignal und Spreizsignal Bei…“) |
|||
| (14 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{quiz-Header|Buchseite= | + | {{quiz-Header|Buchseite=Mobile_Communications/Characteristics_of_UMTS |
}} | }} | ||
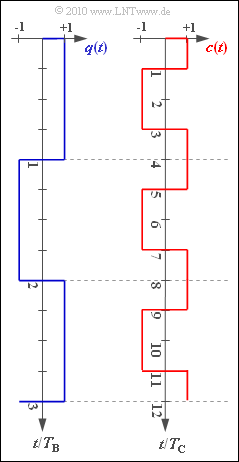
| − | [[File:P_ID2260__Bei_Z_4_5.png|right|frame| | + | [[File:P_ID2260__Bei_Z_4_5.png|right|frame|Source signal and spread signal]] |
| − | + | For UMTS/CDMA, the so-called "PN modulation" is applied: | |
| − | * | + | *The rectangular digital signal $q(t)$ is multiplied by the spread signal $c(t)$ and gives the transmission signal $s(t)$. |
| − | * | + | *This is by the spreading factor $J$ of higher frequency than $q(t)$; this is called "band spreading". |
| − | + | At the receiver the same spreading signal $c(t)$ is added (namely in phase!). This reverses the band spreading ⇒ "band compression". | |
| − | + | The graphic shows exemplary signal characteristics of $q(t)$ und $c(t)$. | |
| Line 17: | Line 17: | ||
| − | '' | + | ''Notes:'' |
| − | * | + | *This task refers to the chapter [[Mobile_Communications/Characteristics_of_UMTS|Characteristics of UMTS]]. |
| − | * | + | *Reference is made to the chapter [[Examples_of_Communication_Systems/Telecommunications_Aspects_of_UMTS|Telecommunications Aspects of UMTS]] in the book "Examples of Communication_Systems". |
| − | * | + | *For the calculation of the chip duration $T_{\rm C}$, please refer to section [[Examples_of_Communication_Systems/UMTS_Network_Architecture#Physical_channels|Physical Channels]] . |
| − | * | + | *There you will find, among other things, the information important for this task, which is transmitted on the so-called "Dedicated Physical Channel" $\rm (DPCH)$ in ten milliseconds exactly $15 \cdot 2560 \ \rm Chips$. |
| − | *In | + | *In subtask '''(5)''', the system asks for "transmitting chips". <br>For example, the "transmitting chip" $s_{3}$ denotes the constant signal value of $s(t)$ in the time interval $2T_{\rm C}$ ... $3T_{\rm C}$. |
| Line 29: | Line 29: | ||
| − | === | + | ===Questionnaire=== |
<quiz display=simple> | <quiz display=simple> | ||
| − | { | + | {Which of the following statements are true? |
|type="[]"} | |type="[]"} | ||
| − | - | + | - For UMTS the bit duration $T_{\rm B}$ is fixed. |
| − | + | + | + For UMTS, the chip duration $T_{\rm C}$ is fixed. |
| − | - | + | - Both values depend on the channel conditions. |
| − | { | + | {Specify the chip duration $T_{\rm C}$ and the chip rate $R_{\rm C}$ in the downlink. |
|type="{}"} | |type="{}"} | ||
$R_{\rm C} \ = \ $ { 3.84 3% } $\ \rm Mchip/s $ | $R_{\rm C} \ = \ $ { 3.84 3% } $\ \rm Mchip/s $ | ||
$T_{\rm C} \hspace{0.18cm} = \ $ { 0.26 3% } $ \ \rm µ s $ | $T_{\rm C} \hspace{0.18cm} = \ $ { 0.26 3% } $ \ \rm µ s $ | ||
| − | { | + | {Which spreading factor can be read from the graph in the data section? |
|type="{}"} | |type="{}"} | ||
$J \ = \ $ { 4 } | $J \ = \ $ { 4 } | ||
| − | { | + | {What is the bit rate with this spreading factor? |
|type="{}"} | |type="{}"} | ||
$R_{\rm B} \ = \ $ { 960 3% } $\ \rm kbit/s $ | $R_{\rm B} \ = \ $ { 960 3% } $\ \rm kbit/s $ | ||
| − | { | + | {What are the "transmitting chips" ⇒ of the signal $s(t)$? |
|type="{}"} | |type="{}"} | ||
$s_{3} \ = \ $ { -1.03--0.97 } | $s_{3} \ = \ $ { -1.03--0.97 } | ||
| Line 64: | Line 64: | ||
{{ML-Kopf}} | {{ML-Kopf}} | ||
| − | '''(1)''' | + | '''(1)''' Correct is the <u>solution 2</u>: |
| − | * | + | *For UMTS, the chip duration $T_{\rm C}$, which is still to be calculated in the subtask '''(2)''', is predefined. |
| − | * | + | *The greater the degree of spreading $J$, the greater the bit duration. |
| − | '''(2)''' | + | '''(2)''' According to the note in the information section, in $10 \ \rm ms$ exactly $15 \cdot 2560 = 38400 \ \rm chips$ are transferred. |
| − | * | + | *With this the chip rate: $R_{\rm C} = 100 \cdot 38400 \ {\rm Chips/s} \ \underline{= 3.84 \ \rm Mchip/s}$. |
| − | * | + | *The chip duration is the reciprocal of this: $T_{\rm C} \ \underline{\approx 0.26 \ \rm µ s}$. |
| − | '''(3)''' | + | '''(3)''' Each data bit consists of four spreading chips ⇒ $\underline{J = 4}$. |
| − | '''(4)''' | + | '''(4)''' The bit rate is calculated with the spreading factor $J = 4$ to $R_{\rm B} = R_{\rm C}/J \ \underline{= 960 \ \rm kbit/s}$. |
| − | * | + | * With the maximum spreading factor $J = 512$ for UMTS, the bit rate is only $7.5 \ \rm kbit/s$. |
| − | '''(5)''' | + | '''(5)''' The following applies to the transmission signal $s(t) = q(t) \cdot c(t)$. |
| − | * | + | *The chips $s_{3}$ and $s_{4}$ of the transmission signal belong to the first data bit $(q_{1} = +1)$: |
:$$s_3 = c_3 \hspace{0.15cm}\underline {= -1},\hspace{0.4cm}s_4 = c_4 \hspace{0.15cm}\underline {= +1}\hspace{0.05cm}.$$ | :$$s_3 = c_3 \hspace{0.15cm}\underline {= -1},\hspace{0.4cm}s_4 = c_4 \hspace{0.15cm}\underline {= +1}\hspace{0.05cm}.$$ | ||
| − | * | + | *On the other hand, the two other transmission chips sought are to be assigned to the second data bit $(q_{2} = -1)$: |
| − | :$$s_5 = -c_5= -c_1 \hspace{0.15cm}\underline {= -1},\hspace{0.4cm}s_6 = -c_6= -c_2 \hspace{0.15cm}\underline {= +1}\hspace{0.05cm}$$ | + | :$$s_5 = -c_5= -c_1 \hspace{0.15cm}\underline {= -1},\hspace{0.4cm}s_6 = -c_6= -c_2 \hspace{0.15cm}\underline {= +1}\hspace{0.05cm}.$$ |
{{ML-Fuß}} | {{ML-Fuß}} | ||
| Line 95: | Line 95: | ||
| − | [[Category: | + | [[Category:Mobile Communications: Exercises|^3.4 Characteristics of UMTS^]] |
Latest revision as of 01:42, 13 November 2022
For UMTS/CDMA, the so-called "PN modulation" is applied:
- The rectangular digital signal $q(t)$ is multiplied by the spread signal $c(t)$ and gives the transmission signal $s(t)$.
- This is by the spreading factor $J$ of higher frequency than $q(t)$; this is called "band spreading".
At the receiver the same spreading signal $c(t)$ is added (namely in phase!). This reverses the band spreading ⇒ "band compression".
The graphic shows exemplary signal characteristics of $q(t)$ und $c(t)$.
Notes:
- This task refers to the chapter Characteristics of UMTS.
- Reference is made to the chapter Telecommunications Aspects of UMTS in the book "Examples of Communication_Systems".
- For the calculation of the chip duration $T_{\rm C}$, please refer to section Physical Channels .
- There you will find, among other things, the information important for this task, which is transmitted on the so-called "Dedicated Physical Channel" $\rm (DPCH)$ in ten milliseconds exactly $15 \cdot 2560 \ \rm Chips$.
- In subtask (5), the system asks for "transmitting chips".
For example, the "transmitting chip" $s_{3}$ denotes the constant signal value of $s(t)$ in the time interval $2T_{\rm C}$ ... $3T_{\rm C}$.
Questionnaire
Musterlösung
(1) Correct is the solution 2:
- For UMTS, the chip duration $T_{\rm C}$, which is still to be calculated in the subtask (2), is predefined.
- The greater the degree of spreading $J$, the greater the bit duration.
(2) According to the note in the information section, in $10 \ \rm ms$ exactly $15 \cdot 2560 = 38400 \ \rm chips$ are transferred.
- With this the chip rate: $R_{\rm C} = 100 \cdot 38400 \ {\rm Chips/s} \ \underline{= 3.84 \ \rm Mchip/s}$.
- The chip duration is the reciprocal of this: $T_{\rm C} \ \underline{\approx 0.26 \ \rm µ s}$.
(3) Each data bit consists of four spreading chips ⇒ $\underline{J = 4}$.
(4) The bit rate is calculated with the spreading factor $J = 4$ to $R_{\rm B} = R_{\rm C}/J \ \underline{= 960 \ \rm kbit/s}$.
- With the maximum spreading factor $J = 512$ for UMTS, the bit rate is only $7.5 \ \rm kbit/s$.
(5) The following applies to the transmission signal $s(t) = q(t) \cdot c(t)$.
- The chips $s_{3}$ and $s_{4}$ of the transmission signal belong to the first data bit $(q_{1} = +1)$:
- $$s_3 = c_3 \hspace{0.15cm}\underline {= -1},\hspace{0.4cm}s_4 = c_4 \hspace{0.15cm}\underline {= +1}\hspace{0.05cm}.$$
- On the other hand, the two other transmission chips sought are to be assigned to the second data bit $(q_{2} = -1)$:
- $$s_5 = -c_5= -c_1 \hspace{0.15cm}\underline {= -1},\hspace{0.4cm}s_6 = -c_6= -c_2 \hspace{0.15cm}\underline {= +1}\hspace{0.05cm}.$$