Contents
Vestigial sideband amplitude modulation
When transmitting signals using single sideband modulation $\rm (SSB–AM)$ the following problems occur:
- To suppress the unwanted sideband, a filter with a very high edge slope must be used.
- Such a steep-edged filters exhibit strong group delay distortions, especially at the limit of the passband.
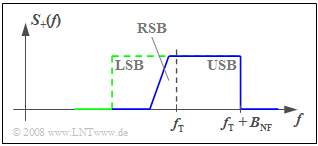
the problem can be greatly mitigated if instead single-sideband AM one uses vestigial sideband amplitude modulation $\rm (VSB–AM)$ , as shown in the adjacent graph.
The present description is based on the textbook [Mäu88][1]. According to it, the VSB-AM can be characterized as follows::
- A certain frequency range of the actually suppressed sideband – in the considered example of the LSB – is additionally used with a relatively flat decreasing transfer function.
- On the receiver side, a selection curve linearly increasing in frequency with a so-called "Nyquist edge" is used in the transition range from the suppressed sideband to the transmitted sideband.
- The demodulation performs a convolution of the sidebands around the carrier, so that as a result the message content of a band with the same amplitude for all frequencies is obtained.
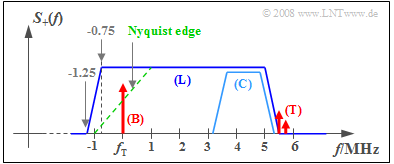
$\text{Example 1:}$ The vestigial sideband method is used for (analog) color television, whose frequency spectrum according to the CCIR standard is shown in the graphic. The frequencies given refer to the PAL–B/G Television format used in Germany.
In this schematic representation, one recognises:
- The radiated spectrum(only positive frequencies are drawn) ranges from $f_{\rm T} - 1.25 \ \rm MHz$ to $f_{\rm T} + 5.75 \ \rm MHz$. Thus, the lower vestigial sideband including the Nyquist edge is approximately $1.25 \ \rm MHz$ wide .
- The green dashed line shows the receiver passband. The image carrier (B) at carrier frequency $f_{\rm T}$ is centered on the Nyquist edge.
- The luminance signal (L) goes up to about $5 \ \rm MHz$. . It contains the information for the image brightness and the color "green".
- The chrominance signal (C) is embedded in the upper part. Two orthogonal carriers are QAM-modulated at $4.43 \ \rm MHz$ for "red" and "blue"; the carrier is suppressed.
- The audio carrier (T) (abbreviation T from German "Ton" i.e., sound/audio) is at $f_{\rm T} + 5.5 \ \rm MHz$ and is $12 \ \rm dB$ lower than the image carrier. If there is a stereo or two-channel sound transmission, a second sound carrier follows at $5.75 \ \rm MHz$ .
Quadrature Amplitude Modulation (QAM)
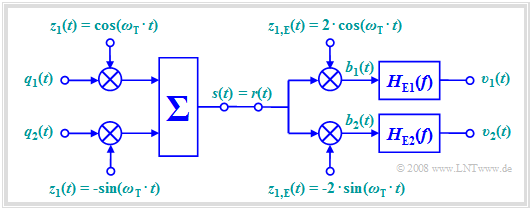
By exploiting the orthogonality of cosine and sine functions, a channel can be used twice for simultaneous transmission of two source signals $q_1(t)$ and $q_2(t)$ without mutual interference. This method is called quadrature amplitude modulation $\rm (QAM)$.
The QAM system has the following characteristics:
- The transmit signal is composed of two mutually orthogonal components:
- $$s(t) = q_1(t) \cdot \cos (\omega_{\rm T}\cdot t) - q_2(t) \cdot \sin (\omega_{\rm T}\cdot t)\hspace{0.05cm}.$$
- For frequency and phase synchronous demodulation, the signal in the upper branch before the low pass is $H_{\rm E1}(f)$:
- $$b_1(t) = q_1(t) \cdot 2 \cdot \cos^2 (\omega_{\rm T}\cdot t) - q_2(t) \cdot 2 \cdot \cos (\omega_{\rm T}\cdot t)\cdot \sin (\omega_{\rm T}\cdot t)= q_1(t)\cdot \big[ 1 + \cos (2 \omega_{\rm T}\cdot t) \big] - q_2(t)\cdot \sin (2 \omega_{\rm T}\cdot t) \hspace{0.05cm}.$$
- Thus, by limiting to frequencies $|f| < f_{\rm T}$ , we obtain in the upper and lower branches, respectively:
- $$v_1(t) = q_1(t),\hspace{0.3cm} v_2(t) = q_2(t)\hspace{0.05cm}.$$
- When there is a phase offset $Δϕ_{\rm T}$ between the transmitted and received carrier signals, in addition to attenuation of the intended participant, crosstalk from the second participant occurs, resulting in nonlinear distortion:
- $$v_1(t) = \alpha_{11} \cdot q_1(t)+ \alpha_{12} \cdot q_2(t) \hspace{0.05cm}, \hspace{0.3cm} v_2(t) = \alpha_{21} \cdot q_1(t)+ \alpha_{22} \cdot q_2(t)$$
- $$\Rightarrow\hspace{0.3cm}\alpha_{11} = \alpha_{22} = \cos(\Delta \phi_{\rm T}) \hspace{0.05cm}, \hspace{0.3cm} \alpha_{12} = -\alpha_{21} = \sin(\Delta \phi_{\rm T}) \hspace{0.05cm}.$$
Incoherent (nichtkohärente) Demodulation
Demodulatoren können in folgender Weise klassifiziert werden:
$\text{Definition:}$ Man bezeichnet einen Demodulator als kohärent, wenn er zur Rekonstruktion des Nachrichtensignals neben der erforderlichen Frequenzsynchronität auch genaue Informationen über die Phase des sendeseitigen Trägersignals $z(t)$ benötigt.
Ist diese Phaseninformation nicht erforderlich, so spricht man von einem inkohärenten Demodulator.
Ein Beispiel für einen inkohärenten (oder nichtkohärenten) Demodulator ist der Hüllkurvendemodulator.
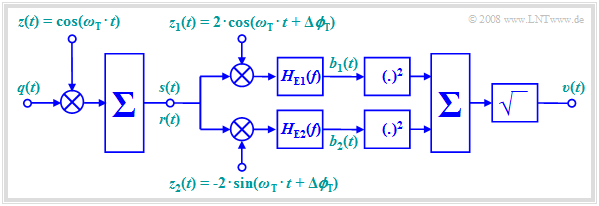
$\text{Beispiel 2:}$ Ein zweites Beispiel zeigt das folgende Blockschaltbild. Im Gegensatz zur Quadratur–Amplitudenmodulation wird hier die Orthogonalität zwischen Cosinus– und Sinusfunktion nicht zur gleichzeitigen Übertragung eines zweiten Quellensignals herangezogen, sondern zur Vereinfachung der Empfangseinrichtung genutzt.
Zu dieser Anordnung ist weiter anzumerken:
- Die empfängerseitigen Trägersignale können gegenüber den Trägersignalen beim Sender einen beliebigen und auch zeitabhängigen Phasenversatz $Δϕ_{\rm T}$ aufweisen, so lange die Phasendifferenz zwischen den beiden Zweigen weiterhin genau $90^\circ$ beträgt.
- Für die Signale im oberen und unteren Zweig – jeweils nach dem Multiplizierer und der Tiefpassfilterung – gilt nämlich:
- $$b_1(t) = \cos(\Delta \phi_{\rm T}) \cdot q(t), $$
- $$b_2(t) = -\sin(\Delta \phi_{\rm T}) \cdot q(t).$$
- Damit ist gewährleistet, dass das Sinkensignal $v(t)$ unabhängig vom Phasenversatz $Δϕ_{\rm T}$ mit dem Quellensignal $q(t)$ zumindest betragsmäßig übereinstimmt:
- $$v(t) = \sqrt{ b_1^2(t) + b_2^2(t)} = \sqrt{ q^2(t) } = \vert q(t) \vert \hspace{0.05cm}.$$
- Voraussetzung für die Funktionsfähigkeit – also für das Ergebnis $v(t) = q(t)$ – ist, dass zu allen Zeiten $q(t) ≥ 0$ ist. Bei einem analogen Nachrichtensystem könnte man diesen Sachverhalt beispielsweise mit dem Modulationsverfahren „ZSB–AM mit Träger” erzwingen.
- Angewandt wird diese Form von nichtkohärenter Demodulation – oder Modifikationen hiervon – vorwiegend bei einigen Digitalen Modulationsverfahren, die im vierten Kapitel dieses Buches ausführlich behandelt werden.
Aufgaben zum Kapitel
Aufgabe 2.12: Zur nichtkohärenten Demodulation
Aufgabe 2.13: Quadratur-Amplitudenmodulation (QAM)
Quellenverzeichnis
- ↑ Mäusl, R.: Analoge Modulationsverfahren. Heidelberg: Dr. Hüthig, 1988.