Exercise 4.12: Calculations for the 16-QAM

Signal space constellation of 16–QAM

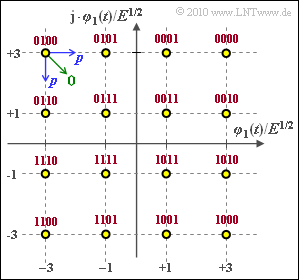
The graphic shows the signal space constellation of the "quadrature amplitude modulation" with $M = 16$ signal space points.

The following should be calculated for this modulation method:

the average energy per symbol or per bit,
the mean symbol error probability $p_{\rm S}$,
the "Union Bound" $p_{\rm UB}$ as upper bound,
the average bit error probability $p_{\rm B}$ with Gray coding.

Notes:

The exercise deals with a partial aspect of the chapter "Carrier Frequency Systems with Coherent Demodulation.
The Gray assignment is given in the graphic (red lettering).
The probability that the upper left symbol is falsified into one of the neighboring symbols is abbreviated to $p$ (blue arrows in the graph).
A diagonal falsification ⇒ two bit falsified (green arrow) is excluded.
For the AWGN channel, with the complementary Gaussian error integral for this auxiliary variable, the following applies: $p = {\rm Q} \left ( \sqrt{ { 2E}/{ N_0} }\right )\hspace{0.05cm}.$
For numerical calculations, use $E = 1 \ \rm mWs$ and $p = 0.4\%$.
The AWGN noise power density $N_0$ can be calculated approximately from these values:

$$p = {\rm Q} \left ( \sqrt{ { 2E}/{ N_0} }\right ) = 0.004 \hspace{0.1cm}\Rightarrow\hspace{0.1cm} { 2E}{ N_0} \approx 2.65^2 \approx 7 \hspace{0.1cm}\Rightarrow\hspace{0.1cm} N_0 = { E}/{ 3.5}\approx 1.4 \cdot 10^{-4}\,{\rm W/Hz} \hspace{0.05cm}.$$

Questions

Solution

(1) Der Quotient $E_{\rm S}/E$ ergibt sich als der mittlere quadratische Abstand der $M = 16$ Signalraumpunkte $\boldsymbol{s}_i$ vom Ursprung.

Mit der gegebenen Signalraumkonstellation der 16–QAM erhält man:

$$E_{\rm S} \hspace{-0.1cm} \ = \ \hspace{-0.1cm} { E}/{ 16} \cdot \left [ 4 \cdot (1^2 + 1^2) + 8 \cdot (1^2 + 3^2) + 4 \cdot (3^2 + 3^2)\right ]={ E}/{ 16} \cdot \left [ 4 \cdot 2 + 8 \cdot 10 + 4 \cdot 18\right ] = 10 \cdot E = \underline{10 \ {\rm mWs}} \hspace{0.05cm}.$$

Zum gleichen Ergebnis kommt man mit der im Theorieteil angegebenen Gleichung

$$E_{\rm S} = \frac{ 2 \cdot (M-1)}{ 3 } \cdot E = \frac{ 2 \cdot 15}{ 3 } \cdot E = 10 E \hspace{0.05cm}.$$

(2) Jedes einzelne Symbol stellt vier Binärsymbole dar. Damit ist die mittlere Energie pro Bit.

$$E_{\rm B} = \frac{ E_{\rm S}}{ {\rm log_2} \hspace{0.05cm}(M)} = 2.5 \cdot E = \underline{2.5 \ {\rm mWs}} \hspace{0.05cm}.$$

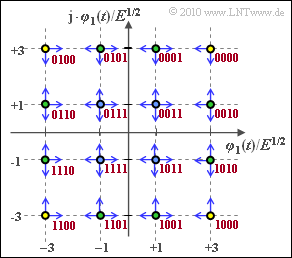
Zur Verdeutlichung der 16–QAM–Fehlerwahrscheinlichkeit

(3) Die Union Bound ist eine obere Schranke für die Symbolfehlerwahrscheinlichkeit.

Sie berücksichtigt nur den Übergang zu benachbarten Entscheidungsregionen aufgrund von AWGN–Rauschen.
Aus der Grafik geht hervor, dass die Ecksymbole (gelb gefüllt) nur zu zwei anderen Symbolen hin verfälscht werden können und die restlichen Randsymbole (grüne Füllung) in drei Richtungen.
Der "worst case" sind die vier inneren Symbole (mit blauer Füllung) mit jeweils vier Verfälschungsmöglichkeiten. Daraus folgt:

$$p_{\rm S} = {\rm Pr}({\cal{E}}) \le 4 \cdot p = \underline{1.6\%}= p_{\rm UB} \hspace{0.05cm}.$$

(4) Zählt man die blauen Pfeile in obiger Grafik, so kommt man auf

$$4 \cdot 2 + 8 \cdot 3 + 4 \cdot 4 = 48.$$

Die mittlere Symbolfehlerwahrscheinlichkeit ist somit gleich

$$p_{\rm S} = { E}/{ 16} \cdot 48 p = 3p = \underline{1.2\%} \hspace{0.05cm}.$$

Zum gleichen Ergebnis kommt man mit der im Theorieteil angegebenen Gleichung

$$p_{\rm S} = 4p \cdot \left [ 1 - { 1}/{ \sqrt{M}} \right ] = 4p \cdot \left [ 1 - { 1}/{ 4} \right ] = 3p \hspace{0.05cm}.$$

Beide Gleichungen gelten nur dann exakt, wenn man wie hier diagonale Verfälschungen ausschließt.

(5) Bei Graycodierung entsprechend der roten Beschriftung in der Grafik bewirkt jeder Symbolfehler genau einen Bitfehler.

Da aber mit jedem Symbol $M = 4$ Binärsymbole übertragen werden, ist

$$p_{\rm B} = \frac{ p_{\rm S}}{ {\rm log_2} \hspace{0.05cm}(M)} = \frac{ 1.2\%}{ 4} = \underline{0.3\%} \hspace{0.05cm}.$$