Exercise 3.7Z: Partial Fraction Decomposition

• Return to book

Pole-zero diagrams

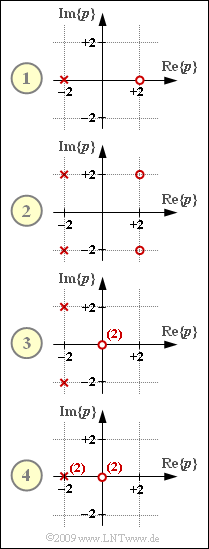
In the graph, four two-port networks are given by their pole–zero diagrams  $H_{\rm L}(p)$ .

• They all have in common that the number  $Z$  of zeros is equal to the number  $N$  of poles.
• The constant factor in each case is  $K=1$.

In the special case  $Z = N$  the residue theorem cannot be applied directly to compute the impulse response  $h(t)$ .

Rather, a partial fraction decomposition corresponding to

$$H_{\rm L}(p) =1- H_{\rm L}\hspace{0.05cm}'(p) \hspace{0.05cm}$$

must be made beforehand. Then,

$$h(t) = \delta(t)- h\hspace{0.03cm}'(t) \hspace{0.05cm}$$ holds for the impulse response.

 $h\hspace{0.03cm}'(t)$  is the inverse Laplace transform of  $H_{\rm L}\hspace{0.05cm}'(p)$ , where the condition  $Z' < N'$  is satisfied.

Two of the four configurations given are so-called all-pass filters.

• This refers to two-port networks for which the Fourier spectral function satisfies the condition  $|H(f)| = 1$   ⇒   $a(f) = 0$ .
• In Exercise 3.4Z it is given how the poles and zeros of such an all-pass filter must be positioned.

Furthermore, in this exercise the  $p$–transfer function

$$H_{\rm L}^{(5)}(p) =\frac{p/A}{\left (\sqrt{p/A}+\sqrt{A/p} \right )^2} \hspace{0.05cm}$$

⇒   "configuration $(5)$" will be examined in more detail, which can be represented by one of the four pole–zero diagrams given in the graph if the parameter  $A$  is chosen correctly.

Please note:

• The exercise belongs to the chapter  Inverse Laplace Transform.
• In particular, reference is made to the page  Partial fraction decomposition.

Questions

1

Which of the sketched two-port networks are all-pass filters?

	Configuration  $(1)$,
	configuration  $(2)$,
	configuration  $(3)$,
	configuration  $(4)$.

2

Which quadripole has the transfer function  $H_{\rm L}^{(5)}(p)$?

	Configuration  $(1)$,
	configuration  $(2)$,
	configuration  $(3)$,
	configuration  $(4)$.

3

Compute the function  $H_{\rm L}\hspace{0.01cm}'(p)$  after a partial fraction decomposition for configuration  (1).
Enter the function value for  $p = 0$ .

$H_{\rm L}\hspace{0.01cm}'(p = 0) \ = \ $

4

Compute  $H_{\rm L}\hspace{0.01cm}'(p)$  for the configuration  $(2)$.  Which statements are true here?

	$H_{\rm L}\hspace{0.01cm}'(p)$  has the same zeros as  $H_{\rm L}(p)$.
	$H_{\rm L}\hspace{0.01cm}'(p)$  has the same poles as  $H_{\rm L}(p)$.
	The constant factor of  $H_{\rm L}\hspace{0.01cm}'(p)$  is  $K' = 8$.

5

Compute  $H_{\rm L}\hspace{0.01cm}'(p)$  for the configuration  $(3)$.  Which statements are true here?

	$H_{\rm L}\hspace{0.01cm}'(p)$  has the same zeros as  $H_{\rm L}(p)$.
	$H_{\rm L}\hspace{0.01cm}'(p)$  has the same poles as  $H_{\rm L}(p)$.
	The constant factor of  $H_{\rm L}\hspace{0.01cm}'(p)$  is  $K' = 8$.

6

Compute  $H_{\rm L}\hspace{0.01cm}'(p)$  for the configuration  $(4)$.  Which statements are true here?

	$H_{\rm L}\hspace{0.01cm}'(p)$  has the same zeros as  $H_{\rm L}(p)$.
	$H_{\rm L}\hspace{0.01cm}'(p)$  has the same poles as  $H_{\rm L}(p)$.
	The constant factor of  $H_{\rm L}\hspace{0.01cm}'(p)$  is  $K' = 8$.

Solution

Solution

(1)  The suggested solutions 1 and 2 are correct:

• According to the criteria given in exercise 3.4Z, there is always an all-pass filter at hand if there is a corresponding zero  $p_{\rm o} = + A + {\rm j} \cdot B$  in the right $p$–half-plane for each pole  $p_{\rm x} = - A + {\rm j} \cdot B$  in the left half-plane.
• Considering  $K = 1$  the attenuation function is then  $a(f) = 0 \ \rm Np$   ⇒   $|H(f)| = 1$.
• The following can be seen from the graph on the information page:   The configurations  $(1)$ and  $(2)$ satisfy exactly these symmetry properties.

(2)  The suggested solution 4 is correct:

• The transfer function  $H_{\rm L}^{(5)}(p)$  is also described by configuration  $(4)$  as the following calculation shows:

$$H_{\rm L}^{(5)}(p) \hspace{0.25cm} = \hspace{0.2cm} \frac{p/A}{(\sqrt{p/A}+\sqrt{A/p})^2} =\frac{p/A}{{p/A}+2+ {A/p}} = \hspace{0.2cm}\frac{p^2}{p^2 + 2A \cdot p + A^2} = \frac{p^2}{(p+A)^2 }= H_{\rm L}^{(4)}(p) \hspace{0.05cm}.$$

• Die doppelte Nullstelle liegt bei  $p_{\rm o} = 0$, der doppelte Pol bei  $p_{\rm x} = -A = -2$.

(3)  Für die Konfiguration  $(1)$  gilt:

$$H_{\rm L}(p) =\frac{p-2}{p+2}=\frac{p+2-4}{p+2}= 1 - \frac{4}{p+2}=1- H_{\rm L}\hspace{-0.05cm}'(p) \hspace{0.3cm} \Rightarrow \hspace{0.3cm}H_{\rm L}\hspace{-0.05cm}'(p) = \frac{4}{p+2} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}\hspace{0.15cm}\underline{H_{\rm L}\hspace{-0.05cm}'(p =0) =2} \hspace{0.05cm}.$$

(4)  In gleicher Weise ergibt sich für die Konfiguration  $(2)$:

$$H_{\rm L}(p) =\frac{(p-2 - {\rm j} \cdot 2)(p-2 + {\rm j} \cdot 2)}{(p+2 - {\rm j} \cdot 2)(p+2 + {\rm j} \cdot 2)}= \frac{p^2 -4\cdot p +8 }{p^2 +4\cdot p +8}= \hspace{0.2cm}\frac{p^2 +4\cdot p +8 -8\cdot p}{p^2 +4\cdot p +8} =1- \frac{8\cdot p}{p^2 +4\cdot p +8}=1- H_{\rm L}\hspace{-0.05cm}'(p)$$

$$\Rightarrow \hspace{0.3cm}H_{\rm L}\hspace{0.05cm}'(p) = 8 \cdot \frac{p}{(p+2 - {\rm j} \cdot 2)(p+2 + {\rm j} \cdot 2)} \hspace{0.05cm}.$$

Richtig sind also die Lösungsvorschläge 2 und 3 im Gegensatz zur Aussage 1:

• Während  $H_{\rm L}(p)$  zwei konjugiert–komplexe Nullstellen aufweist,
• besitzt  $H_{\rm L}\hspace{0.01cm}'(p)$  nur eine einzige Nullstelle bei  $p_{\rm o}\hspace{0.01cm}' = 0$.

(5)  Für die Konfiguration  $(3)$  gilt:

$$H_{\rm L}(p) = \frac{p^2 }{p^2 +4\cdot p +8}=\frac{p^2 +4\cdot p +8 -4\cdot p -8 }{p^2 +4\cdot p +8} = 1- H_{\rm L}\hspace{-0.05cm}'(p)$$

$$\Rightarrow \hspace{0.3cm}H_{\rm L}\hspace{-0.05cm}'(p) = 4 \cdot \frac{p+2}{(p+2 - {\rm j} \cdot 2)(p+2 + {\rm j} \cdot 2)} \hspace{0.05cm}.$$

• Die Nullstelle von  $H_{\rm L}\hspace{0.01cm}'(p)$  liegt nun bei  $p_{\rm o}\hspace{0.01cm}' = -2$.
• Die Konstante ist  $K\hspace{0.01cm}' = 4$   ⇒   richtig ist hier nur der Lösungsvorschlag 2.

(6)  Schließlich gilt für die Konfiguration  $(4)$:

$$H_{\rm L}(p) = \frac{p^2 }{(p+2)^2}=\frac{p^2 +4\cdot p +4 -4\cdot p -4 }{p^2 +4\cdot p +4} = 1- \frac{4\cdot p +4 }{p^2 +4\cdot p +4} \hspace{0.3cm}\Rightarrow \hspace{0.3cm}H_{\rm L}\hspace{0.05cm}'(p) = 4 \cdot \frac{p+1}{(p+2)^2} \hspace{0.05cm}.$$

Richtig ist auch hier der Lösungsvorschlag 2. Allgemein lässt sich sagen:

• Durch die Partialbruchzerlegung wird die Anzahl und die Lage der Nullstellen verändert.
• Die Pole von $H_{\rm L}\hspace{0.01cm}'(p)$ sind dagegen stets identisch mit denen von $H_{\rm L}(p)$.