Consideration of Channel Distortion and Equalization

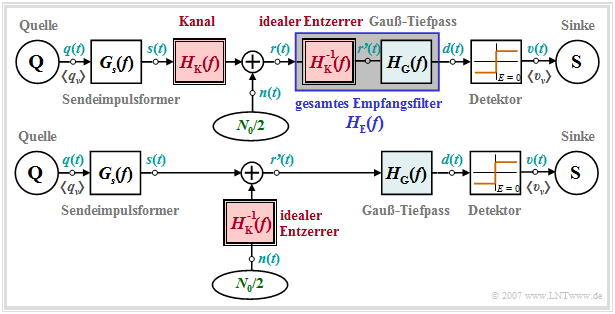
Ideal channel equalizer

For a transmission system whose channel frequency response $H_{\rm K}(f)$ causes severe distortion, we assume the following block diagram (upper graph) and equivalent circuit (lower graph).

Block diagram and equivalent circuit diagram for consideration of a channel frequency response

To these representations the following is to be noted:

The receiver filter $H_{\rm E}(f)$ is – at least mentally – composed of an ideal equalizer $1/H_{\rm K}(f)$ and a low-pass filter $H_{\rm G}(f)$. For the latter we use in this chapter exemplarily a Gaussian low-pass with the cutoff frequency $f_{\rm G}$.

If we now move the ideal equalizer – again purely mentally – to the left side of the noise addition point, nothing changes with respect to the S/N ratio at the sink and with respect to the error probability compared to the block diagram drawn above.

From the equivalent circuit below it can be seen that the channel frequency response $H_{\rm K}(f)$ does not change anything with respect to the signal component of the detection signal $d_{\rm S}(t)$ – originating from the transmitted signal $s(t)$ – if it is fully compensated with $1/H_{\rm K}(f)$. Thus, the signal component has exactly the same shape as calculated in the chapter Error Probability with Intersymbol Interference.

The degradation due to the channel frequency response $H_{\rm K}(f)$ is rather shown by a significant increase of the detection noise power, i.e. the variance of the signal $d_{\rm N}(t)$ – originating from the noise signal $n(t)$:

$$\sigma_d^2 = \frac{N_0}{2} \cdot \int_{-\infty}^{+\infty} |H_{\rm E}(f)|^2 \,{\rm d} f = \frac{N_0}{2} \cdot \int_{-\infty}^{+\infty} \frac{1}{|H_{\rm K}(f)|^2}\cdot |H_{\rm G}(f)|^2 \,{\rm d} f \hspace{0.05cm}.$$

The prerequisite for a finite noise power $\sigma_d^2$ is that the low-pass $H_{\rm G}(f)$ attenuates the noise $n(t)$ at (very) high frequencies more than it is raised by the ideal equalizer $1/H_{\rm K}(f)$.

Note: The channel frequency response $H_{\rm K}(f)$ can be equalized according to magnitude and phase, but only in a limited frequency range given by $H_{\rm G}(f)$. However, a complete phase equalization is only possible at the expense of a (frequency-independent) running time, which will not be considered further in the following.

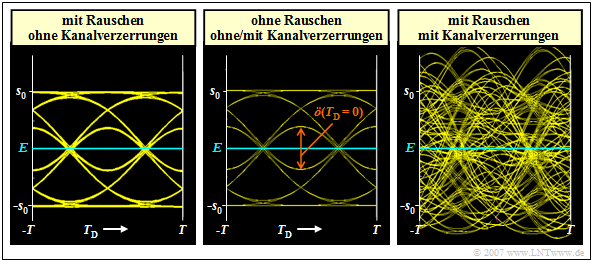
$\text{Example 1:}$ We consider again a binary system with NRZ rectangular pulses and Gaussian receiver filter $H_{\rm E}(f) = H_{\rm G}(f)$ with (normalized) cutoff frequency $f_\text{G, opt} \cdot T = 0.4$.

The middle graph shows the eye diagram of the signal component of the detection signal $d_{\rm S}(t)$ for this case – i.e. without taking the noise into account.
This is identical with the eye diagram shown in the chapter Definition and statements of the eye diagram in $\text{example 3}$ (right diagram).

Binary eye diagrams with intersymbol interferences

The left eye diagram is obtained for ideal channel, i.e., for $H_{\rm K}(f) = 1$ ⇒ $1/H_{\rm K}(f) = 1$. It takes into account the AWGN noise, but here it was assumed to be very small, $10 \cdot {\rm lg}\hspace{0.1cm} E_{\rm B}/N_0 = 30 \ \rm dB$. For this configuration, it was determined by simulation:

$$10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm U}\approx 26.8\,{\rm dB} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm U}< 10^{-40}\hspace{0.05cm}.$$

In contrast, the right diagram applies to a coaxial cable, where the characteristic cable attenuation $a_\star = 40 \ \rm dB$. This results in significantly worse system sizes for the same $E_{\rm B}/N_0$:

$$10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm U}\approx -4.6\,{\rm dB} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm U}\approx 0.28\hspace{0.05cm}.$$

This result can be interpreted as follows:

Assuming an ideal channel equalizer $1/H_{\rm K}(f)$, the same "eye diagram without noise" (left graph) results for the distorting channel as for the ideal channel $H_{\rm K}(f) = 1$ (middle graph).

Channel equalization $1/H_{\rm K}(f)$ extremely amplifies the noise component. In the right-hand example, equally strong equalization is required over a wide frequency range because of the strong distortion.
The noise power $\sigma_d^2$ here is larger by a factor of $1300$ than in the left constellation (no distortion ⇒ no equalization). Thus, the error probability results in $p_{\rm S}\approx p_{\rm U}\approx 50 \%$.

An acceptable error probability results only with smaller noise power density $N_0$. For example, with $10 \cdot {\rm lg}\hspace{0.1cm} E_{\rm B}/N_0 = 50 \ \rm dB$ $($instead of $30 \ \rm dB)$ the following result is obtained:

$$10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm U} = -4.6 +20 \approx 15.4\,{\rm dB} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm U}\approx 2 \cdot 10^{-9} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm S} \ge p_{\rm U}/4 \approx 0.5 \cdot 10^{-9}\hspace{0.05cm}.$$

Increase of the noise power by linear equalization

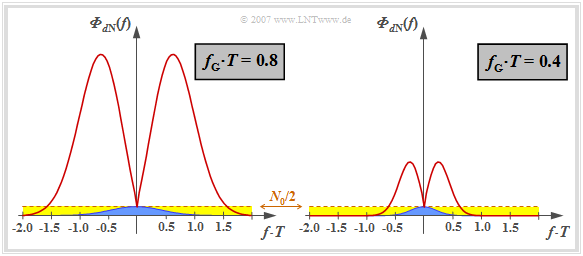
The eye diagrams on the last section impressively document the increase of the noise power $\sigma_d^2$ with unchanged vertical eye opening, if one compensates the channel frequency response $H_{\rm K}(f)$ on the receiving side by its inverse. This result shall now be interpreted in terms of the noise power density ${\it \Phi}_{d{\rm N}}(f)$ after the receiver filter (before the decision), with the following settings:

Let the channel be a coaxial cable with the magnitude frequency response

$$|H_{\rm K}(f)| = {\rm exp}\left [- a_{\star}\cdot \sqrt{2 f T}\hspace{0.05cm} \right ]\hspace{0.2cm}{\rm with}\hspace{0.2cm} a_{\star} = 1.7\,\,{\rm Np}\hspace{0.2cm} ({\rm corresponding to} \hspace{0.2cm} 15\,\,{\rm dB}) \hspace{0.05cm}.$$

The ideal channel equalizer $1/H_{\rm K}(f)$ compensates the channel frequency response completely. No statement is made here about the realization of the attenuation and phase equalization.

For noise power limitation a Gaussian low-pass filter is used:

$$|H_{\rm G}(f)| = {\rm exp}\left [- \pi \cdot \left (\frac{f }{2 f_{\rm G}}\right )^2 \right ]\hspace{0.2cm}{\rm with}\hspace{0.2cm} f_{\rm G} = 0.8/T \hspace{0.2cm} {\rm and} \hspace{0.2cm} f_{\rm G} = 0.4/T \hspace{0.05cm}.$$

Thus, the noise power density before the decision is:

$${\it \Phi}_{d{\rm N}}(f) = \frac{N_0}{2} \cdot \frac{|H_{\rm G }(f)|^2}{|H_{\rm K}(f)|^2} = \frac{N_0}{2} \cdot {\rm exp}\left [2 \cdot a_{\star}\cdot \sqrt{2 f T} - {\pi}/{2} \cdot \left ({f }/{f_{\rm G}}\right )^2 \right ] \hspace{0.05cm}.$$

Noise rise due to distorting channel

This curve is shown on the left for the two (normalized) cutoff frequencies

$f_\text{G} \cdot T = 0.8$ (left) and
$f_\text{G} \cdot T = 0.4$ (right)

Note that here, for reasons of presentation, the characteristic cable attenuation of $a_\star = 15 \ \rm dB$ $($corresponding to $1.7 \ \rm Np)$ is chosen to be significantly smaller than in the right eye diagram in $\text{Example 1}$ in the last section $($valid for $a_\star = 40 \ \rm dB)$.
Let us first consider the left graph for the (normalized) cutoff frequency $f_\text{G} \cdot T = 0.8$ which, according to the calculations in the last chapter represents the optimum for the ideal channel ⇒ $H_{\rm K}(f) = 1$.

The constant noise power density $N_0/2$ at the receiver input is highlighted in yellow. With an ideal channel, this is limited by the Gaussian receive filter $H_{\rm E}(f) = H_{\rm G}(f)$ and results in the detection noise power $\sigma_d^2$ (indicated by the blue area in the graph).

If – as usual for conducted transmission üblich – higher frequencies are strongly attenuated, $|H_{\rm E}(f)| = |H_{\rm G}(f)|/|H_{\rm K}(f)|$ increases very strongly due to the ideal channel equalizer before the attenuating influence of the Gaussian filter becomes effective for $f \cdot T \ge 0.6$ $($only valid for $a_\star = 15 \ \rm dB$ and $f_\text{G} \cdot T = 0.8)$.

The noise power $\sigma_d^2$ is now equal to the area under the red curve, which is about a factor of $28$ larger than the blue area. The effects of these different noise powers can also be seen in the eye diagrams on the last section, but for $a_\star = 40 \ \rm dB$.

The right graph shows the noise power density ${\it \Phi}_{d{\rm N}}(f)$ for the normalized cutoff frequency $f_\text{G} \cdot T = 0.4$. Here the noise power is only increased by a factor of $9$ by the ideal channel equalizer (ratio between the area under the red curve and the blue area).

$\text{Conclusion:}$ From the above graph and the previous explanations it is already clear that with distorting channel ⇒ $H_{\rm K}(f) \ne 1$ the cutoff frequency $f_\text{G} \cdot T = 0.8$ of the Gaussian low-pass filter $H_{\rm G}(f)$ will no longer be optimal after the ideal channel equalizer $1/H_{\rm K}(f)$.

Optimization of the cutoff frequency

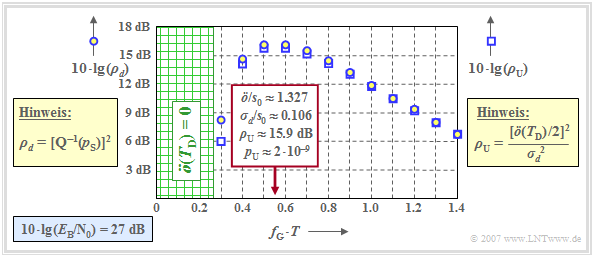
Die Grafik zeigt die Störabstände in Abhängigkeit der Grenzfrequenz $f_{\rm G}$ des gaußförmigen Gesamtfrequenzgangs $H_{\rm G}(f) = H_{\rm K}(f) \cdot H_{\rm E}(f)$. Dieses Bild gilt für

einen koaxialen Übertragungskanal mit der charakteristischen Kabeldämpfung $a_\star = 15 \ \rm dB$,
AWGN–Rauschen mit $10 \cdot {\rm lg}\hspace{0.1cm} E_{\rm B}/N_0 = 27 \ \rm dB$, wobei $E_{\rm B} = s_0^2 \cdot T$ zu setzen ist ⇒ NRZ–Rechteckimpulse.

Optimale Grenzfrequenz des GTP bei verzerrendem Kanal $(a_\star = 15 \ \rm dB)$

Anmerkungen:

Die Kreise zeigen die dB–Werte für $10 \cdot {\rm lg}\hspace{0.1cm} \rho_d$ ⇒ "mittleres" Detektions–SNR (Maß für die mittlere Fehlerwahrscheinlichkeit $p_{\rm S})$.
Die Quadrate zeigen die dB–Werte für $10 \cdot {\rm lg}\hspace{0.1cm} \rho_{\rm U}$ ⇒ "ungünstigstes" SNR $($Maß für die ungünstigste Fehlerwahrscheinlichkeit $p_{\rm U})$.

Man erkennt aus dieser Darstellung und durch Vergleich mit der entsprechenden Grafik im letzten Kapitel, die für $H_{\rm K}(f) = 1$ und $10 \cdot {\rm lg}\hspace{0.1cm} E_{\rm B}/N_0 = 13 \ \rm dB$ gegolten hat:

Auch bei stark verzerrendem Kanal ist $\rho_{\rm U}$ eine geeignete untere Schranke für $\rho_d$ ⇒ $\rho_{d} \ge \rho_{\rm U}$. Entsprechend ist auch $p_{\rm U} \ge p_{\rm S} $ eine sinnvolle obere Schranke für $p_{\rm S}$.

Bei der betrachteten Kabeldämpfung $a_\star = 15 \ \rm dB$ ist die Grenzfrequenz $f_\text{G} \cdot T \approx 0.55$ optimal und es gilt $\ddot{o}/s_0 \approx 1.327$ sowie $\sigma_d/s_0 \approx 0.106$.
Daraus ergeben sich der (ungünstigste) Störabstand $10 \cdot {\rm lg}\hspace{0.1cm} \rho_{\rm U} \approx \ \rm 15.9 \ dB$ und die Worst–Case–Fehlerwahrscheinlichkeit $p_{\rm U} \approx 2 \cdot 10^{-9}.$

Eine kleinere Grenzfrequenz hätte eine deutlich kleinere Augenöffnung zur Folge, ohne dass dadurch auch $\sigma_d$ gleichermaßen verkleinert würde. Für $f_\text{G} \cdot T = 0.4$ gilt:

$$\ddot{o}/s_0 \approx 0.735,\hspace{0.2cm}\sigma_d/s_0 \approx 0.072\hspace{0.3cm}\Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm U}\approx 14.1\,{\rm dB} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm U}\approx 1.8 \cdot 10^{-7}\hspace{0.05cm}.$$

Ist die Grenzfrequenz $f_\text{G}$ zu groß, so wird das Rauschen weniger effektiv begrenzt. Beispielsweise lauten die Werte für die Grenzfrequenz $f_\text{G} \cdot T =0.8$:

$$\ddot{o}/s_0 \approx 1.819,\hspace{0.2cm}\sigma_d/s_0 \approx 0.178\hspace{0.3cm}\Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm U}\approx 14.2\,{\rm dB} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm U}\approx 1.7 \cdot 10^{-7}\hspace{0.05cm}.$$

Die optimalen Werte sind mit $10 \cdot {\rm lg}\hspace{0.1cm} \rho_{d} \approx 16.2 \ \rm dB$ und $10 \cdot {\rm lg}\hspace{0.1cm} \rho_{\rm U} \approx \ \rm 15.9 dB$ deutlich ausgeprägter als bei idealem Kanal.

Bei einem Vergleich der Störabstände ist allerdings zu berücksichtigen, dass hier $10 \cdot {\rm lg}\hspace{0.1cm} E_{\rm B}/N_0 = 27 \ \rm dB$ zugrunde liegt; in der entsprechenden Grafik für den idealen Kanal wurde dagegen von $10 \cdot {\rm lg}\hspace{0.1cm} E_{\rm B}/N_0 = 13 \ \rm dB$ ausgegangen.

Optimale Grenzfrequenz in Abhängigkeit der Kabeldämpfung

Optimale Grenzfrequenz und Systemwirkungsgrad in Abhängigkeit der charakteristischen Kabeldämpfung. Insbesondere gilt:
$\hspace{0.8cm} 10 · \lg \eta\hspace{0.05cm}(a_\star = 0 \ \rm dB) = -1.4 \ dB;$ $\hspace{0.8cm} 10 · \lg \eta\hspace{0.05cm}(a_\star = 80 \ \rm dB) = -78.2 \ dB;$

Wir betrachten weiter

ein Binärsystem mit NRZ–Sendeimpulsen ⇒ $E_{\rm B} = s_0^2 \cdot T$,
ein Koaxialkabel $H_{\rm K}(f)$, charakteristische Dämpfung $a_\star$,
einen Gauß–Gesamtfrequenzgang $H_{\rm G}(f) = H_{\rm K}(f) \cdot H_{\rm E}(f)$.

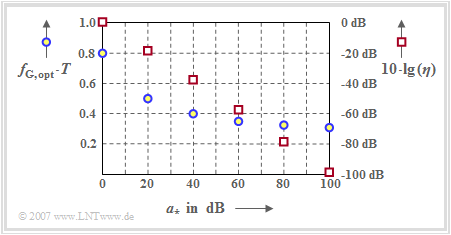
Die blauen Kreise (linke Achsenbeschriftung) markieren die optimale Grenzfrequenzen $f_\text{G, opt}$ für die jeweilige Kabeldämpfung $a_\star$.

Zusätzlich ist in der Grafik mit roten Quadraten der Systemwirkungsgrad (bei Spitzenwertbegrenzung) $\eta$ dargestellt, der das Verhältnis des mit der betrachteten Konfiguration erreichbaren SNR $\rho_{d}$ zum maximal möglichen S/N-Verhältnis $\rho_{d, \ {\rm max}}$ angibt.

Ersetzt man $\rho_d$ durch $\rho_{\rm U}$, also $p_{\rm S}$ durch $p_{\rm U}$, so kann der Systemwirkungsgrad wie folgt dargestellt werden:

$$\eta = \eta_{\rm A}=\frac{\rho_d}{\rho_{d, \hspace{0.05cm}{\rm max \hspace{0.05cm}|\hspace{0.05cm} A}}}= \frac{\rho_d}{2 \cdot E_{\rm B}/N_0}\approx \frac{\rho_{\rm U}}{2 \cdot E_{\rm B}/N_0}.$$

Man erkennt aus der Anordnung der blauen Kreise:

Die optimale Grenzfrequenz $f_\text{G, opt}$ hängt signifikant ab von der Stärke der Verzerrungen des Koaxialkabels, genauer gesagt: ausschließlich von der charakteristischen Kabeldämpfung $a_\star$ bei der halben Bitrate.
Je größer die Kabeldämpfung $a_\star$ und damit der Rauscheinfluss ist, um so niedriger ist die optimale Grenzfrequenz $f_\text{G, opt}$.
Allerdings ist stets $f_\text{G, opt} > 0.27/T$. Andernfalls wäre das Auge geschlossen, gleichbedeutend mit der "Worst–case"–Fehlerwahrscheinlichkeit $p_{\rm U} = 0.5$.

Diskutieren wir nun die Abhängigkeit des Systemwirkungsgrads $\eta$ (rote Quadrate) von der charakteristischen Kabeldämpfung $a_\star$. Die rechte Ordinate beginnt oben bei $0 \ \rm dB$ und erstreckt sich nach unten bis $-100 \ \rm dB$.

Wie nun an einigen Zahlenbeispielen verdeutlicht werden soll, vermeidet die Darstellung $\eta = \eta\hspace{0.05cm}(a_\star)$ einige Probleme, die sich aus dem großen Wertebereich von S/N–Verhältnissen ergeben:

Der Ordinatenwert $10 \cdot {\rm lg}\hspace{0.1cm} \eta\hspace{0.05cm}(a_\star = 0 \ \rm dB) = -1.4 \ \rm dB$ sagt aus, dass der bei idealem Kanal bestmögliche Gaußtiefpass mit Grenzfrequenz $f_\text{G} \cdot T = 0.8$ um $1.4 \ \rm dB$ schlechter ist als der optimale (Matched–Filter–) Empfänger.

Gehen wir von idealem Kanal $(a_\star = 0 \ \rm dB)$ und $10 \cdot {\rm lg}\hspace{0.1cm} E_{\rm B}/N_0 = 10 \ \rm dB$ aus, so besagt die obige Gleichung auch, dass diese Konfiguration zu folgender (worst-case) Fehlerwahrscheinlichkeit führen wird:

$$10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm U} = 10 \cdot {\rm lg}\hspace{0.1cm}{E_{\rm B}}/{N_0} + 10 \cdot {\rm lg}\hspace{0.1cm}(2) + 10 \cdot {\rm lg}\hspace{0.1cm}(\eta) \approx \approx 10\,{\rm dB} \hspace{0.1cm}+\hspace{0.1cm}3\,{\rm dB} \hspace{0.1cm}-\hspace{0.1cm}1.4\, {\rm dB}= 11.6\,{\rm dB} \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm U}\approx 7 \cdot 10^{-5}\hspace{0.05cm}.$$

Soll diese (ungünstigste) Fehlerwahrscheinlichkeit $p_{\rm U} = 7 \cdot 10^{-5}$ ⇒ $10 \cdot {\rm lg}\hspace{0.1cm} \rho_{\rm U} = 11.6 \ \rm dB$ beim Kanal mit der charakteristischen Kabeldämpfung $a_\star = 80 \ \rm dB$ nicht überschritten werden, so muss demnach für das Verhältnis $E_{\rm B}/N_0$ gelten:

\[10 \cdot {\rm lg}\hspace{0.1cm}{E_{\rm B}}/{N_0} \ge 11.6\,{\rm dB} \hspace{0.1cm}-3\,{\rm dB} \hspace{0.1cm}-\hspace{0.1cm}(-78.2)\,{\rm dB}= 86.8\,{\rm dB} \hspace{0.2cm} \Rightarrow \hspace{0.2cm}{E_{\rm B}}/{N_0}\approx 5 \cdot 10^{8}\hspace{0.05cm}.\]

Um dies zu erreichen, muss allerdings die Grenzfrequenz des Gaußtiefpasses entsprechend den blauen Kreisen in der Grafik auf $f_{\rm G}= 0.33/T$ herabgesetzt werden.

Aufgaben zum Kapitel

Aufgabe 3.3: Rauschen bei Kanalentzerrung

Aufgabe 3.3Z: Optimierung eines Koaxialkabelsystems