Exercise 2.2Z: Distortion Power again
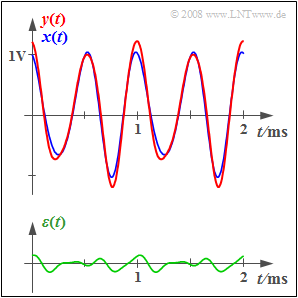
At the input of the considered functional unit, which is not specified in more detail, there is the periodic signal $x(t)$ shown in blue in the graph. This is given by the spectrum of the corresponding analytical signal:
- $$X_+(f) = {1 \,\rm V} \cdot {\rm \delta}(f- {2 \,\rm kHz}) + {0.2 \,\rm V} \cdot {\rm e}^{\rm j \hspace{0.05cm}\cdot \hspace{0.05cm}90^{\circ} } \cdot \delta(f- {3 \,\rm kHz}).$$
This spectral function is obtained from the usual spectrum $X(f)$, by
- truncating all components at negative frequencies, and
- doubling the components at positive frequencies.
For more details on the analytical signal and its spectrum, see the chapter Analytical Signal and its Spectral Function of the book "Signal Representation".
The spectrum of the analytical signal at the output of the functional unit is:
- $$Y_+(f) = {1.1 \,\rm V} \cdot {\rm \delta}(f- {2 \,\rm kHz}) + {0.25 \,\rm V} \cdot {\rm e}^{\rm j \hspace{0.05cm}\cdot \hspace{0.05cm} 60^{\circ} } \cdot \delta(f- {3 \,\rm kHz})+ {0.05 \,\rm V} \cdot {\rm e}^{-\rm j \hspace{0.05cm}\cdot \hspace{0.05cm} 90^{\circ} } \cdot \delta(f- {5 \,\rm kHz}).$$
The bottom sketch shows the difference signal $\varepsilon(t) = y(t) - x(t)$. A measure of the distortion created in the system is the "distortion power" referenced to the resistance $R = 1 \ \rm \Omega$ .
- $$P_{\rm V} = \overline{\varepsilon^2(t)} = \frac{1}{T_{\rm 0}} \cdot \int_{0}^{ T_{\rm 0}} {\varepsilon^2(t) }\hspace{0.1cm}{\rm d}t.$$
It should be noted that the distortion power can also be calculated in the spectral domain – in fact in a simpler way here.
The power $P_x$ of the input signal $x(t)$ is defined in an analogous way. Als quantitatives Maß für die Stärke der Verzerrungen wird das Signal–zu–Verzerrungs–Leistungsverhältnis angegeben, das meistens logarithmisch (in dB) dargestellt wird:
- $$10 \cdot {\rm lg} \hspace{0.1cm}\rho_{\rm V} = 10 \cdot {\rm lg} \hspace{0.1cm}{ P_{x}}/{P_{\rm V}} \hspace{0.05cm}.$$
Please note:
- The exercise belongs to the chapter Classification of the Distortions.
- Alle hier abgefragten Leistungen beziehen sich auf den Widerstand $R = 1 \ \rm \Omega$ und haben somit die Einheit ${\rm V}^2$.
- Die Leistung eines (reellen) Signals $x(t)$ kann auch aus der Spektralfunktion $X(f)$ berechnet werden:
- $$P_{x} =\frac{1}{T_{\rm 0}} \cdot\int_{-\infty}^{ \infty} x^2(t)\hspace{0.1cm}{\rm d}t = \frac{1}{T_{\rm 0}} \cdot \int_{-\infty}^{ \infty} |X(f)|^2\hspace{0.1cm}{\rm d}f.$$
Questions
Solution
- Der größte gemeinsame Teiler von $f_1 = 2 \ \rm kHz$ und $f_2 = 3 \ \rm kHz$ ist $f_0 = 1 \ \rm kHz$.
- Damit beträgt die Periodendauer $T_0 = 1/f_0 = 1 \ \rm ms$.
- Das Signal lautet aufgrund des Phasenterms ${\rm e}^{{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}90^\circ}$:
- $$x(t) = {1 \, \rm V} \cdot {\rm cos}(2\pi f_1 t ) - {0.2 \, \rm V} \cdot {\rm sin}(2\pi f_2 t ).$$
(2) Um die Leistung im Zeitbereich zu berechnen, muss das Signal $x(t) = x_1(t) + x_2(t)$ quadriert und über ein geeignetes Zeitintervall gemittelt werden.
- Für ein periodisches Signal genügt die Mittelung über $T_0$:
- $$P_{\rm V} = \frac{1}{T_{\rm 0}} \cdot \int_{0}^{ T_{\rm 0}} {\left[x_1(t)+ x_2(t) \right]^2 }\hspace{0.1cm}{\rm d}t = \frac{1}{T_{\rm 0}} \cdot \int_{0}^{ T_{\rm 0}} {x_1^2(t) }\hspace{0.1cm}{\rm d}t \hspace{0.1cm}+\hspace{0.1cm} \frac{1}{T_{\rm 0}} \cdot \int_{0}^{ T_{\rm 0}} {x_2^2(t) }\hspace{0.1cm}{\rm d}t \hspace{0.1cm}+\hspace{0.1cm} \frac{2}{T_{\rm 0}} \cdot \int_{0}^{ T_{\rm 0}} { x_1(t) \cdot x_2(t) }\hspace{0.1cm}{\rm d}t.$$
- Das erste Integral liefert:
- $$P_{\rm 1} = \frac{1}{T_{\rm 0}} \cdot \int_{0}^{ T_{\rm 0}} { ({1 \, \rm V})^2 \cdot {\rm cos}^2(2\pi f_1 t )}\hspace{0.1cm}{\rm d}t = \frac{1 \, \rm V^2}{2 T_{\rm 0}}\hspace{0.05cm} \cdot \int\limits_{0}^{ T_{\rm 0}} { \left[ 1+ {\rm cos}(4\pi f_1 t )\right]}\hspace{0.1cm}{\rm d}t = {0.5 \, \rm V^2}.$$
- In gleicher Weise erhält man für die Leistung des zweiten Terms: $P_2 = (0.2 \ {\rm V})^2/2 = 0.02 \ {\rm V}^2.$
- Das letzte Integral liefert keinen Beitrag, da $x_1(t)$ und $x_2(t)$ zueinander orthogonal sind. Somit erhält man für die gesamte Signalleistung:
- $$P_{x} =P_{\rm 1} + P_{\rm 2} = {0.5 \, \rm V^2} + {0.02 \, \rm V^2}\hspace{0.15cm}\underline{ = {0.52 \, \rm V^2}}.$$
Dieses Ergebnis kann man auch aus der Spektralfunktion herleiten, wenn man die Amplituden aller diskreten Spektralanteile quadriert, halbiert und aufsummiert. Die Phasenlagen der einzelnen Spektrallinien müssen dabei nicht berücksichtigt werden.
(3) Unabhängig davon, ob ein lineares oder ein nichtlineares System vorliegt, kann für das analytische Spektrum des Differenzsignals $\varepsilon(t) = y(t) - x(t)$ mit $f_2 = 2 \ \rm kHz$, $f_3 = 3 \ \rm kHz$ und $f_5 = 5 \ \rm kHz$ geschrieben werden:
- $$E_+(f) = Y_+(f) - X_+(f) = {0.1 \,\rm V} \cdot {\rm \delta}(f- f_2) + \left[{0.25 \,\rm V} \cdot {\rm e}^{\rm j \cdot 60^{\circ} } - {0.2 \,\rm V} \cdot {\rm e}^{\rm j \cdot 90^{\circ} } \right] \cdot \delta(f- f_3) + {0.05 \,\rm V} \cdot {\rm e}^{-\rm j \cdot 90^{\circ} } \cdot \delta(f- f_5).$$
- Die komplexe Amplitude des zweiten Terms ist:
- $$C_2 = {0.25 \,\rm V} \cdot \cos( 60^{\circ}) + {\rm j} \cdot{0.25 \,\rm V} \cdot \sin( 60^{\circ}) - {\rm j} \cdot{0.05 \,\rm V} $$
- $$\Rightarrow \hspace{0.3cm} C_2 = {0.25 \,\rm V} \cdot 0.5 + {\rm j} \cdot{0.25 \,\rm V} \cdot 0.866 - {\rm j} \cdot{0.2 \,\rm V} = {0.125 \,\rm V} + {\rm j} \cdot{0.016 \,\rm V}.$$
- Damit ergibt sich für den Betrag:
- $$|C_2| = \sqrt{({0.125 \,\rm V})^2+({0.016 \,\rm V})^2 }= {0.126 \,\rm V}.$$
- Die Phasenlagen müssen bei der Leistungsberechnung nicht berücksichtigt werden. Somit gilt:
- $$P_{\rm V} = \frac{1}{2} \cdot \left[ ({0.1 \,\rm V})^2 + ({0.126 \,\rm V})^2 + ({0.05 \,\rm V})^2\right] \hspace{0.15cm}\underline{= {0.0142 \, \rm V^2}}.$$
(4) Entsprechend der Definition auf der Angabenseite gilt:
- $$\rho_{\rm V} = \frac{ P_{x}}{P_{\rm V}}= \frac{ {0.52 \, \rm V^2}}{0.0142 \, \rm V^2}\hspace{0.05cm}\rm = 36.65\hspace{0.3cm} \Rightarrow \hspace{0.3cm} 10 \cdot {\rm lg}\hspace{0.1cm}\rho_{\rm V} \hspace{0.15cm}\underline{= {15.64 \, \rm dB}}.$$