Envelope Demodulation

1. Modulation Methods
2. Amplitudenmodulation und AM–Demodulation
3. Envelope Demodulation

• Previous page
• Next page

• Previous page
• Next page

Functionality under ideal conditions

Wie first assume the following conditions:

• Let the source signal  $q(t)$  be free of a DC component and limited in magnitude to  $q_{\rm max}$ .
• The transmission is based on the modulation method „DSB–AM with carrier”. 
• For simplicity of representation, the carrier phase is set to  $\mathbf{ϕ_{\rm T} } = 0$  without restricting generality:

$$s(t) = \left(q(t) + A_{\rm T}\right) \cdot \cos (\omega_{\rm T}\cdot t )\hspace{0.05cm}.$$

• Let the modulation depth be $m ≤ 1$.  Therefore, from the definition $m = q_{\rm max}/A_{\rm T}$  it also follows that  $q(t) + A_{\rm T} ≥ 0$.
• Let the channel be ideal, that is, there is no distortion, no attenuation, no delay, and no (noise) interference.
• Thus, when  $H_{\rm K}(f) = 1$  and  $n(t) \equiv 0$  we get

$$r(t) = s(t) = a(t) \cdot \cos (\omega_{\rm T}\cdot t )\hspace{0.05cm}$$

for the received signal.

• In this equation,  $a(t)$  describes the   envelope of  $r(t)$.  The phase function is  $\mathbf{ϕ}(t) = 0$.

$\text{Definition:}$   An   envelope demodulator  detects the envelope  $a(t)$  of its input signal  $r(t)$  and outputs it as a sink signal after eliminating the DC component  $A_{\rm T}$ :

$$v(t) = a(t) - A_{\rm T}\hspace{0.05cm}.$$

The removal of the DC component  $A_{\rm T}$  can be realized, for example, by a high-pass filter that allows all frequencies to pass unimpeded except for $f = 0$ .

• If all the above conditions are met, then $v(t) = q(t)$ holds.
• This means that an ideal message communication system can certainly be realized with an (ideal) envelope demodulator.

Signal waveforms illustrating envelope demodulation

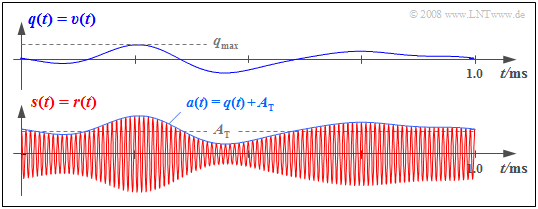
$\text{Example 1:}$   In the graph, the received signal  $r(t) = s(t)$  is shown below, and is based on "DSB–AM with carrier” $($modulation depth  $m = 0.5)$.

• The envelope  $a(t)$  to be evaluated by the envelope demodulator is equal to the sum of the source signal  $q(t)$  and the DC component  $A_{\rm T}$ added at the transmitter.

•  $v(t) = q(t)$ holds for the demodulator output signal after removing the DC component  $A_{\rm T}$  with a high-pass filter, assuming that the source signal  $q(t)$  did not include a DC component.   Such a component would (wrongly) also be removed by the high-pass filter.

Realizing an envelope demodulator

rechts

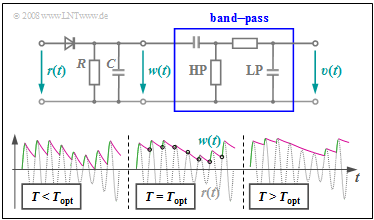
The adjacent graph shows:

• above, a simple possible realization of the envelope demodulator above,
• below, the signals  $r(t)$  and  $w(t)$  to illustrate the principle.

First, consider the middle signal section denoted by  $T = T_{\rm opt}$ .

The first circuit section – consisting of a diode and a parallel connection of a resistor  $R$  and a capacitance $C$  – performs the following tasks:

• If the (light) grey signal $r(t)$ is larger than the voltage  $r(t)$  at  $R$  and  $C$, the diode conducts,  $w(t) = r(t)$  holds, and the capacitance $C$ is charged. In these regions, the signal  $w(t)$  is drawn in green.
• If  $r(t) < w(t)$ , as it does at the times marked in purple, the diode blocks and the capacitance discharges through resistor  $R$. The signal  $w(t)$  decays exponentially with time constant  $T = R · C$ .
• From the times marked with circles,  $r(t) > w(t)$  holds again and the capacitance is recharged. It can be seen from the sketch that  $w(t)$  "approximately" matches the envelope  $a(t)$ .

$\text{Design criteria:}$  

• The deviations between the envelope approximation  $w(t)$  and its nominal function  $a(t)$  decrease the larger the carrier frequency  $f_{\rm T}$  is compared to the bandwidth  $B_{\rm NF}$  of the low frequency message signal. As a guideline,  $f_{\rm T} ≥ 100 · B_{\rm NF}$  is often given.
• At the same time, the time constant  $T$  of the RC –parallel resonant circuit should always be much larger than  $1/f_{\rm T}$  and much smaller than  $1/B_{\rm NF}$ . A good compromise is the geometric mean between the two limits:

$$1/f_{\rm T}\hspace{0.1cm} \ll \hspace{0.1cm} T \hspace{0.1cm} \ll \hspace{0.1cm} 1/B_{\rm NF} \hspace{0.05cm}, \hspace{2cm} T_{\rm opt} = {1}/{\sqrt{f_{\rm T} \cdot B_{\rm NF} } } \hspace{0.05cm}.$$

• If the time constant  $T$  is too small, as in the left area of the sketch above, the capacitor will always discharge too quickly and the deviation  $w(t) \ – \ a(t)$  will be unnecessarily large.
• Also, too large a value  $T > T_{\rm opt}$  will result in deterioration, as shown in the right signal detail. In this case,  $w(t)$  can no longer follow the envelope  $a(t)$ .

$\text{Example 2:}$   For a LF-bandwidth of  $5 \ \rm kHz$ , the carrier frequency should be at least  $500 \ \rm kHz$ .

• The time constant  $T$  must be much larger than  $1/f_{\rm T} = 2 \ \rm µ s$  and at the same time much smaller than  $1/B_{\rm NF} = 200 \ \rm µ s $.
• The optimal value according to the compromise formula is thus:

$$T_{\rm opt} = 1/\sqrt{ 5 \cdot 10^5 \ {\rm Hz} \cdot 5 \cdot 10^3 \ {\rm Hz} } = 20 \ \rm µ s \hspace{0.05cm}.$$

Representation of an envelope demodulator in the frequency domain.

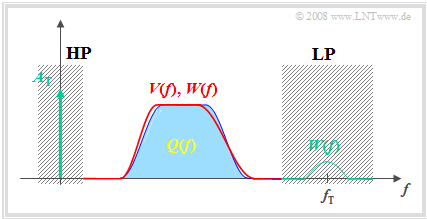
The graph on the right is intended to illustrate the operation of the envelope demodulator in the frequency domain. The spectrum  $W(f)$  of the signal  $w(t)$  at the RC parallel circuit differs from the spectrum  $Q(f)$  of the source signal as follows:

• Due to the carrier signal  $z(t)$  added at the transmitter, the spectral function  $W(f)$  includes a Dirac line at  $f = 0$  with weight  $A_{\rm T}$  (carrier amplitude).
• $W(f)$  also exhibits spectral components in the region around the carrier frequency  $f_{\rm T}$ , which can be explained by the jagged time course of  $w(t)$  (see the first graph in this section).
•  $W(f)$  also differs only slightly from  $Q(f)$  in the LF domain. Here, the error gets smaller as the carrier frequency increases compared to the AF bandwidth.

The first two signal distortions are eliminated by the high-pass and low-pass filters, which together produce a bandpass. However, there also remains a slight deviation between the sink signal  $v(t)$  and the source signal  $q(t)$  in the interesting range  $0 < f < B_{\rm NF}$ , as shown by comparing the output spectrum  $V(f)$  plotted in red and the input spectrum  $Q(f)$  plotted in blue.

Why envelope demodulation fails for   $m > 1$ 

Envelope demodulation when   $m < 1$  (above) and  $m > 1$  (below)

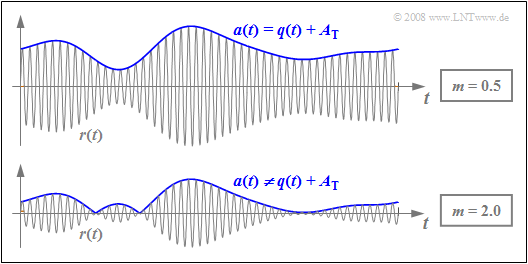
The graph shows the DSB-AM signals for  $m = 0.5$  and  $m = 2$. 

From this picture one can recognise the following differences:

• For a modulation depth  $m ≤ 1$  the envelope of the bandpass signal is characterised by:

$$a(t) = q(t) + A_{\rm T}\hspace{0.05cm}.$$

Here, an ideal demodulation is possible with an envelope demodulator   ⇒ $v(t) = q(t)$, if we ignore unavoidable noise.

• In contrast, when  $m > 1$ , the following relationship holds:

$$a(t) = {q(t) + A_{\rm T} }\hspace{0.05cm}.$$

• Here, envelope demodulation always leads to  nonlinear distortions.
• The sink signal  $v(t)$  now includes new frequencies, which were not present in $q(t)$ .
• For the DC component(expected value) of the envelope der Hüllkurve gilt in diesem Fall:  

$${\rm E}[a(t)] \ne A_{\rm T}\hspace{0.05cm}.$$

• Da nun anstelle von  $A_{\rm T}$  dieser Gleichanteil  ${\rm E}[a(t)]$  durch den Hochpass entfernt wird, kommt es zusätzlich zu einer Pegelverschiebung.

Description using the equivalent low-pass signal

Especially when the source signal  $q(t)$  can be represented as a sum of several harmonic oscillations, a signal description with the equivalent low-pass signal  ${|r_{\rm TP}(t)|}$  is extremely advantageous. This is described in detail in the book  Signal Representation .

$\text{Please note:}$  If noise/interference is disregarded, the received signal can be written as:

$$r(t) = a(t) \cdot \cos (\omega_{\rm T}\cdot t + \phi(t))\hspace{0.05cm}.$$

This equation is valid for any form of amplitude modulation under different boundary conditions:

• Double sideband (DSB) or single sideband (SSB),
• with or without a carrier,
• ideal channel or linear distorting channel.

In general, the associated equivalent low-pass signal is complex and given as:

$$r_{\rm TP}(t) = a(t) \cdot {\rm e}^{\hspace{0.03cm}{\rm j} \hspace{0.05cm} \cdot \hspace{0.05cm} \phi(t)}\hspace{0.05cm}.$$

The time functions  $a(t)$  and  $ϕ(t)$  contained in the equations are identical for both representations:

• $a(t)$  describes the  envelope  (time-dependent amplitude) of the physical signal  $r(t)$  and the magnitude  $\vert r_{\rm TP}(t) \vert $  of the equivalent LP signal, respectively.  This is detected during envelope demodulation.
• $ϕ(t)$  is the  time-dependent phase.  This function contains all information about the position of the zero crossings of  $r(t)$  and indicates whether an additional phase modulation is effective.

In the case of double sideband amplitude modulation, the following holds for an ideal channel:

• The locus curve – by this we mean the time-dependent representation of the signal  $r_{\rm TP}(t)$  in the complex plane – is a horizontal straight line on the real axis.
• It further follows that the phase function can take only two values:  $0$  and  $π$   $(180^\circ)$ .

• When  $m ≤ 1$ , then  $ϕ(t) ≡ 0$  and envelope demodulation can be applied without distortion.
• When  $m > 1$ , a section of the locus curve lies in the left half-plane  ⇒   nonlinear distortions arise when envelope demodulation is applied.

Locus curves illustrating envelope demodulation

$\text{Example 3:}$   We assume that the source signal  $q(t)$  can take on all values in the range  $±1\ \rm V$ .

• Adding a DC component of  $A_{\rm T} = 2\ \rm V$  results in a DSB-AM with modulation depth  $m = 0.5$, whose locus curve can be seen in the left graph.
• $r_{\rm TP}(t)$  always lies in the right half-plane.  The pointer length changes with the message signal  $q(t)$.

The right-hand graph shows the locus curve for  $A_{\rm T} = 0.5 \ \rm V$   ⇒   $m = 2$.

•  $r_{\rm TP}(t)$  now takes on real values between $-0.5\ \rm V$  and  $1.5\ \rm V$ .
• The envelope demodulator cannot distinguish between positive and negative values   ⇒   nonlinear distortions occur.

The physical signals  $q(t)$,  $r(t)$ and  $v(t)$  corresponding to this example can be found in the graph on the previous page.

Special case of a cosine-shaped message signal

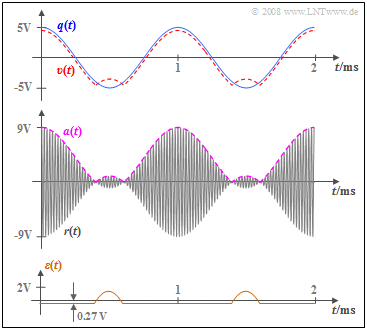
To quantitatively capture the nonlinear distortions due to a modulation depth  $m> 1$ , we assume the following scenario:

Error signal for envelope demodulation

• cosine-shaped source signal:   $q(t) = A_{\rm N} \cdot \cos(\omega_{\rm N} \cdot t);$
• DSB–AM with carrier:   $s(t) = \left ( q(t) + A_{\rm T} \right ) \hspace{-0.05cm}\cdot \hspace{-0.05cm}\cos(\omega_{\rm N} \hspace{-0.05cm}\cdot \hspace{-0.05cm}t),$   $r(t) = s(t);$
• modulation depth:   $m = A_{\rm N}/A_{\rm T} = 1.25;$
• ideal envelope demodulation:   $a(t) = {| q(t) + A_{\rm T}|} $
• elimination of the DC component by low-pass:   $r(t) = a(t) - {\rm E}\big[a(t)\big].$

The graph is valid for the signal parameters  $A_{\rm N} = 5 \ \rm V$,  $f_{\rm N} = 2 \ \rm kHz$,  $A_{\rm T} = 4 \ \rm V$  and  $f_{\rm T} = 100\ \rm kHz$.  It shows

• above, the sink signal  $v(t)$  compared to the source signal  $q(t)$,
• in the middle, the received signal  $r(t)$  as well as the envelope curve  $a(t) = {|r(t)|}$,
• below, the error signal  $ε(t) = v(t) \ – \ q(t)$  due to nonlinear distortions.

Based on the graphs, the following statements can be made:

• A comparison of the signals  $q(t)$  and  $a(t)$  reveals, that the envelope  $a(t)$  correctly reproduces the shape of the source signal  $q(t)$  around   $80\%$  of the time in this example.
• However, the maximum of  $a(t)$  is noticeably larger than the maximum of  $q(t)$ due to the added carrier  $A_{\rm T}$ .
• The sink signal  $v(t)$  differs from the envelope curve  $a(t)$  by the expected value  ${\rm Ε}\big[a(t)\big]$, which is removed by the low-pass of the envelope demodulator.
• Since  ${\rm Ε}\big[a(t)\big] = 4.27 \ \rm V$  does not match  $A_{\rm T} = 4 \ \rm V$ ,  $v(t)$  differs from  $q(t)$  by the constant value  $0.27 \ \rm V$, also in the regions where  $a(t)$  is correctly detected.
• The cosine source signal  $q(t)$  becomes a signal  $v(t)$  with harmonics:

$$v(t) = A_{\rm 1} \cdot \cos(\omega_{\rm N} t ) +A_{\rm 2} \cdot \cos(2\omega_{\rm N} t )+A_{\rm 3} \cdot \cos(3\omega_{\rm N} t )+ \text{...}$$

$$\Rightarrow \hspace{0.3cm}A_{\rm 1} = 4.48\,{\rm V},\hspace{0.3cm}A_{\rm 2} = 0.46\,{\rm V},\hspace{0.3cm}A_{\rm 3} = -0.37\,{\rm V},\hspace{0.3cm} A_{\rm 4} = 0.26\,{\rm V},\hspace{0.1cm}\text{...}$$

• Thus, according to the details in the book Linear and Time Invariant Systems, we obtain for the individual distortion factors as well as the total distortion factor:

$$K_2 ={|A_{\rm 2}|}/{A_{\rm 1}} = 0.102\hspace{0.3cm}K_3 ={|A_{\rm 3}|}/{A_{\rm 1}} = 0.082,\hspace{0.3cm}K_4 = {|A_{\rm 4}|}/{A_{\rm 1}} = 0.058,\hspace{0.1cm}\text{...}$$

$$\Rightarrow \hspace{0.3cm}K = \sqrt{K_2^2 + K_3^2 + K_4^2 +\text{...} } \approx 15 \%.$$

In the chapter  Quality Criteria  it was shown, that this also fixes the SNR at  $ρ_v = 1/K^2 ≈ 44$ .  $ρ_v$  $($but not  $K)$  can also be used as a quality criterion if  $q(t)$  contains more than one frequenczy   ⇒   derivation in the book im Buch  Linear and Time Invariant Systems.

Considering channel distortions

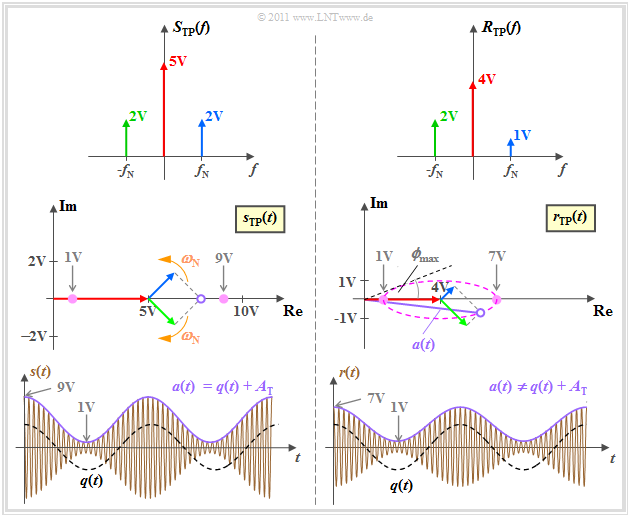
For the following considerations, we assume the modulation method "DSB-AM with carrier" as well as a cosine-shaped source signal $q(t)$ . Let the amplitudes of the message and carrier signals be  $A_{\rm N} = 4 \ \rm V$  and  $A_{\rm T} = 5 \ \rm V $  , respectively ⇒   modulation depth  $m = 0.8$. Thus (ideal) envelope demodulation is applicable in principle anwendbar.

Spectra and signals illustrating envelope demodulation

The graph shows, in descending order

• the spectra $S_{\rm TP}(f)$  and  $R_{\rm TP}(f)$  of the equivalent low-pass signals, which are assumed to be real,
• the equivalent low-pass signals  $s_{\rm TP}(t)$  and  $r_{\rm TP}(t)$  in the complex plane, and lastly
• the physical signals  $s(t)$  and  $r(t)$.

The left half of the image shows the transmitter and at the same time gives the conditions at the receiver for an ideal channel.

• Due to the modulation depth  $m ≤ 1$ , the source signal  $q(t)$ can be recognised in the envelope  $a(t)$ . Consequently, envelope demodulation is applicable without distortion under certain conditions, as shown in  $\text{Example 4}$ .

• The right half considers asymmetrical distortions through the channel. Here the carrier is attenuated by  $α_{\rm T} = 0.8$ , and the upper sideband by  $α_{\rm O} = 0.5$.  Now the envelope  $a(t) ≠ q(t) + A_{\rm T}$  is no longer cosine-shaped   ⇒   here envelope demodulation leads to nonlinear distortions as shown in  $\text{Example 5}$ .

Envelope demodulation with an ideal channel

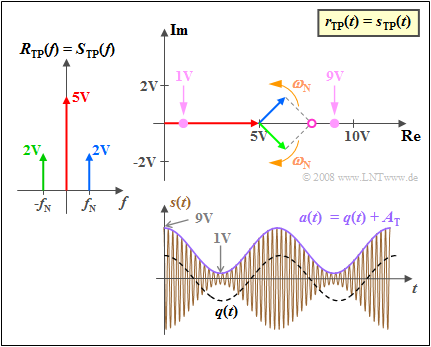
$\text{Example 4:}$   Let  $A_{\rm N} = 4 \ \rm V$  and  $A_{\rm T} = 5 \rm V$   continue to hold ⇒   modulation depth  $m = 0.8$.

The graph illustrates the use of an ideal envelope demodulator with an ideal channel, whereby the following identities are considered:

$$R_{\rm TP}(f) \hspace{-0.05cm}=\hspace{-0.05cm} S_{\rm TP}(f) \hspace{0.02cm}, \hspace{0.15cm} r_{\rm TP}(t) \hspace{-0.05cm}=\hspace{-0.05cm} s_{\rm TP}(t) \hspace{0.02cm}, \hspace{0.15cm} r(t) \hspace{-0.05cm}=\hspace{-0.05cm} s(t) \hspace{0.02cm}.$$

From these representations one can recognise:

• The red pointer of length  $A_{\rm T}$  depicting the carrier is fixed.
• The upper sideband (in blue) rotates in mathematically positive direction, the lower sideband (in green) in the opposite direction.
• Since the blue and green pointers both rotate with the same angular velocity  $ω_{\rm N}$  but in opposite directions, the vector sum of all pointers is always real.
• Should the modulation depth  $m ≤ 1$, then at all times  $r_{\rm TP}(t) ≥ 0$  and  $ϕ(t) = 0$. This means, that the zero crossings of the received signal  $r(t)$  exactly coincide with those of the carrier signal  $z(t)$ .
• The envelope $a(t)$  of the physical signal  $r(t)$  is equal to the resulting pointer length, i.e., equal tot he magnitude of  $r_{\rm TP}(t)$. Because  $m< 1$ ,  $a(t) = q(t) + A_{\rm T}$ holds.
• For the given amplitude values, the locus curve  $r_{\rm TP}(t)$  lies on the real axis between the end-points  $A_{\rm T} \ – \ A_{\rm N} = 1\ \rm V$  and  $A_{\rm T} + A_{\rm N} = 9 \ \rm V$.
• The locus curve on the real axis in the right half-plane is an indicator that the message signal can be extracted without distortion by an envelope demodulator.

Envelope demodulation with a distorting channel

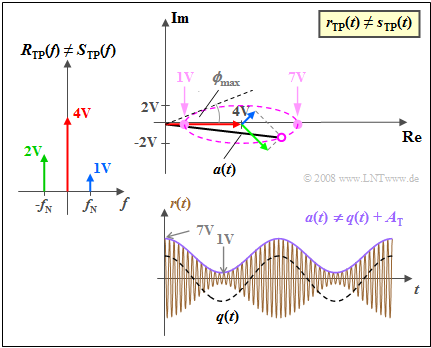
$\text{Example 5:}$   Now let us consider the same graphs for a distorting channel where

• $α_{\rm U} = 1$   ⇒   the lower sideband (LSB) is unchanged,
• $α_{\rm T} = 0.8$   ⇒   the carrier is slightly attenuated,
• $α_{\rm U} = 0.5$  ⇒   the upper sideband (USB) is attenuated more.

The graphs can then be interpreted as follows:

• Due to the different lengths of the green pointer (LSB) and blue pointer (USB), the locus curve becomes and ellipse, whose center is fixed by the (red) carrier.
• The angle between the complex-valued  $r_{\rm TP}(t)$  and the coordinate origin is now no longer continuous  $ϕ(t) = 0$, but instead fluctuates between  $±ϕ_{\rm max}.$
• The maximal phase is equal to the angle of the tangent to the ellipse. In the physical signal,  $ϕ(t) ≠ 0$  leads to shifts of the zero crossings of  $r(t)$  with respect to its nominal positions – as given by the carrier signal  $z(t)$.
• The magnitude  $a(t) = \vert r_{\rm TP}(t) \vert$ – i.e., the envelope of  $r(t)$ – is thus no longer cosine-shaped, and the signal after the envelope demodulator contains harmonicsin addition to the frequency  $f_{\rm N}$ :

$$v(t) = A_{\rm 1} \cdot \cos(\omega_{\rm N}\cdot t ) +A_{\rm 2} \cdot \cos(2\omega_{\rm N}\cdot t )+A_{\rm 3} \cdot \cos(3\omega_{\rm N} \cdot t )+ \text{...}$$

• These lead to nonlinear distortions and are captured by the distortion factor  $K$ .
  When  $A_{\rm N} = 4 \ \rm V$,  $A_{\rm T} = 5 \ \rm V$,  $α_{\rm U} = 1$  and  $α_{\rm T} = 0.8$ , the following numerical values are obtained as a function of  $α_{\rm O}$  :

$α_{\rm O} = 1.00$:     $K = 0$   ⇒   only the carrier is attenuated,

$α_{\rm O} = 0.75$:     $K ≈ 0.4\%$,

$α_{\rm O} = 0.50$:     $K ≈ 1.5\%$  ⇒   this value is used as a basis for the graph,

$α_{\rm O} = 0.25$:     $K ≈ 4\%$,

$α_{\rm O} = 0.00$:     $K ≈ 10\%$   ⇒   complete suppression of USB.

• This graph is valid for  $α_{\rm O} = 0.5$. , in which case we obtain (although difficult to see with the naked eye):

• shifts in the zero crossings of the signal  $r(t)$  by at most  $25^\circ\hspace{-0.05cm}/360^\circ ≈ 7\%$ of the carrier period $T_0$, and
• deviation from the ideal cosine shape   ⇒   $K ≈ 1.5\%$ (allerdings ist beides mit dem bloßen Auge nur schwer zu erkennen) .

Symmetrical channel distortions - attenuation distortions

An important result of the last section was that nonlinear distortions of the message signal occur in the case of asymmetric linear distortions in the channel. If, on the other hand, the lower sideband is attenuated in the same way as the upper sideband, the locus curve is again a horizontal straight line and no nonlinear distortions occur. Rather, the distortions with respect to  $q(t)$  and  $v(t)$ - as well as the distortions with respect to  $s(t)$  and  $r(t)$- are linear in this case, and can be corrected for by a suitably sized filter.

We now assume the following:

• A source signal  $q(t)$  – composed of two cosine components at the frequencies  $f_1$  and  $f_2$  with amplitudes  $A_1$  and  $A_2$, respectively;
• DSB–AM with carrier  ⇒   the transmit signal  $s(t)$  is composed of a total of five cosine oscillations at the frequencies  $f_{\rm T},  f_{\rm T} ± f_1$  and  $f_{\rm T} ± f_2$ 

Spectra in the equivalent lowpass region

• A channel with attenuation distortions, symmetrical about the carrier frequency:

$$H_{\rm K} (f = f_{\rm T})= \alpha_0, \hspace{0.3cm} H_{\rm K} (f = \pm f_{\rm 1})= \alpha_1, \hspace{0.3cm}H_{\rm K} (f = \pm f_{\rm 2})= \alpha_2;$$

• An ideal envelope demodulator as described in this section.

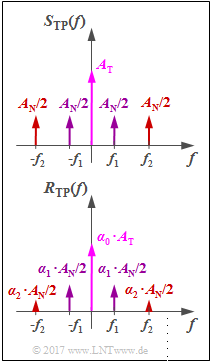
The graph shows the spectral function o the equivalent lowpass signals of the transmit signal and received signal. From this picture, the following statements can be made:

• The equivalent lowpass signal  $r_{\rm TP}(t)$  is real.  The locus curve – i.e., the peak of the pointer composite in the complex plane – again also lies on the real axis.
• Should  $α_0 · A_{\rm T}$  be greater than  $α_1 · A_1 + α_2 · A_2,$, then the "modulation depth of the received signal" is less than   $1$  and nonlinear distortions do not occur.
• The sink signal after ideal envelope demodulation and elimination of the DC component  $α_0 · A_{\rm T}$  by the downstream high-pass filter is:

$$v(t) = \alpha_1 \cdot A_{\rm 1} \cdot \cos(2 \pi f_{\rm 1} t ) + \alpha_2 \cdot A_{\rm 2} \cdot \cos(2 \pi f_{\rm 2} t ) \hspace{0.05cm}.$$

• This means:   Linear distortions (attenuation distortions) occur when  $α_2 ≠ α_1$ .  If the symmetry with respect to  $f_{\rm T}$  were not present, nonlinear distortions would arise.

We refer you to the video tutorial  Linear and nonlinear distortions.

Einfluss von Rauschstörungen

Sinkenstörabstand bei "ZSB-AM mit/ohne Träger" und Hüllkurvendemodulation

Ausgehend von der Systemkonfiguration

• ZSB–Amplitudenmodulation mit Modulationsgrad  $m ≤ 1$  sowie
• bestmöglich angepasste Hüllkurvendemodulation

wird nun der Einfluss von additivem Rauschen abgeschätzt.  Verzerrungen jeglicher Art – beispielsweise bedingt durch einen ungeeigneten Kanal oder die nichtperfekte Realisierung von Modulator und Demodulator – werden ausgeschlossen.

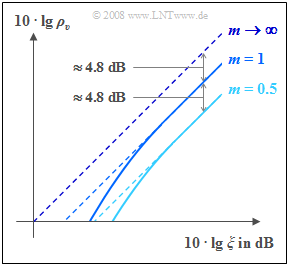
Die Grafik zeigt den Sinkenstörabstand  $10 · \lg \, ρ_v$  bei unterschiedlichem Modulationsgrad  $m$  in Abhängigkeit der logarithmierten Leistungskenngröße

$$10 \cdot {\rm lg }\hspace{0.1cm} \xi = 10 \cdot {\rm lg }\hspace{0.1cm} \frac{\alpha_{\rm K}^2 \cdot P_{\rm S}}{N_0 \cdot B_{\rm NF}} \hspace{0.05cm}.$$

Das Ergebnis des Hüllkurvendemodulators ist mit durchgezogenen Linien markiert, während die gestrichelten Geraden den Synchrondemodulator kennzeichnen.

Wie bereits in Kapitel  Untersuchungen im Hinblick auf Signalverzerrungen  hergeleitet wurde, ergeben sich für den Synchrondemodulator in dieser doppelt–logarithmischen Darstellung

• die Winkelhalbierende  (für  $m → ∞$, ZSB–AM ohne Träger), bzw.
• hierzu parallel verschobene Gerade mit vertikalem Abstand  $10 · \lg \, (1 + 2/m^2$).

Bei Anwendung von Hüllkurvendemodulation sind folgende Unterschiede festzustellen:

• Ein Hüllkurvendemodulator ist für  $m > 1$  nicht sinnvoll, da dadurch starke nichtlineare Verzerrungen entstehen würden.
• Die durchgehend gezeichneten Kurven für den Hüllkurvendemodulator  $\rm (HKD)$  liegen stets unterhalb der für den Synchrondemodulator  $\rm (SD)$  gültigen gestrichelten Geraden, wenn man vom gleichen Modulationsgrad  $m$  ausgeht.
• Ab einem gewissen Wert der Leistungskenngröße  $ξ$  sind die  $\rm HKD$–Kurven von den  $\rm SD$–Geraden innerhalb der Zeichengenauigkeit nicht mehr zu unterscheiden.

Mehr Informationen zu dieser Thematik finden Sie beispielsweise in  [Kam 04].

Argumente für und gegen den Hüllkurvendemodulator

Der wichtigste Grund für die Verwendung des Hüllkurvendemodulators ist  (besser gesagt:  war), dass damit die oft aufwändige Frequenz– und Phasensynchronisation vermieden wurde, so dass dieser preisgünstig realisiert werden konnte.  Der Hüllkurvendemodulator ist somit ein Beispiel eines inkohärenten Demodulators.

Für den  Synchrondemodulator  und gegen den Hüllkurvendemodulator können dagegen mehrere Gründe angeführt werden:

• Bei Hüllkurvendemodulation muss eine Übermodulation  $(m > 1)$  unter allen Umständen vermieden werden.  Dies erreicht man beispielsweise durch die Amplitudenbegrenzung des Quellensignals, was aber ebenfalls nichtlineare Verzerrungen zur Folge hat.
• Bei sonst gleichen Randbedingungen ist ein Modulationsgrad  $m < 1$  nur durch die Erhöhung der Sendeleistung um mindestens den Faktor  $3$  zu erreichen.  Dies ist auch wegen der berechtigten Diskussionen zum Thema „Elektro-Smog” in großen Teilen unserer Gesellschaft problematisch.
• Lineare Kanalverzerrungen können bei einem Hüllkurvendemodulator zu irreversiblen nichtlinearen Verzerrungen führen, während die bei Synchrondemodulation entstehenden linearen Verzerrungen möglicherweise durch besondere Maßnahmen beim Empfänger kompensiert werden können.

Aufgaben zum Kapitel

Aufgabe 2.7: Ist der Modulationsgrad zu groß?

Aufgabe 2.7Z: ZSB-AM und Hüllkurvendemodulator

Aufgabe 2.8: Unsymmetrischer Kanal

Aufgabe 2.9: Symmetrische Verzerrungen