Phase Modulation (PM)

1 # OVERVIEW OF THE THIRD MAIN CHAPTER #
2 Similarities between phase and frequency modulation
3 Signal characteristics of phase modulation
4 Equivalent low-pass signal in phase modulation
5 Interpretation des Besselspektrums
6 Spektralfunktion eines phasenmodulierten Sinussignals
7 Phasenmodulation der Summe zweier Sinusschwingungen
8 Aufgaben zum Kapitel
9 Quellenverzeichnis

# OVERVIEW OF THE THIRD MAIN CHAPTER #

$\Rightarrow \hspace{0.5cm}\text{We are just beginning the English translation of this chapter.}$

The third chapter describes angle modulation $\rm (WM)$ (from the German "Winkelmodulation") – this name is a generic term for phase modulation $\rm (PM)$ and frequency modulation $\rm (FM)$ – as well their the associated demodulators.

In detail, it covers:

the similarities and differences between phase and frequency modulation,
the signal characteristics and spectral functions of angle-modulated signals and the influence of band limiting,
the signal-to-noise power ratio of FM, which is more favorable than that of AM.

Similarities between phase and frequency modulation

It has already been pointed out in the chapter General Model of Modulation that there are substantial similarities between phase modulation $\rm (PM)$ and frequency modulation $\rm (FM)$ . Therefore, these two related modulation methods are summarized under the general term "angle modulation".

$\rm Definition\text{:}$ An angle modulation – abbreviated as $\rm WM$ – is present whenever the modulated signal can be represented as follows:

$$s(t) = A_{\rm T} \cdot \cos\big[\psi(t)\big] = A_{\rm T} \cdot \cos\hspace{-0.1cm}\big[ω_{\rm T} · t + ϕ(t)\big] \hspace{0.05cm}.$$

Here, as in amplitude modulation $A_{\rm T}$ denotes the amplitude of the carrier signal $z(t)$.
However, all the information about the source signal $q(t)$ is now captured by the angular function $ψ(t)$.

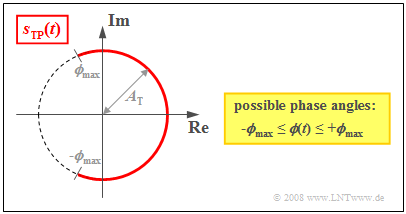
Equivalent low-pass signal in angle modulation

Based on the plot of the equivalent low-pass signal $s_{\rm TP}(t)$ ( subscript from the German "Tiefpass" ⇒ low-pass) on the complex plane (we will refer to such a plot as as a "locus curve") , the following charactistics of angle modulation can be seen:

The locus curve is an arc with radius $A_{\rm T}$. It follows that the envelope of an angle-modulated signal is always constant:

$$a(t) = |s_{\rm TP}(t)|= A_{\rm T}= {\rm const.}$$

The equivalent low-pass signal in angle modulation is always complex and determined by a time-dependent phase function $ϕ(t)$ (in radians), which determines the zero intercepts of $s(t)$ :

$$s_{\rm TP}(t)= A_{\rm T} \cdot {\rm e}^{\hspace{0.05cm}{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}\phi(t)}\hspace{0.05cm}.$$

For a symmetric source signal $q(t)$ , $ϕ(t)$ can take on all values between $±ϕ_{\rm max}$ , where $ϕ_{\rm max}$ indicates the phase deviation . The larger the phase deviation, the more intense the modulation.
For a harmonic oscillation, the phase deviation $ϕ_{\rm max}$ is equal to the modulation index $η$. Thus, the use of $η$ in what follows simultaneously indicates that $q(t)$ only contains a single frequency.
The relationship between the source signal $q(t)$ and the angular function $ψ(t) = \cos\hspace{-0.1cm}\big[ω_{\rm T} · t + ϕ(t)\big]$ like the phase function $ϕ(t)$ that can be derived from it, differs fundamentally in phase and frequency modulation, which will be discussed in detail in the chapter Frequency Modulation .

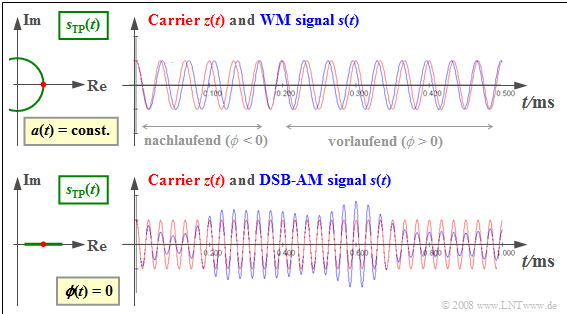
$\text{Example 1:}$ The following graph shows

the transmitted signal $s(t)$ on the right ⇒ blue waveforms compared to the carrier signal $z(t)$ ⇒ red oscillations,
as well as on the left, the equivalent low-pass signal $s_{\rm TP}(t)$ in the complex plane.

We also refer to the (left) plot in the complex plane as the "locus curve " ⇒ green waveforms.

Physikalisches Signal und äquivalentes Tiefpass–Signal bei Winkel- und Amplitudenmodulation

The upper graph applies to to angle modulation $\rm WM$:

The equivalent low-pass signal $s_{\rm TP}(t) = A_{\rm T} · {\rm e}^{ \hspace{0.05cm}{\rm j}\hspace{0.05cm}· \hspace{0.05cm}ϕ(t)}$ describes an arc ⇒ constant envelope $a(t) = A_{\rm T}$.
Thus, the information about the source signal $q(t)$ is exclusively in found in the zero intercepts of $s(t)$.
Should $ϕ(t) < 0$ hold, then the zero crossings of $s(t)$ occur later than those of $z(t)$ .
Otherwise – when $ϕ(t) > 0$ – the zero crossings of $s(t)$ come before $z(t)$&nbsp,.

The lower graph corresponds to Double-sideband Amplitude Modulation $\rm (DSB-AM)$ as described in Chapter 2 beschrieben, characterised bx

the time-dependent envelope $a(t)$ according to the source signal $q(t)$,
equidistant zero crossings of $s(t)$ according to the carrier $z(t)$, and
a horizontal straight line as the locus curve $s_{\rm TP}(t)$.

The present third chapter has been structured according to the following considerations:

Any FM system can be converted into a corresponding PM system by simple modifications and vice versa.
FM is more important for analog systems due to its more favorable noise behaviour. For this reason, considerations concerning the realization of the modulator/demodulator will only be dealt with in the chapter Frequency Modulation (FM).
Phase modulation (PM) is easier to understand compared to FM. Therefore, the basic porperties of an angle modulation system are first presented in this chapter using PM as an example.

Signal characteristics of phase modulation

Without limiting generality, the following assumes:

a cosine carrier signal $z(t) = A_{\rm T} · \cos(ω_{\rm T} · t)$, that is, the carrier phase is always $ϕ_{\rm T} = 0$,
a peak-limited source signal between the limits $\ –q_{\rm max} ≤ q(t) ≤ +q_{\rm max}$.

$\rm Definition\text{:}$ If the phase function $ϕ(t)$ is proportional to the applied source signal $q(t)$, we are dealing with phase modulation $\rm (PM)$, and it holds that:

$$\phi(t)= K_{\rm PM} \cdot q(t)\hspace{0.05cm}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}\psi(t)= \omega_{\rm T} \cdot t + \phi(t)\hspace{0.3cm}\Rightarrow \hspace{0.3cm}s(t) = A_{\rm T} \cdot \cos \big[\psi(t)\big]\hspace{0.05cm}.$$

Here, $K_{\rm PM}$ denotes the modulator constant with appropriate dimensions. If $q(t)$ describes a voltage waveform, this constant has the unit $\rm 1/V$.

The phase modulaton is all the more intensive,

the larger the modulator constant $K_{\rm PM}$ is, or
the larger the maximum value $q_{\rm max}$ of the source signal is.

Quantitatively, this fact is captured by the phase deviation

$$ \phi_{\rm max} = K_{\rm PM} \cdot q_{\rm max}\hspace{0.05cm}.$$

For a harmonic oscillation, the "phase deviation" is also called the modulation index and the following holds with a source signal amplitude apmplitude $A_{\rm N}$ :

$$\eta = \eta_{\rm PM} = K_{\rm PM} \cdot A_{\rm N}\hspace{0.05cm}.$$

The following should be noted about this equation:

The modulation index $η$ is comparable to the modulation depth $m$ in DSB–AM with carrier.
In the locus curve, $ϕ_{\rm max}$ or $η$ describe the half angle of the cricular arc in radians.
For other source signals with the same $η$ – for example: a different phase $ϕ_{\rm N}$ – the locus curve itself does not change, only the temporal movement along the curve does.
The modulation index is also used in describing frequency modulation, but then it is to be calculated somewhat differently.
We therefore distinguish between $η_{\rm PM}$ and $η_{\rm FM}$.

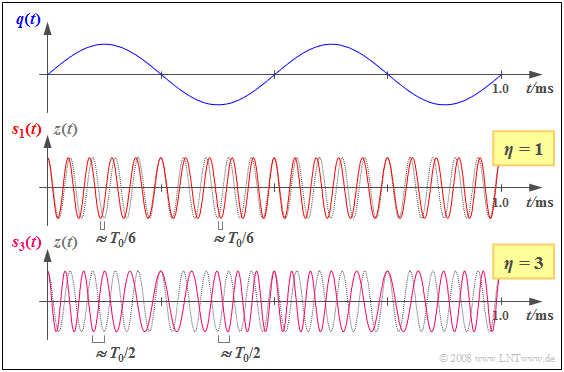
Signalverläufe bei Phasenmodulation mit $η = 1$ bzw. $η = 3$

$\rm Example \ 2\text{:}$ At the top, the graph shows a sinusoidal source signal $q(t)$ with frequency $f_{\rm N} = 2 \ \rm kHz$ and amplitude $A_{\rm N}$ , and the two phase modulated signals drawn below. These differ by the parameters $η = 1$ and $η = 3$, respectively:

$$s_\eta(t) = A_{\rm T} \cdot \cos \hspace{-0.1cm}\big[\omega_{\rm T} \cdot t + \eta \cdot \sin (\omega_{\rm N} \cdot t) \big]\hspace{0.05cm}.$$

Dotted in gray is the cosine carrier signal $z(t)$ , in each case based on $f_{\rm T} = 20 \ \rm kHz$ .

For example, the modulation index $η = 1$ and thus the transmitted signal $s_1(t)$ is given by

$A_{\rm N} = 1 \ \rm V $ and $K_{\rm PM} = \rm 1/V$,
but also by parameter values $A_{\rm N} = 2 \ \rm V$ and $K_{\rm PM} = \rm 0.5/V$.

From the curves, one can see:

The zero crossings of the transmitted signal $s_1(t)$ and the carrier signal $z(t)$ coincide exactly when $q(t) ≈ 0$ .
When $q(t) = +\hspace{-0.05cm}A_{\rm N}$ , the zero crossings of $s_1(t)$ come $1/(2π) ≈ 0.159$ of a carrier period $T_0$ earlier ("leading"), and when $q(t) = -\hspace{-0.05cm}A_{\rm N}$ , come the same period fraction later ("lagging").
If we increase the modulation index to $η = 3$ – either by tripling $A_{\rm N}$ or $K_{\rm PM}$, we get qualitatively the same result, but with a more intense phase modulation.
The zero crossings of $s_3(t)$ are now shifted relative to those of the clock signal by a maximum of $\rm ±3/(2π) ≈ ±0.5$ of a carrier period, i.e., up to $±T_0/2$.

Equivalent low-pass signal in phase modulation

Als Vorbereitung zur Herleitung des Spektrums $S(f)$ eines phasenmodulierten Signals $s(t)$ wird zunächst das äquivalente Tiefpass–Signal $s_{\rm TP}(t)$ analysiert. Dabei gehen wir von folgenden Voraussetzungen aus:

ein sinusförmiges Quellensignal mit Amplitude $A_{\rm N}$ und Frequenz $f_{\rm N}$,
ein cosinusförmiges Trägersignal mit Amplitude $A_{\rm T}$ und Frequenz $f_{\rm T}$,
eine Phasenmodulation mit dem Modulationsindex $η = K_{\rm PM} · A_{\rm N}$.

Damit lauten das phasenmodulierte Signal sowie das dazugehörige äquivalente Tiefpass–Signal:

$$s(t) = A_{\rm T} \cdot \cos \big[\omega_{\rm T} \cdot t + \eta \cdot \sin (\omega_{\rm N} \cdot t) \big]\hspace{0.05cm},$$

$$s_{\rm TP}(t) = A_{\rm T} \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin (\omega_{\rm N} \cdot t) }\hspace{0.05cm}.$$

Dieses Signal ist periodisch und kann somit durch eine komplexe Fourierreihe dargestellt werden. Damit erhält man allgemein:

$$s_{\rm TP}(t) = \sum_{n = - \infty}^{+\infty}D_{n} \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n\hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm}\cdot \hspace{0.05cm} t} \hspace{0.05cm}.$$

In dem hier betrachteten Sonderfall (sinusförmiges Quellensignal, cosinusförmiger Träger) sind die im Allgemeinen komplexen Fourierkoeffizienten $D_n$ alle reell und mit den Besselfunktionen ${\rm J}_n(η)$ erster Art und $n$–ter Ordnung wie folgt gegeben:

$$D_{n} = A_{\rm T}\cdot {\rm J}_n (\eta) \hspace{0.05cm}. \hspace{1cm} $$

$\text{Wichtiges Zwischenergebnis:}$ Nun soll mathematisch nachgewiesen werden, dass das äquivalente Tiefpass–Signal bei Phasenmodulation tatsächlich in die folgende Funktionsreihe umgewandelt werden kann:

$$s_{\rm TP}(t) = A_{\rm T} \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin (\omega_{\rm N} \cdot t) } \hspace{0.3cm}\Rightarrow \hspace{0.3cm} s_{\rm TP}(t) = A_{\rm T} \cdot \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n\hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm}\cdot \hspace{0.05cm} t}.$$

$\text{Beweis:}$ Wir setzen vereinfachend $A_{\rm T} = 1$. Damit lautet das gegebene äquivalente Tiefpass–Signal:

$$s_{\rm TP}(t) = {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin (\omega_{\rm N} \cdot t) }\hspace{0.05cm}.$$

(1) Mit $x = {\rm j} · η · \sin(γ)$ und $γ = ω_{\rm N} · t$ lautet die Potenzreihenentwicklung dieser Gleichung:

$$s_{\rm TP}(t) = {\rm e}^{x } = 1 + x + \frac{1}{2!} \cdot x^2 + \frac{1}{3!} \cdot x^3 + \text{...} = 1 + {\rm j} \cdot \eta \cdot \sin (\gamma)+ \frac{1}{2!} \cdot {\rm j}^2 \cdot \eta^2 \cdot \sin^2 (\gamma)+ \frac{1}{3!} \cdot {\rm j}^3 \cdot \eta^3 \cdot \sin^3 (\gamma) + \text{...}$$

(2) Die einzelnen trigonometrischen Ausdrücke können wie folgt umgeformt werden:

$$ \frac{1}{2!} \cdot {\rm j}^2 \cdot \eta^2 \cdot \sin^2 (\gamma) = \frac{- \eta^2}{2 \cdot 2!} \cdot \big[ 1 - \cos (2\gamma)\big],\hspace{1.0cm} \frac{1}{3!} \cdot {\rm j}^3 \cdot \eta^3 \cdot \sin^3 (\gamma) = \frac{- {\rm j} \cdot \eta^3}{4 \cdot 3!} \cdot \big[ 3 \cdot \sin (\gamma)- \sin (3\gamma)\big],$$

$$ \frac{1}{4!} \cdot {\rm j}^4 \cdot \eta^4 \cdot \sin^4 (\gamma) = \frac{\eta^4}{8 \cdot 4!} \cdot \left[ 3+ 4 \cdot \cos (2\gamma)+ \cos (4\gamma)\right], \text{...} $$

(3) Durch Umordnen erhält man mit ${\rm J}_n(η)$, den Besselfunktionen erster Art und $n$–ter Ordnung:

$$s_{\rm TP}(t) = 1 \cdot {\rm J}_0 (\eta) + 2 \cdot {\rm j}\cdot {\rm J}_1 (\eta)\cdot \sin (\gamma) \hspace{0.2cm} + 2 \cdot {\rm J}_2 (\eta)\cdot \cos (2\gamma) + 2 \cdot {\rm j}\cdot {\rm J}_3 (\eta)\cdot \sin (3\gamma)+ 2 \cdot {\rm J}_4 (\eta)\cdot \cos (4\gamma) + \text{...} $$

(4) Mit dem Satz von Euler kann hierfür auch geschrieben werden:

$$s_{\rm TP}(t) = {\rm J}_0 (\eta) + \big[ {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} \gamma} - {\rm e}^{\hspace{0.05cm}{ - \rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} \gamma} \big]\cdot {\rm J}_1 (\eta) \hspace{0.27cm} +\left[ {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 2\gamma} + {\rm e}^{\hspace{0.05cm}{ - \rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 2\gamma} \right]\cdot {\rm J}_2 (\eta)+ \left[ {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 3\gamma} - {\rm e}^{\hspace{0.05cm}{ - \rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 3\gamma} \right]\cdot {\rm J}_3 (\eta)+ \left[ {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 4\gamma} + {\rm e}^{\hspace{0.05cm}{ - \rm j} \hspace{0.05cm}\cdot \hspace{0.05cm} 4\gamma} \right]\cdot {\rm J}_4 (\eta)+\text{...}$$

(5) Die Besselfunktionen zeigen folgende Symmetrieeigenschaften:

$${\rm J}_{-n} (\eta) = ( - 1)^n \cdot {\rm J}_{n} (\eta)\hspace{0.3cm} \Rightarrow \hspace{0.3cm} {\rm J}_{ - 1} (\eta) = - {\rm J}_{1} (\eta),\hspace{0.3cm}{\rm J}_{ - 2} (\eta) = {\rm J}_{2} (\eta),\hspace{0.3cm}{\rm J}_{ - 3} (\eta) = - {\rm J}_{3} (\eta),\hspace{0.3cm}{\rm J}_{ - 4} (\eta) = {\rm J}_{4} (\eta).$$

(6) Berücksichtigt man diesen Sachverhalt und den bisher weggelassenen Faktor $A_{\rm T}$, so erhält man das gewünschte Ergebnis:

$$s_{\rm TP}(t) = A_{\rm T} \cdot \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n\hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm}\cdot \hspace{0.05cm} t}.$$

$\text{q.e.d.}$

Zur Berechnung der Besselfunktionen

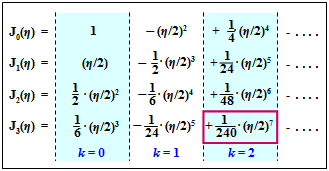
Diese bereits 1844 von Friedrich Wilhelm Bessel eingeführten mathematischen Funktionen sind wie folgt definiert (erste Gleichung) und können gemäß der zweiten Gleichung durch eine Reihe angenähert werden:

$${\rm J}_n (\eta) = \frac{1}{2\pi}\cdot \int_{-\pi}^{+\pi} {{\rm e}^{\hspace{0.05cm}{\rm j}\hspace{0.05cm}\cdot \hspace{0.05cm}(\eta \hspace{0.05cm}\cdot \hspace{0.05cm}\sin(\alpha) -\hspace{0.05cm} n \hspace{0.05cm}\cdot \hspace{0.05cm}\alpha)}}\hspace{0.1cm}{\rm d}\alpha\hspace{0.05cm},$$

$${\rm J}_n (\eta) = \sum\limits_{k=0}^{\infty}\frac{(-1)^k \cdot (\eta/2)^{n \hspace{0.05cm} + \hspace{0.05cm} 2 \hspace{0.02cm}\cdot \hspace{0.05cm}k}}{k! \cdot (n+k)!} \hspace{0.05cm}.$$

Nebenstehende Grafik zeigt die jeweils ersten drei Summanden $(k = 0,\ 1,\ 2)$ der Reihen ${\rm J}_0(η)$, ... , ${\rm J}_3(η).$ Der rot umrandete Term – gültig für $n = 3$ und $k = 2$ – lautet beispielsweise in ausgeschriebener Form:

$$\frac{(-1)^2 \cdot (\eta/2)^{3 \hspace{0.05cm} + \hspace{0.05cm} 2 \hspace{0.02cm}\cdot \hspace{0.05cm}2}}{2\hspace{0.05cm}! \cdot (3+2)\hspace{0.05cm}!} = \frac{1}{240}\cdot (\frac{\eta}{2})^7 \hspace{0.05cm}.$$

Die Besselfunktionen $J_n(η)$ findet man aber auch in Formelsammlungen oder mit dem von uns bereitgestellten Berechnungsmodul Besselfunktionen erster Art.
Sind die Funktionswerte für $n = 0$ und $n = 1$ bekannt, so können daraus die Besselfunktionen für $n ≥ 2$ iterativ ermittelt werden:

$${\rm J}_n (\eta) ={2 \cdot (n-1)}/{\eta} \cdot {\rm J}_{n-1} (\eta) - {\rm J}_{n-2} (\eta) \hspace{0.05cm}.$$

Interpretation des Besselspektrums

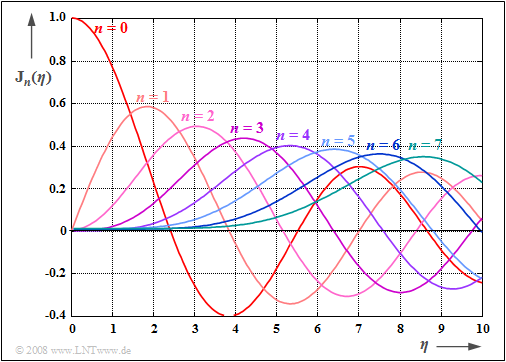
Die Grafik zeigt die Besselfunktionen ${\rm J}_0(η)$, ... , ${\rm J}_7(η)$ abhängig vom Modulationsindex $η$ im Bereich $0 ≤ η ≤ 10$.

Besselfunktionen erster Art und $n$–ter Ordnung

Man findet diese auch in Formelsammlungen wie [BS01]^[1] in tabellarischer Form.

Die erste Art wird durch das $\rm J$ ausgedrückt,
die Ordnung durch den Index $n$.

Anhand dieser Grafik können für das äquivalente Tiefpass-Signal

$$s_{\rm TP}(t) = A_{\rm T} \cdot \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n\hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm}\cdot \hspace{0.05cm} t}$$

folgende Eigenschaften abgeleitet werden:

Das äquivalente Tiefpass–Signal setzt sich aus einem ruhenden Zeiger $(n = 0)$ sowie unendlich vielen im Uhrzeigersinn $(n < 0)$ bzw. entgegen dem Uhrzeigersinn $(n > 0)$ drehenden Zeigern zusammen.

Die Zeigerlängen hängen über die Besselfunktionen ${\rm J}_n(η)$ vom Modulationsindex $η$ ab. Je kleiner $η$ ist, um so mehr Zeiger können allerdings für die Konstruktion von $s_{\rm TP}(t)$ vernachlässigt werden.

Für den Modulationsindex $η = 1$ gilt beispielsweise folgende Näherung:

$$s_{\rm TP}(t) = {\rm J}_0 (1) + {\rm J}_1 (1)\cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm} t}+ {\rm J}_2 (1)\cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}2 \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm} t}+ {\rm J}_3 (1)\cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}3 \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm} t}- {\rm J}_1 (1)\cdot {\rm e}^{-{\rm j} \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm} t}+ {\rm J}_2 (1)\cdot {\rm e}^{-{\rm j} \hspace{0.05cm}2 \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm} t}- {\rm J}_3 (1)\cdot {\rm e}^{-{\rm j} \hspace{0.05cm}3 \hspace{0.05cm}\omega_{\rm N} \hspace{0.05cm} t}\hspace{0.05cm}.$$

Hierbei ist die Symmetriebeziehung ${\rm J}_{–n}(η) = (–1)^n · {\rm J}_n(η)$ berücksichtigt. Es gilt also:

$${\rm J}_{-1}(\eta) = - {\rm J}_{1}(\eta), \hspace{0.3cm}{\rm J}_{-2}(\eta) = {\rm J}_{2}(\eta), \hspace{0.3cm}{\rm J}_{-3}(\eta) = - {\rm J}_{3}(\eta).$$

Weiter erkennt man aus obiger Gleichung, dass sich $s_{\rm TP}(t)$ mit $η = 3$ aus deutlich mehr Zeigern zusammensetzt, nämlich denen mit den Indizes ${\rm J}_{–6}(\eta)$, ... , ${\rm J}_{+6}(\eta)$.

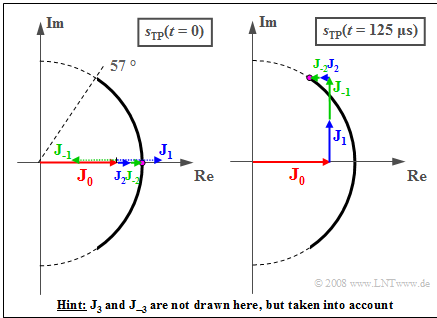
Äquivalentes Tiefpass–Signal bei Phasenmodulation

$\rm Beispiel\ 3\text{:}$ Die Besselfunktionen liefern für den Modulationsindex $η = 1$ folgende Werte:

$${\rm J}_0 = 0.765,\hspace{0.3cm}{\rm J}_1 = - {\rm J}_{ - 1} = 0.440, \hspace{0.3cm}{\rm J}_2 = {\rm J}_{ - 2} = 0.115,\hspace{0.3cm}{\rm J}_3 = - {\rm J}_{ - 3} = 0.020\hspace{0.05cm}.$$

Die Grafik zeigt die Zusammensetzung der Ortskurve aus den sieben Zeigern. Anmerkung:
${\rm J}_3$ und ${\rm J}_{ - 3}$ fehlen in der Skizze, aber nicht im Gesamtsignal.

Die Frequenz des sinusförmigen Quellensignals ist $f_{\rm N} = 2 \ \rm kHz$, woraus sich die Periodendauer $T_{\rm N} = 1/f_{\rm N} = 500 \ \rm µ s$ ergibt. Vereinfachend wird $A_{\rm T} = 1$ gesetzt.

Das linke Bild zeigt die Momentaufnahme zur Zeit $t = 0$.

Wegen ${\rm J}_1 = – {\rm J}_{ – 1}$ und ${\rm J}_3 = – {\rm J}_{ – 3}$ gilt hierfür:

$$s_{\rm TP}(t = 0) = {\rm J}_0 + {\rm J}_{2} + {\rm J}_{ - 2} = 0.765 + 2 \cdot 0.115 = 0.995 \hspace{0.05cm}.$$

Aus dem reellen Ergebnis folgt die Phase ${\mathbf ϕ}(t = 0) = 0$ und der Betrag $a(t = 0) = 1$.
Der geringfügig abweichende Wert $0.995$ zeigt, dass ${\rm J}_4 = {\rm J}_{ – 4}$ zwar klein ist $(≈ 0.002)$, aber nicht identisch Null.

Das rechte Bild zeigt die Verhältnisse zur Zeit $t = T_{\rm N}/4 = 125\ \rm µ s$:

Die Zeiger mit den Längen ${\rm J}_{– 1}$ und ${\rm J}_1$ haben sich im bzw. entgegen dem Uhrzeigersinn um $90^\circ$ gedreht und zeigen nun beide in Richtung der imaginären Achse.
Die Zeiger ${\rm J}_2$ und ${\rm J}_{– 2}$ drehen doppelt so schnell wie ${\rm J}_1$ bzw. ${\rm J}_{– 1}$ und zeigen nun beide in Richtung der negativen reellen Achse.
${\rm J}_3$ und ${\rm J}_{– 3}$ drehen im Vergleich zu ${\rm J}_1$ und ${\rm J}_{– 1}$ mit dreifacher Geschwindigkeit und zeigen jetzt beide nach unten.

Damit erhält man:

$$s_{\rm TP}(t = 125\,{\rm µ s}) = {\rm J}_0 - 2 \cdot {\rm J}_{2} + {\rm j} \cdot (2 \cdot {\rm J}_{1} - 2 \cdot {\rm J}_{3})= 0.535 + {\rm j} \cdot 0.840 $$

$$ \Rightarrow \hspace{0.3cm} a(t = 125\,{\rm µ s}) = \sqrt{0.535^2 + 0.840^2}= 0.996\hspace{0.05cm},$$

$$ \Rightarrow \hspace{0.3cm}\phi(t = 125\,{\rm µ s}) = \arctan \frac{0.840}{0.535} = 57.5^\circ \approx 1\,{\rm rad}\hspace{0.05cm}.$$

Auch zu allen anderen Zeiten ergibt die vektorielle Summe der sieben Zeiger jeweils einen Punkt auf dem Kreisbogen mit Winkel $ϕ(t)$, wobei $\vert ϕ(t) \vert ≤ η = 1\ \rm rad $ gilt.

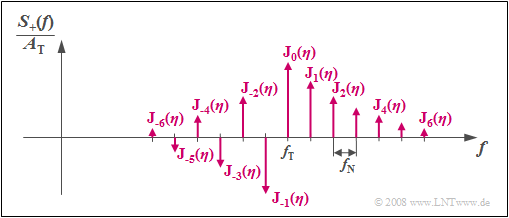
Spektralfunktion eines phasenmodulierten Sinussignals

$\text{Ohne Beweis:}$

Ausgehend vom eben berechneten äquivalenten Tiefpass–Signal erhält man für das analytische Signal:

$$s_{\rm +}(t) = s_{\rm TP}(t) \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm T} \hspace{0.05cm}\cdot \hspace{0.05cm} t}= A_{\rm T} \cdot \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}(\omega_{\rm T}\hspace{0.05cm}+\hspace{0.05cm} n\hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm N}) \hspace{0.05cm}\cdot \hspace{0.05cm} t}$$

Durch Fouriertransformation ergibt sich für das Spektrum des analytischen Signals:

$$S_{\rm +}(f) = A_{\rm T} \cdot \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot \delta \big[f - (f_{\rm T}+ n \cdot f_{\rm N})\big]\hspace{0.05cm}.$$

Das Spektrum des physikalischen Signals erhält man durch Ausweitung auf negative Frequenzen unter Berücksichtigung des Faktors $1/2$:

$$S(f) = \frac{A_{\rm T} }{2} \cdot \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta) \cdot \delta \big[f \pm (f_{\rm T}+ n \cdot f_{\rm N})\big]\hspace{0.05cm}.$$

Spektrum des analytischen Signals bei PM (gültig auch für FM)

Anhand der Grafik sind folgende Aussagen möglich:

Das Spektrum $S_+(f)$ eines phasenmodulierten Sinussignals besteht aus unendlich vielen diskreten Linien im Abstand der Nachrichtenfrequenz $f_{\rm N}$. Es ist somit prinzipiell unendlich weit ausgedehnt.
Die Höhen (Gewichte) der Spektrallinien bei $f_{\rm T} + n · f_{\rm N}$ (wobei $n$ ganzzahlig ist) sind durch den Modulationsindex $η$ über die Besselfunktionen ${\rm J}_n(η)$ festgelegt.
Die Werte der Besselfunktionen ${\rm J}_n(η)$ zeigen, dass man in der Praxis durch Bandbegrenzung das Spektrum nur wenig verändert. Der daraus resultierende Fehler wächst aber mit steigendem $η$.
Die Spektrallinien sind bei sinusförmigem Quellensignal und cosinusförmigem Träger reell und für gerades $n$ symmetrisch um $f_{\rm T}$. Bei ungeradem $n$ ist ein Vorzeichenwechsel zu berücksichtigen.
Die Phasenmodulation einer Schwingung mit anderer Phase von Quellen– und/oder Trägersignal liefert das gleiche Betragsspektrum und unterscheidet sich nur bezüglich der Phasenfunktion.

Setzt sich das Nachrichtensignal aus mehreren Schwingungen zusammen, so ist die Berechnung des Spektrums schwierig, nämlich:

Faltung der Einzelspektren (siehe nächster Abschnitt und Aufgabe 3.3).

Phasenmodulation der Summe zweier Sinusschwingungen

Setzt sich das Quellensignal aus der Summe zweier Sinusschwingungen zusammen, so lauten die Signale am Ausgang des Phasenmodulators:

$$s(t) = A_{\rm T} \cdot \cos \big[\omega_{\rm T} \cdot t + \eta_1 \cdot \sin (\omega_{\rm 1} \cdot t) + \eta_2 \cdot \sin(\omega_{\rm 2} \cdot t)\big]\hspace{0.05cm},$$

$$s_{\rm TP}(t) = A_{\rm T} \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot [\hspace{0.05cm}\eta_1 \hspace{0.05cm}\cdot \hspace{0.08cm}\sin (\omega_{\rm 1} \hspace{0.05cm}\cdot \hspace{0.05cm} t) \hspace{0.05cm}+ \hspace{0.05cm}\eta_2 \hspace{0.05cm}\cdot \hspace{0.08cm}\sin (\omega_{\rm 2} \hspace{0.05cm}\cdot \hspace{0.05cm}t)]}\hspace{0.05cm}.$$

Zur einfacheren Darstellung setzten wir nun $A_{\rm T} = 1$ und erhalten:

$$s_{\rm TP}(t) = {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta_1 \hspace{0.05cm}\cdot \hspace{0.08cm}\sin (\omega_{\rm 1} \hspace{0.05cm}\cdot \hspace{0.05cm} t) } \cdot {\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta_2 \hspace{0.05cm}\cdot \hspace{0.08cm}\sin (\omega_{\rm 2} \hspace{0.05cm}\cdot \hspace{0.05cm} t) }\hspace{0.05cm}.$$

Die Spektralfunktionen der beiden Terme lauten:

$${\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta_1 \hspace{0.05cm}\cdot \hspace{0.08cm}\sin (2 \pi \hspace{0.05cm}\cdot \hspace{0.05cm}f_{\rm 1} \hspace{0.01cm}\cdot \hspace{0.05cm} t) } \hspace{0.2cm}\circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\, \hspace{0.2cm} B_1(f) = \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta_1) \cdot \delta (f - n \cdot f_{\rm 1})\hspace{0.05cm},$$

$${\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}\eta_2 \hspace{0.05cm}\cdot \hspace{0.08cm}\sin (2 \pi \hspace{0.05cm}\cdot \hspace{0.05cm}f_{\rm 2} \hspace{0.01cm}\cdot \hspace{0.05cm} t) } \hspace{0.2cm}\circ\!\!-\!\!\!-\!\!\!-\!\!\bullet\, \hspace{0.2cm} B_2(f) = \sum_{n = - \infty}^{+\infty}{\rm J}_n (\eta_2) \cdot \delta (f - n \cdot f_{\rm 2})\hspace{0.05cm}.$$

Die Besselfunktionen $B_1(f)$ und $B_2(f)$ beschreiben Linienspektren im Frequenzabstand $f_1$ und $f_2$, deren Impulsgewichte durch $η_1$ und $η_2$ bestimmt sind.
Aufgrund der Multiplikation im Zeitbereich ergibt sich für die Spektralfunktion die Faltung:

$$S_{\rm TP}(f) = B_1(f) \star B_2(f)= S_{\rm +}(f + f_{\rm T}) \hspace{0.05cm}.$$

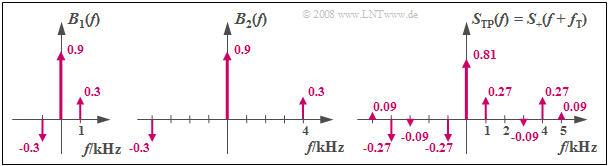
$\rm Beispiel\ 4\text{:}$ Die linke Grafik zeigt die Besselfunktion $B_1(f)$ für $η_1 = 0.64$ und $f_1 = 1 \ \rm kHz$.

Äquivalentes Tiefpass-Spektrum als Faltung zweier Besselspektren

Auf die deutlich kleineren Linien bei $f = ±2 \ \rm kHz$ mit den Gewichten $0.05$ ist der Übersichtlichkeit halber verzichtet.
Die Funktion $B_2(f)$ gilt für den gleichen Modulationsindex $η_2 = η_1$, aber für die Nachrichtenfrequenz $f_2 = 4 \ \rm kHz$.
Das Tiefpass-Spektrum $S_{\rm TP}(f) = B_1(f) \star B_2(f)$ besteht hier aus neun Diraclinien. Es ist im rechten Diagramm skizziert.
Durch Frequenzverschiebung um $f_{\rm T}$ nach rechts erhält man das Spektrum $S_+(f)$ des analytischen Signals $s_+(t)$.
Es gilt also $S_+(f = f_{\rm T}) = S_{\rm TP}(f = 0) = 0.81$.