Frequency Modulation (FM)

1 Instantaneous frequency
2 Signal characteristics with frequency modulation
3 Frequency modulation of a cosine signal
4 WM spectrum of a harmonic oscillation
5 Influence of band-limiting in angle modulation
6 Realisierung eines FM–Modulators
7 PLL–Realisierung eines FM–Demodulators
8 Aufgaben zum Kapitel
9 Quellenverzeichnis

Instantaneous frequency

We will once again assume an angle-modulated signal:

$$s(t) = A_{\rm T} \cdot \cos \big[\psi(t)\big].$$

All the information about the source signal $q(t)$

is thus exclusively captured by the angular function $ψ(t)$ ,
while the envelope $a(t) = A_{\rm T}$ is constant.

$\text{Definitions:}$

The instantaneous angular frequency is the derivative of the angular function with respect to time:

$$\omega_{\rm A}(t) = \frac{ {\rm d}\psi(t)}{ {\rm d}t}\hspace{0.05cm}.$$

Accordingly, the instantaneous frequency is described by:

$$f_{\rm A}(t) = \frac{\omega_{\rm A}(t)}{2\pi} = \frac{1}{2\pi} \cdot \frac{ {\rm d}\hspace{0.03cm}\psi(t)}{ {\rm d}t}\hspace{0.05cm}.$$

The frequency deviation is the maximum deviation $Δf_{\rm A}$ of the time-dependent instantaneous frequency $f_{\rm A}(t)$ from the constant carrier frequency $f_{\rm T}$.

For an angle modulation with carrier frequency $f_{\rm T}$ , the instantaneous frequency oscillates in the range

$$f_{\rm T} - \Delta f_{\rm A} \le f_{\rm A}(t) \le f_{\rm T} + \Delta f_{\rm A}\hspace{0.05cm}.$$

It should be emphasized that there is a fundamental difference between the instantaneous frequency $f_{\rm A}(t)$ and the spectrum $S(f)$ of an angle-modulated signal $s(t)$ that can be measured with a spectrum analyzer, as illustrated by the following example.

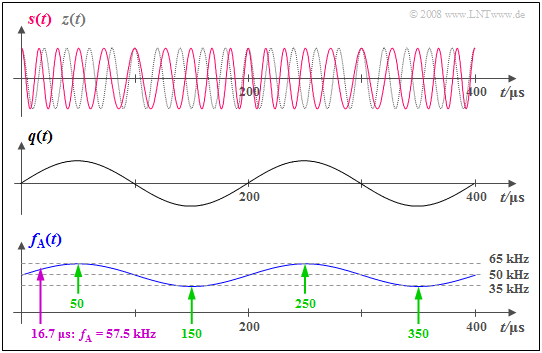
$\text{Example 1:}$ The graph shows the phase-modulated signal

$$s(t) = A_{\rm T} \cdot \cos \hspace{-0.1cm} \big[\psi(t)\big] = A_{\rm T} \cdot \cos \hspace{-0.1cm} \big[2 \pi f_{\rm T} \hspace{0.05cm}t + \eta \cdot \sin (2 \pi f_{\rm N} \hspace{0.05cm} t)\big]$$

as well as the instantaneous frequency plotted beneath

$$f_{\rm A}(t) = \frac{1}{2 \pi} \cdot \frac{ {\rm d}\hspace{0.03cm}\psi(t)}{ {\rm d}t} = f_{\rm T} + \Delta f_{\rm A}\cdot \cos (2 \pi f_{\rm N} \hspace{0.05cm} t) \hspace{0.05cm}.$$

Illustration of instantaneous frequency

Here, the system parameters are:

$f_{\rm T} = 50 \ \rm kHz$,
$f_{\rm N} = 5 \ \rm kHz$,
$η = 3$.

From this, we derive the frequency deviation

$Δf_{\rm A} = η · f_{\rm N} = 15 \ \rm kHz$.

In the middle, the qualitative progression of the sinusoidal source signal $q(t)$ is sketched for reference.
From these graphs, one can see:

The instantaneous frequency $f_{\rm A}(t)$ can take on arbitrary values between $f_{\rm T} + Δf_{\rm A} = 65 \ \rm kHz$ $($at $t = 50\ \rm µ s,\ 250 \ µ s$, etc.$)$ and $f_{\rm T} \ – Δf_{\rm A} = 35 \ \rm kHz$ $($at $t = 150\ \rm µ s, \ 350 \ µ s$, etc.$)$ (see green marks).
At time $t ≈ 16.7 \ \rm µ s$ , for example, $f_{\rm A}(t) = 57.5 \ \rm kHz$ holds (purple arrow).
In contrast, the spectral function $S(f)$ consists of discrete Bessel lines at the frequencies ... , $30,\ 35,\ 40,\ 45,\ \mathbf{50},\ 55,\ 60,\ 65,\ 70$, ... $($each in $\rm kHz)$.
There is no spectral line at $f = 57.5 \ \rm kHz$ , though there is one at $f = 70 \ \rm kHz$.
In contrast, at no time does $f_{\rm A}(t) = 70 \ \rm kHz$ hold.

$\text{Therefore:}$

The instantaneous frequency $f_{\rm A}(t)$ is thus not a physically measurable frequency in the conventional sense, but only an abstract mathematical quantity, namely the derivative of the angular function $ψ(t)$.

Signal characteristics with frequency modulation

As in the chapter Phase Modulation we will assume that the carrier signal $z(t)$ has a cosine waveform and the source signal $q(t)$ is peak-limited.

$\text{Definition:}$ In a communication system, if the instantaneous angular frequency $ω_{\rm A}(t)$ is linearly dependent on the instantaneous value of the source signal $q(t)$, we call this frequency modulation $\rm (FM)$:

$$\omega_{\rm A}(t) = 2 \pi \cdot f_{\rm A}(t) = \omega_{\rm T} + K_{\rm FM} \cdot q(t) \hspace{0.05cm}.$$

Here, $K_{\rm FM}$ denotes a dimensionally restricted constant. If $q(t)$ represents a voltage waveform, $K_{\rm FM}$ has the unit $\rm V^{–1}s^{–1}$.

In frequency modulation, the angular function $\psi(t)$ and the modulated signal $s(t)$ are given by:

$$\psi(t) = \omega_{\rm T} \cdot t + K_{\rm FM} \cdot \int q(t)\hspace{0.1cm}{\rm d}t \hspace{0.05cm}\hspace{0.3cm}\Rightarrow \hspace{0.3cm}s(t) = A_{\rm T} \cdot \cos \big[\psi(t)\big] \hspace{0.05cm}.$$

From this equation, we can immediately see:

Even in the case of frequency modulation, the equivalent low-pass signal moves on an arc because of the constant envelope ⇒ $a(t) = A_{\rm T}$ .
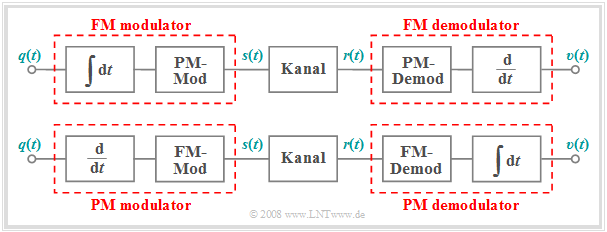
A frequency modulator can be implemented using an integrator and a phase modulator. Consequently, the FM demodulator consists of a PM demodulator and a differentiator, as shown in the upper part of the following diagram.

Relationship between phase and frequency modulation

The lower graph shows the inverse relationship ⇒ a possible description of a PM modulator and demodulator in terms of corresponding FM components.
It can also be seen from the above equation, that the equation encountered earlier on page A very simple (though unfortunately not always correct) modulator equation in the first chapter of this book, will only hold for frequency modulation in very special cases.

In frequency modulation, the conversion

$$s(t) = a(t) \cdot \cos (\omega (t) \cdot t + \phi(t)) \hspace{0.3cm}\Rightarrow \hspace{0.3cm} s(t) = A_{\rm T} \cdot \cos (\omega (t) \cdot t + \phi_{\rm T})$$

ist only allowed sometimes, such as in the nonlinear digital modulation method "Frequency Shift Keying" $\rm (FSK)$ with a right-angled fundamental pulse.

Frequency modulation of a cosine signal

For a cosine source signal $q(t)$ with frequency modulation, the instantaneous angular frequency is:

$$q(t) = A_{\rm N} \cdot \cos (\omega_{\rm N} \cdot t)\hspace{0.3cm}\Rightarrow \hspace{0.3cm} \omega_{\rm A}(t) = \omega_{\rm T} + K_{\rm FM} \cdot A_{\rm N} \cdot \cos(\omega_{\rm N} \cdot t)\hspace{0.05cm}.$$

Integrating this over time, we get the angular function:

$$\psi(t) = \omega_{\rm T} \cdot t + \frac {K_{\rm FM} \cdot A_{\rm N}}{\omega_{\rm N}} \cdot \sin (\omega_{\rm N} \cdot t) \hspace{0.05cm}.$$

A comparison with the propositions in the chapter Phase Modulation makes clear:

The frequency modulation of a cosine signal yields a qualitatively identical transmitted signal $s(t)$ as the phase modulation of a sinusoidal source signal $q(t)$.
However, this requires that the modulator constants are matched according to the ratio $K_{\rm FM}/K_{\rm PM} = ω_{\rm N}$ .
The transmitted signal $s(t)$ can thus be described uniformly for the two configurations "PM – sine signal" as well as "FM – cosine signal":

$$s(t) = A_{\rm T} \cdot \cos \hspace{-0.1cm} \big[\omega_{\rm T} \cdot t + \eta \cdot \sin (\omega_{\rm N} \cdot t)\big] \hspace{0.05cm}.$$

However, when using this equation for the modulation index $η$ different equations must be use for phase and frequency modulation:

$$\eta_{\rm PM} = {K_{\rm PM} \cdot A_{\rm N}},$$

$$\eta_{\rm FM} = \frac {K_{\rm FM} \cdot A_{\rm N}}{\omega_{\rm N}} \hspace{0.05cm}.$$

If the source signal is not a harmonic oscillation, but is instead composed of several frequencies, the time signals also differ qualitatively for phase and frequency modulation. This can be seen in the earlier comparison of PSK and FSK.

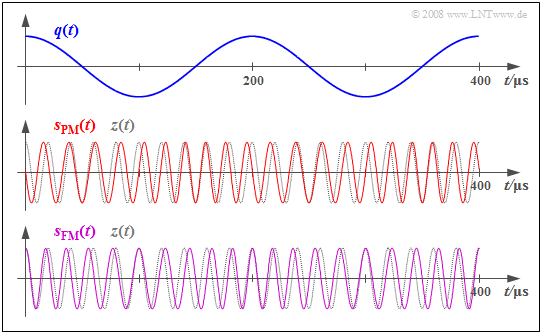
$\text{Example 2:}$ We will now assume a cosine source signal $q(t)$ with amplitude $A_{\rm N} = 3 \ \rm V$ and frequency $f_{\rm N} = 5 \ \rm kHz$ and consider the signal characteristics of phase modulation $\rm (PM)$ and frequency modulation $\rm (FM)$ with the same modulation index $η = 1.5$.

$\rm (PM)$ and $\rm (FM)$ of a cosine signal with $η = 1.5$

Thee middle graph shows the phase-modulated signal (rote curve) for the modulator parameters $f_{\rm T} = 50 \ \rm kHz$ und $K_{\rm PM} = \rm 0.5 V^{–1}$:

$$s_{\rm PM}(t) = A_{\rm T} \cdot \cos \hspace{-0.1cm} \big[\omega_{\rm T} \cdot t + \eta \cdot \cos (\omega_{\rm N} \cdot t)\big ] \hspace{0.05cm}.$$

In phase modulation, $A_{\rm N} = 3 \ \rm V$ yields $η = 1.5 ≈ π/2$ for the phase deviation (modulation index).
The maximum deviation of the zero crossings from their (equidistant) nominal positions is about a quarter of the carrier period.
If the source signal is $q(t) > 0$, the zero crossings arrive early, and if $q(t) < 0$ , they come late.

The lower graph shows the frequency-modulated signal with the same modulation index $η$:

$$s_{\rm FM}(t) = A_{\rm T} \cdot \cos \hspace{-0.1cm} \big[\omega_{\rm T} \cdot t + \eta \cdot \sin (\omega_{\rm N} \cdot t)\big ] \hspace{0.05cm}.$$

In this case, $η = 1.5$ is achieved by the modulator constant

$$K_{\rm FM} = \frac {\eta \cdot \omega_{\rm N} }{A_{\rm N} } = {K_{\rm PM} \cdot \omega_{\rm N} } $$

$$\Rightarrow \hspace{0.3cm} K_{\rm FM} = 0.5\,{\rm V}^{-1} \cdot 2 \pi \cdot 5\,{\rm kHz} = 15708\,{\rm V}^{-1}{\rm s}^{-1} \hspace{0.05cm}.$$

Here, the frequency deviation is $Δf_{\rm A} = η · f_{\rm N} = 7.5 \ \rm kHz$, and instantaneous frequencies occur between $f_{\rm T}-Δf_{\rm A} = 42.5$ and $f_{\rm T}+Δf_{\rm A} =57.5 \ \rm kHz$ .
the zero crossings now match those of the carrier signal $z(t)$ at the maxima and minima of the source signal $q(t)$ , while the maximum phase deviations can be seen at the zero crossings of $q(t)$ . The exact opposite is the case in phase modulation.

WM spectrum of a harmonic oscillation

Now we assume a harmonic oscillation with phase $ϕ_{\rm N}$ for the source signal in general:

$$q(t) = A_{\rm N} \cdot \cos \hspace{-0.1cm} \big[2 \pi f_{\rm N} \cdot t + \phi_{\rm N}\big ].$$

We are interested in the spectral function $S(f)$. For simplicity, the following will consider the magnitude spectrum $|S_+(f)|$ of the analytical signal, from which $|S(f)|$ can be derived in the familiar way.

For any of the types of angle modulation described here – whether it is phase or frequency modulation – and regardless of the phase $ϕ_{\rm N}$ of the source signal, the following holds:

$$|S_{\rm +}(f)| = A_{\rm T} \cdot \sum_{n = - \infty}^{+\infty}|{\rm J}_n (\eta)| \cdot \delta \big[f - (f_{\rm T} + n \cdot f_{\rm N})\big]\hspace{0.05cm}.$$

This equation can be explained as follows:

On the page equivalent low-pass signal in PM this equation was derived for a phase-modulated sine signal, where $η = A_{\rm N} · K_{\rm PM}$ denotes the modulation index and ${\rm J}_n(η)$ denotes the $n$–th order Bessel functions of the first kind. $K_{\rm PM}$ is the modulator constant.
When the message phase $ϕ_{\rm N}$ changes, only the phase function ${\rm arc} \ S_+(f)$ changes, but not the magnitude spectrum $|S_+(f)|$. This important result is confirmed in Exercise 3.3Z .
On the Frequency modulation of a cosine signal page, it was shown that a frequency-modulated signal can be represented in the same way as a phase-modulated signal if the modulation index $η = K_{\rm FM} · A_{\rm N}/ω_{\rm N}$ is used. Consequently, the magnitude spectra for and frequency modulation can also be represented in the same way.

Here we would like to refer you to the second part of the tutorial video Angle modulation – Frequency and phase modulation (currently available in German).

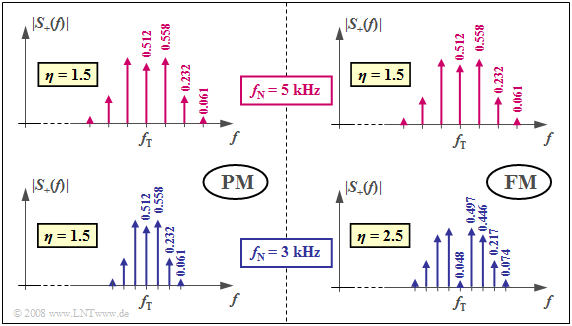
$\text{Example 3:}$ Let us again consider a harmonic oscillation, this time with amplitude $A_{\rm N} = 3 \ \rm V$ according to

discrete spectra in phase and frequency modulation

a phase modulation with $K_{\rm PM} = \rm 0.5 \ \rm V^{–1}$,
a frequency modulation with $K_{\rm FM} = \rm 15708 \ \rm V^{–1}s^{–1}$.

The corresponding signal characteristics are shown in $\text{Example 2}$ .

For both transmission systems, a Bessel spectrum with modulation index $η = 1.5$ is obtained for $f_{\rm N} = 5 \ \rm kHz$ .
The identical magnitude spectra of the analytical signal (positive frequencies only) are shown in the two upper graphs .
Bessel lines with values smaller than $0.03$ are ignored in both cases.

The lower graphs are valid for the message frequency $f_{\rm N} = 3 \ \rm kHz$:

In phase modulation, there is now a narrower spectral function compared to $f_{\rm N} = 5 \ \rm kHz$ , since the spacing of the Bessel lines is only $3 \ \rm kHz$ (lower graph on the left).
Since the modulation index $η = 1.5$ does not changes, we get the same Bessel weights as for $f_{\rm N} = 5 \ \rm kHz$ (upper graph).
Also in frequency modulation, the Bessel lines now occur at $3 \ \rm kHz$ spacing (lower graph on the right).
However, there are significantly more Bessel lines than in the upper right graph (valid for $η = 1.5$&nbsp) due to the larger modulation index $η = 2.5$ .
This follows from the fact that for frequency modulation, $η$ is inversely proportional to $f_{\rm N}$ .

Influence of band-limiting in angle modulation

Let us briefly summarize some previous results of this section, by assuming an example with a carrier frequency $f_{\rm T} = 100 \ \rm kHz$, a message frequency $f_{\rm N} = 5 \ \rm kHz$ and a modulation index $η = π/2 \approx 1.5$ :

The spectrum of an angle-modulated oscillation consists of Bessel lines around the carrier frequency $f_{\rm T}$ at a distance $f_{\rm N}$ from the message frequency and is infinitely extended in theory.

Even if we ignore all spectral lines with magnitudes smaller than $0.01$ , the now finite bandwidth for $η = π/2$ is still $B_{\rm HF} = 8 · f_{\rm N} = 40 \ \rm kHz$.

The locus curve – i.e.m the course of the equivalent low-pass signal in the complex plane – is ideally a circular arc with an opening angle of $±1.57 \ {\rm rad} = ±90^\circ$.

However, this circular arc after vector summation results only if all Bessel lines on the locus rotate with the correct pointer lengths, the correct phase positions and the correct angular frequencies.

Logically, the circular locus curve is altered when the spectral lines are distorted (for example, by linear channel distortions) or missing altogether (for example, by band-limiting).

Since the ideal angle demodulator detects the phase $ϕ_r(t)$ of the received signal and obtains the sink signal $v(t)$ from it, this distorts it, even non-linearly. This means: The distortions are irreversible and cannot be compensated for by a linear filter.

At the same time, this means: due to linear distortions in the channel, nonlinear distortions occur in the demodulated signal $v(t)$ ⇒ as a result, new frequencies (harmonics), are created, which were not in the original source signal $q(t)$ .

Je kleiner die zur Verfügung stehende Bandbreite $B_{\rm HF}$ ist und je größer der Modulationsindex $η$ gewählt wird, desto größer wird der die nichtlinearen Verzerrungen beschreibende Klirrfaktor $K$. Als Faustformel für die erforderliche HF–Bandbreite für einen geforderten Klirrfaktor $K$ gilt nach der so genannten "Carson–Regel":

$$K < 10\%\text{:}\hspace{0.6cm} B_{\rm HF} \ge 2 \cdot f_{\rm N} \cdot (\eta +1)\hspace{0.05cm},$$

$$K < 1\%\text{:}\hspace{0.72cm} B_{\rm HF} \ge 2 \cdot f_{\rm N} \cdot(\eta +2)\hspace{0.05cm}.$$

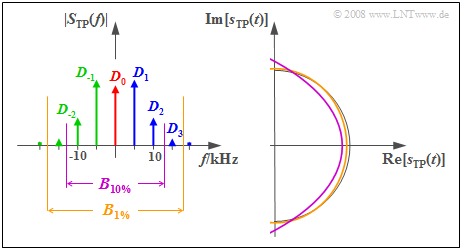
$\text{Beispiel 4:}$ Wir gehen weiterhin von den Systemparametern $f_{\rm T} = 100 \ \rm kHz$, $f_{\rm N} = 5 \ \rm kHz$ und $η = π/2$ aus. Die Grafik zeigt für diesen Fall links das Betragsspektrum $ \vert S_{\rm TP}(f) \vert$ des äquivalenten Tiefpass–Signals und rechts die zugehörige komplexe Zeitfunktion $s_{\rm TP}(t)$.

Einfluss einer Bandbegrenzung bei Winkelmodulation

Um den Klirrfaktor auf Werte $K < 1\%$ zu begrenzen, ist nach der Carson–Regel eine HF–Bandbreite von $B_{1 \%} ≈ 36 \ \rm kHz$ erforderlich.
Das äquivalente Tiefpass–Signal setzt sich dann aus der Konstanten $D_0$ und je drei entgegen dem Uhrzeigersinn $(D_1, D_2, D_3)$ bzw. im Uhrzeigersinn $(D_{-1}, D_{-2}, D_{-3})$ drehenden Zeigern zusammen:

$$\begin{align*}s_{\rm TP}(t) & = \sum_{n = - 3}^{+3}D_n \cdot{\rm e}^{\hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}n \hspace{0.05cm}\cdot \hspace{0.05cm}\omega_{\rm N} \hspace{0.02cm}\cdot \hspace{0.05cm} t }\end{align*}$$

Die ockerfarbene Kurve in der Zeitbereichsdarstellung macht deutlich, dass sich das äquivalente Tiefpass-Signal durch diese Bandbegrenzung nur geringfügig vom verzerrungsfreien Halbkreis (dünne schwarze Linie) unterscheidet.

Gibt man sich mit einem Klirrfaktor $K < 10\%$ zufrieden, so ist bereits die HF–Bandbreite $B_{10 \%} ≈ 26\ \rm kHz$ ausreichend.

Damit werden auch die beiden Fourierkoeffizienten $D_3$ und $D_{-3}$ abgeschnitten und die violett dargestellte Ortskurve beschreibt einen Parabelabschnitt.
Die Simulation dieses Fallbeispiels liefert den Klirrfaktor $K ≈ 6\%$.
Man erkennt: Die Carson–Regel liefert oft ein etwas zu pessimistisches Ergebnis $(10\%$ anstelle von $6\%)$.

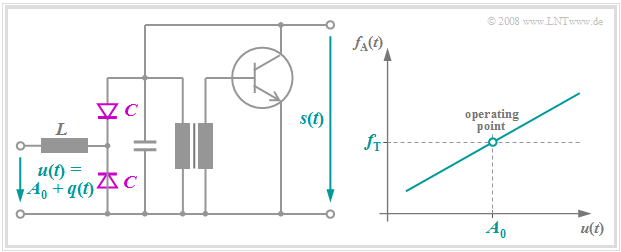
Realisierung eines FM–Modulators

Eine Frequenzmodulation erhält man dann, wenn die Schwingfrequenz eines Oszillators im Rhythmus des modulierenden Signals verändert wird. Als frequenzbestimmende Elemente dienen meist RC–Glieder oder Schwingkreise. Die linke Grafik zeigt eine schaltungstechnische Realisierungsform; die genaue Schaltungsbeschreibung finden Sie in [Mäu 88]^[1]. Rechts ist die idealisierte Frequenz–Spannungskennlinie dargestellt.

Realisierung eines FM–Modulators und dessen Kennlinie

Hier sollen nur einige wenige Anmerkungen gemacht werden:

Die anliegende Spannung $u(t)$ setzt sich additiv aus dem Quellensignal $q(t)$ und einem Gleichanteil $A_0$ zusammen, der den Arbeitspunkt festlegt.
Die Kapazität $C$ der Kapazitätsdiode ist näherungsweise proportional zu $1/u^{2}(t)$, so dass sich die Schwingfrequenz des LC–Oszillators abhängig von $q(t)$ verändert.
Bei nur kleiner Frequenzänderung hängen $u(t)$ und $f_{\rm A}(t)$ linear zusammen. Damit ist die Augenblickskreisfrequenz mit der Steigung $K_{\rm FM}$ der Modulatorkennlinie wie folgt gegeben:

$$\omega_{\rm A}(t) = \omega_{\rm T} + K_{\rm FM} \cdot q(t) \hspace{0.05cm}.$$

Die Gegentaktschaltung aus den beiden Kapazitätsdioden dient unter Anderem zur Kompensation von Unsymmetrien und damit zur Verminderung der quadratischen Verzerrungen.
Mit dem Eingang $A_0+{\rm d}q(t)/{\rm d}t$ erhält man am Ausgang das frequenzmodulierte Signal $s(t)$.

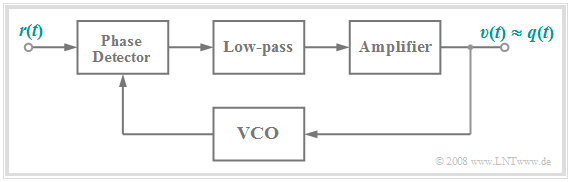
PLL–Realisierung eines FM–Demodulators

Die folgende Grafik zeigt eine zweite Realisierungsmöglichkeit des FM–Demodulators. Eine detaillierte Schaltungsbeschreibung dieses PLL–FM–Demodulators und weitere FM–Demodulatoren – zum Beispiel mittels Flankendiskriminator – finden Sie in [Mäu 88]^[1].

In Stichpunkten lässt sich diese Schaltung, die als Phasenregelschleife $($Phase–Locked–Loop, $\rm PLL)$ arbeitet, wie folgt beschreiben:

PLL – Realisierung eines FM–Demodulators

Der Phasendetektor ermittelt die Phasenunterschiede (Abstände der Nulldurchgänge) zwischen dem Empfangssignal $r(t)$ und dem vom VCO bereitgestellten Vergleichssignal.
Das Ausgangssignal $v(t)$ nach Tiefpass–Filterung und Verstärkung ist dann näherungsweise gleich dem Quellensignal $q(t)$, wenn dieses sendeseitig FM–moduliert wurde.
Das Ausgangssignal $v(t)$ wird gleichzeitig an den Eingang des spannungsgesteuerten Oszillators angelegt. Man bezeichnet diesen auch als Voltage Controlled Oscillator, abgekürzt $\rm VCO$.
Das Ausgangssignal des VCO wird permanent in der Weise nachgeregelt, dass dessen Frequenz möglichst der Augenblicksfrequenz $f_{\rm A}(t)$ des Empfangssignals $r(t)$ entspricht.