Difference between revisions of "Linear and Time Invariant Systems/Laplace Transform and p-Transfer Function"
| (19 intermediate revisions by 2 users not shown) | |||
| Line 11: | Line 11: | ||
[[File:EN_LZI_T_3_2_S1.png |right|frame| General (also non-causal) and causal system model|class=fit]] | [[File:EN_LZI_T_3_2_S1.png |right|frame| General (also non-causal) and causal system model|class=fit]] | ||
| − | For non-causal systems and signals, the [[Signal_Representation/Fourier_Transform_and_Its_Inverse#The_first_Fourier_integral|first Fourier integral]] must always be applied to describe the spectral behavior and the following is valid for the output spectrum: | + | For non-causal systems and signals, the [[Signal_Representation/Fourier_Transform_and_Its_Inverse#The_first_Fourier_integral|»first Fourier integral«]] must always be applied to describe the spectral behavior and the following is valid for the output spectrum: |
:$$Y(f) = X(f) \cdot H(f) \hspace{0.05cm}.$$ | :$$Y(f) = X(f) \cdot H(f) \hspace{0.05cm}.$$ | ||
| − | The Fourier integral also continues to be valid for causal systems and signals, i.e. under the assumption | + | The Fourier integral also continues to be valid for causal systems and signals, i.e. under the assumption |
:$$x(t) = 0 \hspace{0.2cm}{\rm{for}} \hspace{0.2cm} t<0\hspace{0.05cm},\hspace{0.2cm} h(t) = 0 \hspace{0.2cm}{\rm{for}} \hspace{0.2cm} t<0 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} y(t) = 0 \hspace{0.2cm}{\rm{for}} \hspace{0.2cm} t<0 \hspace{0.05cm}.$$ | :$$x(t) = 0 \hspace{0.2cm}{\rm{for}} \hspace{0.2cm} t<0\hspace{0.05cm},\hspace{0.2cm} h(t) = 0 \hspace{0.2cm}{\rm{for}} \hspace{0.2cm} t<0 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} y(t) = 0 \hspace{0.2cm}{\rm{for}} \hspace{0.2cm} t<0 \hspace{0.05cm}.$$ | ||
| − | In this case, however, there are significant advantages in applying the Laplace transformation while taking into account certain restrictions: | + | In this case, however, there are significant advantages in applying the »Laplace transformation« while taking into account certain restrictions: |
| − | + | #The systems treated in this way are always realizable by a circuit. The developer is not tempted to offer unrealistic solutions. | |
| − | + | #The Laplace transform $X_{\rm L}(p)$ is always a real function of the spectral variable $p$. | |
| − | + | #The fact that this variable is derived from the multiplication of the physical angular frequency $ω = 2πf$ by the imaginary unit $\rm j$ according to $p = {\rm j} · 2πf$ does not matter for the user. | |
| + | #The implicit condition $x(t) = 0$ for $t < 0$ specifically allows for simpler analysis of transient <br>behavior after switching-on processes than with the Fourier integral. | ||
==Definition of the Laplace transformation== | ==Definition of the Laplace transformation== | ||
<br> | <br> | ||
| − | Starting from the [[Signal_Representation/Fourier_Transform_and_Its_Inverse#The_first_Fourier_integral|first Fourier integral]] | + | Starting from the [[Signal_Representation/Fourier_Transform_and_Its_Inverse#The_first_Fourier_integral|»first Fourier integral«]] |
:$$X(f) = \int_{-\infty}^{+\infty} { x(t) \hspace{0.05cm}\cdot \hspace{0.05cm} {\rm e}^{-{\rm j}\hspace{0.05cm} 2\pi f t}}\hspace{0.1cm}{\rm d}t,$$ | :$$X(f) = \int_{-\infty}^{+\infty} { x(t) \hspace{0.05cm}\cdot \hspace{0.05cm} {\rm e}^{-{\rm j}\hspace{0.05cm} 2\pi f t}}\hspace{0.1cm}{\rm d}t,$$ | ||
the Laplace transformation is obtained directly by using the formal substitution $p = {\rm j} · 2πf$ for a causal time function $x(t) = 0 \ \ \ \text{for} \ \ \ t < 0.$ | the Laplace transformation is obtained directly by using the formal substitution $p = {\rm j} · 2πf$ for a causal time function $x(t) = 0 \ \ \ \text{for} \ \ \ t < 0.$ | ||
| Line 29: | Line 30: | ||
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
$\text{Definition:}$ | $\text{Definition:}$ | ||
| − | The '''Laplace transform''' of a causal time function $x(t)$ is: | + | The »'''Laplace transform'''« of a causal time function $x(t)$ is: |
:$$X_{\rm L}(p) = \int_{0}^{\infty} { x(t) \hspace{0.05cm}\cdot \hspace{0.05cm} {\rm e}^{-p t} }\hspace{0.1cm}{\rm d}t\hspace{0.05cm}, \hspace{0.3cm}{\rm briefly}\hspace{0.3cm} X_{\rm L}(p) \quad \bullet\!\!-\!\!\!-^{\hspace{-0.25cm}\rm L}\!\!\!-\!\!\circ\quad x(t)\hspace{0.05cm}.$$ }} | :$$X_{\rm L}(p) = \int_{0}^{\infty} { x(t) \hspace{0.05cm}\cdot \hspace{0.05cm} {\rm e}^{-p t} }\hspace{0.1cm}{\rm d}t\hspace{0.05cm}, \hspace{0.3cm}{\rm briefly}\hspace{0.3cm} X_{\rm L}(p) \quad \bullet\!\!-\!\!\!-^{\hspace{-0.25cm}\rm L}\!\!\!-\!\!\circ\quad x(t)\hspace{0.05cm}.$$ }} | ||
| Line 37: | Line 38: | ||
:$$X(f) = X_{\rm L}(p) \Bigg |_{{\hspace{0.1cm} p\hspace{0.05cm}={\rm \hspace{0.05cm} j\hspace{0.05cm}2\pi \it f}}}.$$ | :$$X(f) = X_{\rm L}(p) \Bigg |_{{\hspace{0.1cm} p\hspace{0.05cm}={\rm \hspace{0.05cm} j\hspace{0.05cm}2\pi \it f}}}.$$ | ||
| − | + | #However, if the signal $x(t)$ has periodic components and thus the spectral function $X(f)$ contains Dirac delta functions, then this equation is not applicable. | |
| − | + | #In this case, $p = α + {\rm j} · 2πf$ must be applied and then the limit $α → 0$ must be formed. | |
{{GraueBox|TEXT= | {{GraueBox|TEXT= | ||
$\text{Example 1:}$ | $\text{Example 1:}$ | ||
| − | We assume the unilaterally and exponentially decreasing | + | We assume the unilaterally and exponentially decreasing signal corresponding to [[Linear_and_Time_Invariant_Systems/Conclusions_from_the_Allocation_Theorem#Real_and_imaginary_part_of_a_causal_transfer_function|$\text{Example 1}$]] of chapter »Conclusions from the Allocation Theorem«: |
:$$x(t) = \left\{ \begin{array}{c} 0 \\ 0.5 \\ {\rm e}^{-t/T} \end{array} \right.\quad \quad \begin{array}{c} {\rm{for} } \\ {\rm{for} } \\ {\rm{for} } \end{array}\begin{array}{*{20}c}{ t < 0\hspace{0.05cm},} \\ { t = 0\hspace{0.05cm},} \\{ t > 0\hspace{0.05cm}.} \end{array}$$ | :$$x(t) = \left\{ \begin{array}{c} 0 \\ 0.5 \\ {\rm e}^{-t/T} \end{array} \right.\quad \quad \begin{array}{c} {\rm{for} } \\ {\rm{for} } \\ {\rm{for} } \end{array}\begin{array}{*{20}c}{ t < 0\hspace{0.05cm},} \\ { t = 0\hspace{0.05cm},} \\{ t > 0\hspace{0.05cm}.} \end{array}$$ | ||
| − | Thus, the Laplace transform is: | + | *Thus, the Laplace transform is: |
:$$X_{\rm L}(p) = \int_{0}^{\infty} {\rm e}^{-t/T} \cdot {\rm e}^{-pt} \hspace{0.1cm}{\rm d}t= \frac {1}{p + 1/T} \cdot {{\rm e}^{-(p+1/T) \hspace{0.08cm}\cdot \hspace{0.08cm}t}}\hspace{0.15cm}\Bigg \vert_{t \hspace{0.05cm}=\hspace{0.05cm} 0}^{\infty}= \frac {1}{p + 1/T} \hspace{0.05cm} .$$ | :$$X_{\rm L}(p) = \int_{0}^{\infty} {\rm e}^{-t/T} \cdot {\rm e}^{-pt} \hspace{0.1cm}{\rm d}t= \frac {1}{p + 1/T} \cdot {{\rm e}^{-(p+1/T) \hspace{0.08cm}\cdot \hspace{0.08cm}t}}\hspace{0.15cm}\Bigg \vert_{t \hspace{0.05cm}=\hspace{0.05cm} 0}^{\infty}= \frac {1}{p + 1/T} \hspace{0.05cm} .$$ | ||
| − | Considering $p = {\rm j} · 2πf$, the conventional spectral function with respect to $f$ is obtained: | + | *Considering $p = {\rm j} · 2πf$, the conventional spectral function with respect to $f$ is obtained: |
:$$X(f) = \frac {1}{{\rm j \cdot 2\pi \it f} + 1/T} = \frac {T}{1+{\rm j \cdot 2\pi \it fT}} \hspace{0.05cm} .$$ | :$$X(f) = \frac {1}{{\rm j \cdot 2\pi \it f} + 1/T} = \frac {T}{1+{\rm j \cdot 2\pi \it fT}} \hspace{0.05cm} .$$ | ||
| − | In contrast, if we consider the frequency response of a low-pass filter of first-order whose impulse response $h(t)$ differs from the above time function by the factor $1/T$, then the following holds for the Laplace transform and the Fourier transform, respectively: | + | *In contrast, if we consider the frequency response of a low-pass filter of first-order whose impulse response $h(t)$ differs from the above time function by the factor $1/T$, then the following holds for the Laplace transform and the Fourier transform, respectively: |
:$$H_{\rm L}(p)= \frac {1/T}{p + 1/T}= \frac {1}{1 + p \cdot T} \hspace{0.05cm} , \hspace{0.8cm}H(f) = \frac {1}{1+{\rm j \cdot 2\pi \it fT} } = \frac {1}{1+{\rm j} \cdot f/f_{\rm G} } \hspace{0.05cm} .$$ | :$$H_{\rm L}(p)= \frac {1/T}{p + 1/T}= \frac {1}{1 + p \cdot T} \hspace{0.05cm} , \hspace{0.8cm}H(f) = \frac {1}{1+{\rm j \cdot 2\pi \it fT} } = \frac {1}{1+{\rm j} \cdot f/f_{\rm G} } \hspace{0.05cm} .$$ | ||
| − | In this equation, the | + | *In this equation, the »3 dB cut-off frequency« $f_{\rm G} = 1/(2πT)$ $($German: "Grenzfrequenz" ⇒ subscript "G"$)$ is used instead of the parameter $T$.}} |
==Some important Laplace correspondences== | ==Some important Laplace correspondences== | ||
<br> | <br> | ||
| − | Some important Laplace correspondences are compiled subsequently. All time signals $x(t)$ considered here are assumed to be dimensionless. For this reason, $X_{\rm L}(p)$ then always has the unit | + | Some important Laplace correspondences are compiled subsequently. All time signals $x(t)$ considered here are assumed to be dimensionless. For this reason, $X_{\rm L}(p)$ then always has the unit »second« as an integral over time. |
| − | [[File: | + | [[File:EN_LZI_T_3_2_S3_v2.png |right|frame| Table with some Laplace transforms|class=fit]] |
| − | + | #The Laplace transform of the [[Signal_Representation/Special_Cases_of_Impulse_Signals#Dirac_delta_or_impulse|»Dirac delta function«]] $δ(t)$ is $X_{\rm L}(p) = 1$ $($diagram $\rm A)$. | |
| − | + | #$X_{\rm L}(p) = 1/p$ is obtained for the »unit jump function« $γ(t)$ $($diagram $\rm B)$ by applying the [[Signal_Representation/Fourier_Transform_Laws#Integration_Theorem|»integration theorem«]]. | |
| − | + | #From this the Laplace transform of the »linearly increasing function« $x(t) = t/T$ for $t > 0$ is obtained by multiplication by $1/(pT)$ $($diagram $\rm C)$. | |
| − | + | #The [[Signal_Representation/Special_Cases_of_Impulse_Signals#Rectangular_pulse|»rectangular function«]] can be generated by subtraction of two jump functions $γ(t)$ and $γ(t – T)$ separated by $T$ so that the Laplace transform $X_{\rm L}(p) = (1 – {\rm e}^{–pT})/p$ is obtained according to the [[Signal_Representation/Fourier_Transform_Theorems#Shifting_Theorem|»shifting theorem«]] $($diagram $\rm D)$. | |
| − | + | #By integration the »ramp function« or after multiplication by $1/(pT)$ its Laplace transform is obtained from it $($diagram $\rm E)$. | |
| − | + | #The »exponential function« $($diagram $\rm F)$ has already been considered in the [[Linear_and_Time_Invariant_Systems/Laplace_Transform_and_p-Transfer_Function#Definition_of_the_Laplace_transformation|»last section«]] . Considering the factor $1/T$, this is at the same time the impulse response of a low-pass filter of first-order. | |
| − | + | #The Laplace transform of a second-order low-pass ⇒ function $x(t) = t/T · {\rm e}^{–t/T}$ is obtained by squaring $($diagram $\rm G)$. | |
| + | #In addition to the causal $\rm sinc$–function $($diagram $\rm H)$, the Laplace transforms of the »causal cosine and sine functions« $($diagrams $\rm I$ and $\rm J)$, which result in $p/(p^2 + ω_0^2)$ resp. $ω_0/(p^2 + ω_0^2)$ are also given in the table. | ||
| + | #Here, $ω_0 = 2πf_0 = 2π/T$ denotes the »angular frequency«. | ||
| Line 73: | Line 76: | ||
==Pole-zero representation of circuits== | ==Pole-zero representation of circuits== | ||
<br> | <br> | ||
| − | Any [[Linear_and_Time_Invariant_Systems/System_Description_in_Frequency_Domain#Prerequisites_for_the_application_of_Systems_Theory|linear time-invariant system]] $\rm (LTI)$ which can be realized by a circuit of discrete time-constant components such as | + | Any [[Linear_and_Time_Invariant_Systems/System_Description_in_Frequency_Domain#Prerequisites_for_the_application_of_Systems_Theory|»linear time-invariant system«]] $\rm (LTI)$ which can be realized by a circuit of discrete time-constant components such as |
*resistances $(R)$, | *resistances $(R)$, | ||
*capacitances $(C)$, | *capacitances $(C)$, | ||
| Line 80: | Line 83: | ||
| − | + | have a fractional-rational »'''$p$–transfer function'''«: | |
:$$H_{\rm L}(p)= \frac {A_Z \cdot p^Z +\text{...} + A_2 \cdot p^2 + A_1 \cdot p + A_0} {B_N \cdot p^N +\text{...} \ + B_2 \cdot p^2 + B_1 \cdot p + B_0}= \frac {Z(p)}{N(p)} \hspace{0.05cm} .$$ | :$$H_{\rm L}(p)= \frac {A_Z \cdot p^Z +\text{...} + A_2 \cdot p^2 + A_1 \cdot p + A_0} {B_N \cdot p^N +\text{...} \ + B_2 \cdot p^2 + B_1 \cdot p + B_0}= \frac {Z(p)}{N(p)} \hspace{0.05cm} .$$ | ||
All coefficients of the numerator ⇒ $A_Z, \text{...} \ , A_0$ and of the denominator ⇒ $B_N, \text{...} , B_0$ are real. Furthermore: | All coefficients of the numerator ⇒ $A_Z, \text{...} \ , A_0$ and of the denominator ⇒ $B_N, \text{...} , B_0$ are real. Furthermore: | ||
| − | + | #$Z$ denotes the degree of the numerator polynomial $Z(p)$, | |
| − | + | #$N$ denotes the degree of the denominator polynomial $N(p)$. | |
{{BlaueBox|TEXT= | {{BlaueBox|TEXT= | ||
$\text{Equivalent pole-zero representation:}$ | $\text{Equivalent pole-zero representation:}$ | ||
| − | The following can be formulated for the $p$–transfer function, too: | + | The following can be formulated for the »'''$p$–transfer function«''', too: |
:$$H_{\rm L}(p)= K \cdot \frac {\prod\limits_{i=1}^Z p - p_{\rm o i} } {\prod\limits_{i=1}^N p - p_{\rm x i} }= K \cdot \frac {(p - p_{\rm o 1})(p - p_{\rm o 2})\cdot \text{...} \ \cdot (p - p_{ {\rm o} \hspace{-0.03cm} Z})} {(p - p_{\rm x 1})(p - p_{\rm x 2})\cdot \text{...} \cdot (p - p_{ {\rm x} \hspace{-0.03cm} N})} \hspace{0.05cm} .$$ | :$$H_{\rm L}(p)= K \cdot \frac {\prod\limits_{i=1}^Z p - p_{\rm o i} } {\prod\limits_{i=1}^N p - p_{\rm x i} }= K \cdot \frac {(p - p_{\rm o 1})(p - p_{\rm o 2})\cdot \text{...} \ \cdot (p - p_{ {\rm o} \hspace{-0.03cm} Z})} {(p - p_{\rm x 1})(p - p_{\rm x 2})\cdot \text{...} \cdot (p - p_{ {\rm x} \hspace{-0.03cm} N})} \hspace{0.05cm} .$$ | ||
| − | The $Z + N + 1$ parameters signify: | + | The $Z + N + 1$ parameters signify: |
| − | + | #$K = A_Z/B_N$ is a constant factor. If $Z = N$, this is dimensionless. | |
| − | + | #The solutions of the equation $Z(p) = 0$ result in the $Z$ »zeros« $p_{\rm o1},\text{...} \ , p_{\rm oZ}$ of $H_{\rm L}(p)$. | |
| − | + | #The zeros of the denominator polynomial $N(p)$ yield the $N$ pole locations $($or »poles« for short$)$. }} | |
The transformation is unique. This can be seen from the fact that the $p$–transfer function is also determined only by $Z + N + 1$ free parameters according to the first equation since one of the coefficients $A_Z, \text{...} \ , A_0, B_N, \text{...} \ , B_0$ can be normalized to $1$ without changing the quotient. | The transformation is unique. This can be seen from the fact that the $p$–transfer function is also determined only by $Z + N + 1$ free parameters according to the first equation since one of the coefficients $A_Z, \text{...} \ , A_0, B_N, \text{...} \ , B_0$ can be normalized to $1$ without changing the quotient. | ||
| − | [[File:P_ID1759__LZI_T_3_2_S4_neu.png|right|frame|Considered two-port network and associated pole-zero diagram|class=fit]] | + | {{GraueBox|TEXT= |
| − | + | [[File:P_ID1759__LZI_T_3_2_S4_neu.png|right|frame|Considered two-port network and associated pole-zero diagram|class=fit]] | |
$\text{Example 2:}$ | $\text{Example 2:}$ | ||
| − | We consider the drawn two-port network with an inductance $L$ $($complex resistance $pL)$ in the longitudinal branch as well as | + | We consider the drawn two-port network with |
| + | *an inductance $L$ $($complex resistance $pL)$ in the longitudinal branch as well as | ||
| − | Thus, the $p$–transfer function is: | + | *the series connection of an ohmic resistance $R$ and a capacitance $C$ with the complex resistance $1/(pC)$ in the transverse branch. |
| + | |||
| + | |||
| + | Thus, the $p$–transfer function is: | ||
:$$H_{\rm L}(p)= \frac {Y_{\rm L}(p)} {X_{\rm L}(p)}= \frac {R + {1}/{(pC)} } {pL + R +{1}/{(pC)} }$$ | :$$H_{\rm L}(p)= \frac {Y_{\rm L}(p)} {X_{\rm L}(p)}= \frac {R + {1}/{(pC)} } {pL + R +{1}/{(pC)} }$$ | ||
:$$\Rightarrow \hspace{0.3cm} H_{\rm L}(p)= \frac {1 + p \cdot{RC} } {1 + p \cdot{RC}+ p^2 \cdot{LC} } | :$$\Rightarrow \hspace{0.3cm} H_{\rm L}(p)= \frac {1 + p \cdot{RC} } {1 + p \cdot{RC}+ p^2 \cdot{LC} } | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | If $p = {\rm j} · 2πf$ is set, then the Fourier transfer function (or the frequency response) is obtained. If the numerator and denominator in the above equation are divided by $LC$, then the following is obtained: | + | If $p = {\rm j} · 2πf$ is set, then the Fourier transfer function $($or the »frequency response«$)$ is obtained. If the numerator and denominator in the above equation are divided by $LC$, then the following is obtained: |
:$$H_{\rm L}(p)= \frac {R} {L}\cdot \frac {p + {1}/{(RC)} } {p^2 + {R}/ {L}\cdot p + {1}/{(LC)} }= K \cdot \frac {p - p_{\rm o } } {(p - p_{\rm x 1})(p - p_{\rm x 2})} | :$$H_{\rm L}(p)= \frac {R} {L}\cdot \frac {p + {1}/{(RC)} } {p^2 + {R}/ {L}\cdot p + {1}/{(LC)} }= K \cdot \frac {p - p_{\rm o } } {(p - p_{\rm x 1})(p - p_{\rm x 2})} | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | In the right-hand side of the equation, the transfer function $H_{\rm L}(p)$ is given in pole-zero notation. By comparison of coefficients the following values are obtained for $R = 50 \ \rm Ω$, $L = 25\ \rm µ H$ and $C = 62.5 \ \rm nF$ : | + | ⇒ In the right-hand side of the equation, the transfer function $H_{\rm L}(p)$ is given in »'''pole-zero notation'''«. |
| − | + | ||
| − | + | By comparison of coefficients the following values are obtained for $R = 50 \ \rm Ω$, $L = 25\ \rm µ H$ and $C = 62.5 \ \rm nF$ : | |
| − | + | #the constant $K = R/L = 2 · 10^6 \cdot 1/{\rm s}$, | |
| − | :$$p^2 + \frac {R} {L}\cdot p + \frac{1}{LC} = 0 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm x 1,\hspace{0.05cm}2 }= -\frac {R} {2L}\pm \sqrt{\frac | + | #the zero $p_{\rm o} = -1/(RC) = -0.32 · 10^6 \cdot 1/{\rm s},$ |
| + | #the two poles $p_{\rm x1}$ and $p_{\rm x2}$ as the solution of the equation | ||
| + | ::$$p^2 + \frac {R} {L}\cdot p + \frac{1}{LC} = 0 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm x 1,\hspace{0.05cm}2 }= -\frac {R} {2L}\pm \sqrt{\frac | ||
{R^2} {4L^2}- \frac{1}{LC} }$$ | {R^2} {4L^2}- \frac{1}{LC} }$$ | ||
| − | :$$\Rightarrow \hspace{0.3cm} p_{\rm x 1,\hspace{0.05cm}2 }= -10^6 \cdot {1}/{\rm s} \pm \sqrt{10^{12} \cdot {1} /{\rm s^2}-0.64 \cdot 10^{12} \cdot {1}/ {\rm s^2} }\hspace{0. | + | ::$$\Rightarrow \hspace{0.3cm} p_{\rm x 1,\hspace{0.05cm}2 }= -10^6 \cdot {1}/{\rm s} \pm \sqrt{10^{12} \cdot {1} /{\rm s^2}-0.64 \cdot 10^{12} \cdot {1}/ {\rm s^2} }\hspace{0.6cm} |
\Rightarrow \hspace{0.3cm} p_{\rm x 1 }= -0.4 \cdot 10^6\cdot {1}/ {\rm s},\hspace{0.2cm}p_{\rm x 2 }= -1.6 \cdot 10^6\cdot {1}/ {\rm s} \hspace{0.05cm} .$$ | \Rightarrow \hspace{0.3cm} p_{\rm x 1 }= -0.4 \cdot 10^6\cdot {1}/ {\rm s},\hspace{0.2cm}p_{\rm x 2 }= -1.6 \cdot 10^6\cdot {1}/ {\rm s} \hspace{0.05cm} .$$ | ||
| − | In the above graph, the pole-zero diagram is given on the right-hand side. | + | In the above graph, the pole-zero diagram is given on the right-hand side. |
| − | *The two axes denote the real and imaginary parts of the variable $p$, each normalized to the value $10^6 · \rm 1/s\; (= 1/µs)$. | + | *The two axes denote the real and imaginary parts of the variable $p$, each normalized to the value $10^6 · \rm 1/s\; (= 1/µs)$. |
| + | |||
*The zero at $p_{\rm o} =\, –0.32$ can be seen as a circle and the poles at $p_{\rm x1} = \,–0.4$ and $p_{\rm x2} = \,–1.6$ as crosses.}} | *The zero at $p_{\rm o} =\, –0.32$ can be seen as a circle and the poles at $p_{\rm x1} = \,–0.4$ and $p_{\rm x2} = \,–1.6$ as crosses.}} | ||
==Properties of poles and zeros== | ==Properties of poles and zeros== | ||
<br> | <br> | ||
| − | The transfer function $H_{\rm L}(p)$ of any realizable circuit is fully described by $Z$ | + | The transfer function $H_{\rm L}(p)$ of any realizable circuit is fully described by $Z$ »zeros« and $N$ »poles« together with a constant $K$ where the following restrictions apply: |
| − | *$Z ≤ N$ always holds. The $p$–transfer function would also be | + | *$Z ≤ N$ always holds. The $p$–transfer function would also be »infinitely large« with $Z > N$ in the limiting case for $p → ∞$ $($i.e. for very high frequencies$)$. |
| − | *The zeros $p_{\rm oi}$ and the poles $p_{ {\rm x}i}$ are generally complex, and have like $p$ the unit $\rm 1/s$. If $Z < N$ holds, then the constant $K$ has also a unit. | + | |
| − | *The poles and zeros can be real as shown in the last example. If they are complex, then two poles | + | *The zeros $p_{\rm oi}$ and the poles $p_{ {\rm x}i}$ are generally complex, and have like $p$ the unit $\rm 1/s$. If $Z < N$ holds, then the constant $K$ has also a unit. |
| − | *All poles lie in the left half-plane or on the imaginary axis (limiting case). This property follows from the required and assumed causality together with the [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform#Some_results_of_the_theory_of_functions|fundamental | + | |
| − | *Zeros can occur in both the left and right $p$–half-planes or also on the imaginary axis. An example of zeros in the right half-plane can be found in [[Aufgaben:Exercise_3.4Z:_Various_All-Pass_Filters|Exercise 3.4Z]], which deals with all-pass filters. | + | *The poles and zeros can be real as shown in the last example. If they are complex, then two poles resp. two zeros always occur as complex conjugates, since $H_{\rm L}(p)$ always represents a real fractional-rational function. |
| − | *In so-called | + | |
| + | *All poles lie in the left half-plane or on the imaginary axis $($limiting case$)$. This property follows from the required and assumed causality together with the [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform#Some_results_of_the_theory_of_functions|»fundamental function theory«]], which will be stated in the next chapter. | ||
| + | |||
| + | *Zeros can occur in both the left and right $p$–half-planes or also on the imaginary axis. An example of zeros in the right half-plane can be found in [[Aufgaben:Exercise_3.4Z:_Various_All-Pass_Filters|$\text{Exercise 3.4Z}$]], which deals with all-pass filters. | ||
| + | |||
| + | *In so-called »minimum-phase systems«, not only poles are forbidden in the right $p$–half-plane but also zeros. The real part of all singularities here is never positive. | ||
| Line 141: | Line 156: | ||
{{GraueBox|TEXT= | {{GraueBox|TEXT= | ||
$\text{Example 3:}$ | $\text{Example 3:}$ | ||
| − | Starting from the [[Linear_and_Time_Invariant_Systems/Laplace_Transform_and_p-Transfer_Function#Pole-zero_representation_of_circuits |two-port network]] $(L$ in the longitudinal branch, $R$ and $C$ in the transverse branch$)$ the characteristic quantities of the transfer function can be given as follows: | + | Starting from the [[Linear_and_Time_Invariant_Systems/Laplace_Transform_and_p-Transfer_Function#Pole-zero_representation_of_circuits |»two-port network«]] $(L$ in the longitudinal branch, $R$ and $C$ in the transverse branch$)$ the characteristic quantities of the transfer function can be given as follows: |
| − | :$$K = 2A, \hspace{0.2cm}p_{\rm x 1,\hspace{0.05cm}2 }= -A \pm \sqrt{A^2-B^2}, | + | [[File:EN_LZI_T_3_2_S5_neu_v2.png|right|frame|Location of the zero and the poles for $Z = 1$ and $N = 2$|class=fit]] |
| − | The graph shows three different diagrams with different capacitance values $C$. | + | :$$K = 2A, \hspace{0.2cm}p_{\rm x 1,\hspace{0.05cm}2 }= -A \pm \sqrt{A^2-B^2},$$ |
| + | :$$p_{\rm o }= - \frac{B^2}{2A} \hspace{0.05cm} \hspace{0.2cm} {\rm with } \hspace{0.2cm} A = \frac {R} {2L}, \hspace{0.2cm}B = \frac{1}{\sqrt{LC} } \hspace{0.05cm}.$$ | ||
| + | The graph shows three different diagrams with different capacitance values $C$. | ||
| + | *$R = 50 \ \rm Ω$ and $L = 25 \ \rm µ H$ always hold. | ||
| − | + | *The axes are normalized to $A = R/(2L) = 10^6 · \rm 1/s$. | |
| − | *For $B < A$, '''two real poles''' and | + | *The constant factor is $K = 2A = 2 · 10^6 · \rm 1/s.$ |
| + | <br clear=all> | ||
| + | $\text{Example 3.1:}$ <br> | ||
| + | For $B < A$, »'''two real poles'''« and »one zero« to the right of $-A/2$ are obtained. The following arises as a result for $C = 62.5 \ \rm nF$ ⇒ $ {B}/ {A}= 0.8 $ $($left diagram$)$: | ||
:$$ p_{\rm x 1}/A = -0.4 , \hspace{0.2cm}p_{\rm x 2}/A= -1.6 , \hspace{0.2cm}p_{\rm o}/A= -0.32 \hspace{0.05cm} .$$ | :$$ p_{\rm x 1}/A = -0.4 , \hspace{0.2cm}p_{\rm x 2}/A= -1.6 , \hspace{0.2cm}p_{\rm o}/A= -0.32 \hspace{0.05cm} .$$ | ||
| − | + | ||
| + | $\text{Example 3.2:}$ <br> | ||
| + | For $B > A$, »'''two conjugate-complex poles'''« and »one zero« to the left of $-A/2$ are obtained. For $C = 8 \ \rm nF$ ⇒ $ {B}/ {A}= \sqrt{5} $ $($right diagram$)$: | ||
:$$p_{\rm x 1,\hspace{0.05cm}2 }/A= -1\pm {\rm j}\cdot 2,\hspace{0.2cm}p_{\rm o}/A\approx -2.5 \hspace{0.05cm} .$$ | :$$p_{\rm x 1,\hspace{0.05cm}2 }/A= -1\pm {\rm j}\cdot 2,\hspace{0.2cm}p_{\rm o}/A\approx -2.5 \hspace{0.05cm} .$$ | ||
| − | + | ||
| + | $\text{Example 3.3:}$ <br> | ||
| + | The case $A = B$ leads to »'''a real double pole'''« and »one zero« at $– A/2$. For $C = 400 \ \rm nF$ ⇒ $ {B}/ {A}= 1 $ $($middle diagram$)$: | ||
:$$ p_{\rm x 1}/A= p_{\rm x 2}/A= -1, \hspace{0.2cm}p_{\rm o}/A= -0.5 \hspace{0.05cm} .$$ | :$$ p_{\rm x 1}/A= p_{\rm x 2}/A= -1, \hspace{0.2cm}p_{\rm o}/A= -0.5 \hspace{0.05cm} .$$ | ||
| − | The impulse responses $h(t)$ are obtained according to the following chapter [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform|Inverse Laplace Transform]] as follows: | + | The impulse responses $h(t)$ are obtained according to the following chapter [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform|»Inverse Laplace Transform«]] as follows: |
| − | + | #For the left constellation, $h(t)$ is [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform#Aperiodically_decaying_impulse_response|»aperiodically decaying«]]. | |
| − | + | #For the right constellation, $h(t)$ is [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform#Attenuated-oscillatory_impulse_response|»attenuated-oscillatory«]]. | |
| − | + | #The middle constellation is called the [[Linear_and_Time_Invariant_Systems/Inverse_Laplace_Transform#Critically_attenuated_case|»critically attenuated case«]]. }} | |
==Graphical determination of attenuation and phase== | ==Graphical determination of attenuation and phase== | ||
<br> | <br> | ||
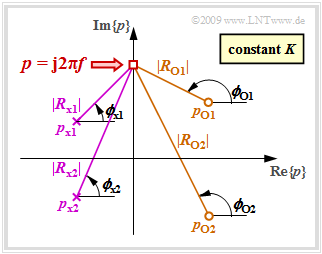
| − | [[File:EN_LZI_T_3_2_S6.png|right|frame| | + | [[File:EN_LZI_T_3_2_S6.png|right|frame|For the computation of attenuation and phase|class=fit]] |
The $p$–transfer function is given in pole-zero notation: | The $p$–transfer function is given in pole-zero notation: | ||
:$$H_{\rm L}(p)= K \cdot \frac {\prod\limits_{i=1}^Z (p - p_{\rm o i})} {\prod\limits_{i=1}^N (p - p_{\rm x i})}= K \cdot \frac {(p - p_{\rm o 1})(p - p_{\rm o 2})\cdot \text{...} \cdot (p - p_{ {\rm o} \hspace{-0.03cm} Z})} {(p - p_{\rm x 1})(p - p_{\rm x 2})\cdot \text{...} \cdot (p - p_{ {\rm x} \hspace{-0.03cm} N})} | :$$H_{\rm L}(p)= K \cdot \frac {\prod\limits_{i=1}^Z (p - p_{\rm o i})} {\prod\limits_{i=1}^N (p - p_{\rm x i})}= K \cdot \frac {(p - p_{\rm o 1})(p - p_{\rm o 2})\cdot \text{...} \cdot (p - p_{ {\rm o} \hspace{-0.03cm} Z})} {(p - p_{\rm x 1})(p - p_{\rm x 2})\cdot \text{...} \cdot (p - p_{ {\rm x} \hspace{-0.03cm} N})} | ||
\hspace{0.05cm} .$$ | \hspace{0.05cm} .$$ | ||
| − | The conventional frequency response $H(f)$ is obtained by replacing the argument $p$ of $H_{\rm L}(p)$ by ${\rm j} \cdot 2πf$ : | + | *The conventional frequency response $H(f)$ is obtained by replacing the argument $p$ of $H_{\rm L}(p)$ by ${\rm j} \cdot 2πf$ : |
:$$H(f)= K \cdot \frac {({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm o 1})({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm o 2})\cdot \text{...} \cdot ({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm o} \hspace{-0.03cm} Z})} {({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm x 1})({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm x 2})\cdot \text{...}\cdot ({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm x} \hspace{-0.03cm} N})} \hspace{0.05cm} .$$ | :$$H(f)= K \cdot \frac {({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm o 1})({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm o 2})\cdot \text{...} \cdot ({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm o} \hspace{-0.03cm} Z})} {({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm x 1})({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm x 2})\cdot \text{...}\cdot ({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm x} \hspace{-0.03cm} N})} \hspace{0.05cm} .$$ | ||
| − | We now consider a fixed frequency | + | *We now consider a fixed frequency $f$ and describe the distances and angles of all »zeros« $($circles$)$ by vectors: |
:$$R_{ {\rm o} i} = {\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm o} i}= |R_{{\rm o} i}| \cdot {\rm e}^{\hspace{0.03cm}{\rm j}\hspace{0.03cm}\cdot\hspace{0.03cm}\phi_{ {\rm o} i} }, | :$$R_{ {\rm o} i} = {\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm o} i}= |R_{{\rm o} i}| \cdot {\rm e}^{\hspace{0.03cm}{\rm j}\hspace{0.03cm}\cdot\hspace{0.03cm}\phi_{ {\rm o} i} }, | ||
\hspace{0.3cm}i= 1, \text{...}\ , Z \hspace{0.05cm} .$$ | \hspace{0.3cm}i= 1, \text{...}\ , Z \hspace{0.05cm} .$$ | ||
| − | We proceed in the same way for the | + | *We proceed in the same way for the »poles« $($crosses$)$: |
:$$R_{ {\rm x} i} = {\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm x} i}= |R_{ {\rm x} i}| \cdot {\rm e}^{\hspace{0.03cm}{\rm j}\hspace{0.03cm}\cdot\hspace{0.03cm}\phi_{ {\rm x} i} }, \hspace{0.3cm}i= 1, \text{...}\ , N \hspace{0.05cm} .$$ | :$$R_{ {\rm x} i} = {\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm x} i}= |R_{ {\rm x} i}| \cdot {\rm e}^{\hspace{0.03cm}{\rm j}\hspace{0.03cm}\cdot\hspace{0.03cm}\phi_{ {\rm x} i} }, \hspace{0.3cm}i= 1, \text{...}\ , N \hspace{0.05cm} .$$ | ||
| − | The graph shows the magnitudes and phase angles for a system | + | *The graph shows the magnitudes and phase angles for a system with |
| − | + | #$Z = 2$ zeros (circles) in the right half-plane | |
| − | + | #$N = 2$ poles (crosses) in the left half-plane. | |
| − | + | #constant $K$. | |
| − | + | *The following can be written for the frequency response with this vector representation: | |
| − | |||
| − | The following can be written for the frequency response with this vector representation: | ||
:$$H(f)= K \cdot \frac {|R_{ {\rm o} 1}| \cdot |R_{ {\rm o} 2}|\cdot ... \cdot |R_{ {\rm o} \hspace{-0.03cm} Z}|} {|R_{ {\rm x} 1}| \cdot |R_{ {\rm x} 2}|\cdot \text{...} \cdot |R_{ {\rm x} \hspace{-0.03cm} N}|} \cdot {\rm e^{\hspace{0.03cm}{\rm j} \hspace{0.05cm}\cdot [ \phi_{ {\rm o} 1}\hspace{0.1cm}+ \hspace{0.1cm}\phi_{ {\rm o} 2} \hspace{0.1cm}+ \hspace{0.1cm}\hspace{0.1cm}\text{...}. \hspace{0.1cm} + \hspace{0.1cm}\phi_{ {\rm o} \hspace{-0.03cm}{\it Z}}\hspace{0.1cm}- \hspace{0.1cm}\phi_{ {\rm x} 1}\hspace{0.1cm}- \hspace{0.1cm}\phi_{ {\rm x} 2} \hspace{0.1cm}- \hspace{0.1cm}... \hspace{0.1cm} | :$$H(f)= K \cdot \frac {|R_{ {\rm o} 1}| \cdot |R_{ {\rm o} 2}|\cdot ... \cdot |R_{ {\rm o} \hspace{-0.03cm} Z}|} {|R_{ {\rm x} 1}| \cdot |R_{ {\rm x} 2}|\cdot \text{...} \cdot |R_{ {\rm x} \hspace{-0.03cm} N}|} \cdot {\rm e^{\hspace{0.03cm}{\rm j} \hspace{0.05cm}\cdot [ \phi_{ {\rm o} 1}\hspace{0.1cm}+ \hspace{0.1cm}\phi_{ {\rm o} 2} \hspace{0.1cm}+ \hspace{0.1cm}\hspace{0.1cm}\text{...}. \hspace{0.1cm} + \hspace{0.1cm}\phi_{ {\rm o} \hspace{-0.03cm}{\it Z}}\hspace{0.1cm}- \hspace{0.1cm}\phi_{ {\rm x} 1}\hspace{0.1cm}- \hspace{0.1cm}\phi_{ {\rm x} 2} \hspace{0.1cm}- \hspace{0.1cm}... \hspace{0.1cm} | ||
- \hspace{0.1cm} \phi_{ {\rm x} \hspace{-0.03cm}{\it N} }]} } \hspace{0.05cm} .$$ | - \hspace{0.1cm} \phi_{ {\rm x} \hspace{-0.03cm}{\it N} }]} } \hspace{0.05cm} .$$ | ||
If $H(f)$ is expressed by the attenuation function $a(f)$ and the phase function $b(f)$ according to the generally valid relation $H(f) = {\rm e}^{-a(f)\hspace{0.05cm}- \hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}b(f)}$, then the following result is obtained by comparing with the above equation: | If $H(f)$ is expressed by the attenuation function $a(f)$ and the phase function $b(f)$ according to the generally valid relation $H(f) = {\rm e}^{-a(f)\hspace{0.05cm}- \hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}b(f)}$, then the following result is obtained by comparing with the above equation: | ||
| − | *With a suitable normalization of all dimensional quantities the following holds for the attenuation function in Neper $(1 \ \rm Np$ corresponds to $8.686 \ \rm dB)$: | + | *With a suitable normalization of all dimensional quantities the following holds for the »attenuation function« in Neper $(1 \ \rm Np$ corresponds to $8.686 \ \rm dB)$: |
:$$a(f) = -{\rm ln} \hspace{0.1cm} K + \sum \limits_{i=1}^N {\rm ln} \hspace{0.1cm} |R_{ {\rm x} i}|- \sum \limits_{i=1}^Z {\rm ln} \hspace{0.1cm} |R_{ {\rm o} i}| \hspace{0.05cm} .$$ | :$$a(f) = -{\rm ln} \hspace{0.1cm} K + \sum \limits_{i=1}^N {\rm ln} \hspace{0.1cm} |R_{ {\rm x} i}|- \sum \limits_{i=1}^Z {\rm ln} \hspace{0.1cm} |R_{ {\rm o} i}| \hspace{0.05cm} .$$ | ||
| − | *The phase function in radian $\rm (rad)$ arises as a result according to the upper sketch | + | *The »phase function« in radian $\rm (rad)$ arises as a result according to the upper sketch: |
:$$b(f) = \phi_K + \sum \limits_{i=1}^N \phi_{ {\rm x} i}- \sum \limits_{i=1}^Z \phi_{ {\rm o} i}\hspace{0.2cm}{\rm with} \hspace{0.2cm} \phi_K = \left\{ \begin{array}{c} 0 \\ \pi \end{array} \right. \begin{array}{c} {\rm{for} } \\ {\rm{for} } \end{array}\begin{array}{*{20}c} { K > 0\hspace{0.05cm},} \\ { K <0\hspace{0.05cm}.} \end{array}$$ | :$$b(f) = \phi_K + \sum \limits_{i=1}^N \phi_{ {\rm x} i}- \sum \limits_{i=1}^Z \phi_{ {\rm o} i}\hspace{0.2cm}{\rm with} \hspace{0.2cm} \phi_K = \left\{ \begin{array}{c} 0 \\ \pi \end{array} \right. \begin{array}{c} {\rm{for} } \\ {\rm{for} } \end{array}\begin{array}{*{20}c} { K > 0\hspace{0.05cm},} \\ { K <0\hspace{0.05cm}.} \end{array}$$ | ||
| − | |||
{{GraueBox|TEXT= | {{GraueBox|TEXT= | ||
$\text{Example 4:}$ | $\text{Example 4:}$ | ||
| − | The graphic illustrates the calculation | + | The graphic illustrates the calculation of |
| − | + | [[File:P_ID1762__LZI_T_3_2_S6b_neu.png |right|frame| For the computation of the attenuation and phase functions $($screen capture of the program »Causal Systems & Laplace Transformation» in an earlier German version$)$|class=fit]] | |
| − | + | # the attenuation function $a(f)$ ⇒ red curve, and | |
| + | # the phase function $b(f)$ ⇒ green curve | ||
| + | |||
| + | of a two-port network which is defined by | ||
| + | *the factor $K = 1.5$, | ||
| + | *one zero at $-3$ and | ||
| + | *two poles at $–1 \pm {\rm j} · 4$. | ||
| − | |||
The given numerical values are valid for the frequency $2πf = 3$: | The given numerical values are valid for the frequency $2πf = 3$: | ||
| Line 206: | Line 233: | ||
:$$ b\big [f = {3}/({2\pi}) \big ] = -8.1^\circ \hspace{0.05cm} .$$ | :$$ b\big [f = {3}/({2\pi}) \big ] = -8.1^\circ \hspace{0.05cm} .$$ | ||
| − | The derivation of these numerical values is illustrated in the framed block. For the magnitude frequency response $\vert H(f)\vert$ ⇒ blue curve, a | + | The derivation of these numerical values is illustrated in the framed block. For the magnitude frequency response $\vert H(f)\vert$ ⇒ blue curve, a band-pass-like curve shape is obtained with |
:$$\vert H(f = 0)\vert \approx 0.25\hspace{0.05cm},$$ | :$$\vert H(f = 0)\vert \approx 0.25\hspace{0.05cm},$$ | ||
| − | :$$\vert H(f = { | + | :$$\vert H(f = {3}/(2\pi)\vert \approx 0636\hspace{0.05cm},$$ |
:$$\vert H(f \rightarrow \infty)\vert= 0 \hspace{0.05cm}.$$}} | :$$\vert H(f \rightarrow \infty)\vert= 0 \hspace{0.05cm}.$$}} | ||
Latest revision as of 17:56, 19 November 2023
Contents
Considered system model
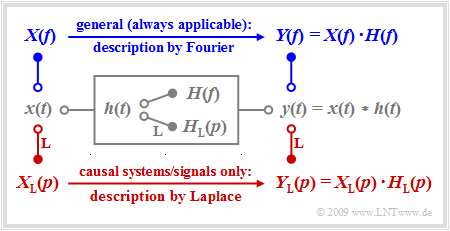
We consider a linear time-invariant system with the impulse response $h(t)$, whose input the signal $x(t)$ is applied to. The output signal $y(t)$ is then obtained as the convolution product $x(t) ∗ h(t)$.
For non-causal systems and signals, the »first Fourier integral« must always be applied to describe the spectral behavior and the following is valid for the output spectrum:
- $$Y(f) = X(f) \cdot H(f) \hspace{0.05cm}.$$
The Fourier integral also continues to be valid for causal systems and signals, i.e. under the assumption
- $$x(t) = 0 \hspace{0.2cm}{\rm{for}} \hspace{0.2cm} t<0\hspace{0.05cm},\hspace{0.2cm} h(t) = 0 \hspace{0.2cm}{\rm{for}} \hspace{0.2cm} t<0 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} y(t) = 0 \hspace{0.2cm}{\rm{for}} \hspace{0.2cm} t<0 \hspace{0.05cm}.$$
In this case, however, there are significant advantages in applying the »Laplace transformation« while taking into account certain restrictions:
- The systems treated in this way are always realizable by a circuit. The developer is not tempted to offer unrealistic solutions.
- The Laplace transform $X_{\rm L}(p)$ is always a real function of the spectral variable $p$.
- The fact that this variable is derived from the multiplication of the physical angular frequency $ω = 2πf$ by the imaginary unit $\rm j$ according to $p = {\rm j} · 2πf$ does not matter for the user.
- The implicit condition $x(t) = 0$ for $t < 0$ specifically allows for simpler analysis of transient
behavior after switching-on processes than with the Fourier integral.
Definition of the Laplace transformation
Starting from the »first Fourier integral«
- $$X(f) = \int_{-\infty}^{+\infty} { x(t) \hspace{0.05cm}\cdot \hspace{0.05cm} {\rm e}^{-{\rm j}\hspace{0.05cm} 2\pi f t}}\hspace{0.1cm}{\rm d}t,$$
the Laplace transformation is obtained directly by using the formal substitution $p = {\rm j} · 2πf$ for a causal time function $x(t) = 0 \ \ \ \text{for} \ \ \ t < 0.$
$\text{Definition:}$ The »Laplace transform« of a causal time function $x(t)$ is:
- $$X_{\rm L}(p) = \int_{0}^{\infty} { x(t) \hspace{0.05cm}\cdot \hspace{0.05cm} {\rm e}^{-p t} }\hspace{0.1cm}{\rm d}t\hspace{0.05cm}, \hspace{0.3cm}{\rm briefly}\hspace{0.3cm} X_{\rm L}(p) \quad \bullet\!\!-\!\!\!-^{\hspace{-0.25cm}\rm L}\!\!\!-\!\!\circ\quad x(t)\hspace{0.05cm}.$$
The relationship between the Laplace transform $X_{\rm L}(p)$ and the physical spectrum $X(f)$ is often given as follows:
- $$X(f) = X_{\rm L}(p) \Bigg |_{{\hspace{0.1cm} p\hspace{0.05cm}={\rm \hspace{0.05cm} j\hspace{0.05cm}2\pi \it f}}}.$$
- However, if the signal $x(t)$ has periodic components and thus the spectral function $X(f)$ contains Dirac delta functions, then this equation is not applicable.
- In this case, $p = α + {\rm j} · 2πf$ must be applied and then the limit $α → 0$ must be formed.
$\text{Example 1:}$ We assume the unilaterally and exponentially decreasing signal corresponding to $\text{Example 1}$ of chapter »Conclusions from the Allocation Theorem«:
- $$x(t) = \left\{ \begin{array}{c} 0 \\ 0.5 \\ {\rm e}^{-t/T} \end{array} \right.\quad \quad \begin{array}{c} {\rm{for} } \\ {\rm{for} } \\ {\rm{for} } \end{array}\begin{array}{*{20}c}{ t < 0\hspace{0.05cm},} \\ { t = 0\hspace{0.05cm},} \\{ t > 0\hspace{0.05cm}.} \end{array}$$
- Thus, the Laplace transform is:
- $$X_{\rm L}(p) = \int_{0}^{\infty} {\rm e}^{-t/T} \cdot {\rm e}^{-pt} \hspace{0.1cm}{\rm d}t= \frac {1}{p + 1/T} \cdot {{\rm e}^{-(p+1/T) \hspace{0.08cm}\cdot \hspace{0.08cm}t}}\hspace{0.15cm}\Bigg \vert_{t \hspace{0.05cm}=\hspace{0.05cm} 0}^{\infty}= \frac {1}{p + 1/T} \hspace{0.05cm} .$$
- Considering $p = {\rm j} · 2πf$, the conventional spectral function with respect to $f$ is obtained:
- $$X(f) = \frac {1}{{\rm j \cdot 2\pi \it f} + 1/T} = \frac {T}{1+{\rm j \cdot 2\pi \it fT}} \hspace{0.05cm} .$$
- In contrast, if we consider the frequency response of a low-pass filter of first-order whose impulse response $h(t)$ differs from the above time function by the factor $1/T$, then the following holds for the Laplace transform and the Fourier transform, respectively:
- $$H_{\rm L}(p)= \frac {1/T}{p + 1/T}= \frac {1}{1 + p \cdot T} \hspace{0.05cm} , \hspace{0.8cm}H(f) = \frac {1}{1+{\rm j \cdot 2\pi \it fT} } = \frac {1}{1+{\rm j} \cdot f/f_{\rm G} } \hspace{0.05cm} .$$
- In this equation, the »3 dB cut-off frequency« $f_{\rm G} = 1/(2πT)$ $($German: "Grenzfrequenz" ⇒ subscript "G"$)$ is used instead of the parameter $T$.
Some important Laplace correspondences
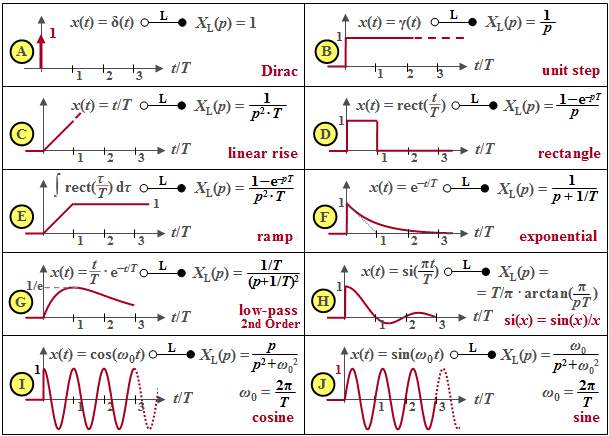
Some important Laplace correspondences are compiled subsequently. All time signals $x(t)$ considered here are assumed to be dimensionless. For this reason, $X_{\rm L}(p)$ then always has the unit »second« as an integral over time.
- The Laplace transform of the »Dirac delta function« $δ(t)$ is $X_{\rm L}(p) = 1$ $($diagram $\rm A)$.
- $X_{\rm L}(p) = 1/p$ is obtained for the »unit jump function« $γ(t)$ $($diagram $\rm B)$ by applying the »integration theorem«.
- From this the Laplace transform of the »linearly increasing function« $x(t) = t/T$ for $t > 0$ is obtained by multiplication by $1/(pT)$ $($diagram $\rm C)$.
- The »rectangular function« can be generated by subtraction of two jump functions $γ(t)$ and $γ(t – T)$ separated by $T$ so that the Laplace transform $X_{\rm L}(p) = (1 – {\rm e}^{–pT})/p$ is obtained according to the »shifting theorem« $($diagram $\rm D)$.
- By integration the »ramp function« or after multiplication by $1/(pT)$ its Laplace transform is obtained from it $($diagram $\rm E)$.
- The »exponential function« $($diagram $\rm F)$ has already been considered in the »last section« . Considering the factor $1/T$, this is at the same time the impulse response of a low-pass filter of first-order.
- The Laplace transform of a second-order low-pass ⇒ function $x(t) = t/T · {\rm e}^{–t/T}$ is obtained by squaring $($diagram $\rm G)$.
- In addition to the causal $\rm sinc$–function $($diagram $\rm H)$, the Laplace transforms of the »causal cosine and sine functions« $($diagrams $\rm I$ and $\rm J)$, which result in $p/(p^2 + ω_0^2)$ resp. $ω_0/(p^2 + ω_0^2)$ are also given in the table.
- Here, $ω_0 = 2πf_0 = 2π/T$ denotes the »angular frequency«.
Pole-zero representation of circuits
Any »linear time-invariant system« $\rm (LTI)$ which can be realized by a circuit of discrete time-constant components such as
- resistances $(R)$,
- capacitances $(C)$,
- inductances $(L)$ and
- amplifier elements,
have a fractional-rational »$p$–transfer function«:
- $$H_{\rm L}(p)= \frac {A_Z \cdot p^Z +\text{...} + A_2 \cdot p^2 + A_1 \cdot p + A_0} {B_N \cdot p^N +\text{...} \ + B_2 \cdot p^2 + B_1 \cdot p + B_0}= \frac {Z(p)}{N(p)} \hspace{0.05cm} .$$
All coefficients of the numerator ⇒ $A_Z, \text{...} \ , A_0$ and of the denominator ⇒ $B_N, \text{...} , B_0$ are real. Furthermore:
- $Z$ denotes the degree of the numerator polynomial $Z(p)$,
- $N$ denotes the degree of the denominator polynomial $N(p)$.
$\text{Equivalent pole-zero representation:}$ The following can be formulated for the »$p$–transfer function«, too:
- $$H_{\rm L}(p)= K \cdot \frac {\prod\limits_{i=1}^Z p - p_{\rm o i} } {\prod\limits_{i=1}^N p - p_{\rm x i} }= K \cdot \frac {(p - p_{\rm o 1})(p - p_{\rm o 2})\cdot \text{...} \ \cdot (p - p_{ {\rm o} \hspace{-0.03cm} Z})} {(p - p_{\rm x 1})(p - p_{\rm x 2})\cdot \text{...} \cdot (p - p_{ {\rm x} \hspace{-0.03cm} N})} \hspace{0.05cm} .$$
The $Z + N + 1$ parameters signify:
- $K = A_Z/B_N$ is a constant factor. If $Z = N$, this is dimensionless.
- The solutions of the equation $Z(p) = 0$ result in the $Z$ »zeros« $p_{\rm o1},\text{...} \ , p_{\rm oZ}$ of $H_{\rm L}(p)$.
- The zeros of the denominator polynomial $N(p)$ yield the $N$ pole locations $($or »poles« for short$)$.
The transformation is unique. This can be seen from the fact that the $p$–transfer function is also determined only by $Z + N + 1$ free parameters according to the first equation since one of the coefficients $A_Z, \text{...} \ , A_0, B_N, \text{...} \ , B_0$ can be normalized to $1$ without changing the quotient.
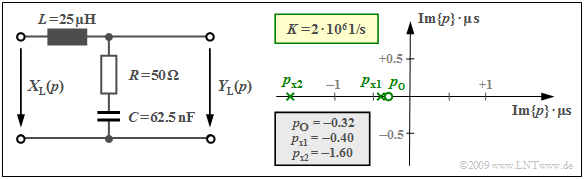
$\text{Example 2:}$ We consider the drawn two-port network with
- an inductance $L$ $($complex resistance $pL)$ in the longitudinal branch as well as
- the series connection of an ohmic resistance $R$ and a capacitance $C$ with the complex resistance $1/(pC)$ in the transverse branch.
Thus, the $p$–transfer function is:
- $$H_{\rm L}(p)= \frac {Y_{\rm L}(p)} {X_{\rm L}(p)}= \frac {R + {1}/{(pC)} } {pL + R +{1}/{(pC)} }$$
- $$\Rightarrow \hspace{0.3cm} H_{\rm L}(p)= \frac {1 + p \cdot{RC} } {1 + p \cdot{RC}+ p^2 \cdot{LC} } \hspace{0.05cm} .$$
If $p = {\rm j} · 2πf$ is set, then the Fourier transfer function $($or the »frequency response«$)$ is obtained. If the numerator and denominator in the above equation are divided by $LC$, then the following is obtained:
- $$H_{\rm L}(p)= \frac {R} {L}\cdot \frac {p + {1}/{(RC)} } {p^2 + {R}/ {L}\cdot p + {1}/{(LC)} }= K \cdot \frac {p - p_{\rm o } } {(p - p_{\rm x 1})(p - p_{\rm x 2})} \hspace{0.05cm} .$$
⇒ In the right-hand side of the equation, the transfer function $H_{\rm L}(p)$ is given in »pole-zero notation«.
By comparison of coefficients the following values are obtained for $R = 50 \ \rm Ω$, $L = 25\ \rm µ H$ and $C = 62.5 \ \rm nF$ :
- the constant $K = R/L = 2 · 10^6 \cdot 1/{\rm s}$,
- the zero $p_{\rm o} = -1/(RC) = -0.32 · 10^6 \cdot 1/{\rm s},$
- the two poles $p_{\rm x1}$ and $p_{\rm x2}$ as the solution of the equation
- $$p^2 + \frac {R} {L}\cdot p + \frac{1}{LC} = 0 \hspace{0.3cm}\Rightarrow \hspace{0.3cm} p_{\rm x 1,\hspace{0.05cm}2 }= -\frac {R} {2L}\pm \sqrt{\frac {R^2} {4L^2}- \frac{1}{LC} }$$
- $$\Rightarrow \hspace{0.3cm} p_{\rm x 1,\hspace{0.05cm}2 }= -10^6 \cdot {1}/{\rm s} \pm \sqrt{10^{12} \cdot {1} /{\rm s^2}-0.64 \cdot 10^{12} \cdot {1}/ {\rm s^2} }\hspace{0.6cm} \Rightarrow \hspace{0.3cm} p_{\rm x 1 }= -0.4 \cdot 10^6\cdot {1}/ {\rm s},\hspace{0.2cm}p_{\rm x 2 }= -1.6 \cdot 10^6\cdot {1}/ {\rm s} \hspace{0.05cm} .$$
In the above graph, the pole-zero diagram is given on the right-hand side.
- The two axes denote the real and imaginary parts of the variable $p$, each normalized to the value $10^6 · \rm 1/s\; (= 1/µs)$.
- The zero at $p_{\rm o} =\, –0.32$ can be seen as a circle and the poles at $p_{\rm x1} = \,–0.4$ and $p_{\rm x2} = \,–1.6$ as crosses.
Properties of poles and zeros
The transfer function $H_{\rm L}(p)$ of any realizable circuit is fully described by $Z$ »zeros« and $N$ »poles« together with a constant $K$ where the following restrictions apply:
- $Z ≤ N$ always holds. The $p$–transfer function would also be »infinitely large« with $Z > N$ in the limiting case for $p → ∞$ $($i.e. for very high frequencies$)$.
- The zeros $p_{\rm oi}$ and the poles $p_{ {\rm x}i}$ are generally complex, and have like $p$ the unit $\rm 1/s$. If $Z < N$ holds, then the constant $K$ has also a unit.
- The poles and zeros can be real as shown in the last example. If they are complex, then two poles resp. two zeros always occur as complex conjugates, since $H_{\rm L}(p)$ always represents a real fractional-rational function.
- All poles lie in the left half-plane or on the imaginary axis $($limiting case$)$. This property follows from the required and assumed causality together with the »fundamental function theory«, which will be stated in the next chapter.
- Zeros can occur in both the left and right $p$–half-planes or also on the imaginary axis. An example of zeros in the right half-plane can be found in $\text{Exercise 3.4Z}$, which deals with all-pass filters.
- In so-called »minimum-phase systems«, not only poles are forbidden in the right $p$–half-plane but also zeros. The real part of all singularities here is never positive.
These properties are now illustrated by three examples.
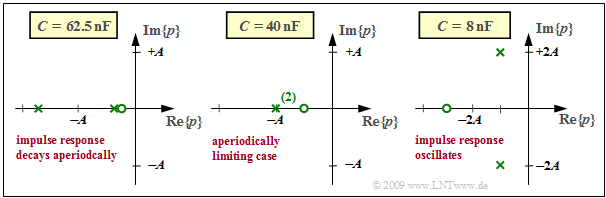
$\text{Example 3:}$ Starting from the »two-port network« $(L$ in the longitudinal branch, $R$ and $C$ in the transverse branch$)$ the characteristic quantities of the transfer function can be given as follows:
- $$K = 2A, \hspace{0.2cm}p_{\rm x 1,\hspace{0.05cm}2 }= -A \pm \sqrt{A^2-B^2},$$
- $$p_{\rm o }= - \frac{B^2}{2A} \hspace{0.05cm} \hspace{0.2cm} {\rm with } \hspace{0.2cm} A = \frac {R} {2L}, \hspace{0.2cm}B = \frac{1}{\sqrt{LC} } \hspace{0.05cm}.$$
The graph shows three different diagrams with different capacitance values $C$.
- $R = 50 \ \rm Ω$ and $L = 25 \ \rm µ H$ always hold.
- The axes are normalized to $A = R/(2L) = 10^6 · \rm 1/s$.
- The constant factor is $K = 2A = 2 · 10^6 · \rm 1/s.$
$\text{Example 3.1:}$
For $B < A$, »two real poles« and »one zero« to the right of $-A/2$ are obtained. The following arises as a result for $C = 62.5 \ \rm nF$ ⇒ $ {B}/ {A}= 0.8 $ $($left diagram$)$:
- $$ p_{\rm x 1}/A = -0.4 , \hspace{0.2cm}p_{\rm x 2}/A= -1.6 , \hspace{0.2cm}p_{\rm o}/A= -0.32 \hspace{0.05cm} .$$
$\text{Example 3.2:}$
For $B > A$, »two conjugate-complex poles« and »one zero« to the left of $-A/2$ are obtained. For $C = 8 \ \rm nF$ ⇒ $ {B}/ {A}= \sqrt{5} $ $($right diagram$)$:
- $$p_{\rm x 1,\hspace{0.05cm}2 }/A= -1\pm {\rm j}\cdot 2,\hspace{0.2cm}p_{\rm o}/A\approx -2.5 \hspace{0.05cm} .$$
$\text{Example 3.3:}$
The case $A = B$ leads to »a real double pole« and »one zero« at $– A/2$. For $C = 400 \ \rm nF$ ⇒ $ {B}/ {A}= 1 $ $($middle diagram$)$:
- $$ p_{\rm x 1}/A= p_{\rm x 2}/A= -1, \hspace{0.2cm}p_{\rm o}/A= -0.5 \hspace{0.05cm} .$$
The impulse responses $h(t)$ are obtained according to the following chapter »Inverse Laplace Transform« as follows:
- For the left constellation, $h(t)$ is »aperiodically decaying«.
- For the right constellation, $h(t)$ is »attenuated-oscillatory«.
- The middle constellation is called the »critically attenuated case«.
Graphical determination of attenuation and phase
The $p$–transfer function is given in pole-zero notation:
- $$H_{\rm L}(p)= K \cdot \frac {\prod\limits_{i=1}^Z (p - p_{\rm o i})} {\prod\limits_{i=1}^N (p - p_{\rm x i})}= K \cdot \frac {(p - p_{\rm o 1})(p - p_{\rm o 2})\cdot \text{...} \cdot (p - p_{ {\rm o} \hspace{-0.03cm} Z})} {(p - p_{\rm x 1})(p - p_{\rm x 2})\cdot \text{...} \cdot (p - p_{ {\rm x} \hspace{-0.03cm} N})} \hspace{0.05cm} .$$
- The conventional frequency response $H(f)$ is obtained by replacing the argument $p$ of $H_{\rm L}(p)$ by ${\rm j} \cdot 2πf$ :
- $$H(f)= K \cdot \frac {({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm o 1})({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm o 2})\cdot \text{...} \cdot ({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm o} \hspace{-0.03cm} Z})} {({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm x 1})({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{\rm x 2})\cdot \text{...}\cdot ({\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm x} \hspace{-0.03cm} N})} \hspace{0.05cm} .$$
- We now consider a fixed frequency $f$ and describe the distances and angles of all »zeros« $($circles$)$ by vectors:
- $$R_{ {\rm o} i} = {\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm o} i}= |R_{{\rm o} i}| \cdot {\rm e}^{\hspace{0.03cm}{\rm j}\hspace{0.03cm}\cdot\hspace{0.03cm}\phi_{ {\rm o} i} }, \hspace{0.3cm}i= 1, \text{...}\ , Z \hspace{0.05cm} .$$
- We proceed in the same way for the »poles« $($crosses$)$:
- $$R_{ {\rm x} i} = {\rm j} \cdot 2\pi \hspace{-0.05cm}f - p_{ {\rm x} i}= |R_{ {\rm x} i}| \cdot {\rm e}^{\hspace{0.03cm}{\rm j}\hspace{0.03cm}\cdot\hspace{0.03cm}\phi_{ {\rm x} i} }, \hspace{0.3cm}i= 1, \text{...}\ , N \hspace{0.05cm} .$$
- The graph shows the magnitudes and phase angles for a system with
- $Z = 2$ zeros (circles) in the right half-plane
- $N = 2$ poles (crosses) in the left half-plane.
- constant $K$.
- The following can be written for the frequency response with this vector representation:
- $$H(f)= K \cdot \frac {|R_{ {\rm o} 1}| \cdot |R_{ {\rm o} 2}|\cdot ... \cdot |R_{ {\rm o} \hspace{-0.03cm} Z}|} {|R_{ {\rm x} 1}| \cdot |R_{ {\rm x} 2}|\cdot \text{...} \cdot |R_{ {\rm x} \hspace{-0.03cm} N}|} \cdot {\rm e^{\hspace{0.03cm}{\rm j} \hspace{0.05cm}\cdot [ \phi_{ {\rm o} 1}\hspace{0.1cm}+ \hspace{0.1cm}\phi_{ {\rm o} 2} \hspace{0.1cm}+ \hspace{0.1cm}\hspace{0.1cm}\text{...}. \hspace{0.1cm} + \hspace{0.1cm}\phi_{ {\rm o} \hspace{-0.03cm}{\it Z}}\hspace{0.1cm}- \hspace{0.1cm}\phi_{ {\rm x} 1}\hspace{0.1cm}- \hspace{0.1cm}\phi_{ {\rm x} 2} \hspace{0.1cm}- \hspace{0.1cm}... \hspace{0.1cm} - \hspace{0.1cm} \phi_{ {\rm x} \hspace{-0.03cm}{\it N} }]} } \hspace{0.05cm} .$$
If $H(f)$ is expressed by the attenuation function $a(f)$ and the phase function $b(f)$ according to the generally valid relation $H(f) = {\rm e}^{-a(f)\hspace{0.05cm}- \hspace{0.05cm}{\rm j} \hspace{0.05cm}\cdot \hspace{0.05cm}b(f)}$, then the following result is obtained by comparing with the above equation:
- With a suitable normalization of all dimensional quantities the following holds for the »attenuation function« in Neper $(1 \ \rm Np$ corresponds to $8.686 \ \rm dB)$:
- $$a(f) = -{\rm ln} \hspace{0.1cm} K + \sum \limits_{i=1}^N {\rm ln} \hspace{0.1cm} |R_{ {\rm x} i}|- \sum \limits_{i=1}^Z {\rm ln} \hspace{0.1cm} |R_{ {\rm o} i}| \hspace{0.05cm} .$$
- The »phase function« in radian $\rm (rad)$ arises as a result according to the upper sketch:
- $$b(f) = \phi_K + \sum \limits_{i=1}^N \phi_{ {\rm x} i}- \sum \limits_{i=1}^Z \phi_{ {\rm o} i}\hspace{0.2cm}{\rm with} \hspace{0.2cm} \phi_K = \left\{ \begin{array}{c} 0 \\ \pi \end{array} \right. \begin{array}{c} {\rm{for} } \\ {\rm{for} } \end{array}\begin{array}{*{20}c} { K > 0\hspace{0.05cm},} \\ { K <0\hspace{0.05cm}.} \end{array}$$
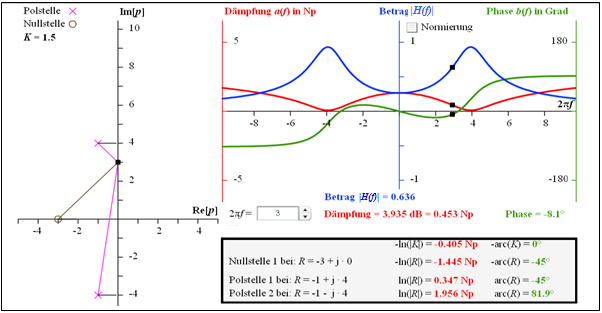
$\text{Example 4:}$ The graphic illustrates the calculation of
- the attenuation function $a(f)$ ⇒ red curve, and
- the phase function $b(f)$ ⇒ green curve
of a two-port network which is defined by
- the factor $K = 1.5$,
- one zero at $-3$ and
- two poles at $–1 \pm {\rm j} · 4$.
The given numerical values are valid for the frequency $2πf = 3$:
- $$a \big [f = {3}/({2\pi}) \big ] = 0.453\,\,{\rm Np}= 3.953\,\,{\rm dB}$$
- $$\Rightarrow \hspace{0.4cm}\big \vert H \big [f = {3}/({2\pi}) \big ]\big \vert = 0.636,$$
- $$ b\big [f = {3}/({2\pi}) \big ] = -8.1^\circ \hspace{0.05cm} .$$
The derivation of these numerical values is illustrated in the framed block. For the magnitude frequency response $\vert H(f)\vert$ ⇒ blue curve, a band-pass-like curve shape is obtained with
- $$\vert H(f = 0)\vert \approx 0.25\hspace{0.05cm},$$
- $$\vert H(f = {3}/(2\pi)\vert \approx 0636\hspace{0.05cm},$$
- $$\vert H(f \rightarrow \infty)\vert= 0 \hspace{0.05cm}.$$
Exercises for the chapter
Exercise 3.2: Laplace Transform
Exercise 3.2Z: Laplace and Fourier
Exercise 3.3: p-Transfer Function
Exercise 3.3Z: High- and Low-Pass Filters in p-Form
Exercise 3.4: Attenuation and Phase Response
Exercise 3.4Z: Various All-Pass Filters